Operation Manual HORIBA APDA-371
Particulate Monitor
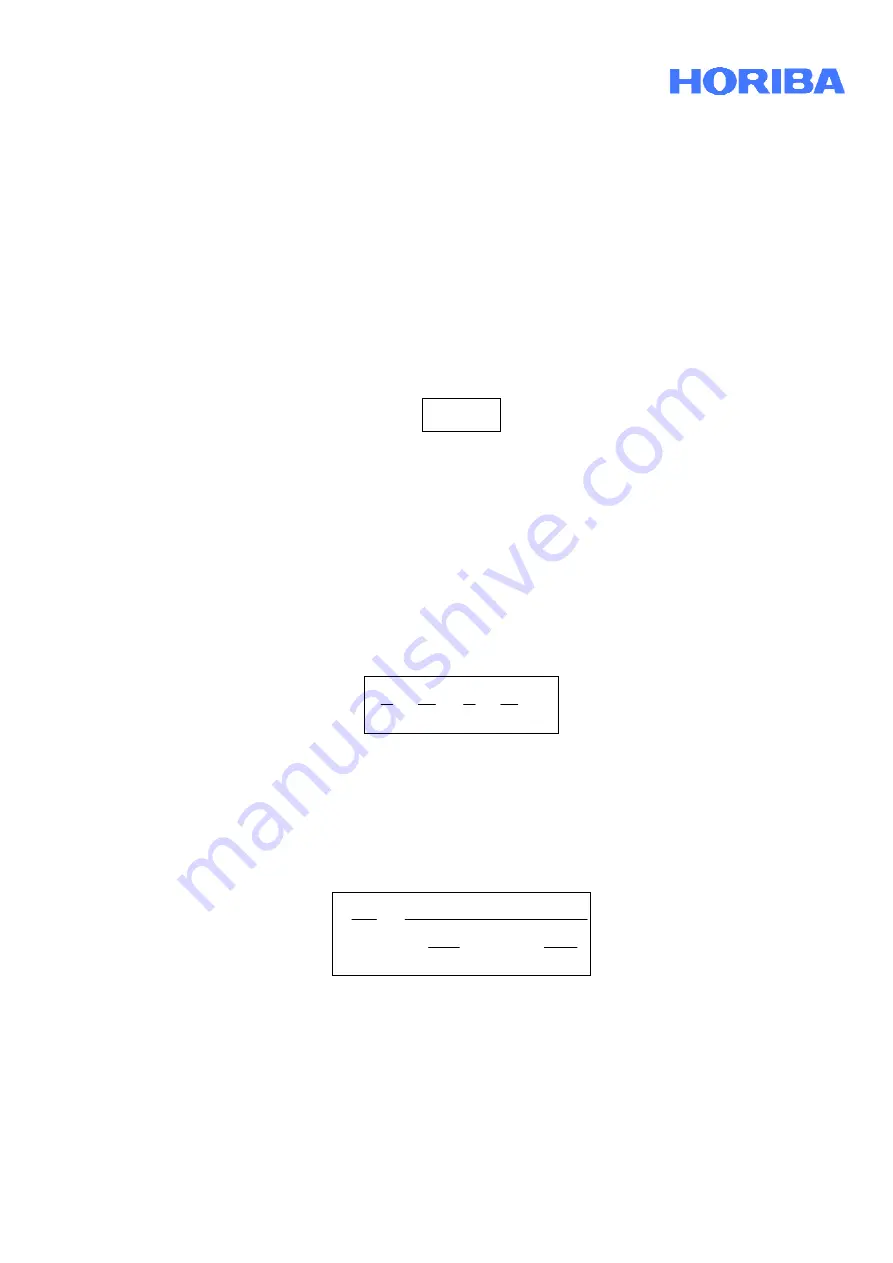
Date:
April, 2010
______________________________________________________________________________________________________________________
______________________________________________________________________________________________________________________
HORIBA Europe GmbH, Julius-Kronenberg-Str. 9, D-42799 Leichlingen,
: +49(0)2175-8978-0,
: +49(0)2175-8978-50
Page 4
11 THEORY OF OPERATION and MATHEMATICAL ANALYSIS
When the high-energy electrons emanating from the radioactive decay of
14
C (carbon-14) interact with nearby matter
they loose their energy and, in some cases, are absorbed by the matter. These high-energy electrons emitted through
radioactive decay are known as beta rays and the process is known as beta-ray attenuation. When matter is placed
between the radioactive
14
C source and a device designed to detect beta rays, the beta rays are absorbed and/or their
energy diminished. This results in a reduction in the number of beta particles detected. The magnitude of the reduction
in detected beta particles is a function of the mass of the absorbing matter between the
14
C beta source and the
detector.
The number of beta particles passing through absorbing matter, such as dust deposited on a filter tape, decrease nearly
exponentially with the mass through which they much pass. Equation 1 shows this relationship.
Equation 1
x
e
I
I
µ
−
=
0
In Equation 1, I is the measured beta ray intensity (counts per unit time), of the attenuated beta ray (dust laden filter
tape), I
0
is the measured beta ray intensity of the un-attenuated beta ray (clean filter tape),
µ
is the absorption cross
section of the material absorbing the beta rays (cm
2
/g), and x is the mass density of the absorbing matter (g/cm
2
).
Equation 1 very closely resembles the Lambert-Beers Law, which is used in spectrometric analysis. Just as the
Lambert-Beers Law is an idealization of what is actually observed, Equation 1 is also an idealized simplification of the
true processes occurring meant to simplify the corresponding mathematics. However, experimental measurement
shows that in properly designed monitors, such as the APDA-371, the use of this equation introduces no substantial
error.
Equation 1 may be rearranged to solve for x, the mass density of the absorbing matter. This is shown in Equation 2.
Equation 2
x
=
=
−
I
I
ln
µ
1
I
I
ln
µ
1
0
0
In practice, the absorption cross section is experimentally determined during the calibration process. Once I and I
0
are
experimentally measured, it is a simple matter to calculate x, the predicted mass density.
In practice, ambient air is sampled at a constant flow rate (Q) for a specified time
∆
t. This sampled air is passed through
a filter of surface area A. Once x, the mass density of collected particles, has been determined, it is possible to
calculate the ambient concentration of particulate matter (
µ
g/m
3
) with Equation 3.
Equation 3
=
g
cm
∆
t(min)
µ
min
liter
Q
)
A(cm
10
m
µ
g
c
2
2
6
3
In Equation 3, c is the ambient particulate concentration (
µ
g/m
3
), A is the cross sectional area on the tape over which
dust is being deposited (cm
2
), Q is the rate at which particulate matter is being collected on the filter tape (liters/
minute), and
∆
t is the sampling time (minutes). Combining these equations yields to the final expression for the ambient
particulate concentration in terms of measured quantities. This is shown in Equation 4.




















