FXAlg #911: Mono LaserVerb ¥ FXAlg #912: LaserVerb Lite ¥ FXAlg #913: LaserVerb
Algorithm Reference-136
FXAlg #911: Mono LaserVerb ¥
FXAlg #912: LaserVerb Lite ¥
FXAlg #913: LaserVerb
A bizarre reverb with a falling buzz
Allocation Units:
1 for Mono LaserVerb; 2 for LaserVerb Lite; 3 for LaserVerb
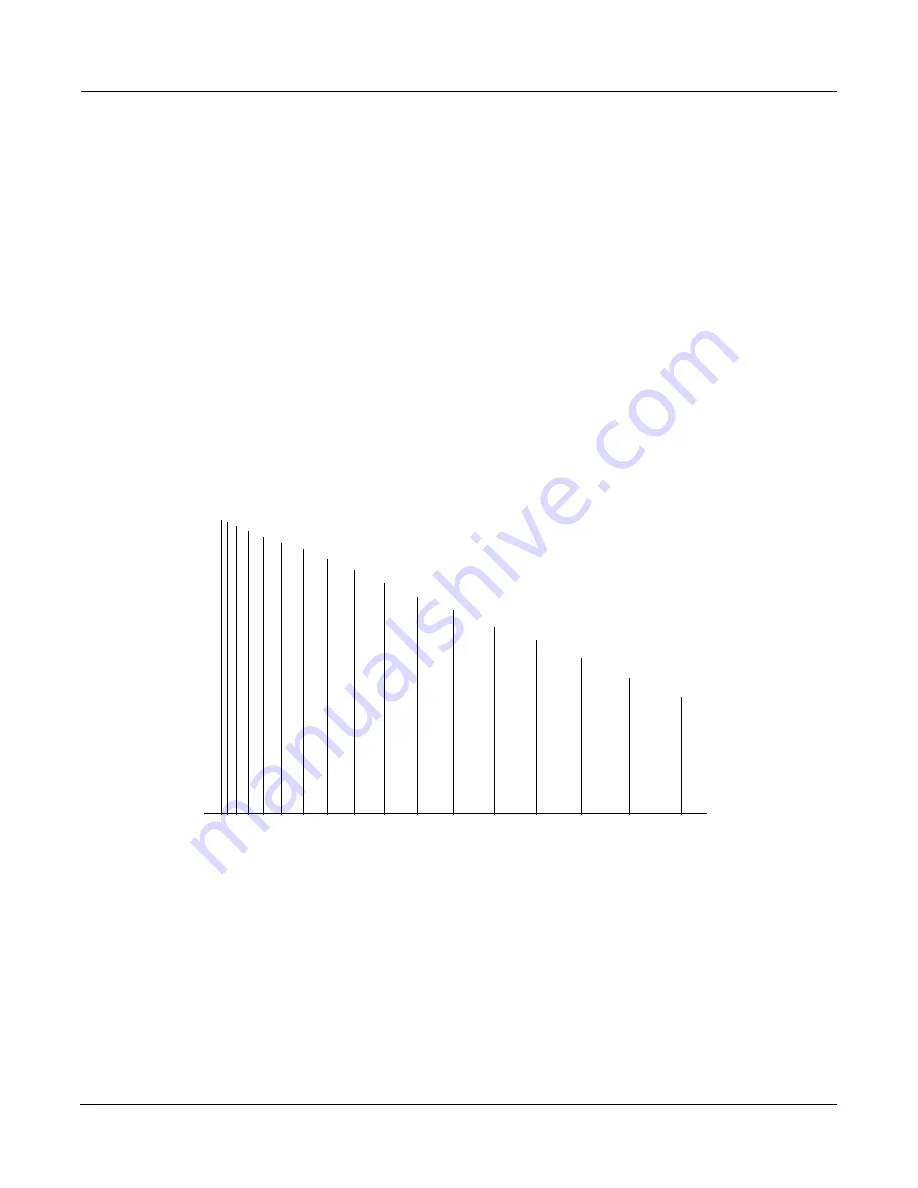
LaserVerb is a new kind of reverb sound that has to be heard to be believed! When it is fed an impulsive sound such
as a snare drum, LaserVerb plays the impulse back as a delayed train of closely spaced impulses, and as time passes,
the spacing between the impulses gets wider. The close spacing of the impulses produces a discernible buzzy pitch
which gets lower as the impulse spacing increases. The following figure is a simplified representation of the
LaserVerb impulse response. (An impulse response of a system is what you would see if you had an oscilloscope
on the system output and you gave the system an impulse or a spike for an input.)
Simplified Impulse Response of LaserVerb
With appropriate parameter settings this effect produces a descending buzz or whine somewhat like a diving
airplane or a siren being turned off. The descending buzz is most prominent when given an impulsive input such
as a drum hit. When used as a reverb, it tends to be highly metallic and has high pitched tones at certain parameter
settings. To get the descending buzz, start with about half a second of delay, set the Contour parameter to a high
value (near 1), and set the HF Damping to a low value (at or near 0). The Contour parameter controls the overall
shape of the LaserVerb impulse response. At high values the response builds up very quickly and decays slowly.
As the Contour value is reduced, the decay becomes shorter and the sound takes longer to build up. At a setting of
zero, the response degenerates to a simple delay.
The Spacing parameter controls the initial separation of impulses in the impulse response and the rate of their
subsequent separation. Low values result in a high initial pitch (impulses are more closely spaced) and takes longer
for the pitch to go down.
t = 0
time
















































