2 LOCK-IN BASICS
2.1 L
OCK
-I
N
A
MPLIFICATION
The lock-in amplifier is a measuring instrument for extracting and amplifying low signals
from noise by means of a correlation analysis. For this method, it is necessary to know
about the time dependence of the signal to be measured. Practically this signal is
modulated at a certain reference frequency
ω
ref
and the resulting frequency spectrum is
simultaneously analyzed at
ω
ref
. For analyzing the input signal the lock-in amplifier
transforms the time-dependent signal at
ω
ref
into the frequency domain and averages it
during the time
τ
.
In other words, the lock-in amplifier can be described as a narrow-
band amplifier with the center frequency
ω
ref
and a bandwidth that is determined by the
time constant
τ
.
A schematic diagram with the basic components of the lock-in amplifier is shown in
Figure 1 on page 5. First of all the received signal is amplified and digitized. Divided into
two separate channels, the signal is multiplied by the mentioned reference signal (with
frequency
ω
ref
) and the 90° phase-shifted reference signal respectively. The reference
signal is generated with the lock-in's digital oscillator. An additional implemented phase
displacement
Ψ
enables to compensate phase differences
caused by the measuring equipment. After multiplication, the
resulting signals are low-pass filtered and provide now
information about the real part
X
and the imaginary part
Y
of
the analyzed signal relating to the phase position of the
reference signal. Out of them the amplitude
R
as well as the
relative phase shift
φ
are calculated.
2.2 M
ATHEMATICAL
D
ESCRIPTION
In this chapter, a short mathematical description of the multiplication and integration
steps as the key procedures of a digital lock-in amplifier is given.
For instance, one tries to analyze the noisy signal
s
(
t
) that depends on a value
v
(
t
).
v
(
t
)
itself may consist of a constant part
v
0
and an additional periodic time-dependent part
with the amplitude
v
1
and the frequency
ω
ref
:
v
t
=
v
0
v
1
cos
ref
t
. The latter
term modulates the analyzed signal amplitude
s
v
t
.
Then,
s
v
t
can be developed into a Taylor series:
s
v
0
v
1
cos
ref
t
=
∑
k
=
0
∞
s
k
v
0
⋅
v
1
k
k
!
⋅
cos
k
ref
t
(1a)
with the derivatives
s
k
v
0
=
d
k
s
d
v
k
v
=
v
0
k
∈ ℕ
.
(1b)
Inside the lock-in amplifier
s
v
t
is multiplied by the reference signal with frequency
ω
ref
and integrated over the time τ. That means, in order to determine the value of s(t)
at the time t, all measured values in the past time interval
τ
have to be taken into
account. Therefore τ should be a multiple of
2
π/
ω
ref
or bigger because it should contain
at least one period of oscillation to receive correct results.
Beside the modulation at
ω
ref
, modulations of s(t) at the frequencies m
·
ω
ref
with m = 1, 2,
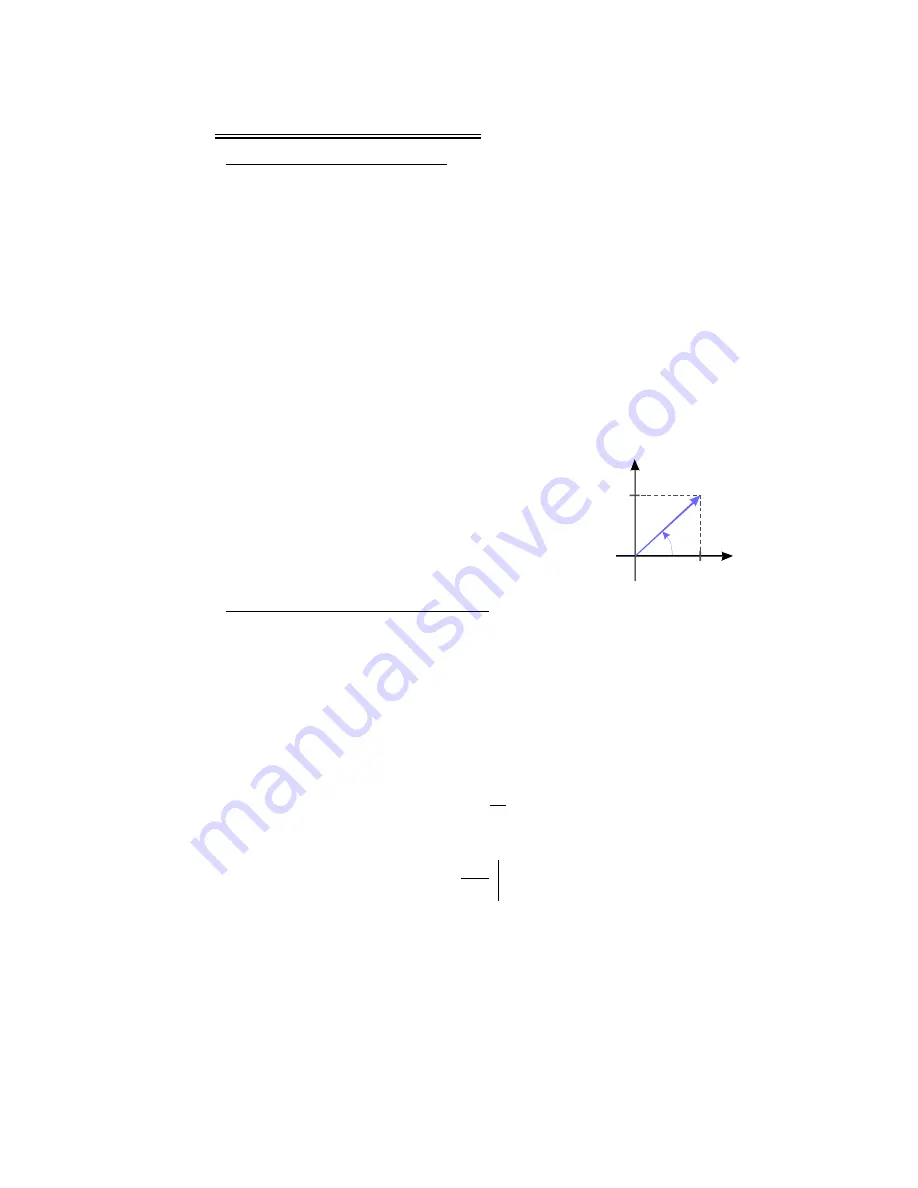
10 (58)
R
X
Y
Summary of Contents for eLockIn 203
Page 52: ...7 APPENDICES 7 1 SCHEMATIC DIAGRAM OF THE SIGNAL PATHS 52 58...
Page 53: ...7 2 DIAGRAM OF THE LOCKIN AMPLIFICATION PATHS Diagram of the preamplifier switches 53 58...
Page 54: ...7 3 FRONTPANEL DIMENSIONS 54 58...
Page 55: ...7 4 INSTRUMENT SPECIFIC TEST SHEETS insert with 10 pages of data 55 58...
Page 56: ...7 5 PREAMPLIFIER CONNECTOR PINOUT 56 58...
Page 58: ...7 7 TRACEABILITY CHART 58 58...











































