9-19
IM 01F06A00-01E
9. GENERAL DESCRIPTION
■
Reference
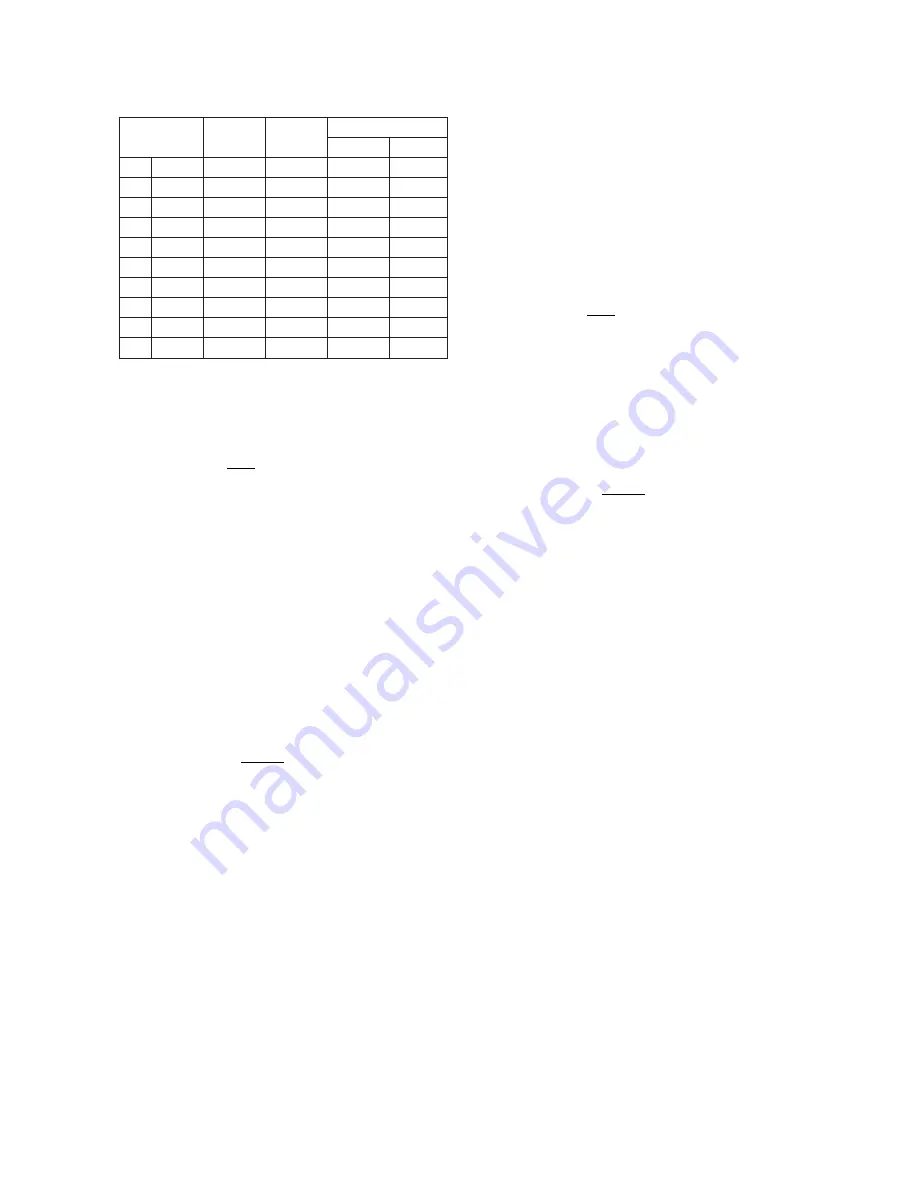
Table 9.5.7 Inner Diameter and Nominal value
Model Code
Inner
Diameter
mm
Nominal
K-Factor
Pulse/L
Nominal Pulse Rate
Hz/m/s Hz/m
3
/h
DY015
DY025
DY040
DY050
DY080
DY100
DY150
DY200
DY250
DY300
DY025-/R1
DY040-/R1
DY050-/R1
DY080-/R1
DY100-/R1
DY150-/R1
DY200-/R1
—
—
—
14.6
25.7
39.7
51.1
71.0
93.8
138.8
185.6
230.8
276.2
376
65.6
18.7
8.95
3.33
1.43
0.441
0.185
0.0966
0.0563
62.7
35.5
23.1
18.3
13.2
9.88
6.67
5.00
4.04
3.37
104
19.1
5.19
2.49
0.925
0.397
0.123
0.0514
0.0268
0.0156
DYF Tab-14
■
Pressure Loss
Calculation of pressure loss for general type
obtained from the following equations.
∆
P = 108
×
10
-5
·
ρ
f
·
υ
2
········· (1)
or
∆
P = 135
×
ρ
f
· Q
f2
··············· (2)
D
4
where,
∆
P : Pressure loss (kPa )
ρ
f
: Density at operating condition (kg/m
3
)
υ
: Flow velocity (m/s)
Qf : Actual flow rate (m
3
/h)
D
: Internal Diameter of detector (mm)
(Example)
DY050, hot water: 80
°
C, flowrate: 30 m
3
/h
1. Since the density of water at 80
°
C is 972 kg/m
3
,
substitute this value in equation (2):
∆
P = 135
×
972
×
30
2
/ 51.1
4
= 17.3 kPa
2. Obtain the pressure loss using equation (1). The
flow velocity when the flow rate is 30 m
3
/h is given
by:
υ
= 354
×
Q
f
/D
2
=
354
×
30
= 4.07 m/s
51.1
2
Therefore, substitute this value in equation (1):
∆
P = 108
×
10
-5
×
972
×
4.07
2
= 17.3 kPa
Calculation of pressure loss for reduced bore type
(Option code: /R1)
obtained from the following equations.
∆
P = 124
×
10
-5
×
ρ
f
×
υ
2
········· (3)
or
∆
P = 155
×
ρ
f
×
Qf
2
/ D
4
········
(4)
(Example)
DY040-/R1, hot water: 50 deg C flowrate: 10 m
3
/h
1. Since the density of water at 50 deg C is
992 kg/cm
3
, substitute this value in equation (4):
∆
P = 155
×
992
×
10
2
/ 25.7
4
= 35.3 kPa
2. Obtain by using equation (3). The flow velocity
when the flow rate is 10 m3/h is given by:
υ
= 354
×
Qf
×
/D
2
= 354
×
10
×
25.7
2
= 5.4 m/s
Therefore, substitute this value in equation (3):
∆
P = 124
×
10
-5
×
992
×
5.4
2
= 35.3 kPa
Calculation of pressure loss for reduced bore type
(Option code: /R2)
obtained from the following equations.
∆
P = 138
×
10
-5
·
ρ
f
·
υ
2
········· (5)
or
∆
P = 173
×
ρ
f
· Q
f2
··············· (6)
D
4
(Example)
DY050-/R2, hot water: 50 deg C, flowrate: 15 m
3
/h
1. Since the density of water at 50 deg C is 992 kg/
cm
3
, substitute this value in equation (6):
∆
P = 173
×
992
×
15
2
/ 25.7
4
= 88.5 kPa
2. Obtain by using equation (5). The flow velocity
when the flow rate is 20m
3
/h is given by:
υ
= 354
×
Q
f
/D
2
=
354
×
15
= 8.0 m/s
25.7
2
Therefore, substitute this value in equation (5):
∆
P = 138
×
10
-5
×
992
×
8.0
2
=
88.5 kPa
■
Cavitation
(Minimum Back Pressure, Liquid service only):
Cavitation occurs when the flow line pressure is low
and flow velocity is high during fluid measurement,
preventing correct measurement of flow rate. The
optimum line pressure can be obtained from the
following equation.
P = 2.7 ·
∆
P + 1.3 · Po
··············· (5)
Where,
P
: Line pressure, 2 to 7 times as large as internal
diameter on downstream of flowmeter body
surface. (kPa absolute).
∆
P : Pressure loss (kPa).
Refer to the item above.
Po : Saturation liquid vapor pressure at operating
temperature (kPa absolute).
(Example) Confirmation of presence of cavitation
Suppose that the line pressure is 120 kPa abs and the
flow rate scale is 0 to 30 m
3
/h. It is only necessary to
confirm the pressure at the maximum flow rate ;
therefore, the saturated steam pressure of water at 80
°
C
is as follows from the table of saturated steam
pressures:
Po = 47.4 kPa abs
Therefore, substitute this value in equation (5):
P = 2.7
17.3 + 1.3
47.4
= 108.3 kPa abs
Since the operating pressure of 120 kPa abs is higher
than 108.3 kPa abs, no cavitation occurs.
















































