HP 50G, Руководство пользователя

Страница: 507 / 887

Page 16-30
The fitting is somewhat acceptable for 0<t<2, although not as good as in the
previous example.
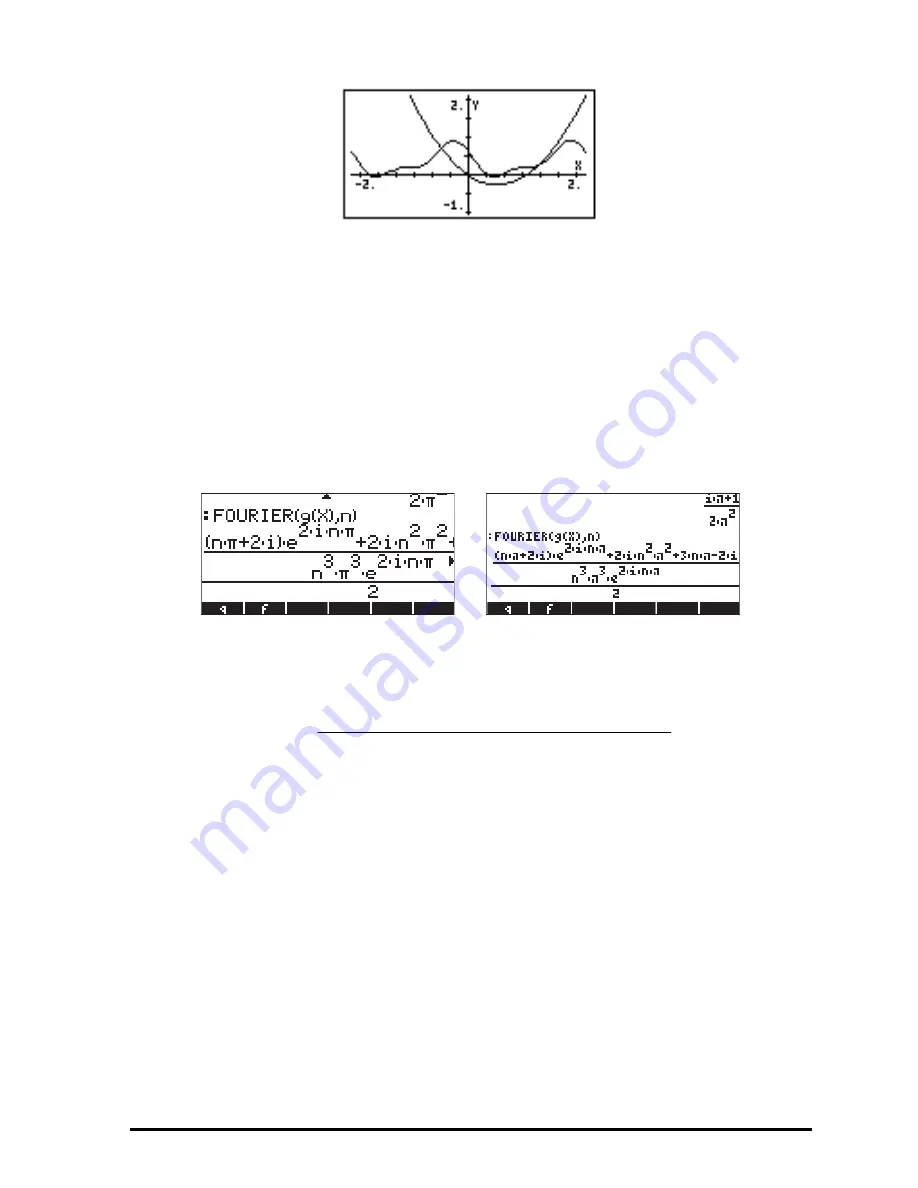
A general expression for c
n
The function FOURIER can provide a general expression for the coefficient c
n
of
the complex Fourier series expansion. For example, using the same function g(t)
as before, the general term c
n
is given by (figures show normal font and small
font displays):
The general expression turns out to be, after simplifying the previous result,
We can simplify this expression even further by using Euler’s formula for
complex numbers, namely, e
2in
π
= cos(2n
π
) + i
⋅
sin(2n
π
) = 1 + i
⋅
0 = 1, since
cos(2n
π
) = 1, and sin(2n
π
) = 0, for n integer.
Using the calculator you can simplify the expression in the equation writer
(
‚O
) by replacing e
2in
π
= 1. The figure shows the expression after
simplification:
π
π
π
π π π
in
in
n
e n
i n n i e i n
c
2 3 3
2 2 2 2
2
2 3 2 ) 2 (
⋅
− + + ⋅ +
=