HP 48GII, Руководство пользователя, Страница: 509 / 864

Page 16-31
A general expression for c
n
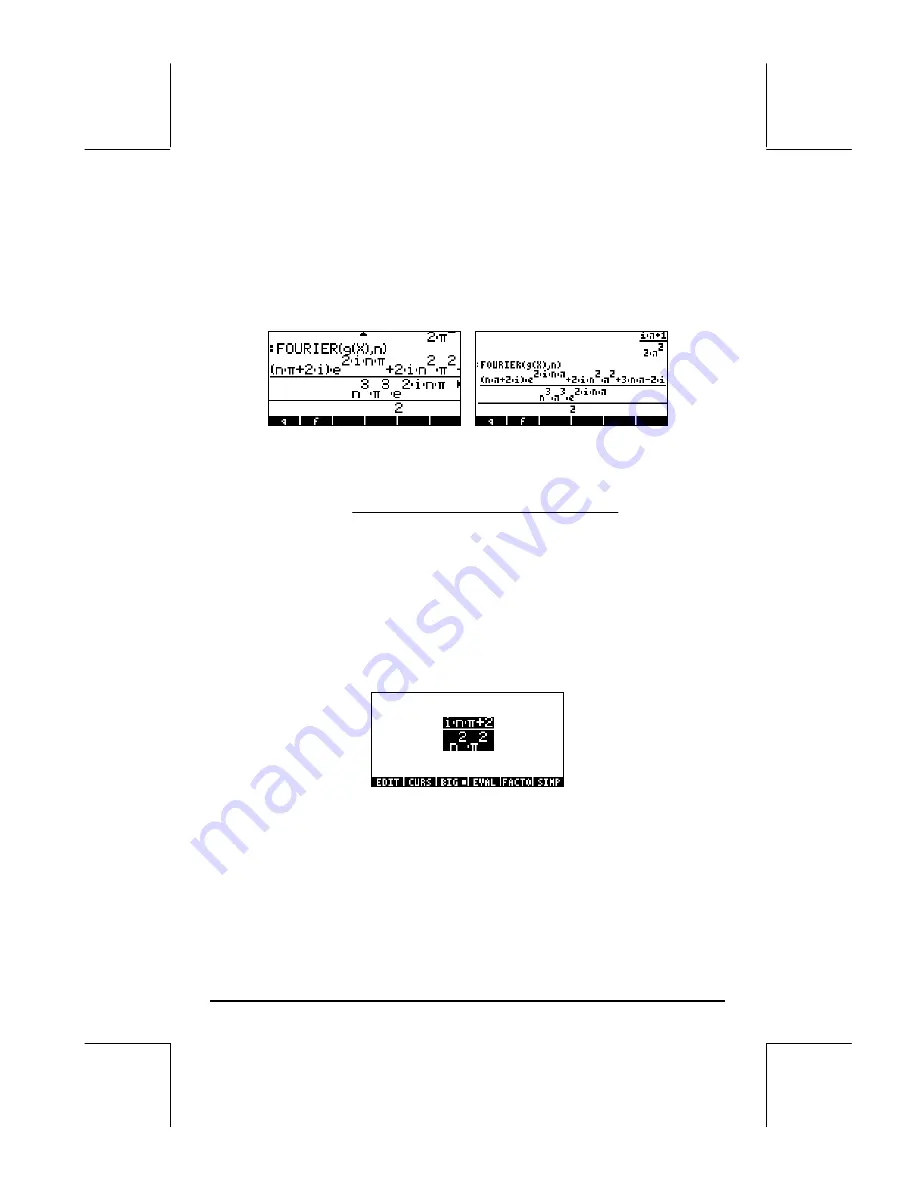
The function FOURIER can provide a general expression for the coefficient c
n
of the complex Fourier series expansion. For example, using the same
function g(t) as before, the general term c
n
is given by (figures show normal
font and small font displays):
The general expression turns out to be, after simplifying the previous result,
π
π
π
π π π
in
in
n
e n
i n n i e i n
c
2 3 3
2 2 2 2
2
2 3 2 ) 2 (
⋅
− + + ⋅ +
=
We can simplify this expression even further by using Euler’s formula for
complex numbers, namely, e
2in
π
= cos(2n
π
) + i
⋅
sin(2n
π
) = 1 + i
⋅
0 = 1, since
cos(2n
π
) = 1, and sin(2n
π
) = 0, for n integer.
Using the calculator you can simplify the expression in the equation writer
(
‚O
) by replacing e
2in
π
= 1. The figure shows the expression after
simplification:
The result is c
n
= (i
⋅
n
⋅π
+2)/(n
2 ⋅π 2
).
Putting together the complex Fourier series
Having determined the general expression for c
n
, we can put together a finite
complex Fourier series by using the summation function (
Σ
) in the calculator as
follows: