HP 48GII, Руководство пользователя, Страница: 484 / 864

Page 16-6
of constants result from factoring out the exponential terms after the Laplace
transform solution is obtained.
Example 2 – Using the function LDEC, solve the non-homogeneous ODE:
d
3
y/dx
3
-4 ⋅ (d
2
y/dx
2
)-11 ⋅ (dy/dx)+30 ⋅ y = x
2
.
Enter:
'X^2'
`
'X^3-4*X^2-11*X+30'
`
LDEC
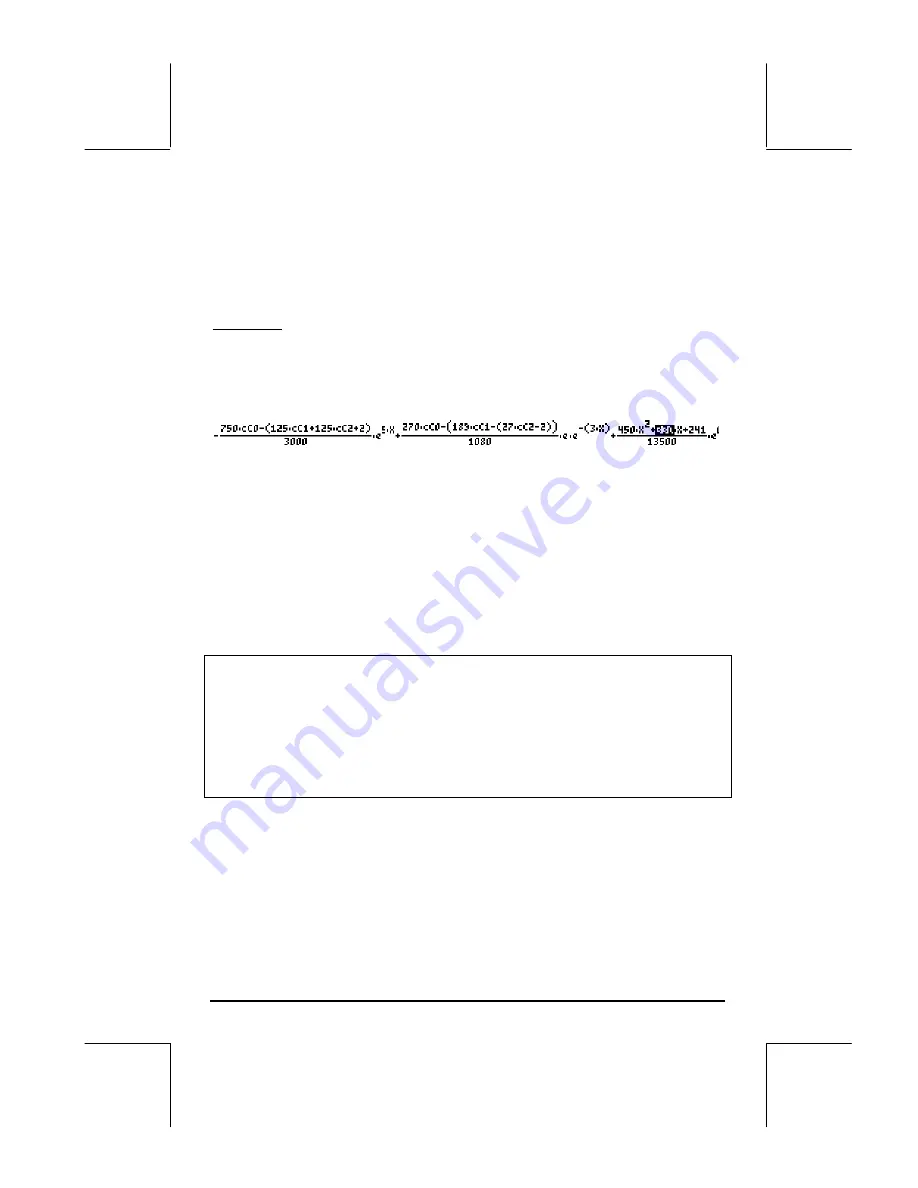
The solution, shown partially here in the Equation Writer, is:
Replacing the combination of constants accompanying the exponential terms
with simpler values, the expression can be simplified to y = K
1
⋅ e
–3x
+ K
2
⋅ e
5x
+
K
3
⋅ e
2x
+ (450 ⋅ x
2
+330 ⋅ x+241)/13500.
We recognize the first three terms as the general solution of the homogeneous
equation (see Example 1, above). If y
h
represents the solution to the
homogeneous equation, i.e., y
h
= K
1
⋅ e
–3x
+ K
2
⋅ e
5x
+ K
3
⋅ e
2x
. You can prove
that the remaining terms in the solution shown above, i.e., y
p
=
(450 ⋅ x
2
+330 ⋅ x+241)/13500, constitute a particular solution of the ODE.
Note
: This result is general for all non-homogeneous linear ODEs, i.e., given
the solution of the homogeneous equation, y
h
(x), the solution of the
corresponding non-homogeneous equation, y(x), can be written as
y(x) = y
h
(x) + y
p
(x),
where y
p
(x) is a particular solution to the ODE.
To verify that y
p
= (450 ⋅ x
2
+330 ⋅ x+241)/13500, is indeed a particular
solution of the ODE, use the following:
'd1d1d1Y(X)-4*d1d1Y(X)-11*d1Y(X)+30*Y(X) = X^2'
`
'Y(X)=(450*X^2+330*X+241)/13500'
`
SUBST EVAL