IM 01C22K01-01E
7-4
7. OPERATION
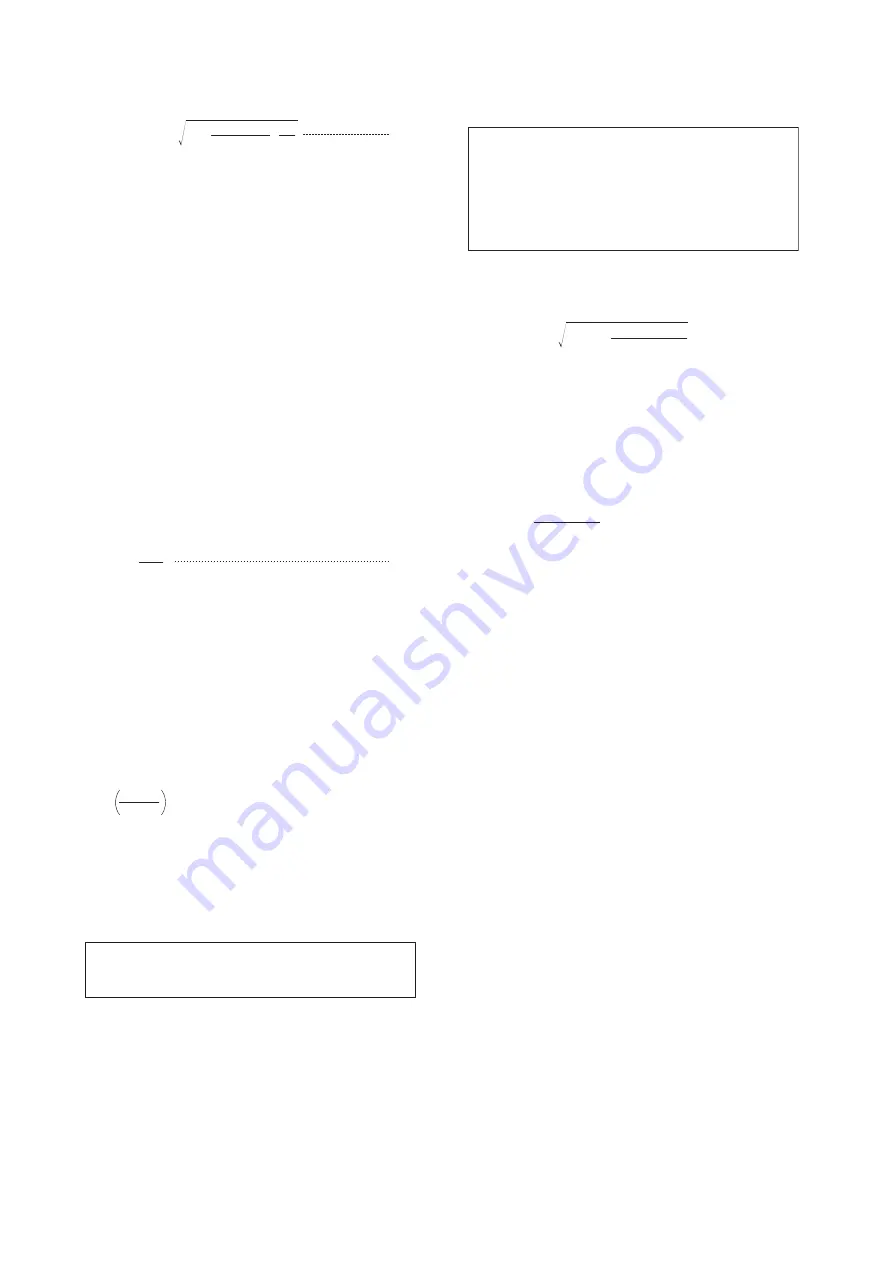
Equivalent Air Flow Calculation
Q
o
= 0.5356 . Qn
ρ
n . .
(2)
273.15 + t
101.325+ p
Zf
Zn
Where, Qo: Air equivalent volumetric flow at 0
°
C,
1 atm (Nm
3
/h)
Qn: Volumetric gas flow at 0
°
C, 1 atm (Nm
3
/h)
n: Specific gas density at 0
°
C, 1 atm (kg/Nm
3
)
Zn: Compression factor of gas at 0
°
C, 1 atm
Zf: Compression factor of gas at operations
conditions (t
°
C, p kPa)
(b) Obtain a differential pressure from the above
equivalent water or air flow using the nomograph
shown in Figure 7.5.1 or 7.5.2. In this procedure,
multiply Qw or Qo by 1000/60 to convert the flow
unit into liter/min.
(c) Select an orifice bore, taking into considerations
pressure loss, etc.
(d) As necessary, calculate Reynolds number at normal
flow rate and correct the differential pressure
obtained from the procedure (b).
Reynolds Number Calculation
Re = 354
(3)
W
D.
Where, Re: Reynolds number at normal flow rate
W: Weight flow at normal flow rate (kg/h)
(Note)
D:
Orifice bore (mm)
:
Viscosity (mPa·s)
Note: Determination of W
· For liquid, W=Qf·
f
· For gas, W=Qn·
n
Differential Pressure Correction using
Reynolds Number
!
P =
2
.
!
P
0
1
Kaf/Ka
Where,
!
P: Corrected differential pressure
!
P
0
: Differential pressure obtained from proce-
dure (b)
Kaf/ka: Correction factor obtained from Figure
7.5.3
For details concerning determination of differential
pressure correction using Reynolds number, pressure loss,
etc., refer to TI 01C20K00-01E.
F0713.EPS
7.5.2 Example of Calculation
F0714.EPS
Fluid: N
2
gas (Nitrogen gas)
Flow range:
0 to 25 Nm
3
/h (flow rate at 0
°
C, 1 atm)
Normal flow rate: 18 Nm
3
/h
Specific density: 1.251 kg/Nm
3
(specific density at 0
°
C,
1 atm)
Temperature: 30
°
C
Pressure: 100
kPa
Viscosity: 0.018
mPa·s
From Equation (2), air equivalent volumetric flow Qo
is:
Q
o
= 0.5356
×
25 1.251
×
= 18.38 Nm
3
/h = 306.3 Nl/min
273.15 + 30
101.325 + 100
A differential pressure range of 0 to 2400 mmH
2
O is
obtained from Figure 7.5.2 applying an orifice bore of
6.350 mm (where, Zf/Zn=1 is assumed).
From Equation (3), Reynolds number at normal flow
rate Re is:
Re = 354
×
= 6.97
×
10
4
18
×
1.251
6.35
×
0.018
Since the correction factor (1.00) is constant at this
Reynolds number, no differential pressure correction is
required. Consequently, the differential pressure range
is determined as 0 to 2400 mmH
2
O.






























