29
W
D
L
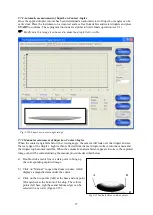
Fig. 9.17 Shape of a pendant drop
9.9 Surface Tension
The instrument must be placed on a stable, horizontal table to give correct results. A sufficiently big
pendant droplet must be pumped out at the dispensing tip to determine the surface tension from the
droplet shape (Bashfort-Adams). The critical dimensions are calculated from a polynomial curve
fitting at sub-pixel resolution with an accuracy of 10
-6
mm, which offers an accuracy of ± 0,2 dyne/cm.
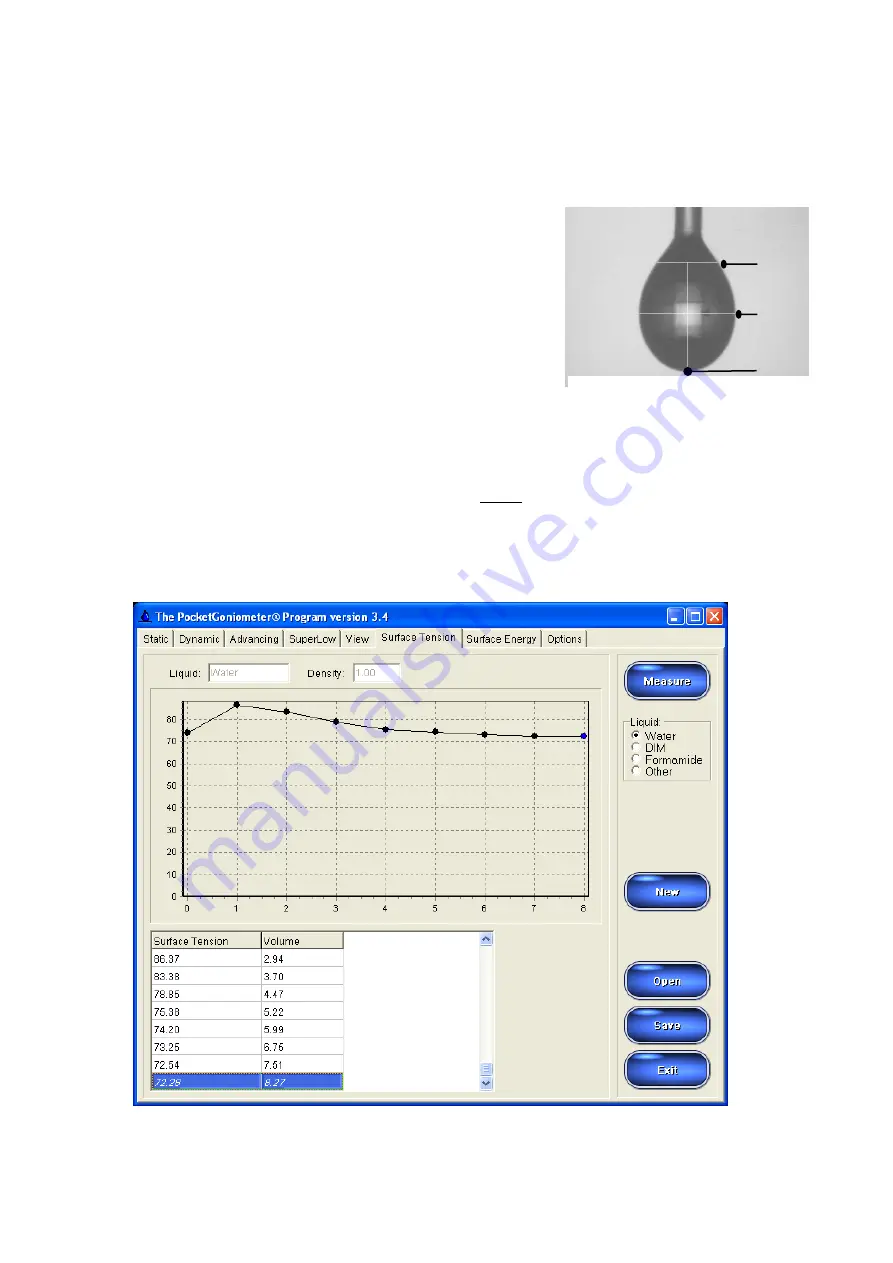
A “too small” pendant droplet at the dispensing tip will give a too
high reading. When the droplet size is gradually increased, gravity
will influence the droplet shape and the surface tension will
gradually decrease. At a certain droplet size the droplet becomes
unstable, wobbling from side to side, which may give an erroneous
result. The correct droplet size is the volume used immediately
before the droplet begins to wobble.
The algorithm used determines the maximum diameter “D” and
the lowest point “L” of the droplet contour (Fig 9.17). The droplet
width “W” is then established at a distance “D” above the point
“L”. The parameters “D” and “W” are finally used to calculate the
liquid’s surface tension from the droplet shape.
Please note the surface tension value is a function of the density of the liquid. This correction
is done automatically if the Density field is updated before the measurement is done.
The AutoRepeat function (see 9.3) is available also for Surface Tension measurements
.
The Remote Control function
offers selection of droplet size and different drop application modes
directly from the PC keyboard (see section 10 for details).
Fig 9.18 Surface tension as a function of droplet size