YASKAWA CIMR-M5 Series Скачать руководство пользователя страница 276

Страница: 276 / 305

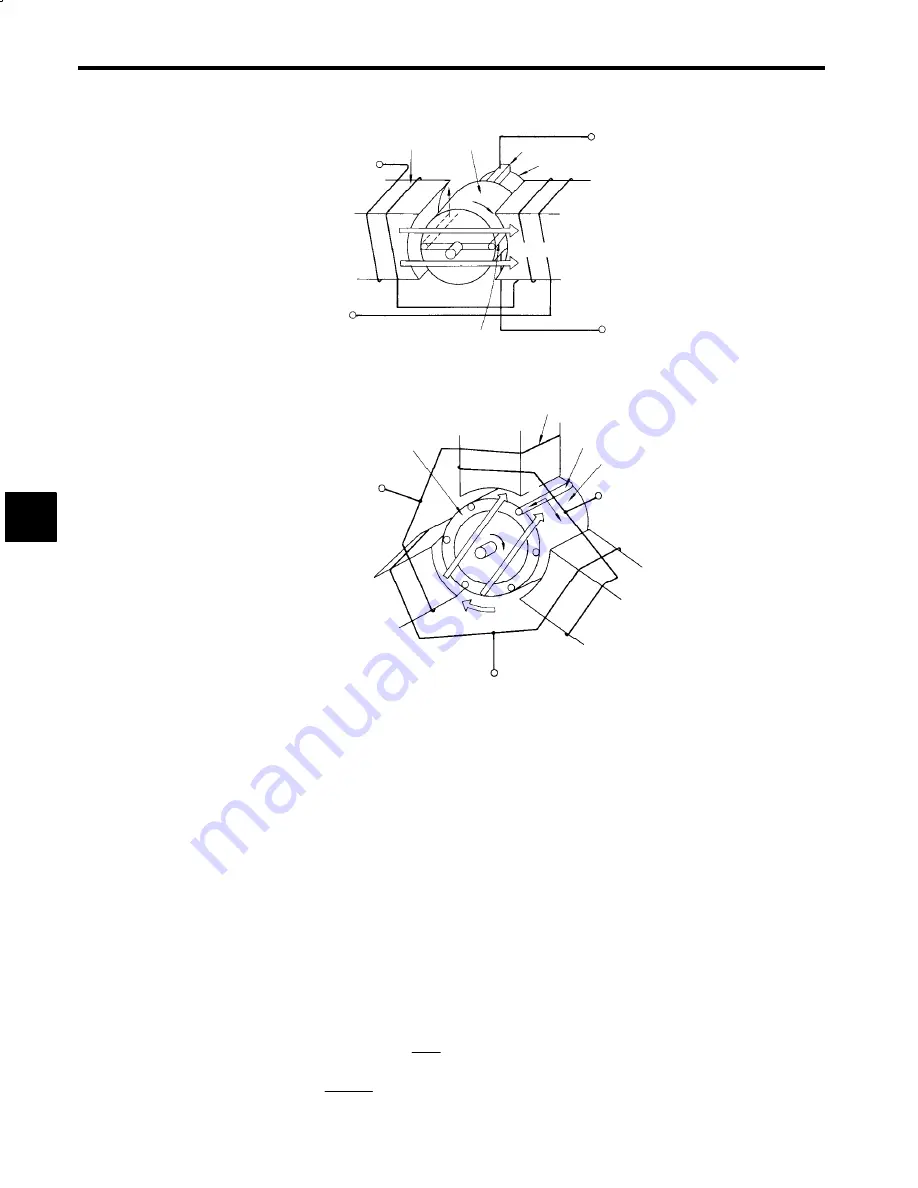
Appendix

15.1.3 Squirrel Cage Induction Motor Characteristics

-4

Magnetic field

winding

Stator

Flange

Commutator

Torque

Magnetic flux

Stator winding

−

Magnetic

field

(a) DC Motor

Stator winding

Rotator conductor

Rotator

Terminal V

Torque

Stator

Short-circuit ring

Terminal U

Terminal W

(b) Squirrel Cage Induction Motor

Magnetic
flux

Fig 1

Motor Model Diagrams

As shown in Fig. 15.5, the rotator is positioned inside the stator; i.e., within the rotation magnetic field.

When there is a difference between the angular velocity

of the rotator and the angular velocity

the rotation magnetic field, the rotator conductor cuts the alternating magnetic field of the differential an-

gular velocity. Consequently, secondary induction electromotive force

is generated in the rotator con-

ductor due to the effect of magnetic field induction. Also, counterelectromotive force

is generated in

the stator due to the effects of electromagnetic induction, and interlinkage between the magnetic flux of

the rotation magnetic field and the stator winding.

= k

= 2

Both tips of the rotator conductor are connected to the short-circuit ring, hence the name “squirrel cage.”

The secondary current

thus flows due to the secondary induction electromotive force

. This is equiva-

lent to the armature current of the DC motor.
Torque is generated using electromagnetic force proportional to the accumulation of secondary current

and magnetic flux

in the same way as the DC motor, causing the rotator to rotate. The ratio of the rotator

to the speed differential of the rotator magnetic field is called the induction motor “slip.” If the rotator is

rotating at the same speed as the rotation magnetic field, the relative position of both will not change, so

the electromagnetic induction effect does not occur, and torque will not be generated. This is called syn-

chronous speed. Synchronous speed and slip are expressed using the following formulas.

Synchronous speed

120

(min

−

)

Slip

−

Содержание CIMR-M5 Series

Страница 1: ...SER S MANUAL INVERTER VS 626M5 MODEL CIMR M5 200V CLASS 3 7 2 2 TO 37 30kW 5 3 TO 50 40HP 400V CLASS 5 5 3 7 TO 45 37kW 7 5 5 TO 60 50HP CONVERTER VS 656MR5 MODEL CIMR MR5 200V CLASS 3 7 2 2 TO 37 30k...

Страница 2: ...bed in this manual D Any illustrations photographs or examples used in this manual are provided as examples only and may not apply to all products to which this manual is applicable D The products and...

Страница 3: ...erateinjurytoperson nel and damage to equipment It may also be used to alert against unsafe practices Evenitemsdescribedin CAUTION mayresultinavitalaccidentinsomesituations Ineithercase follow these i...

Страница 4: ...on may result in a fire 2 5 D Install a fan or other cooling device to keep the ambient temperature of Inverter and Converter below 55_C 131_F and the intake air temperature to heatsink below 45_C 113...

Страница 5: ...tage test of the Inverter and the Converter It may cause semi conductor elements to be damaged 3 2 D Make sure to connect the Inverter and the Converter as shown in the connection dia grams The Invert...

Страница 6: ...Close upper and lower covers before powering up the Inverter or the Converter To open the covers make sure to shut OFF the molded case circuit breaker Failure to observe this warning may result in an...

Страница 7: ...ltinanelectricshockorpersonalinjuryandwillinvalidatethewar ranty CAUTION D Do not store or transport the equipment in locations where halogen fluorine chlorine bromine or iodine is present Failure to...

Страница 8: ...lt in electrical shock CAUTION D Use only a specified combination of Inverter and Motor Failure to observe this caution may result in fire or malfunctions D Never use at locations exposed to water spl...

Страница 9: ...revent them from being touched Failure to observe this warning may result in personal injury D The motor shaft extension is coated withanticorrosive paint Beforeinstallation wipeoff thepaint with a cl...

Страница 10: ...his warning may result in electrical shock CAUTION D Perform trial operation as follows Secure the Motor and disconnect it from load machine system check operations then reconnect the Motor to the loa...

Страница 11: ...nt D If it becomes necessary to disassemble the Motor contact your YASKAWA representative J Warning Label Warning labels are displayed on the upper cover and the front cover of the Inverter and the Co...

Страница 12: ...Notes for Motor xi...

Страница 13: ...However if the Yaskawa Authorized Service Center determines that the problem with a Yaskawa product is not due to defects in Yaskawa s workmanship or materials then the customer will be re sponsible f...

Страница 14: ...on atomic or electric power or underwa ter use must contact their Yaskawa representatives or the nearest Yaskawa sales office be forehand D This product has been manufactured under strict quality cont...

Страница 15: ...ing aids are used to indicate certain types of information for easier reference Indicates application examples Indicates supplemental information Indicates important information that should be memoriz...

Страница 16: ...ontrol Signals 5 5 Operating the Digital Operator 6 6 Trial Operation 7 7 Wide Fixed output Control Using Coil Switching 8 8 Orientation Control Using an Encoder 9 9 Magnetic Sensor Orientation Contro...

Страница 17: ...xvi CONTENTS 12 12 Troubleshooting 13 13 Maintenance and Inspection 14 14 Specifications 15 15 Appendix...

Страница 18: ...2 1 Installation Site 2 5 2 2 2 Operating Ambient Temperature 2 6 2 2 3 Protecting the Inverter and Converter from Foreign Matter 2 6 2 2 4 Storage 2 6 2 3 CLEARANCES 2 7 2 3 1 External Heatsink Cool...

Страница 19: ...2 Setting Sequence Output Signals 4 12 4 4 3 Status Display of Sequence Output Signals 4 12 4 4 4 Details on Sequence Output Signals 4 13 4 5 Analog Monitor Signals 4 18 4 6 Encoder Pulse Input Circui...

Страница 20: ...2 09 and C3 09 and Positioning Completion Cancel Width ZCAN C2 10 and C3 10 6 13 6 4 14 Orientation Speed SORT C2 11 and C3 11 6 14 6 4 15 BCD Stop Position Reference Resolution PBCD C2 12 and C3 12 6...

Страница 21: ...9 6 9 4 2 Magnet 9 6 9 4 3 Magnetic Sensor 9 7 9 5 Connections between Devices 9 8 9 5 1 Magnetic Sensor Signal 9 8 9 5 2 Stop Position References 9 8 9 6 Control Signal Connector Terminal Arrangement...

Страница 22: ...r Total Indicator Readings 14 37 14 2 7 Encoders 14 38 14 2 8 Encoder Connector 14 39 14 2 9 Spare Motor Parts 14 40 14 2 10 Replacing the Motor Cooling Fan 14 41 14 3 Options and Peripheral Units 14...

Страница 23: ...M5A Stand alone Drive 15 21 15 4 4 Analog Monitor Signals M5A Stand alone Drive 15 22 15 4 5 YENET1200 Signals M5N NC Drive 15 22 15 5 Inverter Converter Cooling Design 15 23 15 5 1 Temperature Rise...

Страница 24: ...f the VS 626M5 Inverter and VS 656MR5 Converter and describes their functions and components 1 1 Overview 1 2 1 1 1 Features 1 2 1 1 2 Inverter Models 1 3 1 1 3 Converter Models 1 4 1 2 Identifying Co...

Страница 25: ...he downsizing of the spindle motor was enabled by the optimum electromagnetic design of the system ensuring ideal heat distribution improvements in the core and cooling construction of the system and...

Страница 26: ...M5A20370 M5A 5 5 CIMR M5A45P5 CIMR M5A45P55 CIMR M5A45P50 7 5 CIMR M5A47P5 CIMR M5A47P55 CIMR M5A47P50 11 CIMR M5A4011 CIMR M5A40115 CIMR M5A40110 15 CIMR M5A4015 CIMR M5A40155 CIMR M5A40150 400 V cla...

Страница 27: ...10 15 CIMR MR5A4015 CIMR MR5A40155 CIMR MR5A40150 400 V class 18 5 CIMR MR5A4018 CIMR MR5A40185 CIMR MR5A40180 22 CIMR MR5A4022 CIMR MR5A40225 CIMR MR5A40220 30 CIMR MR5A4030 CIMR MR5A40305 CIMR MR5A4...

Страница 28: ...ARGE LED Lower Cover Front Cover A1 r A2 t R L1 S L2 T L3 P1 N1 CHARGE 8 8 N1 P1 1CN 5CN P N R L1 S L2 T L3 A1 r A2 t P N Mounting Base Case Upper Cover 4 Mounting Holes Heatsink Control Power Supply...

Страница 29: ...Nameplate Control Power Supply Input Main Circuit Power Supply Input Ground Upper and Lower Covers Opened Mounting Base Case Upper Cover 4 Mounting Holes Heatsink 9CN 10CN Optional Inverter Outputs 52...

Страница 30: ...2 4 2 2 Checking and Controlling the Installation Site 2 5 2 2 1 Installation Site 2 5 2 2 2 Operating Ambient Temperature 2 6 2 2 3 Protecting the Inverter and Converter from Foreign Matter 2 6 2 2 4...

Страница 31: ...oose Use a screwdriver or other tools to check for tightness If any of the above checkpoints are not satisfactory contact your Yaskawa representative 2 1 1 Inverter Nameplate Information J Nameplate I...

Страница 32: ...mple of a Model for 200 VAC 15 HP 11 kW Serial No Converter Model Input Spec Converter Spec Output Spec PROM number Mass YASKAWA ELECTRIC CORPORATION INPUT AC 3PH 200 220 V 50 Hz 200 230 V 60 Hz 19 kV...

Страница 33: ...onth and year of manufacture Model number Number of phases Ratings Bearing number load side motor side Serial number Number of poles Fig 2 7 Motor Nameplate J Motor Model Designations Voltage class 20...

Страница 34: ...t If installing the Inverter or Converter in the control panel care must be taken when planning this instal lation to prevent oil mist from entering the panel thorough gaps in the welded sections D In...

Страница 35: ...e reliability of operation the Inverter and Converter should be installed in an environment free from extreme temperature increases If the Inverter or Converter is installed in an enclosed environ men...

Страница 36: ...o 45_C 32_F to 113_F S External heatsink cooling type Inside of heatsink 0_C to 45_C 32_F to 113_F Inside of unit 0_C to 55_C 32_F to 131_F 3 Near the heatsink cooling air speed should be 2 5 m s for...

Страница 37: ...mm 5 91 inches or more Fig 2 10 Clearances for Open Chassis Type When using an Open chassis Converter 11 kW or more in combination with an Inverter 7 5 kW or less follow the installation procedure sh...

Страница 38: ...as an option The Exclusive use Extension Cable 72616 W5301 or 72616 W5303 is required when connecting the Digital Operator with the Inverter Use 3CN to attach the digital operator firmly as follows D...

Страница 39: ...th a maximum capacity of 15 11 kW and the external diameter is 260 mm 10 2 inches or less Frequency of vibration acceleration is 10 to 60 Hz constant amplitude or 60 to 2 500 Hz constant acceleration...

Страница 40: ...If an excessive radial load is imposed on the motor shaft the motor bearings will be adversely affected and the service life of the bearings will be decreased Check that the angle of contact of the b...

Страница 41: ...s of Main Circuit Terminals 3 13 3 3 3 Main Circuit Configuration 3 15 3 3 4 Main Circuit Connection Diagrams 3 19 3 3 5 Wiring the Main Circuit 3 21 3 4 Wiring Control Circuit Signals 3 24 3 4 1 Cont...

Страница 42: ...ed voltage of the Converter coincides with the AC power supply voltage Failure to observe this caution can result in personal injury or a fire D Do not perform a withstand voltage test of the Inverter...

Страница 43: ...Class or 400 VAC 3 phase 400 V Class Molded case Circuit Breaker 1MCCB or Ground Fault Interrupter Magnetic Contactor 1MC 2 Molded case Circuit Breaker 3MCCB or Ground Fault Interrupter Grounding VS 6...

Страница 44: ...achine and servo units refer to the manual for the NC ma chine R S T r t VS 656MR5 Converter Unit 5CN P N N1 P1 VS 626M5 52CN P N 51CN N1 P1 2CN PG M 52CN P N 51CN 2CN PG M 52CN P N 51CN 2CN PG M 4CN...

Страница 45: ...8 29 FC0 FC1 FC2 FC3 30 COM2 BCD BIN 1 1 2 2 4 4 8 8 1 2 4 8 U T1 V T2 W T3 W Z1 Z2 Z3 10 20 40 80 100 200 400 800 10 20 40 80 16 32 64 128 256 512 1024 2048 EXTCOM 24VCOM 0VCOM D1 D2 D3 D4 D5 D6 D7 D...

Страница 46: ...3CN For Terminal Unit For Monitoring Zero speed 4CN 4 6 R S SW1 1CN 7 8 13 14 17 P ALM ALMC PAO PAO SS U T1 V T2 W T3 R1 S1 T1 Z1 Z2 Z3 U V W IM 2CN 5V 0V 4 5 6 1 2 3 PA PA PB PB PC PC 16 17 18 19 14...

Страница 47: ...2 94 1 27P5 R L1 S L2 T L3 M5 2 35 10 5 3 3 5 2 3 5 27P5 A1 r A2 t M5 2 35 14 2 1 2 2 2 M4 1 2 2 0 10 5 3 3 5 2 3 5 P N M6 2 2 94 1 2011 R L1 S L2 T L3 M6 3 4 4 9 8 8 4 8 3 5 8 2011 A1 r A2 t M4 1 2...

Страница 48: ...2 0 14 2 1 2 2 2 M6 M6 3 4 to 4 9 10 5 3 3 5 2 2 P N M6 2 2 94 1 4015 R L1 S L2 T L3 M6 3 4 to 4 9 10 5 3 3 5 2 3 5 4015 A1 r A2 t M4 1 2 to 2 0 14 2 1 2 2 2 M6 M6 3 4 to 4 9 10 5 3 3 5 2 3 5 P N M6...

Страница 49: ...13 3 14 8 14 M6 2 3 4 to 4 9 8 8 4 8 5 5 5 5 P N M6 2 2 94 1 2015 U T1 V T2 W T3 M8 6 47 4 21 2 22 14 22 M6 2 3 4 to 4 9 6 13 3 14 8 8 P N M6 2 2 94 1 2018 U T1 V T2 W T3 M8 6 47 3 26 7 30 14 30 M6 2...

Страница 50: ...4 4018 M5 2 2 1 to 2 5 8 8 4 8 5 5 5 5 M5 2 M6 3 4 to 4 9 8 8 4 8 5 5 5 5 P N M6 2 2 94 1 4022 U T1 V T2 W T3 M6 3 4 to 4 9 6 13 3 14 8 14 4022 M5 2 2 1 to 2 5 8 8 4 8 5 5 5 5 M5 2 M6 3 4 to 4 9 8 8 4...

Страница 51: ...B 15 M8 M4 2018 A 19 M8 M4 B 19 M10 M4 2022 A 22 M8 M4 B 22 M10 M4 2030 J 30 M10 M4 B 30 M10 M4 2037 J 37 M10 M4 M4 Table 3 6 Terminal Screws for 400 V Class Motors Inverter Standard Motor Winding Sel...

Страница 52: ...75 18 M3 5 1 25 to 3 5 0 75 18 M4 1 25 to 4 1 25 16 M3 5 1 25 to 3 5 1 25 16 M4 1 25 to 4 M3 5 2 to 3 5 M4 2 to 4 2 14 M5 2 to 5 M6 2 to 6 M8 2 to 8 M4 5 5 to 4 3 5 to 5 5 12 to 10 M5 5 5 to 5 3 5 to...

Страница 53: ...VDC For inverter main circuit power supply P1 N1 Control power supply output 282 to 325 VDC For inverter control power supply Grounding Ground terminal Ground resistance 100 or less R L1 S L2 T L3 Mai...

Страница 54: ...0 to 230 VAC 60 Hz U T1 V T2 W T3 Inverter output Inverter output to motor Grounding Ground terminal Ground resistance 100 or less P N Main circuit power supply input 540 to 650 VDC Supplied from conv...

Страница 55: ...1 N1 5CN 24V 0V P N U T1 V T2 W T3 P1 N1 51CN 52CN 0V Note Control Circuit Power Supply RCC 24V Note P N R L1 S L2 T L3 A1 r A2 t P1 N1 5CN 24V 0V P N U T1 V T2 W T3 P1 N1 51CN 52CN 24V 0V Converter V...

Страница 56: ...5CN 24V 0V P1 N1 A11 r1 A21 t1 P N P1 N1 24V 0V 52CN U T1 V T2 W T3 51CN A12 r2 A22 t2 24 V Power Supply RCC Control Circuit Power Supply Note Control Circuit Power Supply RCC Note Heatsink Cooling F...

Страница 57: ...r Supply Note Control Circuit Power Supply RCC Note Converter VS 656MR5 Inverter VS 626M5 CIMR MR5j40115 to 40455 CIMR M5j40115 to 40455 P N R L1 S L2 T L3 A1 r A2 t P1 N1 5CN 24V 0V P N U T1 V T2 W T...

Страница 58: ...MR5 Inverter VS 626M5 CIMR MR5j40110 to 40220 CIMR M5j40110 to 40220 Internal Cooling Fan 24 V Power Supply RCC Control Circuit Power Supply Note Control Circuit Power Supply RCC Note Heatsink Cooling...

Страница 59: ...ain Circuit Connections for 200 V Class External Heatsink Cooling Type J 200 V Class Open Chassis Type Converter VS 656MR5 CIMR MR5j23P70 to 27P50 Inverter VS 626M5 CIMR M5j23P70 to 27P50 R S T r t R...

Страница 60: ...rnal Heatsink Cooling Type J 400 V Class Open Chassis Type Converter VS 656MR5 CIMR MR5j45P50 to 47P50 Inverter VS 626M5 CIMR M5j45P50 to 47P50 R S T r t R L1 S L2 T L3 A1 r A2 t VS 656MR5 P N VS 626M...

Страница 61: ...nal Block Connection Sequence Main circuit power supply input phases can be connected to any terminal regardless of the order of R L1 S L2 and T L3 on the terminal block Installation of AC Reactor Mak...

Страница 62: ...cting the Converter Control Power Supply Output Connect converter control power supply output terminals P1 and N1 to inverter left side control power supply input terminals P1 and N1 using exclusive u...

Страница 63: ...to ground the ground terminal 200 V class Ground to 100 or less 400 V class Ground to 10 or less D Never ground the inverter or the converter in common with welding machines motors or other large cur...

Страница 64: ...nit 8830E 068 170LD 32 8822E 034 171D 4 2 3 1 special cable KEL Corp Control PC Board VS 626M5 1CN 36P Control signals 10236 52A2JL S 10136 3000VE S 10336 52A0 008 case 36 19 18 1 0 2 mm2 Sumitomo 3M...

Страница 65: ...s for wires are not sold separated Refer to 14 3 6 Connector Kit Some of the connectors attached with control PCboard and option cards are of the same type Therefore make sure to mount the cards to th...

Страница 66: ...g 3 4 2 Terminal Arrangement of Control Signal Connector 3 26 3 4 2 Terminal Arrangement of Control Signal Connector Figures 3 18 and 3 19 show the terminal arrangements of the control signal connecto...

Страница 67: ...ARGE 8 CHARGE 4CN 6CN 45 FLTCOM 20 EXTCOM0 30 D12 12 PCO P1 8 8 P1 1CN 44 FLTNC 19 EXTCOM0 29 D11 11 PCO N1 N1 1CN 43 FLTNO 18 MGR 28 D10 10 BAT 1CN 42 COM1 17 LGR 27 D9 9 BAT 1CN 2CN 8CN 41 FLTL 16 O...

Страница 68: ...13 12 6 5 OP1 0 10 12V 3 0V 12 11 5 4 5 V 0 V RX 9 2 11 10 4 3 5 V 0 V RX 8 1 SS 10 9 3 2 5 V 0 V TX 9 8 1 5 V OP2 0 V TX 8 1 OP2 0 V O 0 V Note Terminal arrangement is as when the connectors on the...

Страница 69: ...oder phase B signal input RS 422A specification Line receiver PB 19 Encoder phase B signal input Line receiver 5 V PC 14 Encoder phase C signal input 5 V PC 15 Encoder phase C signal input 2CN THSA 8...

Страница 70: ...sup ply common 6CN 24VCOM 22 23 Sequence input signal power sup ply 24V 0VCOM 24 25 Sequence input signal power sup ply 0V FC0 26 Fault code 0 FC1 27 Fault code 1 Open collector output FC2 28 Fault c...

Страница 71: ...der phase B signal output Line driver 5 V option SPCO 2 Encoder phase C signal output 5 V SPCO 3 Encoder phase C signal output SS 1 Shield 0V SIG 13 Magnetic sensor signal SIG 14 Magnetic sensor signa...

Страница 72: ...the external common method prepare a 24 V 20 to 26 V power supply for the input signal D The 1CN common and 6CN common terminals are insulated Therefore it is possible to used the com mon terminals i...

Страница 73: ...D The following diagram is a sequence output signal circuit Fig 3 21 Output Interface Circuit 3 4 6 Precautions for Control Signal Wiring For proper wiring between devices pay attention to the followi...

Страница 74: ...lded wires for motor encoder signal lines and connect both ends as shown below Shielded Sheath Armor To inverter shielded sheath terminal Insulate these parts with insulating tape To encoder shielded...

Страница 75: ...of the Motor Inverter and Converter The screw terminals of the motor fan power supply and the electromagnetic contactor for winding selection The mounting bolts of the Motor Inverter and Converter D...

Страница 76: ...3 4 1 4 Details on Sequence Input Signals 4 3 4 2 Analog Speed Reference 4 9 4 3 Using a 12 bit Digital Speed Reference 4 10 4 4 Sequence Output Signals 4 12 4 4 1 Connecting Sequence Output Signals 4...

Страница 77: ...nce Input Signals Some functions of sequence input signals are selected by settings constants Set the constants as shown in the following table for the desired functions Table 4 1 Sequence Input Signa...

Страница 78: ...ked instantly and the motor current will be shut off D While the RDY signal is OFF the motor will not start unless the FWD and REV signals are turned OFF together D Always keep the RDY signal ON if th...

Страница 79: ...signal functions when 6CN 7 turns OFF D The main circuit capacitor will be charged after the EMG signal is turned ON The motor will be ready to operate a maximum of 2 5 s after the EMG signal is turn...

Страница 80: ...lection signals SEL1 is turned OFF and bit 1 is turned ON Bit 1 0 C1 36 The INC signal functions when 6CN 11 turns ON D Used for incremental operation under orientation control D The INC signal will b...

Страница 81: ...election 6CN 15 will be the LM10 signal if bit 04 of the C1 36 selection signals SEL1 is turned ON Bit 4 C1 36 The LM10 signal functions when 6CN 15 turns ON Not used for NC system M5N D The LM10 sign...

Страница 82: ...he gate will be blocked until the actual winding is switched over If this status continues for the preset time a winding selection fault AL 20 will result and the system will stop ON ON ON Winding Hig...

Страница 83: ...signal will be selected when 6CN 17 is turned ON D The MGR and LGR signals are used to change parameters such as the gear ratio and gain to ensure the optimum control of the load according to the gea...

Страница 84: ...he level is set to 105 when bit 5 is turned OFF and 110 when bit 5 is turned ON D The SCOM signal has an input impedance of 50 k D The speed together with the rotating direction is determined by the S...

Страница 85: ...ignal can be switched only when the system is not in operation D If the binary BCD or internal speed setting method is selected the forward or reverse rotation of the motor is selected with the extern...

Страница 86: ...512 200 20 D11 29 1024 400 40 D12 30 2048 800 80 D All signals will be ONwith the rated speed reference set in C1 26 if the 12 bit binary setting is selected D If the 3 or 2 digit BCD setting is sele...

Страница 87: ...Related Constants 1 33 ZSPD Zero speed C1 19 Zero speed detection level 2 34 AGR Speed agree C1 20 Speed agree detection width C1 38 Bit 6 AGR output condition selection 3 35 SDET Speed detection C1...

Страница 88: ...etween 3 and 60 min 1 D The ZSPD signal is output regardless of the status of the FWD or REV output Therefore the ZSPD signal can be used as an interlock signal for hazard prevention J AGR Speed Agree...

Страница 89: ...will turn ON when the torque reference reaches the preset value or less D Once TDET turns OFF the status is held for 50 ms D The torque detection level is set between 5 and 120 of the 30 minute ratin...

Страница 90: ...constant speed Winding High speed Low speed CHW CHWE AGR ON ON ON ON ON J ORE Orientation Completion Signal Connector number 6CN Pin number 39 42 D The ORE signal will turn ON when the ORT signal inp...

Страница 91: ...ether with the protective circuit D Turn OFF the FWD REV or ORT signal while the FLT signal is output and then display the fault at the host system D The fault number is displayed when the FLTL is out...

Страница 92: ...re is a minor fault in an optional function such as an orientation fault J FLTL Fault Signal Connector number 6CN Pin numbers 30 41 D The FLTL signal will turn OFF if a fault occurs The FLTL signal is...

Страница 93: ...ated SM signal output 10 V will turn ON when the motor is rotating at the speed set in C1 26 S100 the rated speed set constant D The SM signal level can be adjusted with C1 16 SMADJ the control consta...

Страница 94: ...er pulse C J Input Circuit Configuration The input circuit is a line receiver with RS 422 A specifications Inverter Line receiver equivalent to SN75175 Output line driver equivalent to SN75174 Encoder...

Страница 95: ...line receiver with specifications matching the RS 422A specifications for signal exchange as shown in the following connection example Phase A Phase B Phase C Output line driver SN75174 Receiver circu...

Страница 96: ...f the Digital Operator 5 2 5 2 Display Mode Configuration 5 5 5 3 Key Operations and Display 5 6 5 3 1 Indication at Power ON 5 6 5 3 2 Switching Display Functions 5 6 5 3 3 Operation Status Display M...

Страница 97: ...ecifications Chapter 10 Control Constants lists the control constants J Display of Protective Functions If an error occurs during operation protective functions are displayed 12 2 Converter Faults and...

Страница 98: ...write set values Numeral Change Key Use these keys to change values such as set values and constant Nos Increment key Decrement key Digit Selection Key Use this key to select a position in a set val u...

Страница 99: ...and Letters by 7 segment LED Numbers Letters 0 A N 1 B O 2 C P 3 D Q 4 E R 5 F S 6 G T 7 H U 8 I V 9 J W G K X L Y M Z Note is not displayed 1 ON 0 OFF Selection signal 0 OFF indicates and 1 ON indic...

Страница 100: ...ayed for 0 5 s U1 01 motor speed data is displayed The operating status is displayed The control constants are displayed C1 37 bits 1 and 0 turned ON The Digital Operator operation reference is displa...

Страница 101: ...is disconnected 5 3 2 Switching Display Functions Depress DSPL key on the Digital Operator to change the mode of display Description Key Sequence Digital Operator Display Remarks S Motor speed U1 01...

Страница 102: ...ol constant display mode do as follow The following shows the example where C1 10 soft start time is to be changed Description Key Sequence Digital Operator Display Remarks S C1 01 is displayed S Move...

Страница 103: ...play C1 37 data con tents DATA ENTER S Select the digit of bit 1 second place from the right RESET S Change set value Turn ON lower two bits RESET S Write in set value DATA ENTER Displayed for 0 5 sec...

Страница 104: ...ed in the Digital Operator operation mode Rotating direction is se lected by FWD REV key and run stop by RUN or STOP key Table 5 3 Key Operations in Digital Operator Operation Mode Key Name Function D...

Страница 105: ...verspeed d2 occurred CPU built in A D error Display Example D Displays the second fault No D Displays the first fault No D Displays the third fault No Notes on resetting faults S To reset a fault by t...

Страница 106: ...st fault No D Displays the second most recent fault No D Displays the third most recent fault No D Displays the fourth most recent fault No D Displays the fifth most recent fault No D Displays the six...

Страница 107: ...erter Control Power Supply Is Turned ON 6 7 6 3 3 Display when an Error Occurs 6 8 6 4 Constant Settings 6 9 6 4 1 User Constant Functions Table 6 9 6 4 2 Soft Start Time Setting TSFS C1 10 6 10 6 4 3...

Страница 108: ...th ZFIN C2 09 and C3 09 and Positioning Completion Cancel Width ZCAN C2 10 and C3 10 6 13 6 4 14 Orientation Speed SORT C2 11 and C3 11 6 14 6 4 15 BCD Stop Position Reference Resolution PBCD C2 12 an...

Страница 109: ...an result in personal injury CAUTION D Never touch the heatsink because the temperature is very high Failure to observe this caution can result in harmful burns to the body D Verify the safe working r...

Страница 110: ...Address If using an NC Drive M5N set the Inverter YENET1200 node address correctly using rotary switch SW1 5 5 Turn ON Control Power Supply Turn ON the control power supply and check that there are n...

Страница 111: ...opti mum operation according to the characteristics can be achieved 6 2 2 Setting the YENET1200 Node Address Before turning ON the control power supply set the YENET1200 node address using rotary swi...

Страница 112: ...f the Motor shaft If the rotation direction is reversed or if the Motor vibrates but does not rotate even when a RUN signal is sent the phase sequence of the power supply cable and the encoder signal...

Страница 113: ...hat the Inverter is not operating baseblock condition Indicates that the Inverter is operating Error number Error details Indicates an error Alternately displays the error number and the error details...

Страница 114: ...will be saved Consequently the order in which the errors occurred can be viewed The display switches automatically Example Overcurrent error number 01 and main circuit overvoltage error number 11 hav...

Страница 115: ...signal tti Zero speed Detection Level ZSPD C1 19 ON ON q p g settings Speed agree Signal AGR C1 20 and C1 38 bit 6 ON ON Speed Detection Signal SDET C1 21 and C1 22 ON ON Torque Detection Signal TDET...

Страница 116: ...of the load factor meter using control constant C1 18 The setting can be between 120 and 500 6 4 4 Zero Speed Detection Level ZSLVL C1 19 Use this constant to set the detection level for the zero spee...

Страница 117: ...on level can be set between 5 and 120 taking 30 minutes rated torque to be 100 As shown in the following diagram the operation point has an hysterisis of 10 Lit when TDET is ON U1 10 display Torque re...

Страница 118: ...6 000 1 500 7 5 5 5 164 2011 07 UAASKB 11FZj 064 6 000 1 500 11 7 5 176 2015 08 UAASKB 15FZj 065 4 800 1 000 15 11 164 2018 09 UAASKB 19FZj 066 4 800 1 000 18 5 15 148 2022 0A UAASKB 22FZj 067 4 800 1...

Страница 119: ...el Width ZCAN C2 10 and C3 10 Make sure the Motor is stopped before setting the Positioning Completion Detection Width and Position ing Completion Cancel Width The orientation completion signal turns...

Страница 120: ...each machine separately and then determine the orientation speed by referring to the dia gram below This speed is the upper limit so the orientation speed can be set to a lower value than this one For...

Страница 121: ...resolution can be set between 0 5_ and 180 0_ but the stop position refer ence must be within 360_ For example when the set value 90_ the resolution will be 90_ with a stop position reference of 1 180...

Страница 122: ...rd rotation PWD Digital Analog Is speed reference analog or digital After acceleration is completed check the motor speed using U1 01 Does motor speed agree with the ref erence Higher than reference A...

Страница 123: ...l adjustment value C1 16 P U 1 5 1 0 0 9 0 0 9 1 0 1 5 C1 16 Load factor meter display maximum value 120 of rated torque for 30 min Speed ometer signal C1 16 Actual measured motor speed Speedometer di...

Страница 124: ...ing winding selec tion 7 1 Features of the Winding Selection Wide Constant Power Drive 7 2 7 2 Winding Selection Motor Standard Connections 7 3 7 3 Motor Characteristics 7 4 7 4 Winding Selection Oper...

Страница 125: ...e using the AC main shaft drive the Motor current must also be expanded in the low speed area and the controller capacity must also be increased When using winding selection a constant power control o...

Страница 126: ...FC0 FC1 FC2 FC3 30 COM2 BCD BIN 1 1 2 2 4 4 8 8 1 2 4 8 P r1 t1 IM X Y Z r1 t1 U V 2CN 10 20 40 80 100 200 400 800 10 20 40 80 16 32 64 128 256 512 1024 2048 EXTCOM 2 24VCOM 0VCOM 31 32 33 19 20 21 22...

Страница 127: ...ow speed and high speed windings between SBH and SML so winding selection is performed within this speed range If the same output occurs in both the low speed and high speed coils between SBH and SML...

Страница 128: ...ary contact Winding selection completion signal CHWE Control constant Motor current Low speed constant High speed constant Approx 30 ms Preparing to switch Switching Reset after switching Reference Co...

Страница 129: ...peed agree AGR Switching completed CHWE NO YES YES YES YES NO YES YES NO NO NO YES YES NO NO K NO Speed reference Speed reference SCHW Speed reference S reference K Winding selection CHW turns OFF Win...

Страница 130: ...7 5 Winding Selection Methods 7 7 Speed reference Speed M41 S500 M42 S2000 M41 S500 M41 M42 AGR CHWE CHW OFF ON OFF ON OFF ON 0 0 500 min 1 2000 min 1 500 min 1 SCHW Fig 7 5 Timing Chart 7...

Страница 131: ...oil CHW Turns OFF Winding selection completed CHWE End Motor speed SCHW nS SDET ON High speed winding Select low speed winding CHW Turns ON Winding selection completed CHWE YES YES YES NO NO NO YES YE...

Страница 132: ...nce and the Inverter speed detection signal SDET Refer to the table below Compared with the changing method that uses only the speed detection signal signal processing is increased but the fre quency...

Страница 133: ...he speed detection signal detection width C1 22 SDHYS Table 7 1 Winding Selection Conditions Speed Speed Reference SCHW S SCHW S Speed SCHW S SDET OFF High speed winding selected Winding selection not...

Страница 134: ...high D If using the main shaft drive on the lathe automatic winding selection will be performed when chang ing speed is reached even during cutting As shown in the following diagram during rough cutt...

Страница 135: ...Load Shaft Mounted Encoder NE 1024 2MDF 068 8 6 8 5 Load Shaft Encoder Connector Terminal Arrangement 8 7 8 6 Important Points for Encoder Mounting and Wiring 8 8 8 7 Stop Position Reference Signals...

Страница 136: ...oder signal Transmission mechanism gear belt etc Tool 1 1 Main shaft Stop position reference Orientation Orientation signal gear selection VS 626M5 6CN 1CN 2CN AC main shaft motor No Encoder Orientati...

Страница 137: ...1 S L2 T L3 r t 2MCCB 2MC A1 r A2 t 1CN P N U V IM BCD BIN 1 1 2 2 4 4 8 8 10 20 40 80 100 200 400 800 10 20 40 80 16 32 64 128 256 512 1024 2048 10 20 40 80 100 200 400 16 32 64 128 256 512 1024 19 2...

Страница 138: ...6CN 47 48 49 50 SM 0V 0V LM SM LM SPOA SPOA SPOB SPOB SPOC SPOC SS 9CN 4 5 6 2 3 1 7 P P P P 8CN 4 5 6 1 2 3 SPA SPA SPB SPB SPC SPC 16 17 18 19 14 15 20 SS 5V 0V P P K A N C R B P E H 0V PA PA PB PB...

Страница 139: ...ger for rapid load variations 8 3 2 Load Shaft Encoder Specifications The load shaft encoder specifications are shown in the following table Table 8 2 Load Shaft Encoder Specifications Item Details Mo...

Страница 140: ...d Shaft Mounted Encoder NE 1024 2MDF 068 Unit mm Four 5 5 dia mounting holes Key position 20 5 0 012 0 3 0 05 0 56 0 2 68 0 5 28 3 5 2 1 15 0 014 0 33 0 5 102 1 97F3102E20 29P 68 0 5 56 0 2 50 86 0 00...

Страница 141: ...02A20 29P Cables MS3106A20 29S Straight plug solid shell MS3106B20 29S Straight plug 2 piece shell MS 3108B20 29S L plug 2 piece MS3057 12A Cable Clamp A B C D E F G H I PA PC PB FG 5 V K L M N P R S...

Страница 142: ...een B1 Blue and white blue B2 Yellow and white yellow B3 Green and white green B4 Orange and white orange B5 Purple and white purple B6 Gray and white gray All wires are twisted pair wires 1 Blue and...

Страница 143: ...signal is ON the indicator is lit Fig 8 9 Stop Position Reference Signal Status Display 8 7 3 Details of the Stop Position Reference Signal This section explains the stop position reference signals J...

Страница 144: ...pend on the sign or If the sign is positive the total number of bit pulses input is added 0 0 1 0 1 0 0 1 0 0 1 256 64 8 1 329 If the sign is negative the negative of sum of the number of bit pulses i...

Страница 145: ...tation signal is input while the motor is rotating or while the motor is stopped the load shaft immediately decelerates or accelerates to the orientation speed that has been set After attaining the se...

Страница 146: ...me time In this mode the shaft advances by the specified rotation travel amount only whenever an orientation sig nal is input The timing chart for the incremental positioning operation is shown in the...

Страница 147: ...ernal force If the ORT signal is input when the detected position of the origin signal is cleared the motor will rotate to detect the origin signal D If the ORT signal is input when the detected posit...

Страница 148: ...stop at ori gin Perform error diagnosis and correct error Select control constant display for load shaft origin C2 01 Set the origin data and press the ENTER Key Shaft stops at new origin 1 Initial Se...

Страница 149: ...rned ON Adjust Control Constants Adjust Virtual Stop Position Offset C2 13 S Perform adjustment so that final run on is not delayed and there is no overshooting S If the load inertia changes the chara...

Страница 150: ...ETC62614X 9 6 9 4 2 Magnet 9 6 9 4 3 Magnetic Sensor 9 7 9 5 Connections between Devices 9 8 9 5 1 Magnetic Sensor Signal 9 8 9 5 2 Stop Position References 9 8 9 6 Control Signal Connector Terminal...

Страница 151: ...t for positioning references a Magnetic Sensor and a Magnetic Sensor Orientation Card In addition after performing positioning using the Magnetic Sensor user set position stop control can be per forme...

Страница 152: ...100 200 400 16 32 64 128 256 512 1024 Z1 Z2 Z3 W U T1 V T2 W T3 12 3 1 EXTCOM 2 24VCOM 0VCOM D2 D3 D4 D5 D6 D7 D8 D9 D10 D11 D12 D1 31 32 33 20 21 22 23 24 25 26 27 28 29 30 19 3 6CN SM 47 0V 48 SM LM...

Страница 153: ...the range 2_ using control constants Stop Position Repeat Accuracy 1 0 2_ max Resistance Torque 1 Continuous rated torque 0 1_ displacement 2 Orientation Card Code number ETC62614X Magnet Model MG 137...

Страница 154: ...V max 1 5 k 0 Output Displacement Position Signal for Monitor Range Offset 30_ min 1 24 V max 0 5 V max 0 Output Displacement Ambient Operating Tempera ture 10 to 50_C Output Terminals Supplied with...

Страница 155: ...sor Orientation Card can be shipped after being mounted to the Unit at the factory Fig 9 3 Dimensions of Magnetic Sensor Orientation Card 9 4 2 Magnet J MG 1378BS Four 4 3 dia mounting holes Different...

Страница 156: ...mimusen TRC116 12A 10 7F Plug Tajimimusen Detail at A 36 18 6 5 1 40 50 60 1 4 5 2 7 22 16 4 SPC 0 5 t 12 18 2 5 4 10 10 37 5 37 5 95 A 25 13 1 2 M4 28 20 500 mm Unit mm Fig 9 6 Dimensions of FS 1378C...

Страница 157: ...iring distance is 20 m maxi mum 2 P indicates twisted pair cable Fig 9 8 Connection between Devices for FS 1378C J Using the FS 200A Orientation Card Position signal Green White Red Black 12 V SG 13 1...

Страница 158: ...nector 10114 3000VE 10314 52A0 008 case Notes 1 The terminal arrangement is viewed from the PCB connector 2 Connectors are manufactured by Sumitomo 3M Corporation J FS 1378C Magnetic Sensor F A B G E...

Страница 159: ...ed to the Magnet 1 60 to 70 mm 60 to 70 mm L Gap between center of Magnet and Magnetic Sensor 2 6 mm 6 to 8 mm 5 mm 3 to 7 mm L Gap between tip of Mag net and Magnetic Sen sor 2 1 to 2 mm 1 to 2 mm S1...

Страница 160: ...Sensor do not damage them The Magnet rotates at high speed so damage can result in unexpected accidents Also the Magnetic Sensor is a high precision device so if external force is applied resulting in...

Страница 161: ...on Control 9 12 Load shaft Magnetic Sensor head pin groove Magnet detection standard hole a MG 1378BS FS 1378C Load shaft FS 200 printed on the back b MG 1444S FS 200 Fig 9 15 Magnet and Magnetic Sens...

Страница 162: ...2047 pulses 000 to 7FF hexadecimal BCD Sign 1 bit to BCD Sign 1 bit Data 3 digits 11 bits to 799 to 799 decimal D When the sign bit is ON the sign is negative and when the sign bit is OFF the sign is...

Страница 163: ...ligned according to the servo loop which uses the motor encoder signal from the point that the load shaft Magnet passes the stop position and stops at the fixed position using the Magnetic Sensor sign...

Страница 164: ...EV Orientation signal ORT Orientation completion signal ORE Incremental signal INC Position signal for control Magnetic Sensor output Fixed position stop position Incremental operation 0 ABS 0 0 ON ON...

Страница 165: ...Does load shaft stop at home position Perform error diagnosis and correct error Select control constant display for load shaft home position C3 01 Set the home position data and press the ENTER Key S...

Страница 166: ...tuneup operation again Tuneup completed Bit 4 of C3 22 turned ON S Turn ON tuneup operation selection C3 22 bit 4 Adjust Control Constants Adjust Virtual Stop Position Offset C3 13 S Perform adjustmen...

Страница 167: ...rtional gain C3 04 Turn OFF orientation signal ORT Note If there is no L gear selection in the machine specifications omit the following L gear adjustment procedure Check L Gear Selection Interface in...

Страница 168: ...g the Digital Operator option The control constants includes user constants C1 op tional encoder orientation constants C2 and magnetic sensor orientation constants C3 10 1 User Constants 10 2 10 2 Enc...

Страница 169: ...ed i e MGR and LGR are OFF or when high speed winding is selected i CHW i OFF i d i SV i ON Yes ms 100 1000 C1 06 g Constant H VHS g p g i e CHW is OFF in servo mode i e SV is ON Torque reference I To...

Страница 170: ...During deceleration SDLVL SDHYS is detected Expressed as a percent of the motor rated speed C1 26 Yes 1 00 0 00 C1 23 Torque Detec tion Signal Op Torque detection signal TDET activation level used to...

Страница 171: ...30 C1 34 Servo Mode Base Speed Ra i M L Base speed ratio when low speed gear is selected i e MGR or LGR is ON or when low speed winding is selected i e CHW is ON in servo d i SV i ON No 1 00 5 00 C1...

Страница 172: ...rated output signal No 00000000 C1 39 Signal Selec tions 4 SEL4 4 Signal selections for control mode and level S Bit 0 Orientation control method 0 Encoder 1 Magnetic sensor When the setting has chan...

Страница 173: ...l after subtracting from SMOFS Yes 5 4 0 200 C1 54 2 nal Offset Ad justment Value SMOFS signal after subtracting from SMOFS Yes 5 4 mV 0 200 C1 55 2 Load Meter Sig nal Offset Ad Offset adjustment valu...

Страница 174: ...the C1 61 value listed on the terminal box No Pulse 7 100 C1 61 When the setting has changed turn the control power supply OFF then ON again No Pulse 7 0 1 Change change during operation column Indic...

Страница 175: ...is selected i e MGR or LGR is ON or when high speed winding is selected i e CHW i ON i i i l i ORT i ON Yes Hz 40 255 C2 07 p L KVLO g p g CHW is ON in orientation control i e ORT is ON Torque referen...

Страница 176: ...rotation direction S Bit 2 Selection for fixed rotation direction 0 Forward rotation of load shaft 1 Reverse rotation of load shaft S Bit 3 Stop position reference code 0 12 bit binary 1 3 digit BCD...

Страница 177: ...ation gain 00 10 01 15 No 10000000 C2 24 01 15 10 20 11 30 S Bits 7 and 6 Flux level for positioning servo loop control 00 100 01 80 10 60 11 40 No 10000000 1 Change change during operation column Ind...

Страница 178: ...ms 100 5 C3 07 Speed Control Pro portional Gain M L Speed control proportional gain when low speed gear is selected i e MGR or LGR is ON or when low speed winding is selected i CHW i ON i i i l i ORT...

Страница 179: ...15 mm 0 59 inches MG 1444S 7 mm 0 28 inches No Deg 5 0 5 0 C3 21 C3 21 C3 22 Orientation Control Signal Selections 1 SEL M1 2 Control mode setting signals e g for specifying the direction of rotation...

Страница 180: ...5 and 4 Speed reference differential compensation gain 00 10 01 15 10 20 11 30 No 10000000 C3 24 11 30 S Bits 7 and 6 Flux level for positioning servo loop control 00 100 01 80 10 60 11 40 No 1000000...

Страница 181: ...The operating status displays include Inverter operation U1 optional encoder orientation control U2 and magnetic sensor orien tation control U3 11 1 Inverter Operating Status 11 2 11 2 Encoder Orienta...

Страница 182: ...us Sequence output signal ON OFF status 2 ZSPD ORG AGR ORE SDET CHWE TDET FLT TLE TALM FC0 FC1 FC2 FC3 FLTL U1 11 Inverter capacity Inverter unit 30 minute rated capacity kW U1 12 Inverter internal te...

Страница 183: ...etion signal 2 ms Note The LED segments for ON I O signals will light 11 3 Magnetic Sensor Orientation Control Status The status displays for magnetic sensor orientation control are listed in the foll...

Страница 184: ...ed in the following table Table 11 4 Miscellaneous Operating Status Displays No Signal Name Explanation Unit U7 01 Motor temperature Detected temperature for motor overheat protection C U7 02 Slip fre...

Страница 185: ...nverter and Converter fault displays the faults caused by motor malfunctions and the corrective actions to be taken 12 1 Troubleshooting Outline 12 2 12 2 Converter Faults 12 3 12 3 Inverter Faults 12...

Страница 186: ...cannot solve the problem contact your YASKAWA representative immediately To restart turn ON the reset input signal of NC machine press the RESET Key or shut OFF the main circuit power supply once to r...

Страница 187: ...oltage detection lev el Check the control supply voltage Servo unit power supply fault Control supply voltage supplied to Ser vo Unit was not normal Check the control supply voltage Power supply fre q...

Страница 188: ...No Name Meaning Corrective Actions CPU built in A D error Built in A D Converter error ROM error Memory PROM error EEPROM error Memory EEPROM error Replace the control PCB CPU error CPU error Control...

Страница 189: ...ON again make sure that no short circuit or ground fault oc curs at the Inverter output FFFF Main circuit fuse blown DC circuit fuse was blown S Check for damage to transistor short circuits on load...

Страница 190: ...main circuit or other power lines S Check the control constants FFff Excessive speed devi ation Speed falls to less than 50 of refer ence value S Check if the load is heavy or a tool is jammed S Chec...

Страница 191: ...tive cooling FfFF Internal cool ing fan fault Inverter internal cooling fan is stopped Replace the internal cooling fan FfFF tuneup incomplete Encoder method orientation Orientation command was input...

Страница 192: ...ic sensor or magnetizer S Perform tuneup again Ffff INC signal er ror Magnetic sensor meth od orienta tion INC signal input timing error minor fault After carrying out absolute posi tioning change cir...

Страница 193: ...om mand error 1 YENET1200 communications com mand error undefined warning oc curred Communica tions com mand error 2 YENET1200 communications com mand error orientation tuneup dis abled Communica tion...

Страница 194: ...l origin S Check the wiring of the C phase signal of the encoder S Replace the encoder or motor S Replace the control card fFff Encoder Pulse Number Error For IPM mo tors only The encoder pulse number...

Страница 195: ...56 fffF Motor code unrecorded Motor code set in C1 25 is not re corded S Check motor model and motor code C1 25 S Check setting list for correct PROM version of motor code C1 25 fffF Constant set ting...

Страница 196: ...Control cir cuit fault 2 operator transmission error Transmission between the Inverter and the Digital Operator is estab lished once after supplying power but later transmission fault contin ues for m...

Страница 197: ...ken bearing S Check motor shaft rotation manually S Replace the motor Control PCB fault Replace the control PCB M t d t t t Power of Converter s main circuit is not turn ON S Turn ON power supply S Ch...

Страница 198: ...trol PCB fault Replace the control PCB Inverter output disconnection Check wiring between Inverter and motor Grounding error of motor or Inverter Check continuity of motor and Inverter to see if they...

Страница 199: ...ay U1 10 Fault of Orientation Card or control PCB Replace the Orientation Card or the control PCB Incorrect setting of stop position reference Check whether the position reference is correct on operat...

Страница 200: ...y the machine data for transmission ratio values C1 27 to 29 Orientation completion signal is not output Position control proportional gain is high S Confirm that no vibration occurs in the forward an...

Страница 201: ...chapter describes basic maintenance and inspection procedures for the VS 626M5 and the VS 656MR5 13 1 Maintenance and Inspection 13 3 13 1 1 Daily Inspections 13 3 13 1 2 Periodic Inspections 13 3 13...

Страница 202: ...after the main circuit power supply and control power supply have been turned OFF The capacitors are still charged and can be dangerous D Only authorized personnel should be permitted to perform maint...

Страница 203: ...on nectors etc Loose connectors Tighten Heatsink Build up of dust and dirt Blow with dry compressed air of 39 2 104 to 58 8 104 Pa 4 to 6kg cm2 pressure Inverter and Converter Printed circuit boards A...

Страница 204: ...wn in the following table Refer to the table for parts replacement and maintenance Table 13 2 Parts Replacement Schedule Area Parts Interval Approx Remarks Cooling fan 2 to 3 years Replace with new on...

Страница 205: ...Torque Speed Characteristics 14 19 14 2 4 Dimensions 14 30 14 2 5 Tolerance Radial Loads 14 37 14 2 6 Motor Total Indicator Readings 14 37 14 2 7 Encoders 14 38 14 2 8 Encoder Connector 14 39 14 2 9 S...

Страница 206: ...6 182 3 249 1 Motor Output Torque at Base Speed Con tinuous Rating lb ft kgf m 10 4 1 43 17 4 2 40 25 8 3 57 35 8 4 87 51 7 7 14 70 6 9 74 86 9 12 0 134 18 6 183 25 4 Rotor Inertia J GD2 4 lb ft2 kg m...

Страница 207: ...tage fluctuation 10 to 15 allowable frequency fluctuation 5 Required power capacity 100 VA for independent drive system 250 VA for NC system C Co Control Method Power regenerative control 120_ current...

Страница 208: ...98 Control Method Sine wave PWM Inverter Vector control ter Speed Control Range 40 min 1 to maximum motor speed verte Speed Regulation 0 2 maximum speed or less Inv Overload Capacity 3 120 60s of 30...

Страница 209: ...s guaranteed when input voltage is three phase 400 V 50 60 Hz 440 V 50 60 Hz 460 V 60Hz If input voltage is lower than 400 V rated output power is not guaranteed 2 A For stand alone drive system N for...

Страница 210: ...614 0 068 1 970 0 083 2 563 0 108 6 146 0 259 11 22 0 473 13 00 0 548 13 00 0 548 Overload Capacity 120 60s of 30 minute rating Vibration m V5 V10 Noise Level 75dB A or less 80dB A or less Ambient Te...

Страница 211: ...ght 13 78 350 mm 3 Depth 12 60 320 Power Supply AC Reactor Code No X010058 X010059 X010060 X010061 X010062 X010063 X010064 Model CIMR M5j 2 CIMR MR5j 2 25P5 27P5 2011 2015 2018 2022 2030 Ambient Tempe...

Страница 212: ...ed Control Range 40 min 1 to maximum motor speed verter Speed Regulation 0 2 maximum speed or less Inve Overload Capacity 120 60s of 30 minute rating I Approx Mass lb kg 11 5 27 12 35 16 Applicable Ma...

Страница 213: ...s2 0 2G at 20 to 50 Hz Protective Structure IEC IP00 Protected so that parts of the human body cannot reach electrically charged parts from the front 1 UAASKB 30FZ E 30 20 kW is 20 minutes 50 ED Cont...

Страница 214: ...50 13 78 320 12 6 100 3 94 330 12 99 10 0 39 190 7 48 130 5 12 12 26 M5 class 2018 5 91 13 78 12 6 3 94 12 99 0 39 7 48 5 12 26 2022 2030 200 7 87 350 13 78 320 12 6 150 5 91 330 12 99 10 0 39 190 7 4...

Страница 215: ...5 M5 class 2018 5 91 18 5 12 76 3 94 17 91 0 26 16 5 2022 16 5 36 2030 200 7 87 470 18 5 324 12 76 150 5 91 455 17 91 6 5 0 26 21 5 47 M5 2037 300 11 81 470 18 50 324 12 76 250 9 84 455 17 91 7 0 28 3...

Страница 216: ...30 12 99 10 0 39 190 7 48 130 5 12 12 26 M5 class 2018 5 91 13 78 12 6 3 94 12 99 0 39 7 48 5 12 26 2022 2030 200 7 87 350 13 78 320 12 6 150 5 91 330 12 99 10 0 39 190 7 48 130 5 12 16 35 M5 2037 300...

Страница 217: ...6 5 0 26 16 5 M5 class 2018 5 91 18 5 12 76 3 94 17 91 0 26 16 5 2022 16 5 36 2030 200 7 87 470 18 5 324 12 76 150 5 91 455 17 91 6 5 0 26 21 5 47 M5 2037 300 11 81 470 18 50 324 12 76 250 9 84 455 1...

Страница 218: ...10 0 39 18 0 71 28 1 10 300 11 8 22 0 87 M5 0 24 dia class 2030 199 7 83 150 5 91 24 5 0 96 4 5 0 18 20 0 79 20 0 79 190 7 48 350 13 8 330 13 0 10 0 39 18 0 71 28 1 10 300 11 8 22 0 87 M5 0 24 dia 203...

Страница 219: ...tinu ous 30 min ute Con tinu ous 30 min ute Con tinu ous 30 min ute Con tinu ous 30 min ute Con tinu ous 30 min ute Con tinu ous 30 min ute Con tinu ous 30 min ute Con tinu ous 30 min ute Open Chassi...

Страница 220: ...tinu ous 30 min ute Con tinu ous 30 min ute Con tinu ous 30 min ute Con tinu ous 30 min ute Con tinu ous 30 min ute Open Chassis Type Total calorific value W 58 73 76 88 117 138 185 209 193 210 233 2...

Страница 221: ...transfer contact structure specialized for winding selection are provided For detailed specifications of the magnetic contactor refer to 14 3 3 Mag netic Contactor Specifications for Winding Selection...

Страница 222: ...le Table 14 13 Common Motor Specifications Isolation F type Cooling Fan Fan motor Thermostat auto resetting 3 phase 200 V 50 60 Hz 220 V 50 60 Hz 230 V 60 Hz 400 V 50 60 Hz 440 V 50 60 Hz 460 V 60 Hz...

Страница 223: ...kW 200 V Series 1500 0 2 4 8000 kgf m N m 0 0 14 0 23 5 28 3 1 43 2 40 2 88 1500 8000 4 4 kW 1 min rating 3 7 kW 15 min rating 50 ED 2 2 kW Continuous rating Motor speed min 1 Output kW 1 min rating...

Страница 224: ...kW 200 V and 400 V Series 1500 0 4 8 12 6000 1500 0 0 47 7 70 0 84 0 4 87 7 14 8 57 6000 Output kW 13 2 kW 1 min rating 11 kW 30 min rating 50 ED 7 5 kW Continuous rating Motor speed min 1 Torque Moto...

Страница 225: ...V Series 1500 0 8 24 16 6000 1500 6000 0 12 0 14 3 17 1 0 117 6 140 0 168 1 26 4 kW 1 min rating 22 kW 30 min rating 50 ED 18 5 kW Continuous rating Motor speed min 1 Output kW 30 min rating 50 ED 1...

Страница 226: ...eed min 1 Output kW Motor speed min 1 Continuous rating 30 min rating 50 ED 1 min rating Torque kgf m N m 45 37 kW 400 V Series 1150 54 45 37 4500 3800 26 kW 36 kW 30 kW 1150 0 306 8 373 7 448 4 4500...

Страница 227: ...ng 50 ED 3 7 kW Continuous rating Motor speed min 1 Low speed winding output kW 1 min rating Continuous rating Low speed winding 30 min rating 50 ED Torque Motor speed min 1 kgf m N m 0 2 4 6 8 6000 1...

Страница 228: ...r speed min 1 Low speed winding output kW 1 min rating Continuous rating 30 min rating 50 ED Torque Motor speed min 1 Low speed winding kgf m N m 0 2 4 6 8 10 1500 6000 6000 0 0 1500 57 3 47 7 35 0 5...

Страница 229: ...Low speed winding output kW 1 min rating Continuous rating 30 min rating 50 ED Torque Motor speed min 1 Low speed winding kgf m N m 1500 6000 0 2 4 6 8 10 12 14 6000 0 0 47 7 70 0 84 0 4 87 7 14 8 57...

Страница 230: ...or speed min 1 Low speed winding output kW 1 min rating Continuous rating Low speed winding 30 min rating 50 ED Torque Motor speed min 1 kgf m N m 4800 0 4 12 16 8 20 1000 4800 0 0 105 143 172 10 7 14...

Страница 231: ...1 Low speed winding output kW Torque Motor rating min 1 Continuous rating Low speed winding 30 min rating 50 ED 1 min rating kgf m N m 1000 0 5 15 20 10 4800 0 0 143 177 212 14 6 18 0 21 6 1000 4800 H...

Страница 232: ...or speed min 1 Low speed winding output kW Torque 1 min rating 20 min rating 50 ED Continuous rating Low speed winding Motor speed min 1 kgf m N m 4800 0 5 15 20 10 1000 25 4800 0 0 177 210 252 18 0 2...

Страница 233: ...20 min rating 50 ED Continuous rating Low speed winding Motor speed min 1 kgf m N m 4000 0 30 20 10 1000 31 2 kW 26 kW 4800 4000 0 0 191 286 344 19 5 29 2 35 1 1000 4800 36 kW 1 min rating 30 kW 2 min...

Страница 234: ...204 8 03 11 7 5 321 12 6 156 6 14 112 4 41 204 8 03 95 3 74 89 3 5 10 0 39 269 10 6 75 2 95 42 5 1 67 590 23 2 220 8 66 215 8 46 269 10 6 70 2 76 12 0 47 204 8 03 15 11 261 10 3 196 7 72 160 6 3 260...

Страница 235: ...M5 18 5 15 110 4 33 90 3 54 1 0 039 0 0 016 48 9 0 35 5 5 0 22 14 0 55 40 1 57 M5 22 18 5 110 4 33 90 3 54 1 0 039 0 030 0 011 55 10 0 39 6 0 24 16 0 63 45 1 77 M5 30 22 140 5 51 110 4 33 2 0 079 0 0...

Страница 236: ...6 23 5 215 8 46 0 0 04 180 204 8 03 16 0 63 250 9 84 486 19 1 110 4 33 15 0 59 204 8 03 270 10 6 42 5 1 67 157 6 18 204 8 03 15 11 568 22 4 265 10 4 0 0 046 230 250 9 84 20 0 79 300 11 8 458 18 110 4...

Страница 237: ...M5 18 5 15 110 4 33 90 3 54 1 0 039 0 0 016 48 9 0 35 5 5 0 22 14 0 55 40 1 57 M5 22 18 5 110 4 33 90 3 54 1 0 039 0 030 0 011 55 10 0 39 6 0 24 16 0 63 45 1 77 M5 30 22 140 5 51 110 4 33 2 0 079 0 0...

Страница 238: ...19 9 75 2 95 61 2 4 822 5 32 4 420 16 5 425 16 7 444 5 17 5 149 5 87 24 0 94 385 15 2 22 18 5 409 16 1 321 12 6 225 8 86 380 15 178 7 01 174 5 6 87 21 0 83 505 19 9 75 2 95 61 2 4 872 5 34 4 420 16 5...

Страница 239: ...0 4 33 15 0 59 260 10 2 343 13 5 42 5 1 67 182 5 7 19 250 9 84 11 7 5 668 26 3 265 10 4 0 0 046 230 250 9 84 20 0 79 300 11 8 558 22 110 4 33 15 0 59 260 10 2 343 13 5 42 5 1 67 182 5 7 19 250 9 84 15...

Страница 240: ...011 55 10 0 39 6 0 24 16 0 63 45 1 77 M5 15 11 140 5 51 110 4 33 2 0 079 0 030 0 011 60 11 0 43 7 0 28 18 0 71 50 1 97 M6 18 5 15 140 5 51 110 4 33 1 0 039 0 030 0 011 70 12 0 47 7 5 0 3 20 0 79 60 2...

Страница 241: ...g tables J Foot mounted Motors Table 14 15 Leg mounted Motor TIR Item Standard Motor Winding Selection Motor Accuracy Degree of Shaft Paral lax 7 5 5 5 kW max 0 03 lax 11 7 5 to 22 18 5 kW 5 5 3 7 to...

Страница 242: ...l resolution is the same as the main axis encoder and in machinery where the motor axis and main axis correspond 1 1 the motor encoder can be used as the main axis encoder The relationship between the...

Страница 243: ...s Fig 14 13 Encoder Signal Connector Terminal Arrangement J Dimensions Receptacle housing ELR 12V 24 6 14 8 Plug housing ELP 12V 24 6 14 8 14 6 Pin contacts LLM 01T 1 3E 11 pins pins 1 to 9 11 and 12...

Страница 244: ...is UAASKJ 37FZ 3 When specifying the Cooling Fan Motor also specify the casing The casing must be replaced at the same time as the cooling fan motor Table 14 18 400 V Series Spare Motor Parts Stan da...

Страница 245: ...emove the cooling fan lead cables inside the terminal box and pull out the lead cables from the lead cable vent 3 Remove the four screws used to mount the casing to the motor unit and the screws withi...

Страница 246: ...M6 10 0 39 7 0 28 M8 8 17 6 90 2030 160 0 07 X010 064 3 210 8 27 100 3 94 210 8 27 175 5 6 89 0 2 110 4 33 75 2 95 80 3 15 205 8 07 2 3 0 09 M6 10 0 39 7 0 28 M10 12 26 5 100 2037 200 0 05 X010 120 3...

Страница 247: ...of Mounting Bolts I B1 U X V Y W Z U X V Y W Z E H B B2 I B1 L K C2 D F A C1 Fig 1 Fig 2 L K C2 D F A C1 B1 X Y Z U V W E H B I A1 Fig 3 Details of Mounting Bolts Nameplate 6 M Terminals 6 M Terminals...

Страница 248: ...7 25 0 98 M6 10 0 39 7 0 28 M10 12 26 5 100 2037 200 0 05 X002557 210 8 27 115 4 53 182 8 7 2 175 6 89 75 2 95 95 3 74 205 8 07 25 0 98 M6 10 0 39 7 0 28 M10 15 0 59 110 400 V Class Model Cur Induc Fi...

Страница 249: ...A C X Y Z U V W E H B 4 5 F D K B1 L A C X Y Z U V W E H B 4 5 F D K Nameplate 6 M Terminals Details of Mounting Bolts Fig 1 Fig 2 Details of Mounting Bolts Nameplate 6 M Terminals 4 J Mounting Bolts...

Страница 250: ...and MC from the table below according to Converter VS 656MR5 model Voltage Class Converter Model Power Capacity MCCB Rated Current A MC Rated Current A Class Model CIMR MR5 Capacity kVA 1MCCB 2MCCB 3...

Страница 251: ...kg 11 lb Ambient Temperature 10 to 55 C 14 to 131 F Humidity 10 to 95 non condensing Applicable Inverter 200 V class 5 HP to 20 HP 3 7 kW to 15 kW 25 HP to 40 HP 18 5 kW to 30 kW 50 HP 37 kW Applicab...

Страница 252: ...4 5 6 Main contact 3NC Open Closed 7 8 9 10 11 12 Main contact 3NO Closed Open 15 16 Auxiliary contact 1NO Open Closed 17 18 200 V power supply 1 2 7 8 3 4 9 10 5 6 11 12 a HV AP4 Standard 1 2 7 8 3...

Страница 253: ...When mounting the Busbar use only the screws supplied Otherwise mounting may be incorrect J Selecting Busbar and Cable Kits Refer to the following table when selecting Busbar and Cable Kits Table 14 2...

Страница 254: ...5 72626 W100100 47P5 4011 72626 W100150 45P5 4015 45P5 47P5 4018 47P5 4022 4030 72626 W100250 4037 4045 45P5 72626 W150100 47P5 4011 72626 W150150 4011 4015 4015 4015 4018 4018 4018 4022 4022 4022 403...

Страница 255: ...5 8 76 2 280 11 1 150 5 91 1 72626 W100300 4 317 5 12 5 122 5 4 82 98 5 3 88 2 330 13 1 150 5 91 1 72626 W150100 2 147 5 5 81 102 5 4 04 2 100 3 94 1 250 9 84 1 72626 W150150 3 195 7 68 125 4 92 2 180...

Страница 256: ...ube Width 3 mm Busbar Cutaway Diagram 3 10 L1 47 L 10 15 47 25 25 Two 7x12 Oblong holes Insulation tube Width 3 mm Busbar Cutaway Diagram 4 L1 47 L 15 25 25 10 125 10 101 49 L2 22 Two 7x12 Oblong hole...

Страница 257: ...bar 1 Turn OFF the power supply to the Converter and wait at least 5 minutes until the CHARGE indicator is no longer lit before opening the upper cover 2 Remove the Converter and Inverter power supply...

Страница 258: ...ing protection function operations can be performed by mounting an optional JVOP 132 Digital Operator to the Inverter J Digital Operator and Extension Cables A Digital Operator Extension Cable 72616 W...

Страница 259: ...24 Mounting Method Features Item Method 1 Method 2 1 Method 3 1 Remarks Ease of mounting Extent of panel Excellent Good Some difficulty Extent of panel manipulation 3 screw holes 3 screw holes 5 scre...

Страница 260: ...panel 1 54 0 63 18 8 0 74 LOCAL REMOTE DIGITAL OPERATOR JVOP 132 DSPL DATA ENTER FWD REV RESET RUN STOP DRIVE PRGM JOG DRIVE FWD REV REMOTE REF SEQ 140 5 51 70 2 76 140 5 51 70 2 76 Fig 14 21 Mounting...

Страница 261: ...nd by drilling mounting holes and making a cutout in the panel When attaching the cable to the resin part open the cable connector cover fully and insert the connector until the cable audibly snaps in...

Страница 262: ...E 10350 52A0 008 1 6CN Card 14 10114 3000VE 10314 52A0 008 1 9CN 72626 CA03 CIMR M5A MDR 36 10136 3000VE 10336 52A0 008 1 1CN M5A M5A Magnet 20 10120 3000VE 10320 52A0 008 1 2CN M5A Magnet ic Sensor O...

Страница 263: ...Control connector 1CN MDR 36P 2CN MDR 20P 6CN MDR 50P 72626 CA01 1CN MDR 36P 2CN MDR 20P 6CN MDR 50P 8CN MDR 20P 9CN MDR 14P 1CN MDR 36P 2CN MDR 20P 6CN MDR 50P 10CN MDR 14P 1CN MDR 36P 2CN MDR 20P 1...

Страница 264: ...36 7 5 0 3 5 1 0 2 2 3 0 091 2 9 0 11 6 6 0 26 19 3 0 76 15 Unit mm inches No of Poles Product Code A B C Relevant Connector Number Manufacturer 14 10114 3000VE 7 62 0 3 13 8 0 54 18 2 0 72 9CN or 10...

Страница 265: ...0 0 28 12 0 0 47 10 0 0 39 12 7 0 5 5 2 0 2 23 8 0 94 39 0 1 54 14 0 0 55 8 0 0 31 17 0 0 67 14 0 0 55 39 0 1 54 23 8 0 94 5 2 0 2 12 7 0 5 No of Poles Product Code A B C Relevant Connector Number Man...

Страница 266: ...e Filters Input Reduce radio noise by using a filter that suppresses transmission of high frequency noise generated by the Inverter to the power supply If used in a location where magnetic field stren...

Страница 267: ...07 1 55 2011 FN258L 75 34 FIL001067 1 75 FN258 75 34 1 75 2015 FN258L 100 35 FIL001068 1 100 FN258 100 35 1 100 2018 FN258L 100 35 FIL001068 1 100 FN258 100 35 1 100 2022 FN258L 130 35 FIL001069 1 130...

Страница 268: ...L001067 1 75 FN258 75 34 1 75 4030 FN258L 100 35 FIL001068 1 100 FN258 100 35 1 100 4037 FN258L 130 35 FIL001069 1 130 FN258 130 35 1 130 4045 FN258L 130 35 FIL001069 1 130 FN258 130 35 1 130 A For st...

Страница 269: ...1 2 15 7 0 047 414 16 3 80 3 15 6 5 0 26 3 0 12 M10 7 5 16 53 FN258L 180 07 3 438 1 5 17 2 0 059 240 9 45 110 0 8 4 33 0 031 400 1 2 15 7 0 047 413 16 3 80 3 15 6 5 0 26 500 19 7 4 0 16 15 0 59 M10 5...

Страница 270: ...A H C L F J O G B D E A C J O F B D Diagram 1 Diagram 2 P E A H C F J O G B D 516 1 5 20 3 0 059 45 5 564 1 5 27 0 2 100 0 5 M5 8 64 1 2 52 0 039 9 0 2 210 0 5 210 0 5 64 2 3 0 2 60 0 5 40 60 0 3 220...

Страница 271: ...8 27 95 3 74 33 1 3 10 0 39 13 0 51 1 6 3 53 LNFD 4203HY 72600 D4203HY 270 10 6 155 6 1 125 4 92 240 9 45 125 4 92 43 1 69 9 0 35 11 0 43 2 2 4 85 LNFD 4303HY 72600 D4303HY 270 10 6 155 6 1 125 4 92...

Страница 272: ...is turned ON and OFF can affect the Inverter control signal wires J Specifications and Model Numbers The surge absorber specifications and model numbers are shown in the following table Device Surge...

Страница 273: ...Capacity 15 11 15 3 2 Acceleration deceleration Capacity 15 15 15 3 3 Calculating Start and Stop Times 15 17 15 3 4 Intermittent Load Operating Capacity 15 18 15 4 Interface Design 15 19 15 4 1 Seque...

Страница 274: ...wave AC voltage can be obtained as the output as shown in Fig 15 2 The frequency of the AC output voltage is proportional to the speed at which the switches are turned ONand OFF in other words inverse...

Страница 275: ...squirrel cage induction motor characteristics are contrasted with the DC motor and the principle of torque generation is explained below Fig 15 5 shows the model diagrams for each from the direction o...

Страница 276: ...the stator due to the effects of electromagnetic induction and interlinkage between the magnetic flux of the rotation magnetic field and the stator winding E1 k 1 m 2 kf1 m Both tips of the rotator co...

Страница 277: ...magnetized current Im as per the set value inside the motor and performs control so that the required torque is generated The VS 626M5 control block is shown in Fig 15 6 The speed controller performs...

Страница 278: ...ases and decreases proportional to the shifting gears so taking motor axis speed NM min 1 and load axis speed NL min 1 the motor axis calcu lated torque TM NSm can be expressed using the following for...

Страница 279: ...ertial Moment InertialmomentJ1 duringrotationalong an axiscentered onthe massm1 kg andradius r1 m of acylinder can be expressed using the following formula J1 m1r1 2 2 kg m2 r1 m L m Mass m1 kg Fig 15...

Страница 280: ...n and example b shows vertical operation Both horizontal and vertical inertial mo ments JH and JV take the full load mass mL kg counterweight mass mC kg and ball screw reed PB and can be expressed usi...

Страница 281: ...ation axis passes through the center of gravity Right angle box D2 b2 c2 3 b c Cylinder D2 L2 3 D0 2 4 D0 L Sphere D2 2 5 D0 2 D 0 Hollow sphere D2 2 5 D0 5 D1 5 D0 3 D1 3 D0 D1 Cone D2 3 10 D0 2 D 0...

Страница 282: ...I Unit Conversion Force and Load kgf N 1 kgf 9 80665 N Weight kgf The metric unit for weight and the SI unit for mass are the same Mass expressed us ing SI units for bodies in the metric mea t f i ht...

Страница 283: ...f calculating load drive capacity that are described are typical examples Table 15 3 Typical Load Torque Speed Characteristics Load Characteristics Load Examples Speed Torque Characteristics Motor Cap...

Страница 284: ...nst it to perform the cutting operation so the motive force PLC required to cut can be ex pressed using the following equation PC KS dLV 60 1000 C dLV SC C kW V DNs 1000 m min KS Cutting resistance N...

Страница 285: ...7 to 0 85 D Drill radius mm f Feed speed mm min SD Cutting efficiency i e cutting amount per 1 kW per minute CC kW min D f Fig 15 16 Example of Drill Processing J Gravity Load Drive The motive force r...

Страница 286: ...ments of conveyers such as crane movement and table drives are friction loads The motive force PF required taking the friction coefficient to be can be expressed using the following formu la PF umL V...

Страница 287: ...speed min 1 Table 15 4 Acceleration Formulas Acceleration Method Control Method Explanatory Diagram Remarks Current limited Accel eration This method suppresses the current during accel eration to a f...

Страница 288: ...speed NB 1 500 min 1 kW 0 0 1500 6000 min 1 Max output 120 30 min rating Output Maximum Output During Acceleration de celeration 120 of 30 min rated output J Calculations In the example shown in Tabl...

Страница 289: ...stics NB NM NMAX NB NM Acceleration deceleration Time t 2 60 JM 1 TM NM 2 N2 B 2NB t 2 60 2 JM 1000 PM NM 2 NB 2 2 Fixed Torque Fixed Output Characteris tics NB NM NMAX 0 NM Acceleration deceleration...

Страница 290: ...motor equivalence efficiency torque TR is less than the inverter continuous rated torque The maximum value of TP will be 120 of the Inverter 30 min rating TR N m TP 2 tr tf TL 2 ts tC Motor torque Mo...

Страница 291: ...the 24 V common or the external common which uses a separate 24 V power supply 20 to 26 V The wiring differs depending on the input method so refer to Fig 15 21 to perform the wiring correctly Also th...

Страница 292: ...VCOM 3 3 k 390 a 0 V Common EXTCOM 24VCOM 0VCOM 3 3 k 390 b 24 V Common EXTCOM 24VCOM 0VCOM 3 3 k 390 0 V or 24 V 24 V or 0 V c External Common Signal Name Pin Number 1CN 6CN EXTCOM 31 19 20 21 24VCOM...

Страница 293: ...y so that multi step speed operations can also be used The digital speed reference 1CN input circuit is the same as the se quence input signal 6CN The signal circuit common can be selectedindependentl...

Страница 294: ...Signals M5N NC Drive The YENET1200 communications system for Yaskawa NC Units sends and receives data such as speed references sequence I O signals motor speed and failure information usingserial comm...

Страница 295: ...ximum control pan el ambient temperature to be 40 C 103 F Consequently heat caused by the generated loss ofthe Invertermust be discharged using forced air currents and a heat exchanger For the calorif...

Страница 296: ...H x D 0 31 HEATEX02 250 W 10 C 50 F 440 x 924 x 50 17 3 x 36 4 x 1 97 W x H x D 0 45 The calorific content of the Cooling Ability column is the calorific tolerance when the internal air tempera ture...

Страница 297: ...ing example shows independent operation using a Digital Operator Fixed position stop control using RUN stop and a motor encoder is possible so this operation is useful for test operations As shown in...

Страница 298: ...12 RDY EMG FWD REV TLH 13 14 5 15 16 17 18 RST CHW DAS PPI ORT LGR MGR 3 4 2 33 34 35 SCOM 0V SS ZSPD 36 37 38 39 40 42 TDET TLE ORG ORE CHWE COM1 SDET AGR 43 44 45 FLT 26 27 28 29 FC0 FC1 FC2 FC3 30...

Страница 299: ...PLC PLC 16 17 18 19 14 15 P P P PAO PAO PBO PBO PLCO PLCO 13 14 15 16 11 12 P P P SS 17 1CN TLL INC SSC SV 19 20 21 22 23 24 25 EXTCOM0 2 24VCOM 0VCOM Output Module 6CN 6 7 8 9 10 11 12 RDY FWD REV TL...

Страница 300: ...SS 8 7 9 1 2 3 4 5 6 7 8 9 11 12 10 PG TS P PA PA PB PB PLC PLC 16 17 18 19 14 15 P P P TLL INC SSC SV 19 20 21 22 23 24 25 EXTCOM0 2 24VCOM 0VCOM Output Module 3 6CN 6 7 8 9 10 11 12 RDY EMG FWD REV...

Страница 301: ...points EEPROM Constants Motor encoder signal outputs Speedometer output 10 V Load meter output 10 V PG pulse proces sor DIO 12 bit digital references 12 points 1CN 2CN TS 3CN Error codes 4 points I O...

Страница 302: ...eleration Fre quency times min Acceleration de celeration time 0 Top s Speedometer 1 Not used 2 Used Speedometer full scale min 1 Load Meter 1 Not used 2 Used Load meter full scale Over continuous rat...

Страница 303: ...heatsink cooling without fan Connection Diagram Remarks J Converter Specifications Model CIMR MR5 Servo Control Power Supply 1 Without power supply MR5A 2 With power supply MR5N Mounting Configuration...

Страница 304: ...Appendix 15 32 J Remarks If using magnetic sensor orientation enter the mounting radius of the magnetic Magnet R R mm Magnetic emitter 1 MG 1378BS 2 MG1444S Magnetic sensor 1 FS 1378C 2 FS 200A 15...

Страница 305: ...e 55 11 5071 2552 Fax 55 11 5581 8795 YASKAWA ELECTRIC EUROPE GmbH Am Kronberger Hang 2 65824 Schwalbach Germany Phone 49 6196 569 300 Fax 49 6196 569 312 YASKAWA ELECTRIC UK LTD 1 Hunt Hill Orchardto...

Результаты поиска

Содержание CIMR-M5 Series

Отзывы:

Похожие инструкции для CIMR-M5 Series

Бренды по названию

Популярные бренды

YASKAWA CIMR-M5 Series, Инструкция по эксплуатации

Результаты поиска

Содержание CIMR-M5 Series

Отзывы:

Похожие инструкции для CIMR-M5 Series

Бренды по названию

Популярные бренды