VNA Concepts and Features
R&S
®
ZNL/ZNLE
216
User Manual 1178.5966.02 ─ 07
From this equation, it is easy to relate the real and imaginary components of the com-
plex admittance to the real and imaginary parts of
Γ
:
2
2
2
2
0
)
Im(
)
Re(
1
)
Im(
)
Re(
1
)
/
Re(
Y
Y
G
,
)
Im(
)
Re(
1
)
Im(
2
)
/
Im(
2
2
0
Y
Y
B
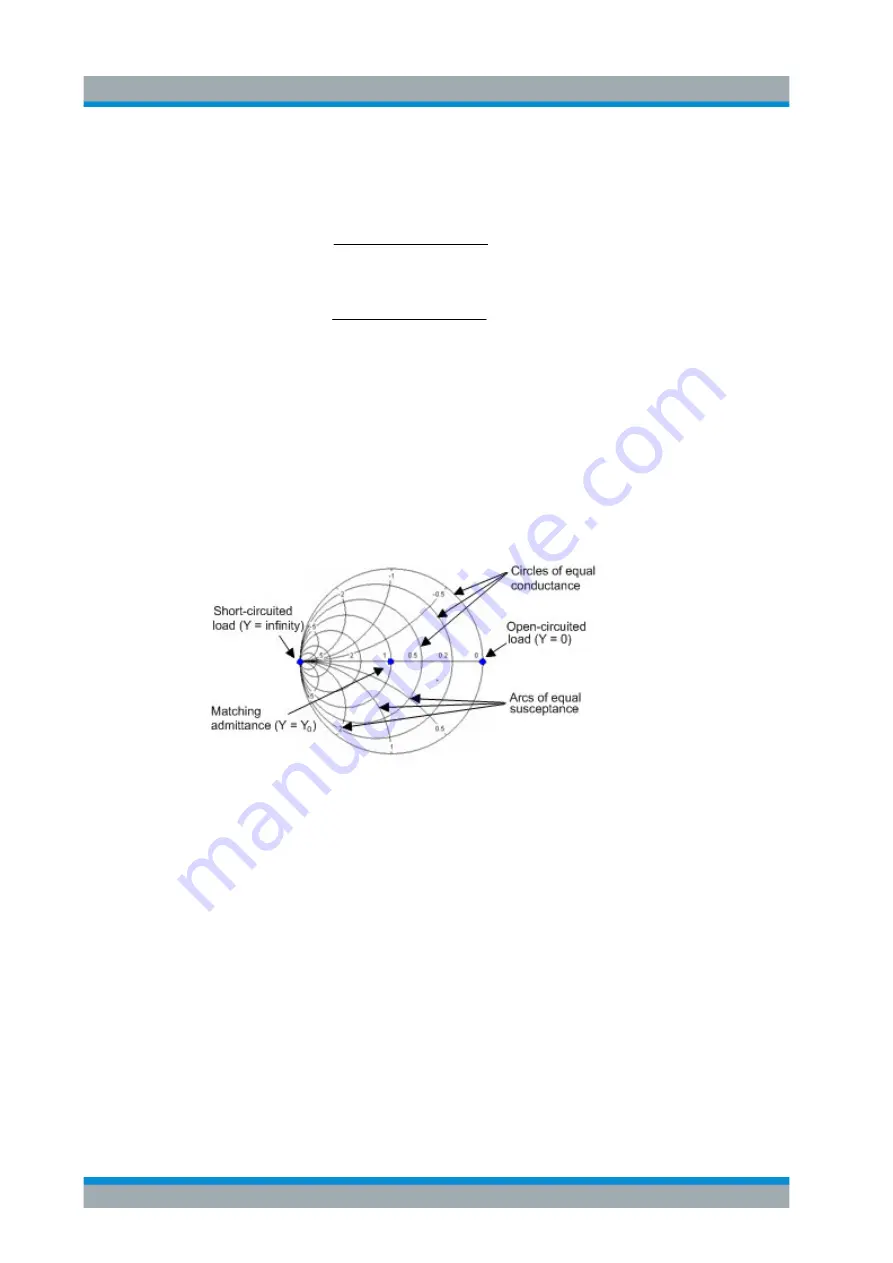
According to the two equations above, the graphical representation in an inverted
Smith chart has the following properties:
●
Real reflection coefficients are mapped to real admittances (conductances).
●
The center of the
Γ
plane (
Γ
= 0) is mapped to the reference admittance Y
0
,
whereas the circle with |
Γ
| = 1 is mapped to the imaginary axis of the Y plane.
●
The circles for the points of equal conductance are centered on the real axis and
intersect at Y = infinity. The arcs for the points of equal susceptance also belong to
circles intersecting at Y = infinity (short circuit point (–1, 0)), centered on a straight
vertical line.
Examples for special points in the inverted Smith chart:
●
The magnitude of the reflection coefficient of a short circuit (Y = infinity, U = 0) is
one, its phase is –180 deg.
●
The magnitude of the reflection coefficient of an open circuit (Y = 0, I = 0) is one, its
phase is zero.
8.2.3.3
Measured Quantities and Trace Formats
The analyzer allows any combination of a display format and a measured quantity. The
following rules can help to avoid inappropriate formats and find the format that is ide-
ally suited to the measurement task.
●
All formats are suitable for the analysis of reflection coefficients S
ii
. The formats
"SWR" , "Smith" and "Inv Smith" lose their original meaning (standing wave ratio,
normalized impedance or admittance) if they are used for transmission S-parame-
ters, ratios and other quantities.
VNA Screen Elements
www.allice.de
Allice Messtechnik GmbH






























