Mandik FDMD, Руководство пользователя

Страница: 29 / 36

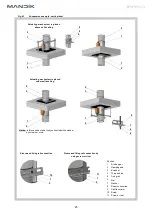
28
TPM 092/13
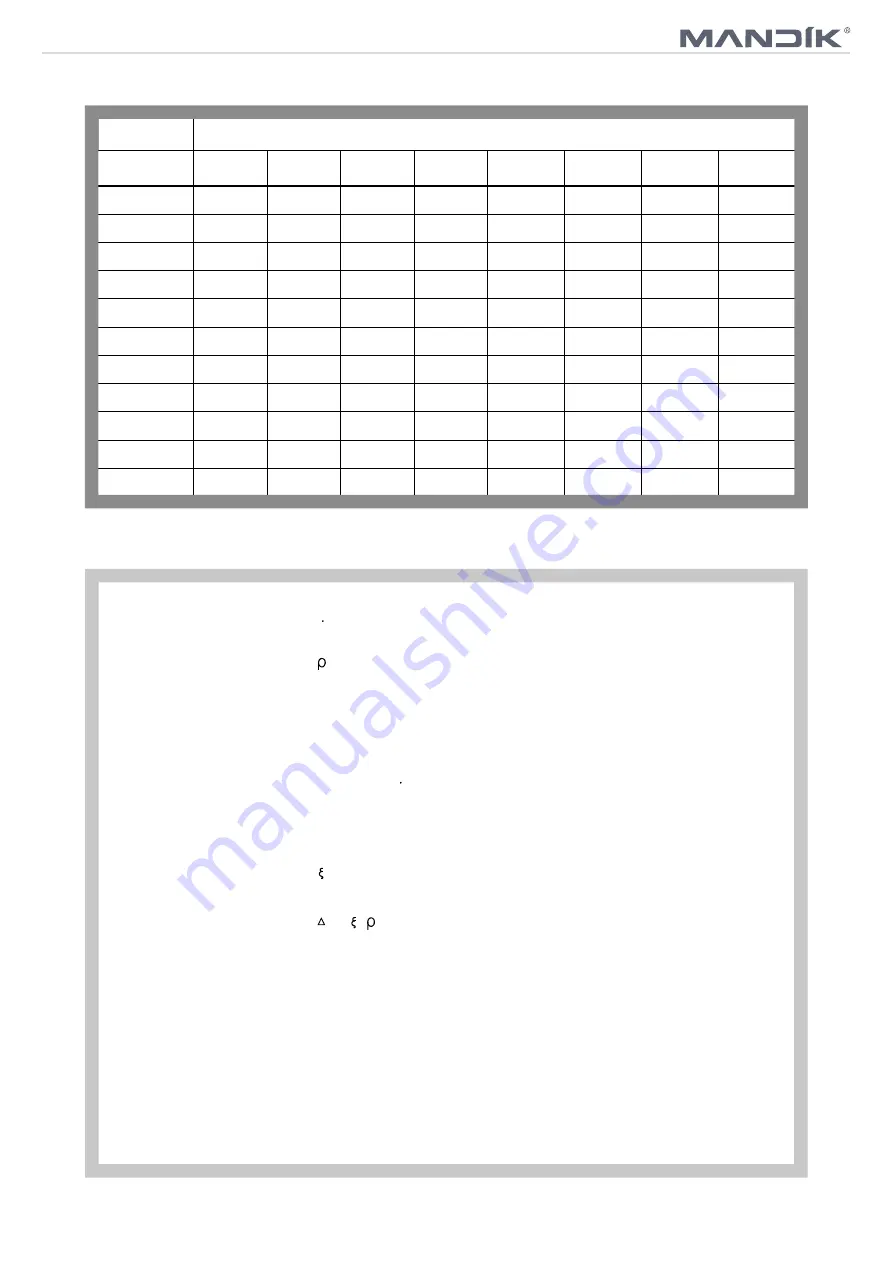
Tab. 10.3.3. Relative level expressing the shape of the spectrum L
rel
f [Hz]
w [m.s ] 63 125 250 500 1000 2000 4000 8000
2
-4,5 -6,9 -10,9 -16,7 -24,1 -33,2 -43,9 -56,4
3
-3,9 -5,3 -8,4 -13,1 -19,5 -27,6 -37,4 -48,9
4 -3,9 -4,5 -6,9 -10,9 -16,7 -24,1 -33,2 -43,9
5 -4 -4,1 -5,9 -9,4 -14,6 -21,5 -30 -40,3
6 -4,2 -3,9 -5,3 -8,4 -13,1 -19,5 -27,6 -37,4
7 -4,5 -3,9 -4,9 -7,5 -11,9 -17,9 -25,7 -35,1
8
-4,9 -3,9 -4,5 -6,9 -10,9 -16,7 -24,1 -33,2
9 -5,2 -3,9 -4,3 -6,4 -10,1 -15,6 -22,7 -31,5
10 -5,5 -4 -4,1 -5,9 -9,4 -14,6 -21,5 -30
11
-5,9 -4,1 -4 -5,6 -8,9 -13,8 -20,4 -28,8
12
-6,2 -4,3 -3,9 -5,3 -8,4 -13,1 -19,5 -27,6
-1
Fig. 44 Calculation example
Given data Fire damper FDMD 200
V = 600 m .h
= 1,2 kg.m
Octave range 1000 Hz
Tab. 4.3.1. S
ef
= 0,0213 m
Calculation: w [m.s ] = (V [m .h ] / 3600) / S
ef
[m ]
w = 7,83 m.s
Tab. 9.1.1. = 0,636
Calculation: p = . . (w /2) = 0,636 . 1,2 . (7,83 /2) = 23,4 Pa
Tab. 10.3.1., Tab. 10.3.2.
and Tab. 10.3.3. L
W1
= 56,5 dB
K
A
= -5,5 dB
L
rel
= -10,7 dB (for 1000 Hz)
Calculation: L
WA
= L
W1
+ 10 log(S
ef
) + K
A
= 56,5 + 10 log(0,0213) - 5,5 = 34,3 dB
L
Woct
= L
W1
+ 10 log(S
ef
) + L
rel
= 56,5 + 10 log(0,0213) - 10,7 = 29,1 dB
3 -1
-3
2
-1 3 -1 2
-1
2
2