18
Speaker and Wiring Impedance
Speaker and Wiring Impedance
Wire Gauge
Run Length
25’
50’
100’
250’
500’
24 GA
1.3Ω
2.6Ω
5.1Ω
12.8Ω
25.7Ω
22 GA
0.8Ω
1.6Ω
3.24Ω
8.1Ω
16.0Ω
20 GA
0.5Ω
1.0Ω
2.0Ω
5.0Ω
10.1Ω
18 GA
0.3Ω
0.6Ω
1.28Ω
3.2Ω
6.4Ω
16 GA
0.2Ω
0.4Ω
0.8Ω
2.0Ω
4.0Ω
14 GA
0.1Ω
0.25Ω
0.5Ω
1.26Ω
2.5Ω
12 GA
0.08Ω
0.16Ω
0.32Ω
0.8Ω
1.6Ω
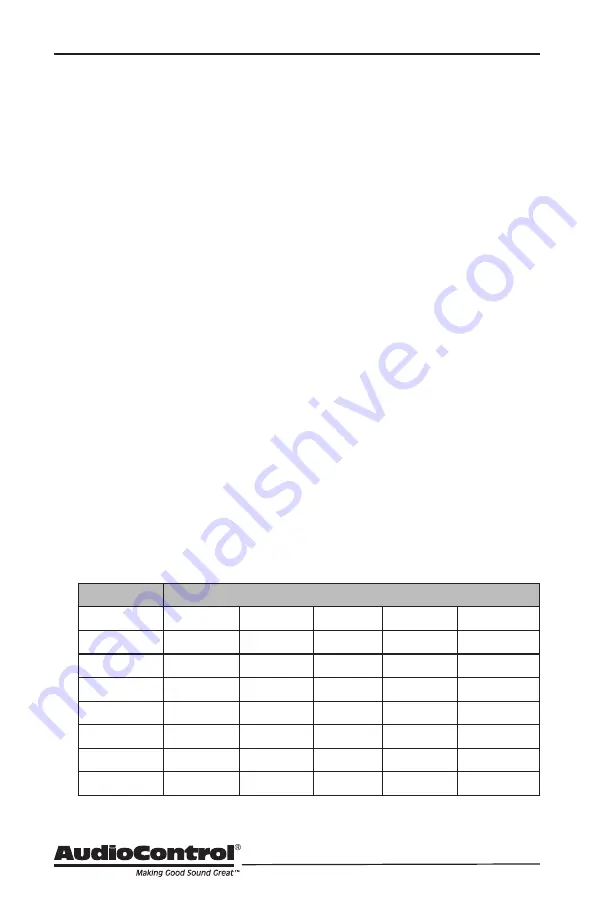
Speaker Wire Resistance:
Wire Gauge versus Run Length
ues in portions of their frequency range,
and speakers that are rated at unusual
impedances, for example 3.5 Ohms. The
Director M6400 and M6800 are tolerant of
lower impedance loads, however, all good
designs use some margin of error.
Your choice of speaker wire gauge and the
length of the runs, also affects the speaker
impedance load presented to the amplifi-
ers. As you can see in this table, even fairly
short speaker runs can have significant
resistance if you use a smaller wire gauge.
This can be a benefit if you are paralleling
lots of speakers. The wire itself acts as
an impedance limiter, since the amplifier
cannot see a speaker load lower than the
resistance of the wire. The downside of
this wire resistance is that you waste some
part of the total power available to the
speakers.
Speakers, like other resistors, when wired
in parallel “show” lower values than the
individual components. Here are two
examples for calculating speakers wired in
parallel:
Calculating Impedance
For three 8 Ohm speakers wired in
parallel (pluses connected to pluses)
the impedance is 1/8 + 1/8 + 1/8 = 3/8
Then take the inverse or 8/3 = 2.66 Ω
For two 8 Ohm speakers wired in
parallel (pluses connected to pluses)
the impedance is 1/8 + 1/8 = 2/8
Then take the inverse or 8/2 = 4 Ω
Often the real world is more complicated
than theory, and for speakers this is the
case. An eight Ohm speaker is not eight
Ohms at all frequencies. Plus passive
crossover networks add their own chang-
ing conditions. Be aware of speakers that
have significant dips from “nominal” val-
















































