12
TB MRI3-ITE(R) 12.00 E
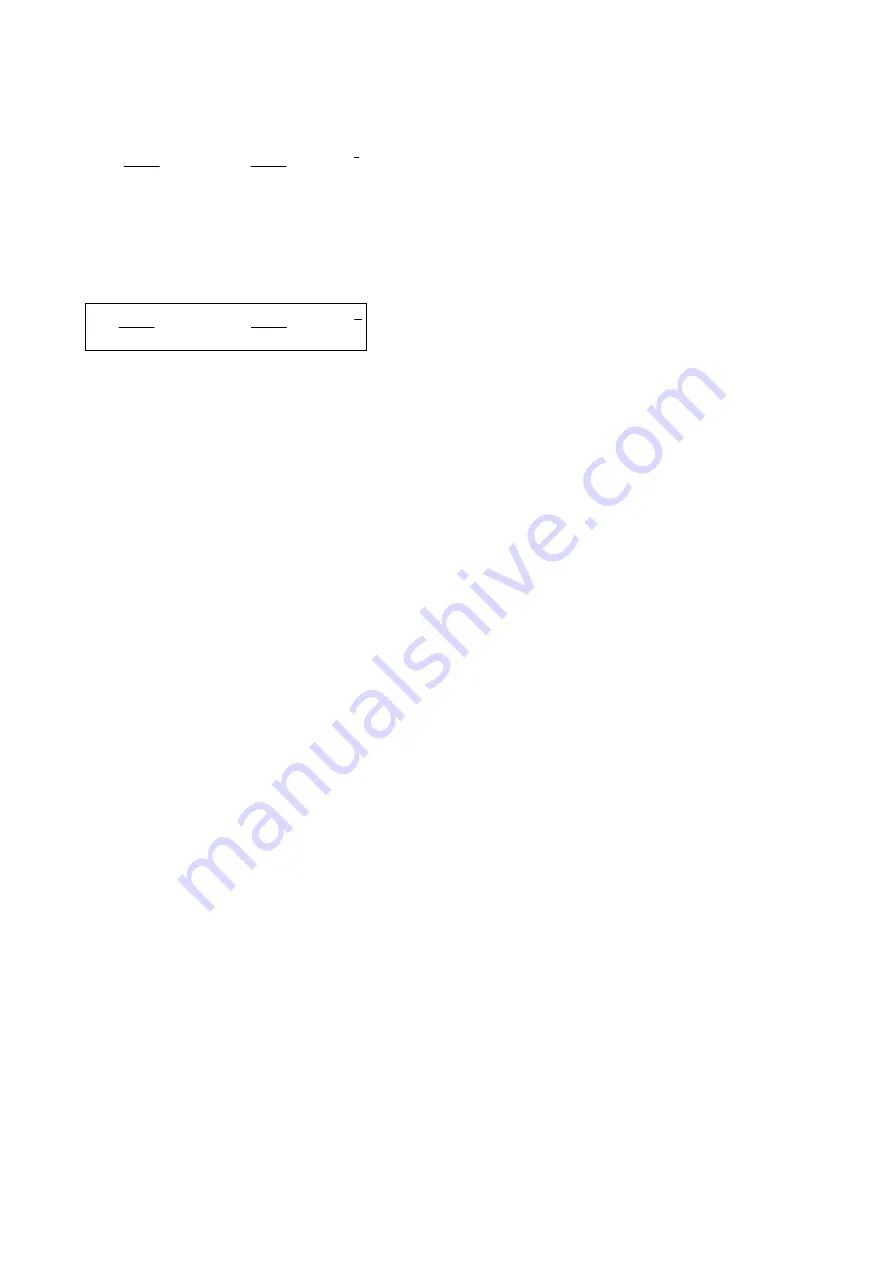
Analogous, for the temperature equivalent
ϑ
with
ϑ
max
the following formula is applied:
ϑ
ϑ
τ
( ')
(
)
(
)
'
t
I
k I
I
k I
e
B
B
t
=
⋅
⋅
+
−
⋅
⋅
⋅
−
2
2
0
2
2
100%
100%
with I = highest measured phase current.
In each new calculation step n, the momentary tem-
perature equivalent is determined as follows:
ϑ
ϑ
τ
n
n
B
n
n
B
dt
I
k I
I
k I
e
=
⋅
⋅
+
−
⋅
⋅
⋅
−
−
2
2
1
2
2
100%
100%
(
)
(
)
with
I
n
:
Highest measured phase current in
calculation step n
dt:
Time interval between the individual
calculation steps
ϑ
n-1
:
Temperature equivalent of the
preceding calculation step
The temperature equivalent
ϑ
n-1
has not been calculated
after start of the protection program (connection of aux-
iliary voltage),
Therefore, the temperature state of the electrical item to
be protected is expected to be cold.
If, however, the electrical equipment has been initially
loaded, it will take approximately 3 x
τ
warming up
, (at con-
stant load) until the thermal equivalent equals the real
state.
Different time constants:
After switching off the electrical equipment (I
n
= 0)
temperature will decrease and tend towards
ϑ
n
= 0
(environmental temperature).
e.g.:
τ
cooling down
= 2
⋅
τ
warming up
Depending on the comparison between the last meas-
ured current and the actual current the cooling down
or warming up constant will be applied for calcula-
tion.
I
n
≥
I
n-1
⇒
warming up
I
n
< I
n-1
⇒
cooling down
If the relay is supplied by the mains to be protected,
i.e. if the relay is not supplied to auxiliary voltage after
tripping of the mains c.b., the following has to be con-
sidered:
After return of the supply voltage, it is assumed that the
electric item is in cold condition. In this case this as-
sumption does not comply with the reality. Therefore
an external supply voltage should be applied.
The tripping criterion for the alarm or tripping element
of the thermal characteristic is:
ϑ
Trip
> k
2
⋅
100%
The effective values of the measured phase currents
are detected by calculating the root out of the integral
of a period’s momentary current squares. Calculation
of the thermal equivalent is always based on the high-
est of the three phase currents.













































