TB MRI3-ITE(R) 12.00 E
11
4.4
Algorithm
Based on the defined thermal model, one can deduce
that an energy Q is stored in the electrical equipment.
After expiry of a long time and with a constant current
load, a stationary condition will be achieved in which
the temperature of the electrical equipment does not
increase anymore. The heat supplied for a unit of time
is equal to that released by cooling down (steady en-
ergy balance).
Q
released
= Q
supplied
The supplied thermal energy as well as the tempera-
ture
ϑ
of the electrical equipment in stationary condi-
tion are in proportion to the square of the phase cur-
rent (e.g. ohmic losses and iron losses in the trans-
former).
Q
∼
I²
or
ϑ
∼
I²
Since the pickup value in the
MRI3-ITE
is determined
by I
B
, the following relation is effective:
ϑ
n
⋅
k²
∼
(I
B
⋅
k)²
For this purpose, the temperature T that really prevails
in the electrical equipment needs to be known. This
temperature
ϑ
(in%) is described in the thermal replica
through the temperature equivalent k
⋅
I
B
. When being
loaded with the maximum permissible operating cur-
rent
ϑ
B
in stationary condition, the electrical equipment
reaches the maximum allowed operating temperature
ϑ
B
. For this load, the temperature equivalent is defined
as k
2
⋅
100%.
ϑ
(%) =
(k I
I
B
2
2
100%
⋅
⋅
)
I.e.: At a load with I = 0.9 x (k
⋅
I
B
) and k
⋅
I
B
= 1.2,
and according to the before indicated definition, the
temperature reaches 81% of the maximum permissible
operating temperature. For an electrical equipment that
- after initial load - will be loaded beyond the admissi-
ble operating current (I > k·I
B
), the following tempera-
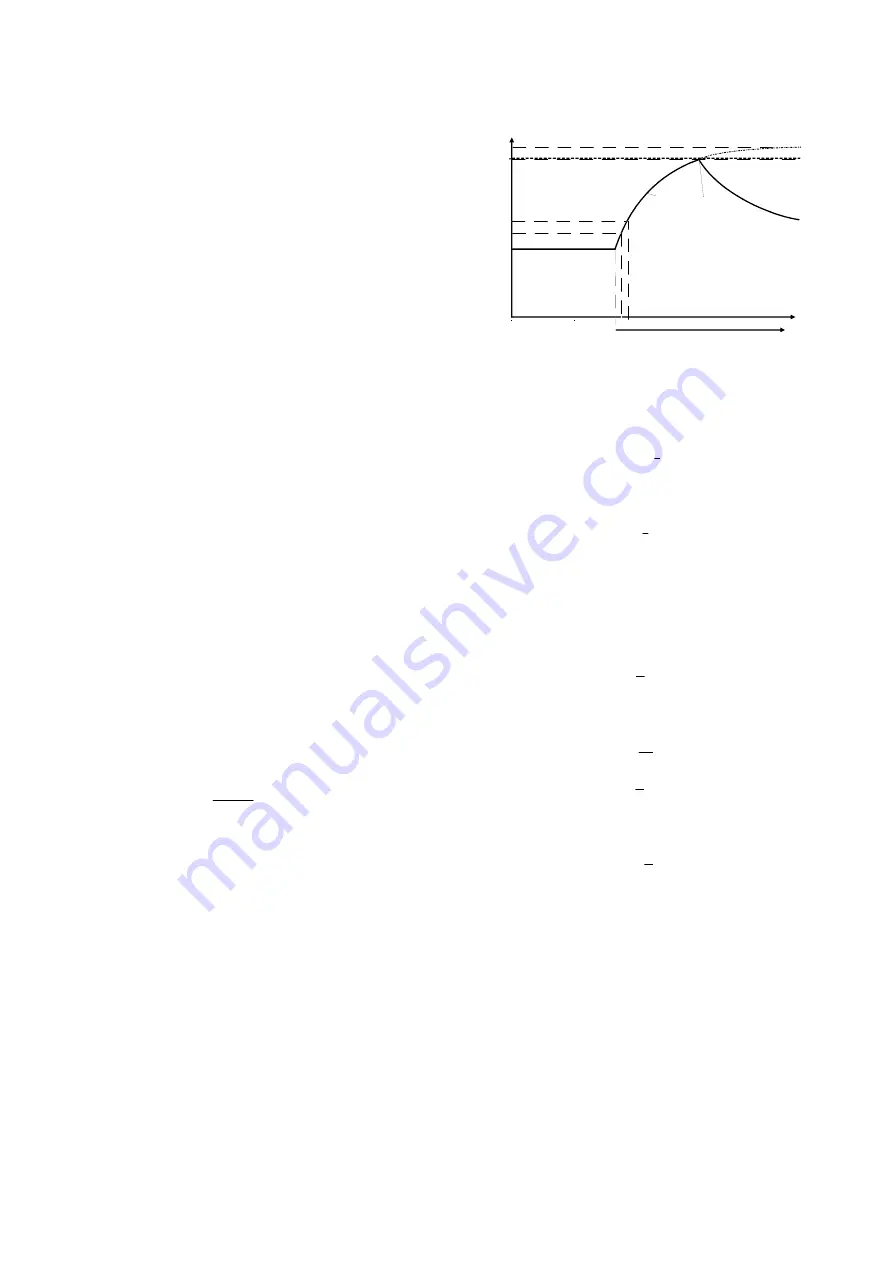
ture curve will result:
T
(%)
t
100%
T
0
T
1
T
2
dt dt
T
max
T(t)
'TRIP'
t0
t'
k ^2 *
Figure 4.3: Warming up of an electrical item
The thermal equivalent ( T =
ϑ
) is described by an ex-
ponential function.
for (
ϑ
>
ϑ
0
):
ϑ
ϑ
ϑ
ϑ
τ
( ')
(
) (
)
max
'
t
e
t
=
+
−
⋅ −
−
0
0
1
after transposition:
ϑ
ϑ
ϑ
ϑ
τ
( ')
(
)
max
max
'
t
e
t
=
+
−
⋅
−
0
If
%
100
)'
t(
k
2
⋅
≥
the alarm or trip element is acti-
vated.
The temperature after dt can be calculated as follows:
ϑ
ϑ
ϑ
ϑ
τ
1
0
=
+
−
⋅
−
max
max
(
) e
dt
After 2 times dt:
ϑ
ϑ
ϑ
ϑ
τ
2
0
2
=
+
−
⋅
−
max
max
(
) e
dt
or
ϑ
ϑ
ϑ
ϑ
τ
2
1
=
+
−
⋅
−
max
max
(
) e
dt
general:
ϑ
ϑ
ϑ
ϑ
τ
n
n
dt
e
=
+
−
⋅
−
−
max
max
(
)
1
Thus, a recursion formula was established from which
in order to calculate the thermal equivalent
ϑ
n
:
•
The last value
ϑ
n-1
,
•
the stationary final value
ϑ
max
for the actual current,
•
the adjusted time constant
τ
,
•
and the time since the last calculation dt must be
known.









































