M-Drive DSP initialization | 25
16 : 5.6. Internal parameters
Click on the cogwheels button to access the Internal parameters window.
16 : 5.5. Pressure Model Operating Mode
The amplifier’s output signal is modified in real time as a result of the combination of the input audio signal and the feedback
loop. The alteration is applied to the input stage so to minimize the difference between the desired output and that measured
and reported by interpolating two the feedback loops.
16 : 5.5.1. Pressure Control (pressure feedback loop)
This loop brings the pressure level measured at the radiating sound field, back to the input stage. This is achieved by
feeding the pressure sensor measurement through a first order low pass filter (LPF) and a linear amplifier. The parameters that
can be set by the user for this feedback-loop therefore are:
f
f
The loop gain, expressed in dB and limited by the system’s stability, defining the
strength of the feedback effect;
f
f
The LPF bandwidth, defining the top frequency of the band at which this feedback loop
is active;
f
f
The HPF bandwidth, defining the bottom frequency of the band at which this feedback
loop is active.
It is important to set the loop gain carefully, as too high a value will bring the system to instability. This results in a loud
“ship’s horn” sound which can potentially damage the system if not silenced after a short time. This can be done by lowering
the feedback loop gain until the sound stops. The entire pressure feedback loop can be bypassed by entering
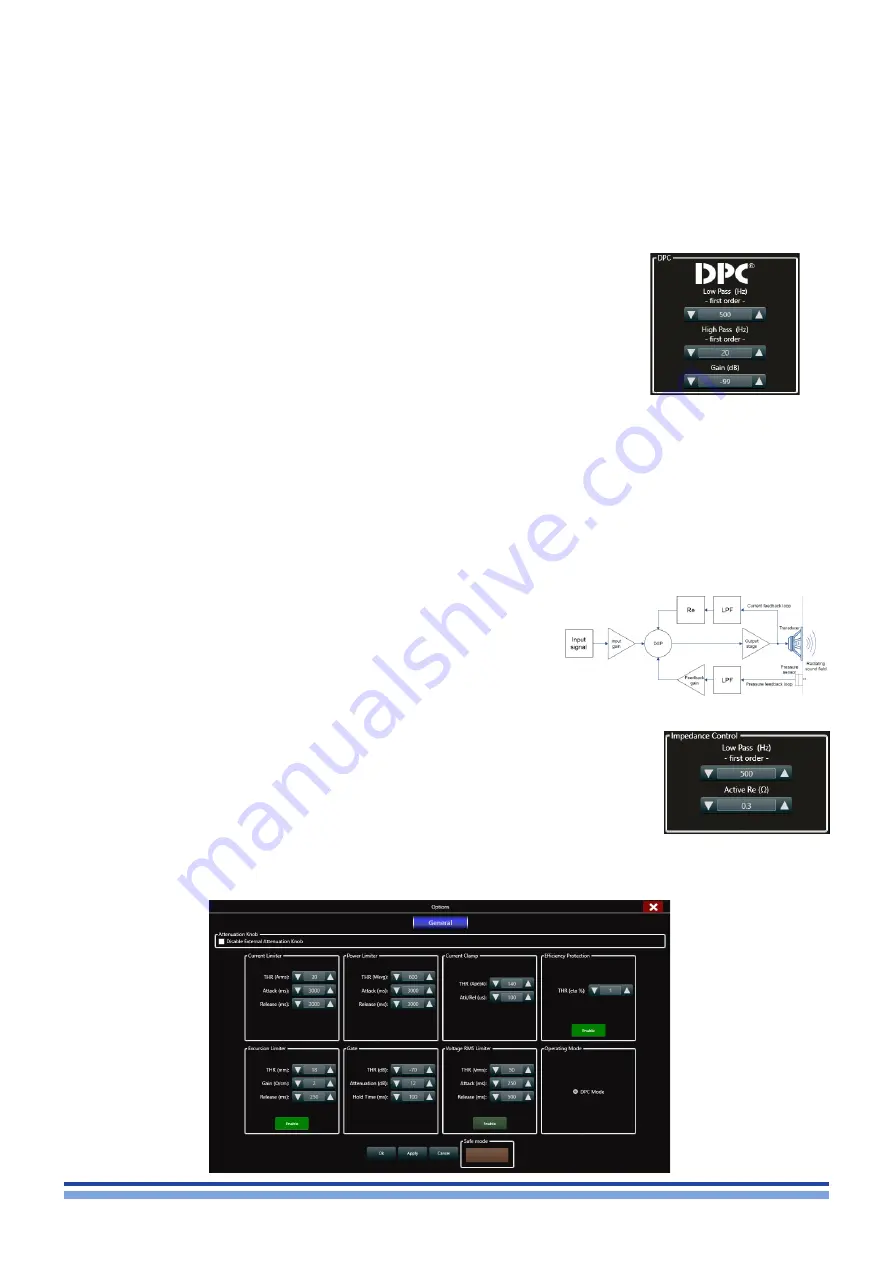
16 : 5.5.2. Impedance Control (current feedback loop)
This loop brings back a voltage signal proportional to the current present at the output stage to the system’s input. The
signal taken from the output is first filtered by a first order low pass filter. Following this step, the current/voltage translation is
carried out by a virtual resistor named “Re”. The importance of this series resistor relies in the difference between traditional
transducers and M-Force.
M-Force has an extremely low impedance. This means that they manifest a
violent, extremely high Q resonant peak which can result in an unnatural sound
reproduction. By adding a virtual series resistor Re, the entire system’s resonant
peak will be slightly flattened and widened in a way that mimic’s a traditional
speaker’s lower Q resonance. This resistor will however not affect the power
transfer from input to output as this is not a “real” physical resistor subject to
ohmic heating. The parameters the user can set for this feedback loop therefore
are:
f
f
The “added Re” value, defining the additional series resistor inserted to simulate a traditional
speaker behavior. This is usually a small number, which aims to return the apparent
loudspeaker resistance to a traditional 2-4 Ohm value;
f
f
The LPF bandwidth, defining the range of frequency at which this feedback loop is active.






























