66
For example: If the power factor of each of the two legs were Cos 30
˚
,
then:
PF
AC
=
√
3/2 = 0.866
PF
BC
=
√
3/2 = 0.866
Load Power Factor can now be represented in terms of these two components
Load PF =
PF
AC
+ PF
BC
= 0.866 + 0.866 = 1 = Cos
θ
(30
˚
)
√
3
1.732
%PF = PF(100) = 100%
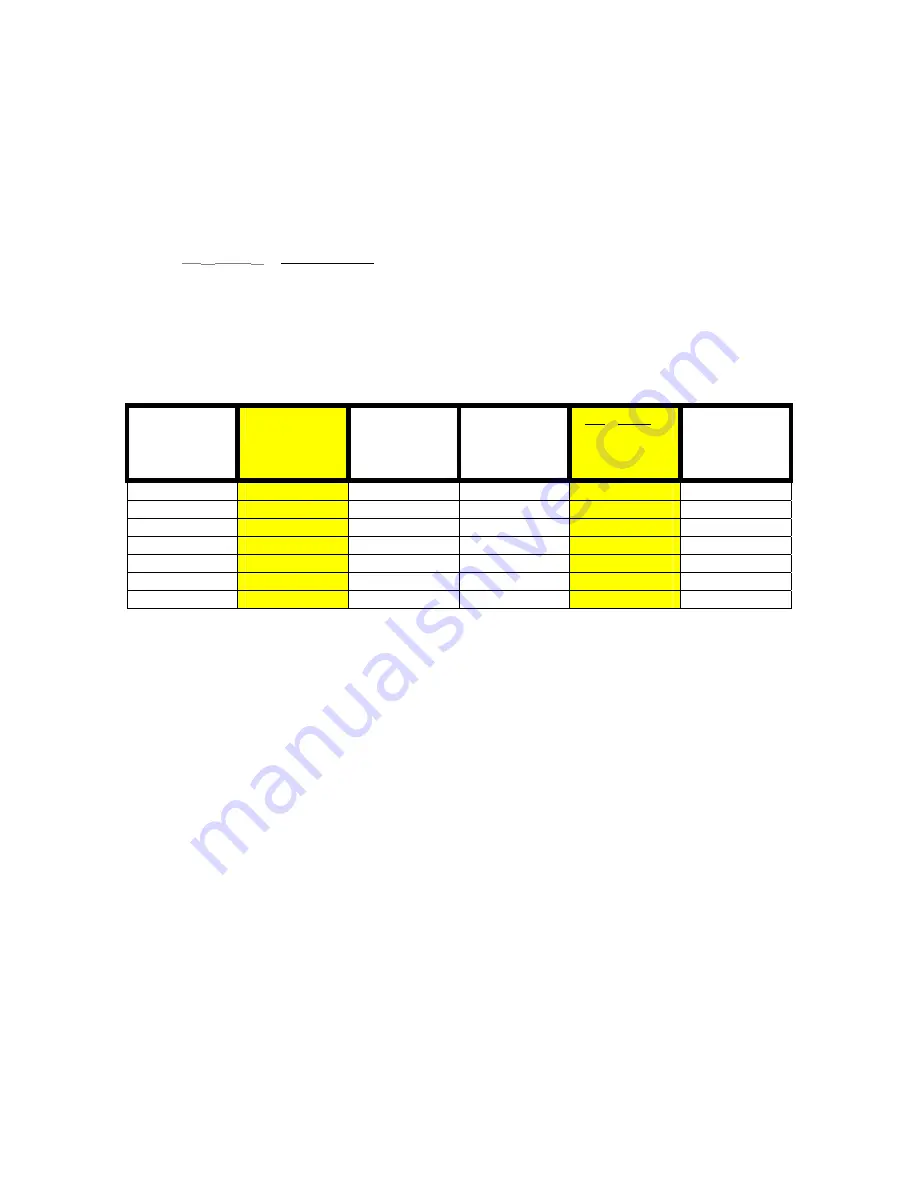
The following chart is provided as an example of the equality shown in the above equation. Power Factor
may be represented in terms of Cos
θ
or in terms of the summed power factors from each leg of the
circuit divided by the
√
3.
Ø
Load
Cos Ø
PF
AC
Cos (30
˚
+Ø)
PF
BC
Cos (30
˚
-Ø)
PF
AC
+ PF
BC
√
3
Total
Power
-90
˚
0 1/2
-1/2 0 0P
-60
˚
½
√
3/2
0
1/2
1/2P
-30
˚
√
3/2 1 1/2
√
3/2
√
3/2P
0
˚
1
√
3/2
√
3/2
1 P
30
˚
√
3/2 1/2 1
√
3/2
√
3/2P
60
˚
½
0
√
3/2
1/2
1/2P
90
˚
0 -1/2 1/2 0 0P
.
address and phone number is shown below:
4271 Bronze Way
Dallas, TX 75237-1088
U.S.A.
Phone: (800)
641-2349
Fax:
(214)331-7334
Summary of Contents for PMM-1
Page 58: ...54 ...

































