Humboldt HS-5001EZ Product Manual Download Page 65

Page: 65 / 72

which is the slope in terms of counts per minute per unit density. Combining

this equation and the equation for precision and accounting for the pre scale

value of 16 yields:

Where:

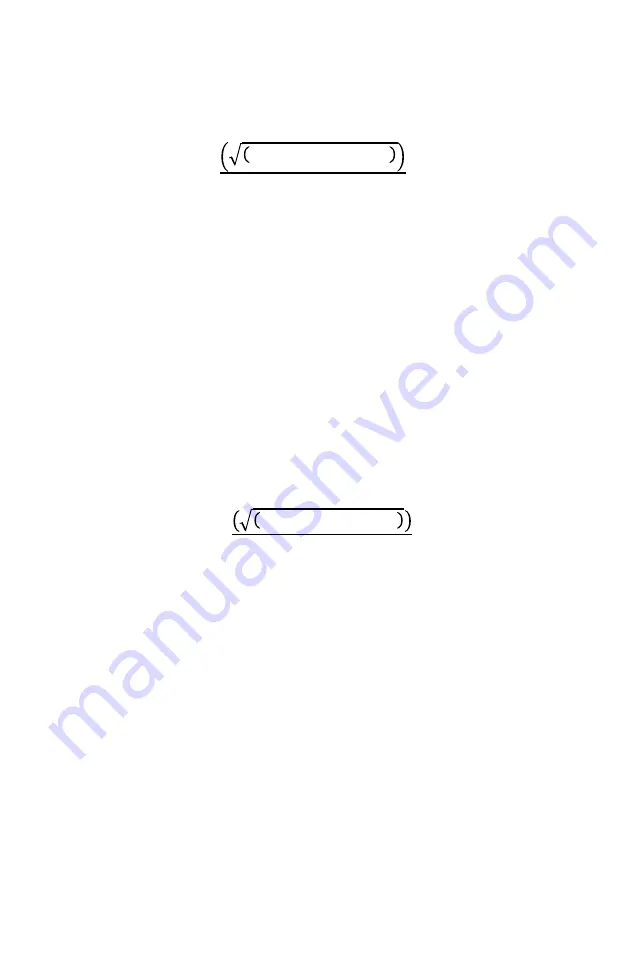
DP=

Density precision at density D

D= Density

DS =

Density standard count

A, B, C =

Calibration constants

S =

Slope

This is for one standard deviation, a 68% confidence factor.

Applying the same procedure to the moisture equation results in an

equation for the moisture precision:

Where:

MP = Moisture precision
M = Moisture
MS = Moisture standard count
E, F = Calibration constants

Both of the above previsions are stated for the one minute (NORM)

measurement period. The values would increase by a factor of two for 0.25

minutes (FAST) and decrease by a factor of two for the four minute (SLOW)

measurement period.
These precisions are the theoretical values and the Gauge should yield these

values if there are no instability problems. Measurement data can be used to

test the Gauge. If a series of measurements are made on the same location,

the values of precision can be calculated by using:

𝑫𝑫𝑫𝑫 =

𝑫𝑫𝑫𝑫 ∗ 𝑨𝑨 ∗ 𝒆𝒆

!𝑩𝑩𝑩𝑩

− 𝑪𝑪

𝟒𝟒𝟒𝟒𝟒𝟒 ∗ 𝑨𝑨 ∗ 𝑩𝑩 ∗ 𝒆𝒆

!𝑩𝑩𝑩𝑩

𝑴𝑴𝑴𝑴 =

𝑴𝑴𝑴𝑴 ∗ (𝑬𝑬 + 𝑭𝑭 ∗ 𝑴𝑴)

𝟒𝟒𝟒𝟒𝟒𝟒 ∗ 𝑭𝑭

Summary of Contents for HS-5001EZ

Page 1: ...product manual HS 5001EZ_MAN_08 12 HS 5001EZ Moisture Density Gauge ...

Page 2: ......

Page 3: ...ble the software in any fashion except as instructed in this manual US copyright laws trademark laws and trade secrets protect the materials Any person s and or organizations that attempt or accomplish the above violation or knowingly aid or abet the violation by supplying equipment or technology will be subject to civil damages and criminal prosecution IMPORTANT NOTICE The information contained h...

Page 4: ... 1 3 7 Mechanical Specifications 5 1 3 7 1 Materials 5 1 3 7 2 Gauge 6 1 3 7 3 Gauge 6 1 3 7 4 Gauge 6 1 3 7 5 Gauge 6 1 3 7 6 Gauge 6 1 3 8 Accessories 6 2 Equipment Description 7 7 2 1 Zippered Accessory Case 7 2 1 1 Scraper Plate Rod 7 2 1 2 Drill Rod 8 2 1 3 Four Pound Hammer 8 2 1 4 Extraction Tool 8 2 2 Transit Case 8 2 2 1 Transit Case 9 2 2 2 Reference Standard 9 2 2 3 Gauge 5001SD 9 2 2 3...

Page 5: ... Menus 29 4 1 1 View Current Measurement 29 4 1 2 Current Standard Statistical Counts 29 4 1 3 Projects Setup 30 4 1 3 1 Edit Store Data 31 4 1 3 2 View Stored Data 31 4 1 3 3 Print Data Report 32 4 2 Setup Menus 32 4 2 1 Date Setup 32 4 2 2 Time Setup 33 4 2 3 Units Setup 34 4 2 4 Measurement Modes Setup 34 4 2 5 Trench Correction Setup 35 4 2 6 Targets Setup 35 4 3 Engineering Menus 35 4 3 1 Cal...

Page 6: ...tery Replacement 43 6 3 Electronic Modules Adjustment Replacement 43 6 3 1 Processor Module 200682 43 6 3 2 Base Plane Board 200112 44 6 3 3 High Voltage Power Supply Module 200088 44 6 3 4 Density Amplifier Module 200087 44 6 3 5 Moisture Amplifier Module 200086 45 6 4 Detector Replacement 45 6 5 Parts List 46 6 6 Service Hints 48 6 7 Calibration 49 7 Theory of Operation 49 7 1 Density Measuremen...

Page 7: ...8 6 Reporting of Loss or Incident 61 8 7 Radiation Profile 61 9 Warranty 63 9 Warranty 93 ...

Page 8: ... degree by other low atomic number elements such as carbon and oxygen The presence of chemical elements such as boron which may absorb or capture thermal neutrons will also have some effect on the accuracy Hydrated minerals such as gypsum or crystals such as mica may cause the largest single error In general a material containing hydrogen which is not removed during an oven dry procedure as outlin...

Page 9: ...pe of the count rate at other densities see 7 3 The precision is a function of time and varies as the square root Increasing the count time of the measurement by a factor of four will improve the precision by a factor of two 1 2 2 Chemical Error An error that is caused by the variations in the chemical composition of the material being tested Gamma attenuation is a function of the electron density...

Page 10: ...06243 Conversion to the SI gravitational system may be made by multiplying by 9 807 to obtain kilonewtons per cubic meter It is common practice to refer to these units in the gravitational system as unit weights and to those units in the absolute system as densities 1 3 Specifications 1 3 1 Density Measurement Backscatter Density at 2000 kg m 125 pcf Direct Transmission Density at 150 mm 6 inch SL...

Page 11: ...he operator without use of a password Two of these are adjustments to the main calibrations to compensate for materials widely different from normal soils No additional equipment is required for the adjustment other than a sample of the material at a known density No additional equipment is re quired for an entirely new calibration other than a suitable set of standards Count rate data is converte...

Page 12: ...ar license 1 3 6 Electrical Specifications Displays 4 lines x 20 characters alphanumeric LCD with backlite Timer Stability 0 01 Power Supply Stability 0 10 Power Source Six alkaline AA size batteries Power Consumption Processor Idle 3 5 mA Active 6 5 mA 8 volts 53 milliwatt 1400 Operating Hours Power Protection Circuit Breaker Short Circuit Proof Auto Alarm for low battery condition Auto shutoff f...

Page 13: ...npadded Gauge meets USDOT 7A without transit case 1 3 7 2 Gauge Size excluding handle 400 x 220 x 140 mm 15 75 x 8 66 x 5 5 in Height with handle 450 or 550 mm 18 or 21 6 in Weight 13 6 kg 30 lbs 1 3 7 3 Reference Standard Size 350 x 200 x 75 mm 25 x 7 8 x 3 in Weight 4 5 kg 10 lbs 1 3 7 4 Transit Case Size 600 x 495 x 356 mm 26 x 14 x 19 5 in Weight 11 8 kg 26 lbs 1 3 7 5 Zippered Accessory Case ...

Page 14: ...d canvas bag It is convenient to carry and decreases the bulk and weight of the transit case which contains the Gauge Reference Standard and Manuals 2 1 1 Scraper Plate Rod Guide When the gauge is to be used on soil the Scraper Plate is used to smooth the site to eliminate as many surface voids as possible Two convenient handles are located so that it may be used to scrape away loose material The ...

Page 15: ...a larger hammer is not needed since it could rapidly damage the drill rod 2 1 4 Extraction Tool This tool is used to assist the removal of the drill rod if it becomes stuck in clay or granular material The usual problem is a vacuum which can exist in the hole when attempting to pull the rod out It does not have to be put into place before driving the rod A slot in the middle is placed on a square ...

Page 16: ...gen in the reference standard determines the moisture standard count The density standard count is determined primarily by the shielding material in the base of the gauge and only slightly by the reference standard The Reference Standard is serialized to match the gauge and they must not be interchanged between gauges or moisture measurement errors may exist 2 2 3 Gauge HS 5001EZ The HS 5001EZ typ...

Page 17: ...6 10 ...

Page 18: ...is feature eliminates the potential damage and soiling of standard connectors in the field 2 2 3 3 Front Panel Touch Screen and Keypad All data entry editing and function selections and other options are available via a 10 key membrane keypad at the front panel Each time a key is pressed a short bleep indicates that the key press has been recognized The key must be pressed and released for the act...

Page 19: ...atory Marshall density or maximum theoretical density The value set into this register must never be set to a value outside the range of normal soil or asphalt densities Anything between 900 kg m 56 PCF and 3000 kg m 200 PCF will not cause processor errors It must never be set to 0 0 Standard Statistical The STD STAT key initiates a 4 or 16 minutes count of both the moisture and density channels w...

Page 20: ...damage The Zippered Accessory Case will prevent loss of its items and if an automobile is used it will protect the trunk space If transport is made by automobile the Transit Case and Gauge should be placed in the trunk to keep it as far away from passengers as possible Van location should be toward the rear and the case secured to prevent shifting In open trucks means must be taken to prevent shif...

Page 21: ...s in the standard counts the display will show Otherwise the display will show the density and the moisture counts with percent errors Errors within 1 for density and 2 for moisture counts are expectable If the errors are outside these limits refer back to important notice above If the above conditions are normal then press F4 ACCEPT AND TAKE NEW STANDARD and take a new standard as above in 3 2 Re...

Page 22: ... 16 minutes the averages of the 16 counts are stored in the DS and MS registers A statistical test then would have been run on the 16 individual counts and an R value displayed for both DS and MS These values should fall between 0 5 and 1 5 If they are only slightly out another test may be made but if the value is greatly outside the 0 5 to 1 5 limits service is needed To run the statistical stand...

Page 23: ...f you wish to take another standard please press F3 otherwise press F4 to use the current standard and go back to main menu If taking new standard the display will show MEAS FAST NORM SLOW STD 4MIN 16MIN TYPE ASPH SOIL THIN DEPTH AUTO MANUAL MEAS FAST NORM SLOW STD 4MIN 16MIN TYPE ASPH SOIL THIN DEPTH AUTO MANUAL DS XXXX MM DD YY MS XXX MM HH TAKE NEW STD USE CURRENT STD TAKING STATISTICS TIME REM...

Page 24: ...mally a laboratory Proctor density For asphaltic materials the Marshall or a maximum density is used The degree of compaction based on a percentage of a Proctors is a function of the measured dry density and is obtained from the result screen after a successful measurement has been obtained PR For asphaltic materials it is a function of the wet density or total density MA Press MAX D to display th...

Page 25: ...value of KVAL until the correct PERCENT MOISTURE is computed by the gauge while retaining the same measurement data in memory C If no other method is available to verity the gauge moisture calibration the PERCENT AIR VOIDS may be utilized to determine if major errors exist Well compacted soils should have PERCENT AIR VOIDS between 2 0 and 5 0 depending on gradation If a result of the void content ...

Page 26: ...3 5 Site Preparation Any site to be measured should be clear of all loose debris before attempting to seat the gauge After removing the loose material from soils the area should be leveled using Scraper Plate to provide a flat surface Any large surface void areas should be filled with native fines even though a direct transmission measurement will be made If hard surfaced areas are involved which ...

Page 27: ...uge toward the control panel end to force the source rod against the side of the prepared hole This is important since a void could exist between the rod tip and the side of the hole 3 7 Taking the Measurement Count The measurement can be taken by simply pressing the MEAS key Most measurements will be made by using the NORM in measure mode which takes an exact one minute count It may be desirable ...

Page 28: ...d due to the destructive nature of making the direct transmission hole Press the MEAS key After counting down from 1 00 the wet density and Marshall will appear in the display Since the moisture channel is actually measuring hydrogen the AC displayed is an approximation of the asphalt content of the mix The depth of measurement will be 100mm 4 inches or even more depending on the actual asphalt co...

Page 29: ... the validity of the results is questionable This gauge uses the nomograph principal where the density of the underlying material is known from prior measurement The top layer density is then calculated The density of the underlying material must be entered in the LWD register To enter LWD press MAIN MENU F2 SETUP F4 SET TARGETS F1 The LWD value is flashing Press F3 to INCREASE or F4 to DECREASE t...

Page 30: ...the SAFE position and note that the display did not change It is not necessary to leave the source in the measurement position exposed while calculations are made So long as measurement data is present in the active registers the handle position in which the data was taken remains in the display Clear the data by MAIN MENU and the display will again correctly indicate SAFE To view the results of t...

Page 31: ... are ones where the nomograph correction is recommended Top Layer Error with no correction for difference in density mm inch 2 4 6 8 10 15 20 25 0 1 0 1 0 2 1 3 1 4 1 5 2 7 8 10 4 37 5 1 5 0 7 1 4 2 1 2 8 3 5 5 3 7 0 50 0 2 0 0 5 0 9 1 4 1 8 2 3 3 4 4 6 62 5 2 5 0 3 0 6 0 8 1 1 1 4 2 1 2 8 75 0 3 0 0 2 0 3 0 5 0 7 0 8 1 2 1 6 87 5 3 5 0 1 0 2 0 3 0 4 0 5 0 7 0 9 100 0 4 0 0 1 0 1 0 2 0 2 0 3 0 4 0...

Page 32: ...om 1 00 the dry density DD percent moisture M wet density WD moisture M and percent proctor PR will appear in the display If you need the actual counts pressing F4 NEXT the DC density counts DS density standard MS moisture standard and MC moisture counts will indicate the counts used to determine the computed data The VR and AV will indicate void ratio and percent air voids The latter is quite use...

Page 33: ...he mode to Soil as described above in 3 7 2 Press MAIN MENU F2 SETUP F3 SET TRENCH COR First place the gauge on its Reference Standard in the same location in the trench where a measurement is to be made With the gauge handle in SAFE press F4 A four minute count is started indicating that a correction is being determined to account for the water in the walls of the trench Four minutes are used to ...

Page 34: ...DENSITY and the PERCENT MOISTURE is obtained by dividing the MOISTURE CONTENT by the DRY DENSITY The processor performs the functions which produce the results without consulting tables or transferring data to a hand held calculator This decreases the potential for operator error The processor also compensates for the attenuation coefficient of hydrogen which is vastly different from soils If the ...

Page 35: ...tion if the maximum density is not known 3 8 3 Percent Air Voids This term is defined as volume of air as a percentage of the total volume The specific gravity of the solids must be known and have been entered as described in 3 3 3 If the calculation is made for asphaltic concrete the normal PERCENT MOISTURE calculation for soils will have to be adjusted by using KVAL to agree with the asphalt con...

Page 36: ...n Subsequently the dry density in the soil mode measurement wet density moisture in the soil mode measurement moisture percent percent Proctor in the soil mode measurement void ratio percent air void percent Marshall in the asphalt mode measurement were calculate at that depth 4 1 2 Current Standard Statistical Counts DATA 06 06 98 SETUP 4 00 00 ENGINEERING DEPTH SAF DATA 06 06 98 SETUP 4 00 00 EN...

Page 37: ...ojects Setup This setup allows the entry of data pertaining to the project s on which the gauge is being used Their entry is made by first selecting the desired function which displays the current value keying in a new value using the function keys DATA 06 06 98 SETUP 4 00 00 ENGINEERING DEPTH SAF CURRENT MEASUREMENT STD STAT PROJECTS SAVE DATA EDIT STORE CURRENT EXAMINE PREVIOUS PRINT REPORT ERAS...

Page 38: ...n 4 1 3 then F2 PROJECT 0 0000001 STA 000 00 TO 000 00 INCREASE NEXT SCREEN OFFSET R 0 0 STA 000 00 LANE E B INCREASE STORE PROJECT 0 0000001 STA 000 00 TO 000 00 INCREASE NEXT SCREEN OFFSET R 0 0 STA 000 00 LANE E B INCREASE STORE DATA COLLECTED DATE mm dd yy TIME hh mm PM NEXT DD XXXX X M XX X WD XXXX X M XX X PR XXX X NEXT MDEPTH BAC DC XXXX X DS XXXX X MC XXX X MS XXX X VR XXX X AV XXX X NEXT ...

Page 39: ... from the main menu This key will allow the users to setup the date time units measurement modes gauge standardization time type of measurement and depth detection 4 2 1 Date Setup Connect IR adapter to Gauge and PC Start PC program START IR DOWNLOAD IR DOWNLINK PROGRESS PLEASE WAIT FOR IR DOWNLOAD TO COMPLETE WARNING ERASING ALL PROJECT DATA CONTINUE ERASE F3 ABORT ERASE F4 EDIT STORE CURRENT EXA...

Page 40: ...3 to DECREASE the value then presses F1 to advance for DD value setup and so on for YY value After the date has been set press F4 to save the new date the display will return to main menu display 4 2 2 Time Setup DATA 06 06 98 SETUP 4 00 00 ENGINEERING DEPTH SAF SETUP 2 SET MEASURE MODES SET TRNCH COR SET TARGETS SET DATE SET TIME UNITS PCF SI TIME HH MM SS AM INCREASE DECREASE SAVE ...

Page 41: ...e will be flashing by pressing F3 the unit will change from PCF English measurement unit system to SI Metric measurement unit system and so on To exit this menu press MAIN MENU key 4 2 4 Measurement Modes Setup DATA 06 06 98 SETUP 4 00 00 ENGINEERING DEPTH SAF SETUP 2 SET MEASURE MODES SET TRNCH COR SET TARGETS SET DATE SET TIME UNITS PCF SI DATA 06 06 98 SETUP 4 00 00 ENGINEERING DEPTH SAF SETUP ...

Page 42: ...section 3 7 2 4 page 26 4 2 6 Targets Setup The value of MAX D will be flashing press F3 to INCREASE or press F4 to DECREASE the value then presses F1 to advance for the LWD value setup Pressing F2 will advance to the next line and KVAL value will be flashing Press F2 again to advance to SPG To exit this menu press MAIN MENU key Refer to section 3 3 for more details The MAXD may also be viewed and...

Page 43: ...irect transmission mode unless there are original calibration errors In the backscatter mode the surface roughness or surface air voids may require some small correction Again original calibration errors account for a large percentage of this error The gauge has a means of altering the factory calibration by a percent value There are nine sets CAL1 through CAL9 available and each set contains a se...

Page 44: ...vice life The recommended range is 10 to 35 C 50 to 95 F The most damaging environment to electronic instruments is humidity While it is possible to hermetically seal the instrument case the cost would be prohibitive It does have gaskets to seal out water from rain but the case must breathe and consequently water vapor moves in and out If the combination of humidity and temperature causes condensa...

Page 45: ...ed to push the rod out and could cause jamming of the shield which will result in faulty STANDARD COUNT repeatability Ultimately the soil will damage the seals between the cavity and the bearing The bottom plate which contains a scraper ring to remove soil from the rod when it is retracted can be removed by using a hex key to take out the two screws Lay the Gauge on its side or end with the bottom...

Page 46: ...ined of the main circuit board Most processors of these wipes allow both of these sources to be wiped with the same filter paper since they are able to determine from which source any contamination came First fill out the form including the Gauge model and serial number the type of radioactive material Cs 137 and Am 241 Be and the gauge serial number some kits also list the sources serial numbers ...

Page 47: ...the measurement The short term fluctuation of binary decay is predictable The predicted standard deviation is the square root of the average count rate m s m The Gauge electronics divide the actual events counted in a one minute period by a factor of 16 before using the number so the above expression is actually m s 4 This equation can be used to predict the standard deviation of the count rate fo...

Page 48: ...ngs it is not necessary to remove the source rod A suitable shield must be available REQUIRES AUTHORIZATION FROM REGULATORY AGENCY ON USERS LICENSE Drop the source rod to the backscatter position Loosen the two hex setscrew 001007 at the top and unscrew the Lift Cap 200667 and the Auto Lift Bumper 200278 to allow complete removal of the source rod and handle Hold the rod by the handle with the tip...

Page 49: ... it It will be easier it the Post Grommet 200109 is removed from the hole If worn or damaged the Bottom Gasket 200149 or Panel Gasket 200351 should be replaced 6 1 6 Top Post and Seals The seals and wipers will wear due to soil abrasion and soil working into them as the source rod is moved up and down Keeping the bottom cavity clean and lightly lubricating the source rod with silicon grease will h...

Page 50: ...lacement The batteries in this Gauge will last a very long time if the Gauge is not stored at a high temperature The expected service life is 1400 hours Replacement will be required within one to two weeks after the LO BAT symbol appears in the Display while in use Replacement batteries should be high quality alkaline AA size cells such as those made by Mallory DURACELL Do not replace with mangane...

Page 51: ... Density or Moisture Modules The entire frame including the detectors is removed by means of the seven screws around the edge of the frame Do not remove the screws which attach the Board to the frame 6 3 3 High Voltage Power Supply Module 200088 This module supplies a highly regulated 900 vdc to the Density and Moisture Amplifier modules and in turn to the detectors From unit to unit the voltage m...

Page 52: ...om the detector It should be set using an oscilloscope to produce average 500 millivolt negative pulses at test point MTP on the base circuit board This pulse height is not very critical and if the adjustment is set at mid range and the STAT test indicates stability the setting is acceptable without the availability of the oscilloscope The high voltage can cause a severe shock Before any replaceme...

Page 53: ...ammer 000176 Transit Case Assembly 200681 Reference Standard 200122 Gauge Padlock 000177 Instruction Manual HS 5001EZ 200688 Radiation Safety Manual 200121 Radioactive Source Certificate 200173 Wipe Test Materials Kit 200177 Leak Test Certificates 200174 Filter Paper 000175 Plastic Bags 000178 Forceps 000181 Lift Cap 200667 Hex Socket SS Sot Screw 6 32 x 3 16 2 001061 Lift Bumper 200278 Index Rods...

Page 54: ...t 200156 Hex Socket Head SS Cap Screw 1 4 20 x 1 4 001009 Lock Washer SS Split Spring 1 4 4 001031 Gauge Base No internal Parts 200027 Bio Shield 200029 Bottom Plate Assembly 200666 Bottom Plate 200665 Scraper Ring 000806 Retainer Ring 000811 Flat Head Hex Socket SS Screw 8 32 x 1 2 2 001010 Sliding Shield 200030 Shield spring SS 000817 Am Be Source Label 200092 Hex Socket SS Set Screw 5 8 18 x 00...

Page 55: ... Replace Processor Module Not Counting Anything Replace High Voltage Power Supply Replace Processor Module Not Counting Moisture Check Pulse Voltage at MTP Replace Moisture Amplifier Module Replace Neutron Detector Not Counting Density Check Pulse Voltage at DTP Replace Density Amplifier Module Replace Gamma Detectors Unstable Moisture Check Pulse Voltage at MTP Replace Moisture Amplifier Module R...

Page 56: ...ince it does involve the chemical elements or molecules which form the materials 7 1 Density Measurement by Gamma Radiation Gamma radiation is a form of electromagnetic radiation similar to the radio frequencies that carry television signals and rays of visible light The only difference is one of frequency At the frequency of gamma radiation materials exposed to it are ionized and this creates a h...

Page 57: ...electron density of the material which is only approximately related to the mass density The relationship is the ratio of the Z atomic number or number of electrons per atom and A atomic mass of the atom The term Z A is used frequently The process is further complicated by the probability that the interaction will or will not occur Atoms are mostly voids so many gammas will simply pass through wit...

Page 58: ... 2 8 0 478 0 0772 Potassium 2 6 0 486 0 0787 Magnesium 2 1 0 498 0 0796 Hydrogen 0 992 0 1600 51 The table below indicates the relative percentage for the most predominant elements in the crust of the earth along with their values of Z A and u p DENSITY Direct Transmission Gauge Calibration L O G O F C O U N T R A T I O B Average B Limestone B Granite B Metal A C ...

Page 59: ...n adjustment in the measured density The equation indicated is not practical for use in a gauge since the mass attenuation coefficient varies with energy which is changing as the gammas pass through materials and the detectors used are not linear with energy While many equations may be used to fit the data the most common is Where CR Count rate or Ratio at the detectors D Density of the material A...

Page 60: ...l have a composition between limestone and granite the metallic values of A and C can be used to calculate a value of B which applies to these materials or other values of B can be determined for any material Experimental data must be used and not the values from the above table The initial gamma energy is known to be 0 662 MeV but the average energy for the interactions would be impossible to det...

Page 61: ...n is Be9 α n C12 When the beryllium nucleus reacts with the alpha particle it becomes an isotope of carbon The C12 is left at an excess energy state and yields a 1 to 10 MeV neutron when it goes to the ground state In the 5001 Americium 241 is used as the source of alpha The 40 mCi source yields an average of 9 X 104 neutrons per second The Americium 241 also yields low energy gammas which are shi...

Page 62: ...ese and iron have a cross section a great deal higher than hydrogen These elements seldom cause errors with the exception of a few areas which have large amounts of boron coastal areas which may have significant sodium chloride in the soil and a few locations where iron oxide may be present in large amounts Helium 3 is an isotope that has a very large capture cross section for thermal neutrons and...

Page 63: ...to determine the values of E and F two moisture standards are required One may be zero since it is easy to obtain and the other must have a known amount of water or contain hydrogen which can be related to water The moisture measurement is sometimes called backscatter but once a neutron has been thermalized by multiple collisions with hydrogen it obeys gas diffusion laws and drifts off in any dire...

Page 64: ... that the predicted precision of the Gauge is directly related to the square root of the number of detector counts accumulated during a measurement Further the precision can be improved either by counting a longer time period or by averaging the count rate for a number of measurements and this improvement is the square root of the number of measurements made While the precision of the Gauge in cou...

Page 65: ...sture precision M Moisture MS Moisture standard count E F Calibration constants Both of the above previsions are stated for the one minute NORM measurement period The values would increase by a factor of two for 0 25 minutes FAST and decrease by a factor of two for the four minute SLOW measurement period These precisions are the theoretical values and the Gauge should yield these values if there a...

Page 66: ...ry small amounts and only a major accident to the Gauge could cause an immediate hazard care should be taken in its use in order to keep exposure as low as reasonably achievable Remember that short time and long distance are the most effective means of minimizing the user s exposure Refer to the Radiation Safety Manual for more complete details of safety procedures 8 1 Licensing Prior to receipt a...

Page 67: ...ay make the wipe and process the material 8 4 Transport Any equipment given to a common carrier for shipment must have a current negative leak test The shipper must have this record in his possession along with a certification that the capsule and transport container meet the US Department of Transportation requirements as specified in Title 49 Parts 172 and 173 of the Code of Federal Regulations ...

Page 68: ...epresent a safety hazard must also be reported 8 7 Radiation Profile The maximum surface and one meter exposure rates for this equipment are listed below in mRem h The Transport Index for the Transit Case and Gauge is Dose Rate in mRem hr 61 0 2 Transit Case Gamma Neutron Total Maximum any surface 10 50 1 50 12 00 Maximum at one meter 0 07 0 10 0 17 5001 Gauge Gamma Neutron Total Rear Surface 17 0...

Page 69: ...ured 08 05 88 using a Ludium Model 14C Survey Meter Neutron does rates were measured 08 05 88 using an Eberline Model PNR 4 Neutron Rem Counter with a 22 9 cm sphere on the gauge surface centerline was approximately 11 cm from the surface 0 0 indicates does rates same as background 62 ...

Page 70: ...FOR COMPLIANCE WITH LOCAL AND NATIONAL LAWS COVERING THE POSSESSION USE AND DISPOSAL OF THE MATERIALS THERE ARE NO WARRANTIES EXPRESS OR IMPLIED INCLUDING WITHOUT LIMITATION ANY IMPLIED WARRANTY OF MERCHANTABILITY OR FITNESS WHICH EXTENDS BEYOND THIS DESCRIPTION THIS EXPRESS WARRANTY EXCLUDES COVERAGE OF AND DOES NOT PROVIDE RELIEF FOR INCIDENTAL OR CONSEQUENTIAL DAMAGES OF ANY KIND OR NATURE INCL...

Page 71: ...64 ...

Page 72: ... com HUMBOLDT Testing Equipment for Construction Materials Humboldt Mfg Co 551 D Pylon Drive Raleigh NC 27606 1487 U S A U S A Toll Free 1 800 537 4183 Voice 1 919 832 3190 Fax 1 919 833 5283 Email hsi humboldtmfg com ...

Search results

Summary of Contents for HS-5001EZ

Reviews:

Related manuals for HS-5001EZ

Brands by name

Popular brands

Humboldt HS-5001EZ, Product Manual

Search results

Summary of Contents for HS-5001EZ

Reviews:

Related manuals for HS-5001EZ

Brands by name

Popular brands