Casio 3950P, Руководство по программированию

Страница: 41 / 48

39
28 Test on a mean with known
(z-tests)
µ
σ
2
The hypothesis of interest is that the mean is equal to a given value , versus the
alternative that it is not equal to . We calculate the test statistics
where is the sample mean and is the sample size. If , we reject the hypothesis
for the significance level .
For different significance levels, the confidence limits
are given below:
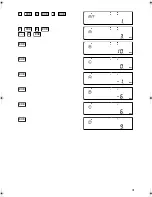
Program
？→ Ａ：？→ Ｂ：（ － Ａ）÷√（Ｂ ÷ ）→ Ｃ：Ｃ ＜
24 STEP
＞
: :
`
INPUT
Ａ
: given value
Ｂ
:population variance
OUTPUT
Ｃ
: value of
Z
Execution Example:
Test : =1.2
：
Data: 1.4, 1.5, 1.3, 1.4, 1.7, 1.5, 1.3 Population variance =0.02
µ
0
µ
0
Z
x
µ
–
σ
n
-------
------------ =
x n Z
1 .96
≥
α
0.05 =
α
Confidence limits
0.10
1.64
0.05
1.96
0.01
2.57
1.96
0
-1.96
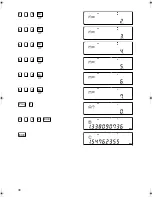
ON MODE MODE MODE 1
PRGM
MODE MODE 1
SD
1
P1
x n
x
SHIFT 2
S-VAR
1
x
n
SHIFT 1
S-SUM
3
n
µ
0
σ
2
H
0
µ
0
H
1
µ
0
1.2
≠
σ
2
関数電卓事例集 .book 39 ページ ２００２年９月２日 月曜日 午後６時５１分