58
KD2 Pro Operator’s Manual
8. KD2 Pro Theory
m
0
is the ambient temperature during heating (which could
include some offset for contact resistance and the heating
element being adjacent to the temperature sensor inside the
needle),
m
2
is the rate of background temperature drift, and
m
3
is the slope of a line relating temperature rise to
logarithm of temperature.
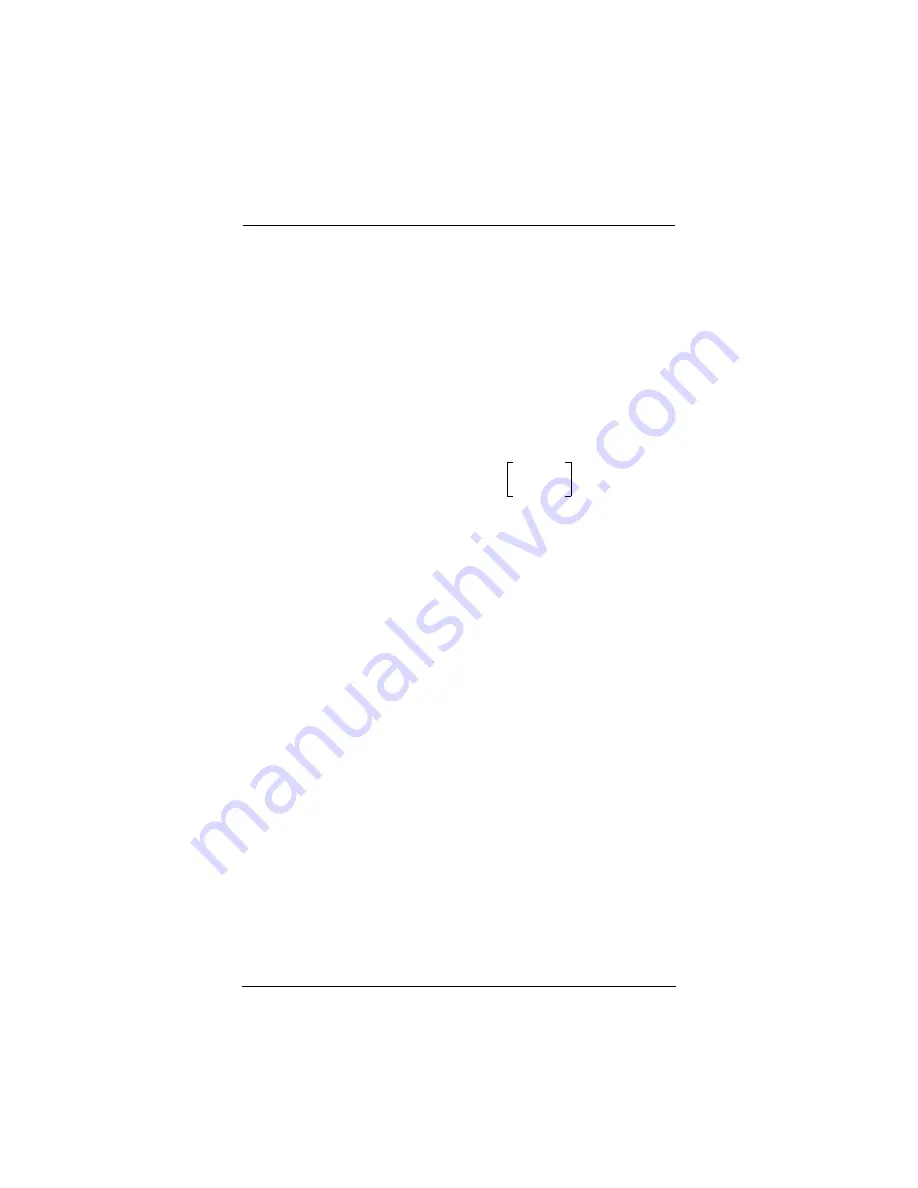
During cooling the model is
(6)
The thermal conductivity is computed from
(7)
Since these equations are long-time approximations to the
exponential integral equations (eq. 1), we use only the final 2/
3 of the data collected (ignore early-time data) during heating
and cooling. This approach has several advantages. One is
that effects of contact resistance appear mainly in these early-
time data, so by analyzing only the later time data the measure-
ment better represents the thermal conductivity of the sample
of interest. Another advantage is that equations 5 and 6 can be
solved by linear least squares, giving a solid and definite result.
The same data, subjected to a non-linear least squares analysis,
T
m
1
m
2
t
m
3
t
t
t
h
–
----------------
ln
+
+
=
k
q
4
m
3
-------------
=











































