Laboratory Teaching Exercises
25
d. Boundary
Conditions
Figure iii
If the conditions at all points of the boundary of the cross section of a soil
mass are fixed and clearly defined, the flow net is uniquely determined. An
example of such a case is represented by the situation shown in Figure ii.
Here the bottoms of basin I and II represent two equipotential lines, the
perimeter of the soil mass along the two walls and the bottom of the tank
represents one flow line, and the perimeter of the part of the impermeable
screen immersed in the soil represents another line.
These two flow lines and two equipotential lines fully determine the shape of
the flow net since there is only one shape of the net of which the above two
pairs of lines can be a part. Under such clearly specified conditions the flow
net is completely determined by its geometrical properties. It can be obtained
directly by a graphical, trial-and-error-sketching method.
Its determination does not require any additional mathematical solutions
(although such solutions exist for some types of boundary conditions). Nor is
it necessary to seek the correct flow line pattern experimentally.
There are, however, many cases of seepage where boundary conditions are
not so clearly defined, as in part b. Flow Nets above. A typical such case is
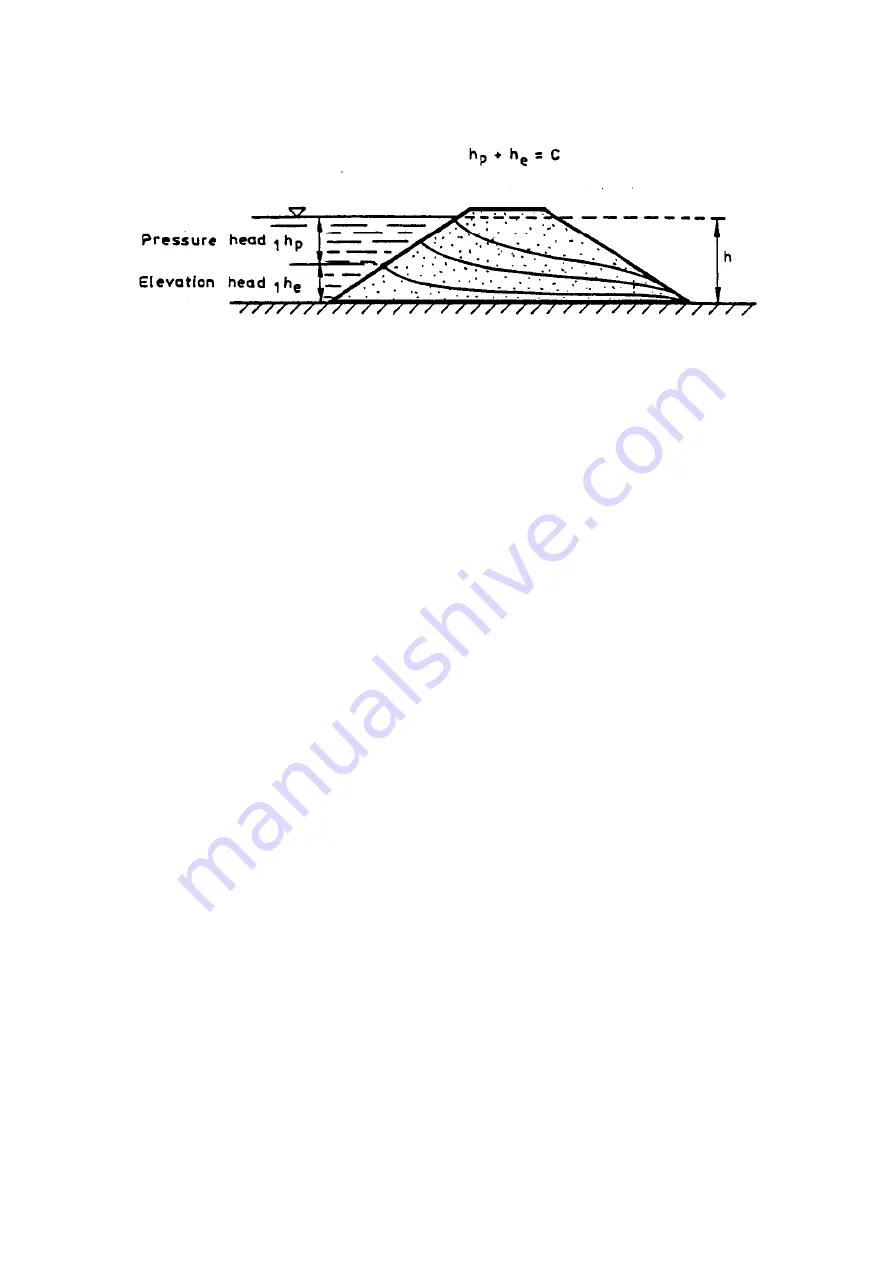
seepage through an earth dam shown above. Here the upstream slope of the
dam represents an equipotential line (the head h consists of the pressure, h
p
and elevation, h
e
heads whose sum is constant for all points of the slope).
Clearly, the impervious bottom represents one boundary flow line. The
second boundary flow line must start at the intersection of the slope with the
upstream water level.
However, since there are no physical obstacles in its path, its geometry is not
defined. The top flow line is under atmospheric pressure and it seeks its path
according to certain physical laws. These must be taken into account since
they act in the same way as the geometrical constraints that were present in
the previous case but missing in this.
To mathematically determine the shape of such free flow lines from the
governing physical laws is not always simple. For this reason an experiment
is often a very valuable and important alternative method of tackling the
problem.