3.6
Manual self-tuning
The optimization aid should be used with units on which the control parameters
shall be set without self-tuning.
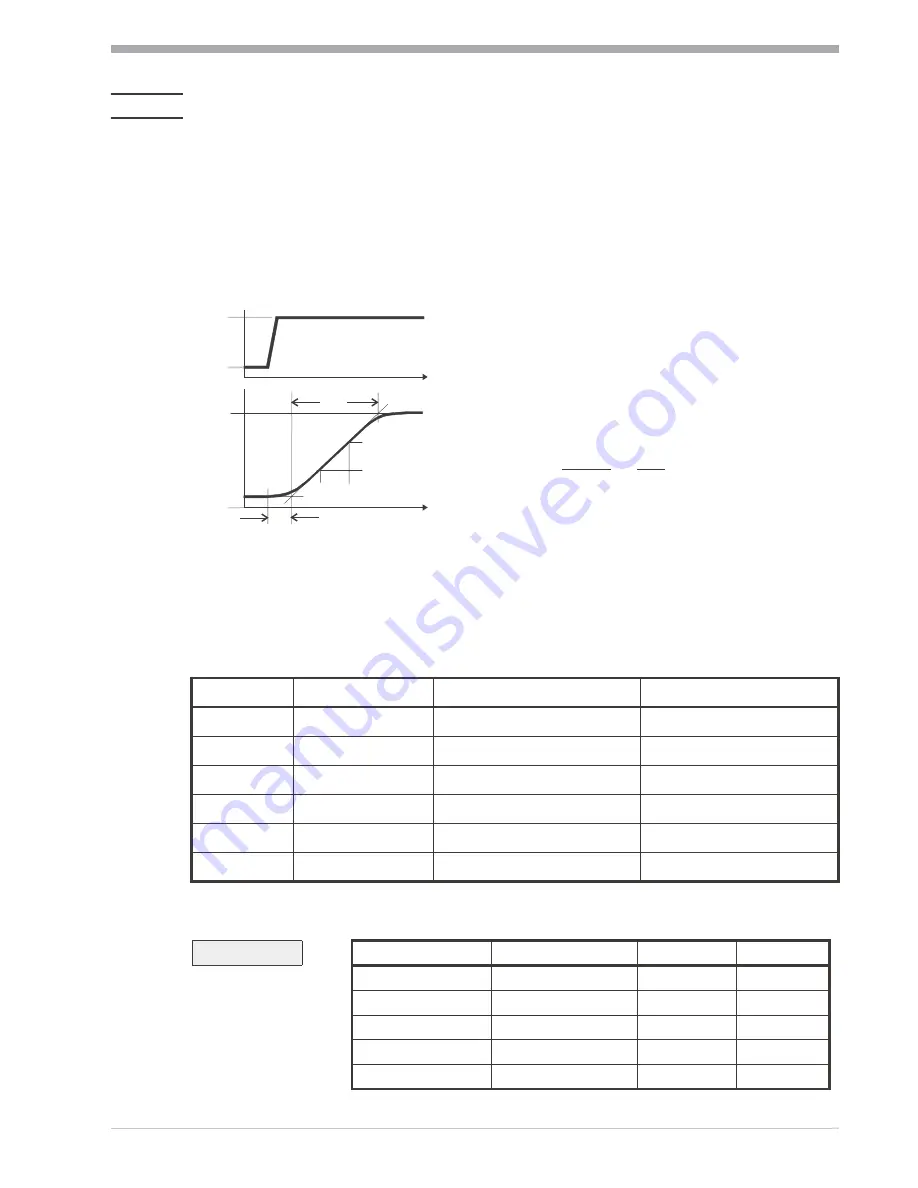
For this, the response of process variable x after a step change of correcting
variable y can be used. Frequently, plotting the complete response curve (0 to
100%) is not possible, because the process must be kept within defined limits.
Values T
g
and x
max
(step change from 0 to 100 %) or
∆
t and
∆
x (partial step
response) can be used to determine the maximum rate of increase v
max
.
The control parameters can be determined from the values calculated for delay
time T
u
, maximum rate of increase v
max
, control range X
h
and characteristic K
according to the
formulas
given below. Increase Xp, if line-out to the set-point
oscillates.
Operation
Operating manual MIR-491
19
Manual self-tuning
Formulas
K = Vmax * Tu
controller behavior
Pb1
[phy. units]
td1
[s]
ti1
[s]
With 2-point and
3-point controllers,
the cycle time must be
adjusted to
t1
/
t2
≤
0,25 * Tu
PID
1,7 * K
2 * Tu
2 * Tu
PD
0,5 * K
Tu
OFF
PI
2,6 * K
OFF
6 * Tu
P
K
OFF
OFF
3-point-stepping
1,7 * K
Tu
2 * Tu
Parameter adjustment effects
Parameter
Control
Line-out of disturbances
Start-up behaviour
Pb1
higher increased damping slower line-out
slower reduction of duty cycle
lower reduced damping
faster line-out
faster reduction of duty cycle
td1
higher reduced damping
faster response to disturbances faster reduction of duty cycle
lower increased damping slower response to disturbances slower reduction of duty cycle
ti1
higher increased damping slower line-out
slower reduction of duty cycle
lower reduced damping
faster line-out
faster reduction of duty cycle
Tu
Tg
t
x
y
100%
0%
t
Yh
Xmax
{
X
{
t
y
= correcting variable
Y
h
= control range
Tu
= delay time (s)
Tg
= recovery time (s)
X
max
= maximum process value
V
max
=
Xmax
Tg
=
{
{
x
t
=
max. rate of
increase of process value






























