InstroTek
55
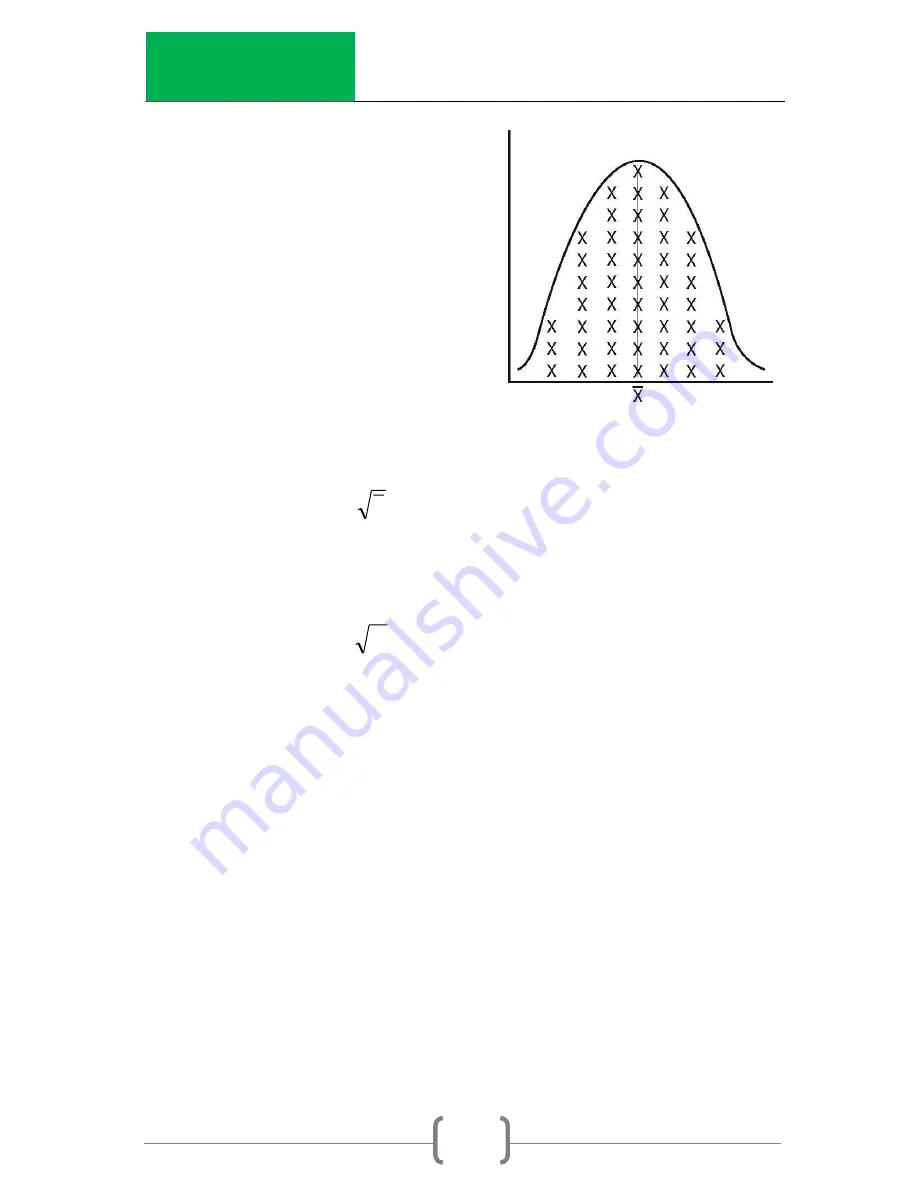
Fig D.2
Since the number of atoms is
very large and the expected
probability of a decay occurring
is very low (source life in years
and measurement time in
minutes), we can use the
Poisson distribution which is a
special case of the binomial
distribution for these conditions.
A special property of the Poisson
distribution is that the expected
standard deviation is equal to
the square-root of the average
value.
x
If the sample is large enough, we can approximate for the
standard deviation of the sample.
This is an important relationship. It means that if repeated
measurements are taken without moving the gauge and the
detector electronics are working properly, then the spread of
the counts will only be dependent upon the average count
rate. This is in contrast to most measurements where the spread
will depend upon the process. Figure D.3 shows the diameter of
a part turned on a new lathe while Figure D.4 shows the same
part turned on an old lathe. Both lathes produce a part with the
same average diameter, but a loose bearing caused the wider
spread for parts manufactured on the older lathe.
















































