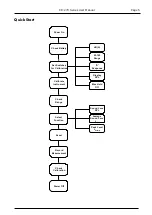
CR:270 Series User Manual
Page 17
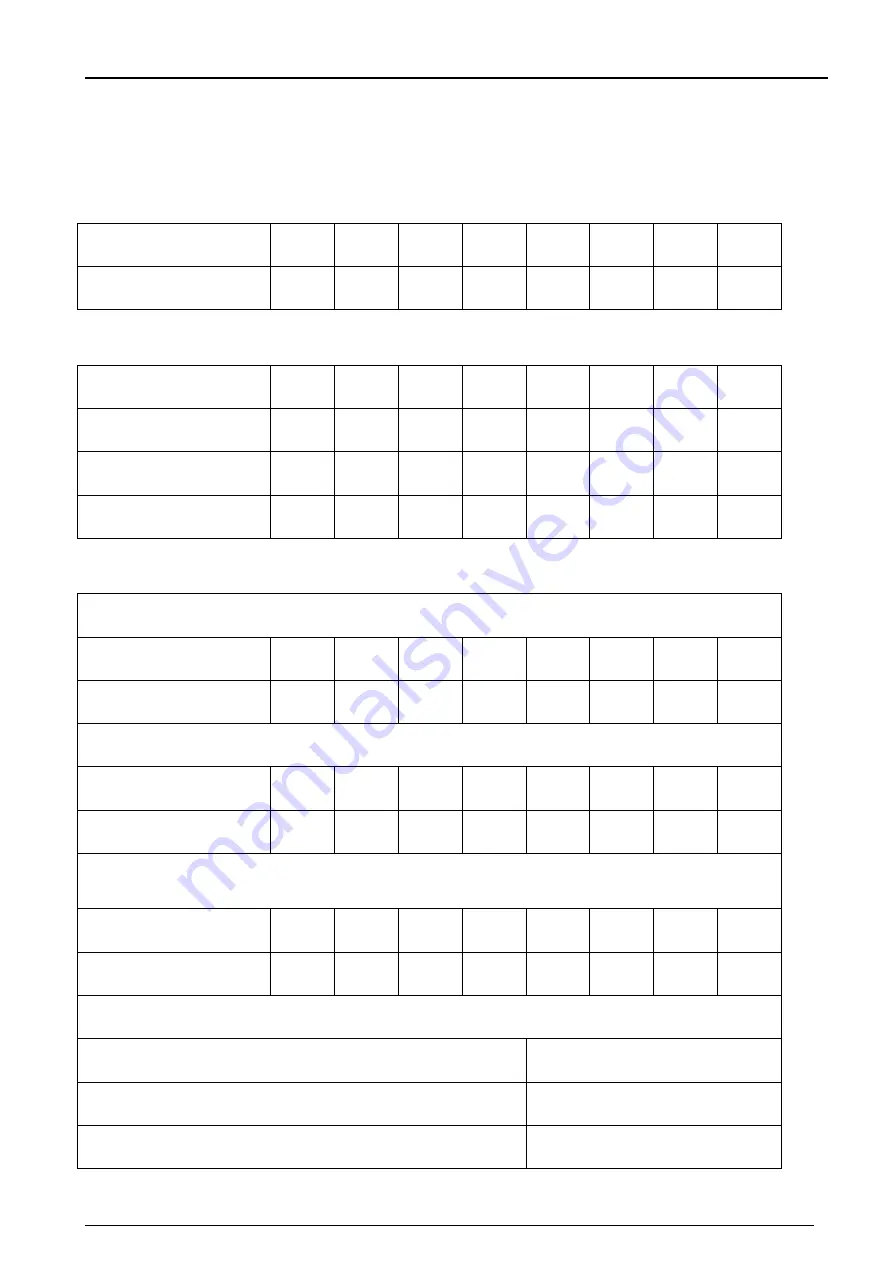
Example of the Octave Band Method
The noise levels measured from a machine are:
L
A
(or L
Aeq
in dB(A)) measured over one (or more) complete cycle:
103.2 dB(A)
Octave Band Centre
Frequency (Hz)
63
125
250
500
1000
2000
4000
8000
Unweighted SPL (dB)
90
92
94
94
96
98
96
94
The ear protector is supplied with the following octave band attenuation data:
Octave Band Centre
Frequency (Hz)
63
125
250
500
1000
2000
4000
8000
Mean Attenuation (dB) 7.4
10.0
14.4
19.6
22.8
29.6
38.8
34.1
Standard Deviation
(dB)
3.3
3.6
3.6
4.6
4.0
6.2
7.4
5.2
Assumed Protection
(APV) (dB)
4.1
6.4
10.8
15.0
18.8
23.4
31.4
28.9
The A-weighted sound pressure level at the ear can now be calculated using the APVs:
When the ear protection is worn, the level at the ear in each octave- band is calculated by
subtracting the APV's from the octave-band sound pressure levels
Octave Band Centre
Frequency (Hz)
63
125
250
500
1000
2000
4000
8000
SPL - APV
7.4
10.0
14.4
19.6
22.8
29.6
38.8
34.1
To calculate the effective A-Weighted sound pressure levels at the ear, L'
A
, A-weighting
factors need to be added to the protected sound pressure levels at each frequency.
A-Weighting Factors
(AW)
-26.2 -16.1 -8.6
-3.2
0.0
1.2
1.0
-1.1
L
A
' = (SPL - APV) +
AW
59.7
69.5
74.6
75.8
77.2
75.8
65.6
64.0
These A-Weighted levels must be summed. To do this the level must first be convereted
from decibels to a value related to energy: antilog (A weighted SPL at the ear/10), which
is equivalent to 10
(LA'/10)
L
A
'/10
5.97
6.95
7.46
7.58
7.72
7.58
6.56
6.4
Antilog (L'
A
/10)
933254 891250
9
288403
15
380189
40
524807
46
380189
40
363078
1
251188
6
Now these values can be summed and the result converted back to an A-weighted sound
pressure level using 10 x log (sum)
Sum:
173347371
A-weighted sound pressure level = 10 x log
10
(173347371)
82.4 dB(A)
Round to the nearest whole number
82 dB(A)