AREA AND VOLUME CALCULATIONS
Although many enclosures can now be purchased in prefabricated form, building your own enclosure is often fun,
acoustically rewarding, or even a necessity to properly fit many vehicles. In order to build such a box there MUST be a
basic understanding of mathematic principals or you will wind up wasting incredible amounts of time and money by making
mistakes in the box design and assembly.
B
ASIC
M
ATHEMATICS FOR
E
NCLOSURE
C
ONSTRUCTION
–
9
–
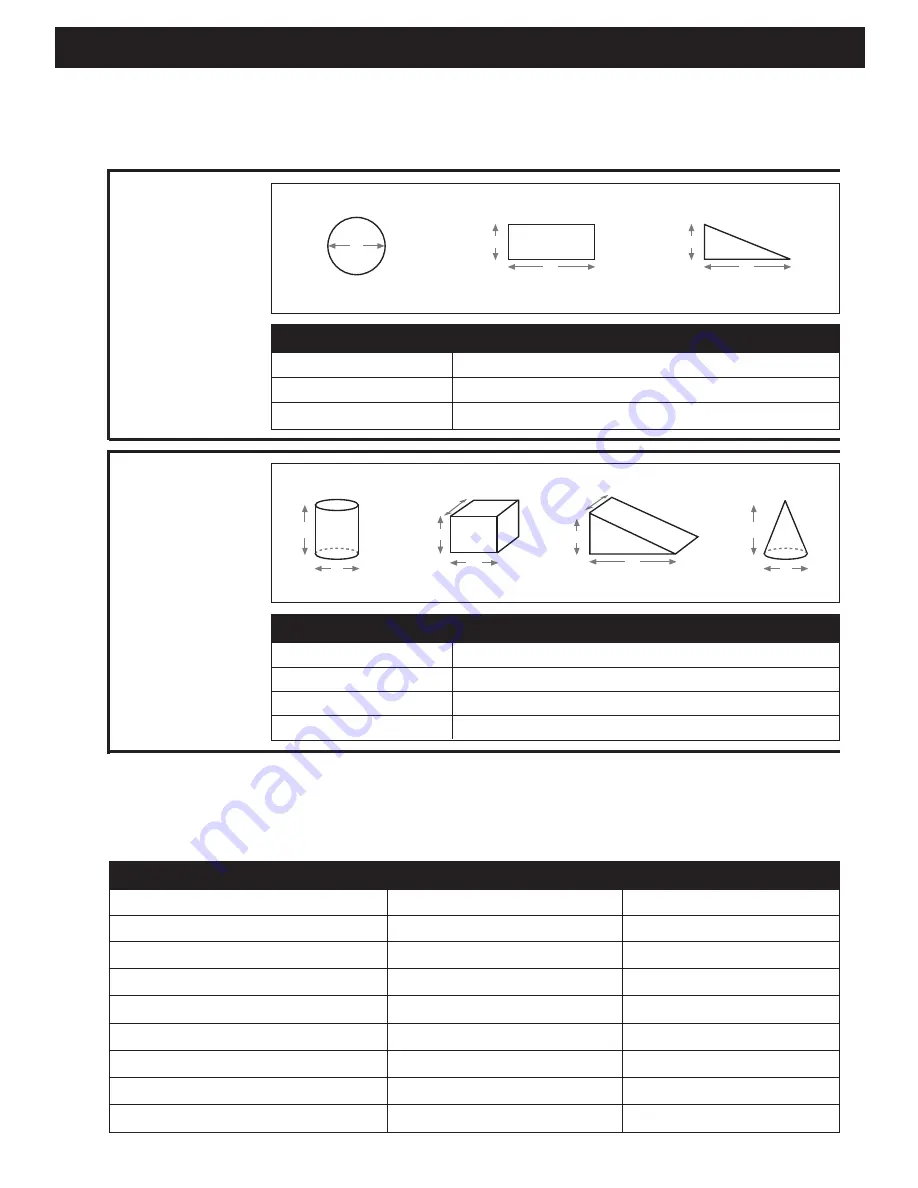
The areas of surfaces or
openings are calculated
in square inches (in2).
Such calculations
depend upon height
and width values or
diameters for circles
d
w
h
w
h
AREAS
circle or a hole
rectangular wall or area
triangle wall or area
AREA CALCULATIONS
Circle or hole
Area = 0.79 x d x d
Rectangular wall or area
Area = h x w
Triangle wall or area
Area = 0.5 x h x w
The volumes of
assemble shapes are
measured in cubic
inches (in3). Such
calculations depend
upon height, width, and
depth calculations for
rectangular shapes and
a diameter and height
for a tube shape.
AREA AND VOLUME CONVERSIONS
Technical data for speakers are often given in English and Metric unit values (e.g., inches and meters). Also, when
calculating dimensions we must often flip between size formats (e.g., cubic inches and cubic feet). To easily convert back
and forth between two formats you can use the conversion table below.
h
d
h
d
d
w
h
h
d
VOLUMES
tube shape
rectangular shape
triangular/wedge shape
cone shape
d
VOLUME CALCULATIONS
Cylinder (port tube)
Volume = 0.79 x d x d x h
Rectangular box
Volume = h x w x d
Triangular box
Volume = 0.5 x h x w x d
Cone shape
Volume = 0.26 x d x d x h
VOLUME AND AREA CONVERSIONS
TO CONVERT YOUR NUMBER FROM:
MULTIPLY YOUR NUMBER BY:
TO GET A NEW VALUE IN
Inches
0.0254
Meters
Meters
39.37
Inches
Square inches
0.007
Square feet
Square feet
144
Square inches
Cubic inches
0.00058
Cubic feet
Cubic feet
1728
Cubic inches
Liters
0.035
Cubic feet
Cubic feet
28.3
Liters




































