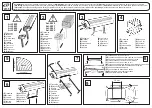
Physical Pendulum Accessory
Model No. ME-9858
8
®
Theory
m
o
= mass of car (including bumper and masses)
M= mass of pendulum
v
o
= speed of the car just before collision
l
= length of physical pendulum (ignoring bumper pin)
x= distance from center of mass (c.m.) to pivot (calculated)
d= distance from pivot to (non-bumper) end of pendulum
(directly measured by student)
r = distance from pivot to rubber band on bumper (directly
measured by student)
I = rotational inertia of pendulum about pivot (calculated)
1. Center of Mass
The center of mass of the physical pendulum can experimentally be found by balancing it on
a knife’s edge, but since the pins have negligible mass, you can assume its center of mass is
at the center (
l
/2). Thus the distance, x (Figure 7), the distance from the center of mass to
the pivot point is
(1).
2.Rotational Inertia
The rotational inertia of a thin rod (total length
l
) about its center of mass is
If the axis of rotation is not about the center of mass, but is instead about a parallel axis a
distance
x
from the center of mass, the Parallel Axis Theorem states that the new rotational
inertia is
Thus, combining these two gives the rotational inertia of the physical pendulum as
x
x
pivot pin
center of
mass
d
l
Figure 7
x=
l
-d
2
1
I
cm
= M
l
2
12
1
(2).
I
I
cm
Mx
2
+
=
(3).
I= M
l
2
+ Mx
2
12
1
(4).