Shock Accelerometer
Page 18
002-096e-01.19
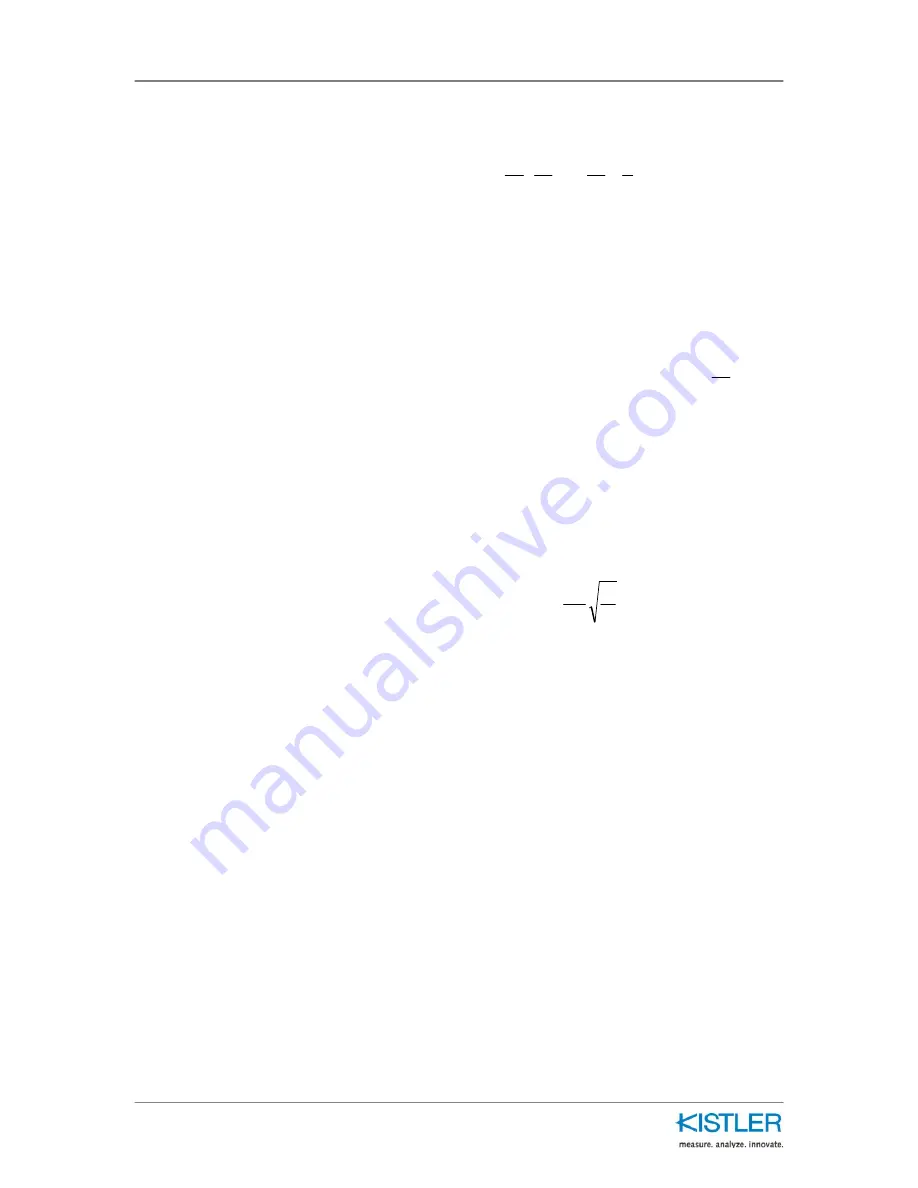
Where:
Quartz accelerometers have a Q of approximately 10 to 40 and
therefore the phase angle can be written as:
Using the basic equation for resonance one can better understand the
basic accelerometer mechanical system and the affect on high
frequency response.
The spring constant (k) is determined by the stiffness of the quartz
crystal and preload stud. The mass (m) is the supported part of the
accelerometer structure. This spring-mass system is the basic
component that converts the input forces into acceleration. As with
any single degree-of-freedom system, a resonance condition will occur
(per the above equation) which causes the sensitivity to increase with
frequency.
It is desirable to have a uniform frequency response over the specified
frequency range. As can be seen, (Figure 13), the sensitivity greatly
increases when approaching resonance. About a 5% amplitude rise
can be expected at 9/40 of the resonant frequency. It is possible to
operate above the maximum specified frequency by applying the
appropriate correction factors, or filtering to compensate for the
resonant rise in response.
resonance
at
increase
amplitude
of
factor
=
1
on
accelerati
reference
of
base
mounting
=
on
accelerati
output
=
(Hz)
curve
the
of
point
given
any
at
frequency
=
(Hz)
frequency
(resonant)
natural
undamped
=
Q
fn
f
a
a
f
f
b
o
n
system
freedom
of
degree
single
a
of
5
2
60
(deg)
Lag
Phase
n
n
f
f
for
f
f
Q
m
k
f
n
2
1





















