The Geometer’s Sketchpad
®
for TI-89, TI-92 Plus, and
© 2000-2002 KCP Technologies, Inc.
15
Voyage™ 200 PLT User Guide and Reference Manual
Next, you’ll make some conjectures about the coordinates of the vertices of
your isosceles triangle when it’s located in different ways on the
coordinate grid. You’ll also try to solve some challenges about the sketch.
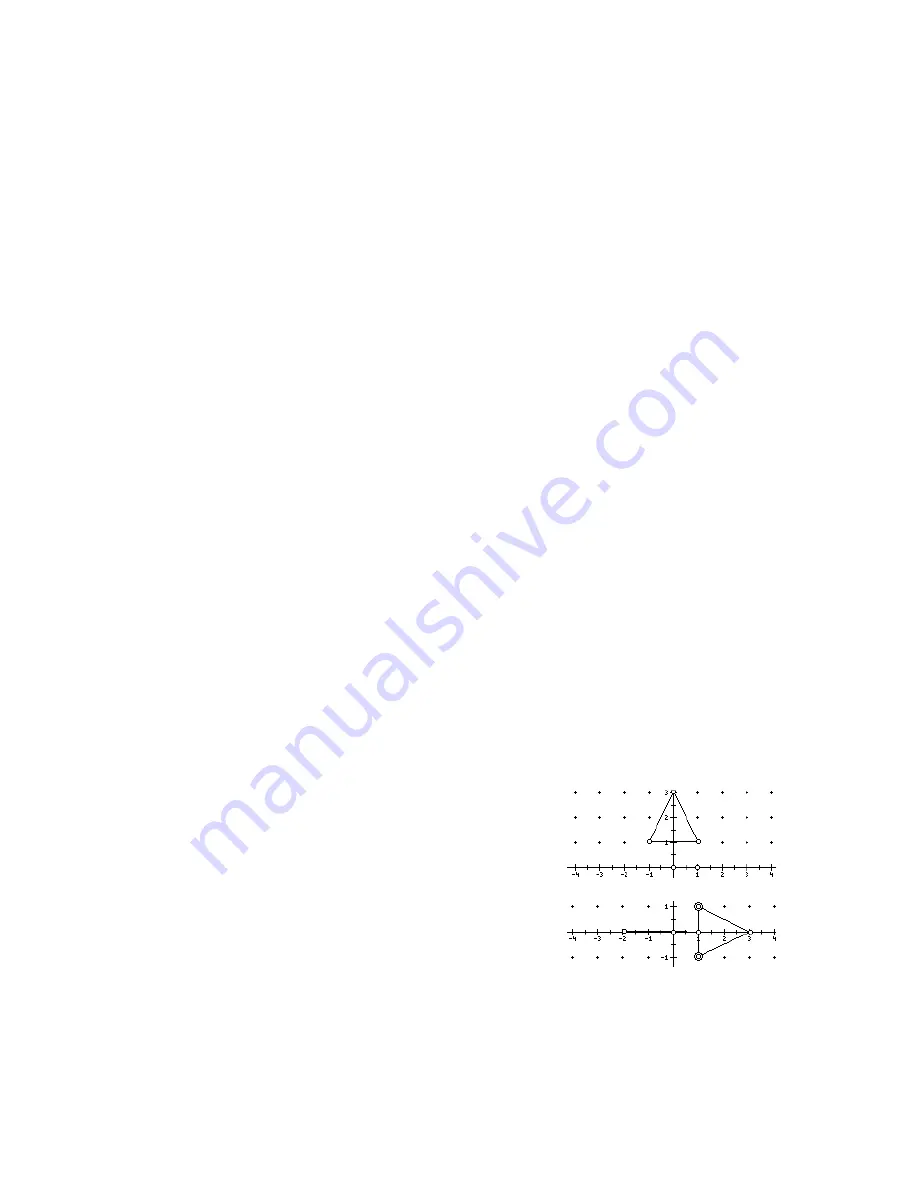
1. Drag the mirror line’s control points so that they lie on the y-axis.
Drag the triangle base vertices around and observe the coordinates. If
a point has coordinates (a, b), what are the coordinates of its mirror
image across the y-axis?
2. Locate the mirror line on the x-axis. Drag the triangle base vertices
around and observe the coordinates. If a point has coordinates (a, b),
what are the coordinates of its mirror image across the x-axis?
3. Drag the mirror line’s control points so that the line’s equation is
y = x. Drag the triangle base vertices around and observe the
coordinates. If a point has coordinates (a, b), what are the coordinates
of its mirror image across the line y = x?
4. See if you can locate the three vertices of the triangle so that each one
has 0 as one of its coordinates. Describe where you located the points.
5. See if you can locate the three vertices of the triangle so that one
vertex is at the origin and each of the two other vertices has 0 as one
of its coordinates. Describe where you located the points and describe
the triangle.
6. Locate the mirror line so that its equation is y = 2x. See if you can
locate the base vertices so that they both lie on the grid—that is, so
that they both have whole-number coordinates. Describe how you did
this. (Hint: You might find this easier if you construct the midpoint of
the base first.)
In this tour and the previous introductory tour, you’ve seen two approaches
to constructing and investigating an isosceles triangle. In the introductory
tour you took a Euclidean approach to the construction, using the drawing
tools and the Construct menu. In this tour you took a Transformational
approach, using a reflection in your construction. You also analyzed your
figure in this tour using a coordinate system. In the process, you were
introduced to the power of Dynamic Geometry as well as all of
Sketchpad’s menus and most of its tools. You may also have discovered
something new about geometry!
The answers to Conjectures and Challenges follow.
1.
(–a, b)
2.
(a, –b)
Conjectures and
Challenges
Summary






























