HP 33S, User Manual

Page: 243 / 388

Mathematics Programs
15–21
File name 33s-English-Manual-040130-Publication(Edition 2).doc Page : 388
Printed Date : 2004/1/30 Size : 13.7 x 21.2 cm
b
0
= a
0
(4 a
2
– a
3
2
) – a
1
2
.
Let y
0
be the largest real root of the above cubic. Then the fourth–order polynomial
is reduced to two quadratic polynomials:
x
2
+ ( J + L ) x + ( K + M ) = 0
x
2
+ ( J – L ) x + ( K – M ) = 0
where J = a
3
/2
K = y
0
/2
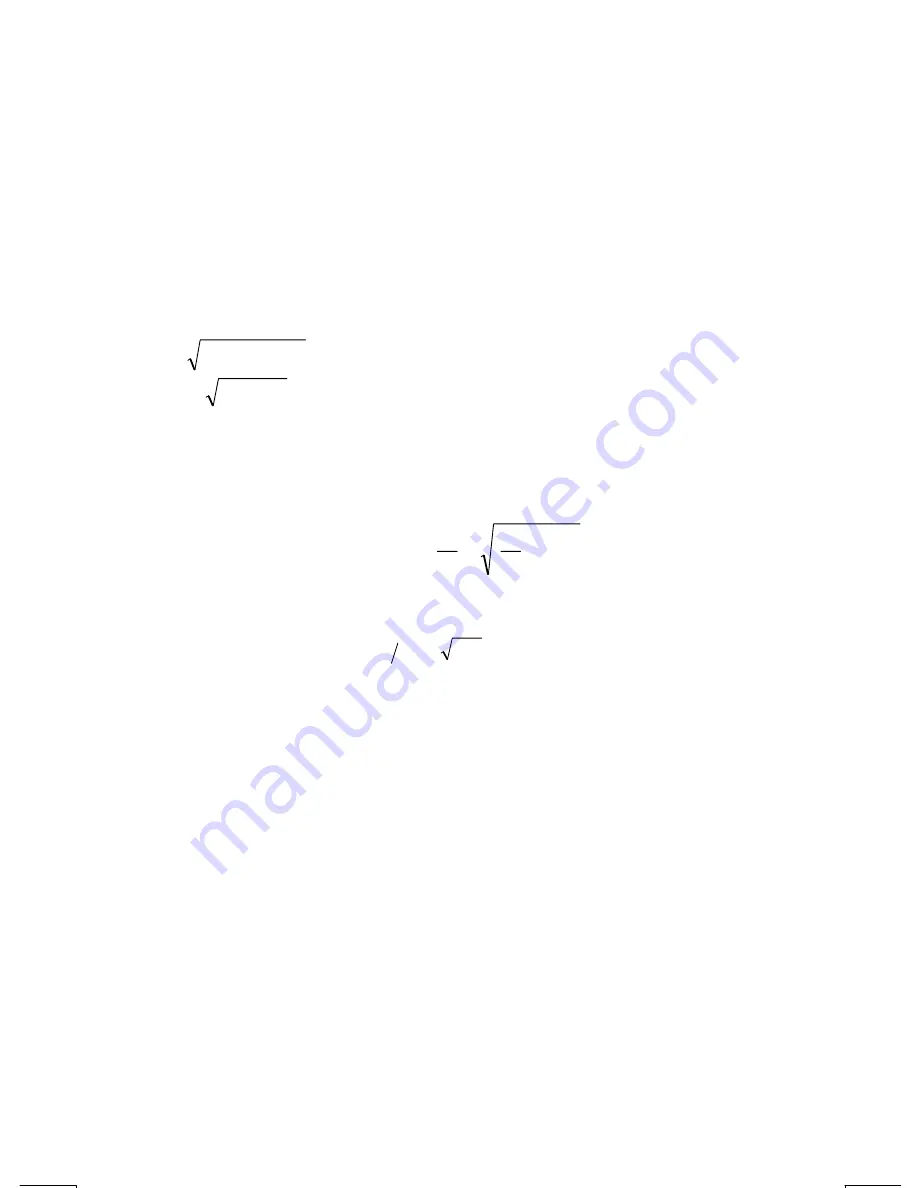
L =
0 2
2
y a J
+ −
×
(the sign of JK – a
1
/2)
M =
0
2
a K
−
Roots of the fourth degree polynomial are found by solving these two quadratic
polynomials.
A quadratic equation
x
2
+
a
1
x +
a
0
= 0 is solved by the formula
0
2
1 1
2 , 1
)
2
(
2
a
a a
x
− ± − =
If the discriminant d = ( a
1
/2)
2
– a
o
≥
0, the roots are real; if d
<
0, the roots are
complex, being
d i a iv u
− ± − = ±
) 2 (
1
.