Harmonic Drive SHA Series, Manual

Page: 79 / 109

2-3 Verifying and examining load weights
79
1
2
3
4
5
6
7
8
9
10
11
付
S
e
le
c
tion gui
de
lin
e
s
Cross roller bearing life with oscillating motion
Use formula (8) to calculate the cross roller bearing life against oscillating movement.
If the oscillating angle is 5° or less, fretting wear may occur because the oil film does not form
effectively on the contact surfaces between the race and rolling elements of the cross roller bearing. In
such cases, consult with our engineering staff.
Verifying static safety coefficients
Static equivalent radial load
Static safety coefficient
Generally, the static equivalent load is limited by the basic static rated load(Co). However, the specific
limit should be calculated according to the using conditions and required conditions. In this case,
calculate the static safety coefficient (fs) by formula (10).
Table 4 shows general values representing using conditions. Calculate the static equivalent radial load
(Po) by formula (9).
◆
Formula (8): Cross roller bearing life (oscillating)
10/3
6
Pc fw
C 90
n 60
10
Loc
1






⋅
×
θ
×
×
=
Symbols used in the formulas
Loc Life hour
―
n
1
Number of reciprocating
oscillation per min.
cpm
―
C Basic dynamic rated load N Refer to Table 1.
Pc Dynamic equivalent radial load N Obtained by formula (6).
fw Load coefficient
－
Refer to Table 3.
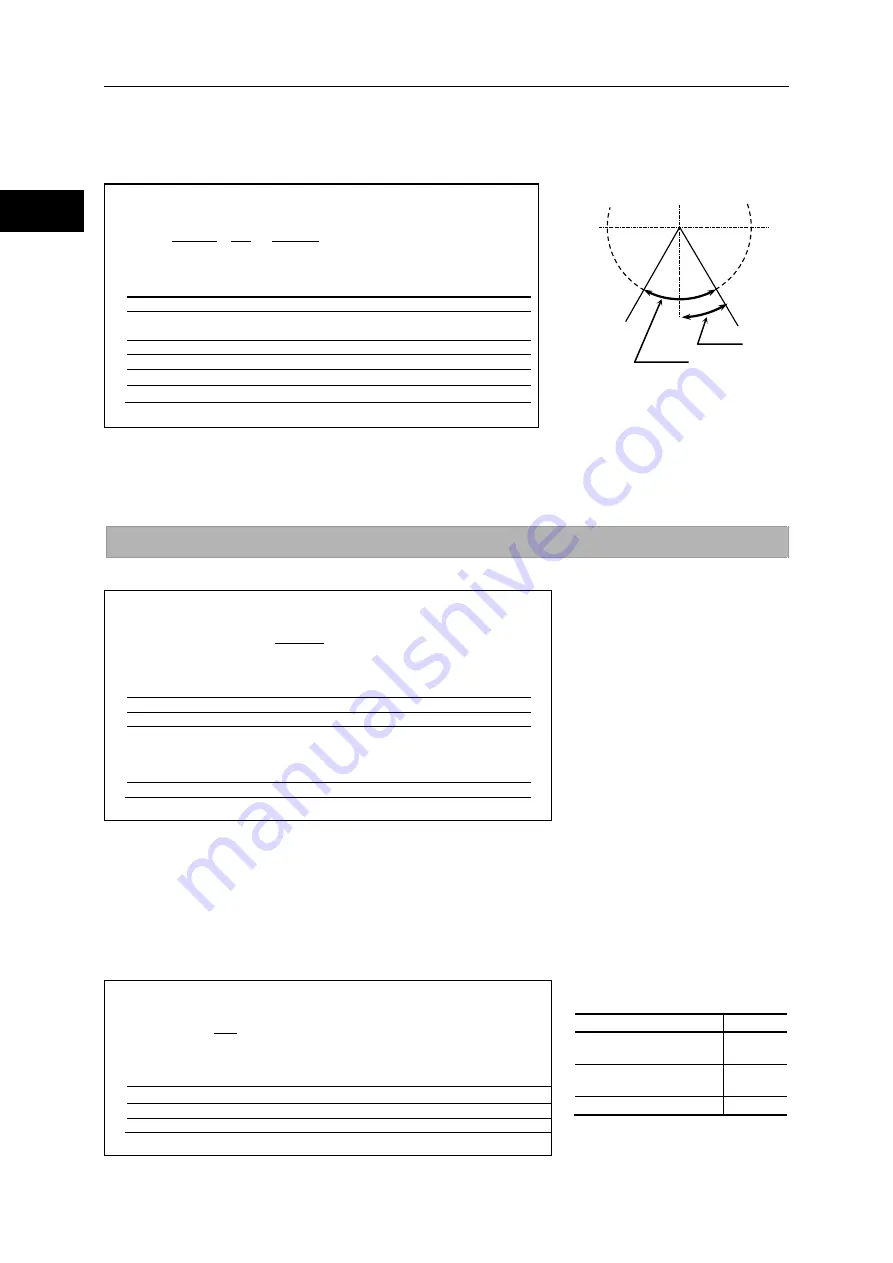
θ
oscillating angle/2
－
Refer to Fig.3.
◆
Formula (10): Static safety coefficient
Po
Co
fs
=
Symbols used in the formulas
fs Static safety coefficient
－
Refer to Table 4.
Co Basic static rated load N Refer to Table 1.
Po Static equivalent radial load N Obtained by formula (9).
◆
Formula (9): Static equivalent radial load
max
max
max
Fa 44 . 0
dp
2M
Fr Po
+ + =
Symbols used in the formulas
Fr max Max. radial load N Refer to Fig.1.
Fa max Max. axial load N Refer to Fig.1.
M max Max. moment load Nm
Refer to the
maximum load
weight calculation
methods.
dp Pitch circle diameter of a roller mm Refer to Table 1.
Table 4: Static safety coefficients
Using conditions fs
High rotational accuracy
is required, etc.
≧
3
Operation subject to
impact/vibration
≧
2
Normal operation
≧
1.5
Oscillating
angle
θ
Fig. 3: Oscillating movement