50 | Description of the menu
Power Quality Controller – PQC | Operating Manual | FRAKO
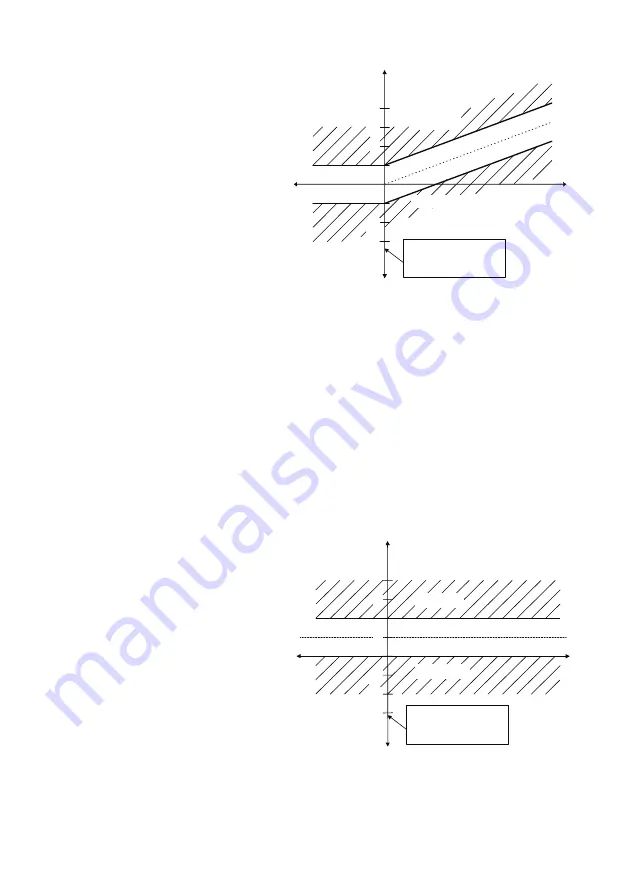
Control characteristic at
cos
φ
target
= 0.92 ind
ind Limitation = 0
Parallel shift = 0
If the system operates outside the
band range, the PQC will try to
return to within the band range with
the minimum number of switching
cycles.
In this diagram, the action of the
PQC during regeneration (feed-in
to the supply network) can be seen. The ‘kink’ in the band (characteristic line) is not
reflected in the regeneration quadrants. Instead, the band extends laterally from the
point where it crosses the reactive power axis (y axis).
By shifting the band into the capacitive range (see Parallel shift below), inductive reac-
tive power during regeneration can be avoided almost completely. When a capacitive
cos
φ
target
is set, the control band is a mirror image from the power draw side to the
regeneration side (see 3rd diagram in the
subsection
).
Parallel shift
This setting causes a parallel shift of the band range shown above through the set
value. It will shift in the inductive direction if a plus sign is used, and in the capacitive
direction if a minus sign is used.
The values -2 to +4 can be set in increments of 0.5. The effects are illustrated by the
two examples in the following diagrams:
Control characteristic at
cos
φ
target
= 1
Limitation = 0
Parallel shift = +1.0 (inductive)
The set cos
φ
target
is therefore the
upper limit of the control band.
Overcompensation is avoided.
Active power
Reactive power
ind
1
3
cap
Regeneration
Switch in
Switch out
One division =
0.65 × smallest cap.
stage
-2
-3
-1
Active power
Reactive power
ind
3
2
cap
Regeneration
Switch in
Switch out
One division =
0.65 × smallest cap.
stage
-3
-2
Active power
Reactive power
ind
1
3
cap
Regeneration
Switch in
Switch out
One division =
0.65 × smallest cap.
stage
-2
-3
-1
Active power
Reactive power
ind
3
2
cap
Regeneration
Switch in
Switch out
One division =
0.65 × smallest cap.
stage
-3
-2






























