22
AIRMOUNT
®
VIBRATION ISOLATION
b) What exact isolation will be attained?
Use the formula:
Where: f
f
= Forced Frequency
f
n
= Natural Frequency
The forced frequency is 850 cpm Read the natural frequency
from the line at the load and pressure closest to the actual situ-
ation, or 106 CPM (@ 80 psig and 4,280 Ibs.):
% Transmission = 1.6%
% Isolation = 100 – % Transmission
% Isolation = 100 – 1.6
% Isolation = 98.4%
Notice that the natural frequency of an Airmount changes only
slightly with variations in pressure and load. Therefore, when
working at pressures other than 40, 60, 80, or 100 psig, % iso-
lation can be calculated quite accurately using the “closest”
natural frequency and the formula above.
DYNAMIC SPRING RATE FORMULA
Spring rate is a different matter. Unlike most conventional
springs, the rate of an Airmount is not constant. It is a function
of the change in effective area, volume, and pressure from
design height. To determine the rate of an Airmount, use the
following formula:
K=[P
g
+14.7] A
c
1.38
–A
e
1.38
–14.7(A
c
–A
e
)
WHERE:
K = Vertical Spring Rate in Ibs./inch
P
g
= Gauge Pressure at design height
A
c
= Effective Area at
1
/
2
inch below design height (in
2
)
A
e
= Effective Area at
1
/
2
inch above design height (in
2
)
V
1
= Internal Volume at design height (in
3
)
V
c
= Internal Volume at
1
/
2
inch below design height (in
3
)
V
e
= Internal Volume at
1
/
2
inch above design height (in
3
)
Consider the same #22 example: What is the vertical spring
rate with a load of 4,100 pounds at a design height of 9.5
inches? Refer to the static data chart on page 61. Again,
our “closest" pressure is 80 psig, so we'll need to read the
appropriate data from the 80 psig curve.
The 80 psig information at
1
/
2
inch above design height
would fall at the 10.0 inch height line, and
1
/
2
inch below
design height would fall at the 9.0 inch height line. (In this
example, we can read loads from the force table). The
information at design height is located in the “Dynamic
Characteristics Block.” So,
K = Unknown
P
g
= 76.6 psig (see page 13)
V
1
= 782 in
3
V
c
= 752 in
3
V
e
= 809 in
3
K=[P
g
+14.7] A
c
1.38
–A
e
1.38
–14.7(A
c
–A
e
)
K=[76.6+14.7] 58.4
1.38
–47.6
1.38
–14.7
(58.4–47.6)
K = 1,324 lbs/inch
NATURAL FREQUENCY FORMULA
Once the spring rate is determined, calculate the Airmount
natural frequency (for an
undamped system) as follows:
Where:
f
n
= Natural Frequency in cycles per minute (cpm)
K = Rate (Ibs/inch)
L = Load (pounds)
in our example:
f
n
= 106.8 cpm
( )
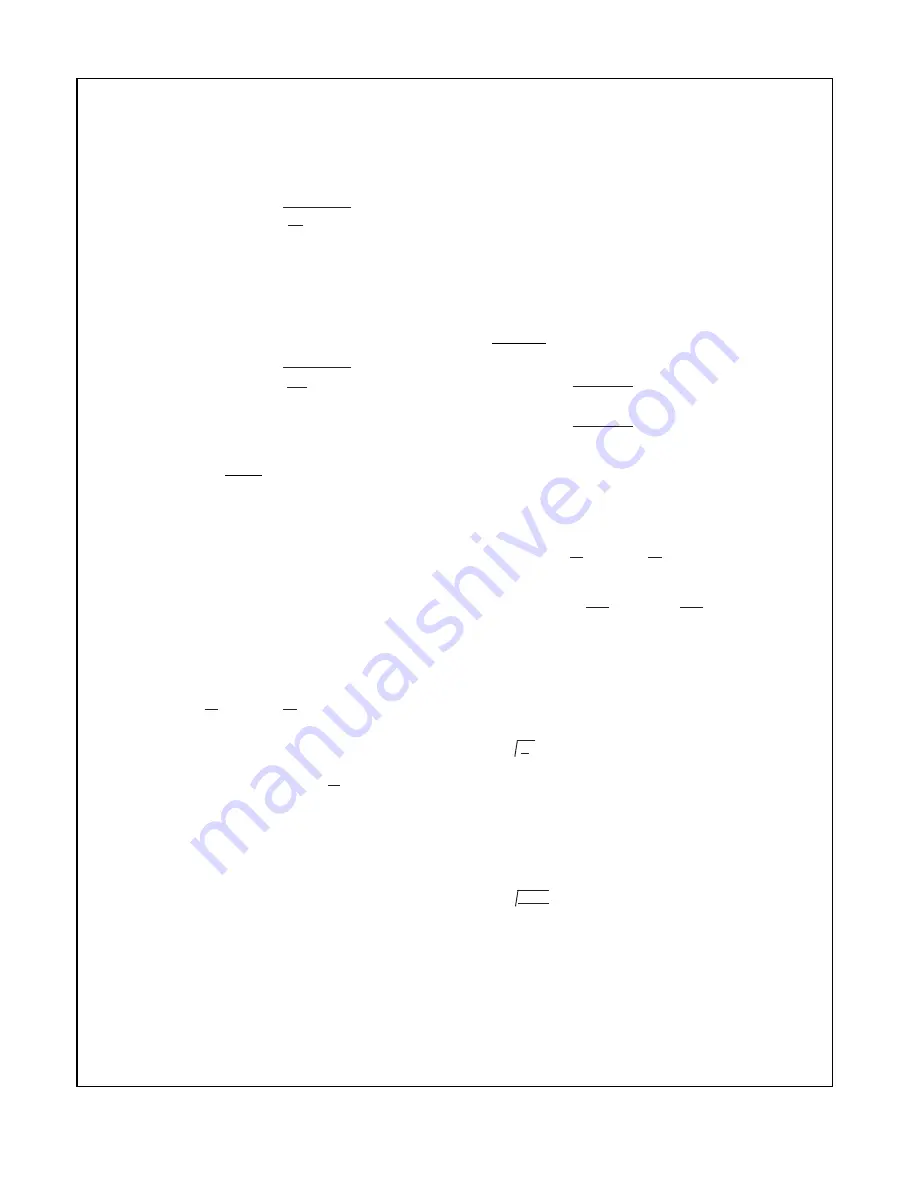
% Transmission = 100
f
f
f
n
– 1
2
( )
% Transmission = 100
106
– 1
2
850
[ ( ) ( ) ]
V
1
V
c
V
1
V
e
[ ( ) ( ) ]
V
1
V
c
V
1
V
e
[ ( ) ( ) ]
782
752
782
809
( )
lbs
in
2
n
A = 58.4 in
2
c
( )
4,670 lbs.
80 lbs/in
2
A = 47.6 in
2
e
( )
3,810 lbs.
80 lbs/in
2
f = 188
√
K
L
n
f = 188
√
1,324
4,100
















































