R&S 1332.4500K22 Скачать руководство пользователя | Manualshive

Страница: 223 / 1709

background image

Concepts and features

R&S

®

ZNA

223

User Manual 1178.6462.02 ─ 20

Table 4-18: Properties of frequency windows

Window

Side lobe sup-
pression

Relative impulse
width

Best for...

No Profiling (Rect-
angle)

13 dB

1

–

Low First Side lobe
(Hamming)

43 dB

1.4

Response resolution: separation of
closely spaced responses with compara-
ble amplitude

Normal Profile
(Hann)

32 dB

1.6

Good compromise between pulse width
and side lobe suppression

Steep Falloff (Boh-
man)

46 dB

1.9

Dynamic range: separation of distant
responses with different amplitude

Arbitrary Side lobes
(Dolph-Chebychev)

User defined
between 10 dB and
120 dB

1.2 (at 32 dB side
lobe suppression)

Adjustment to individual needs; tradeoff
between side lobe suppression and
impulse width

4.7.2.4

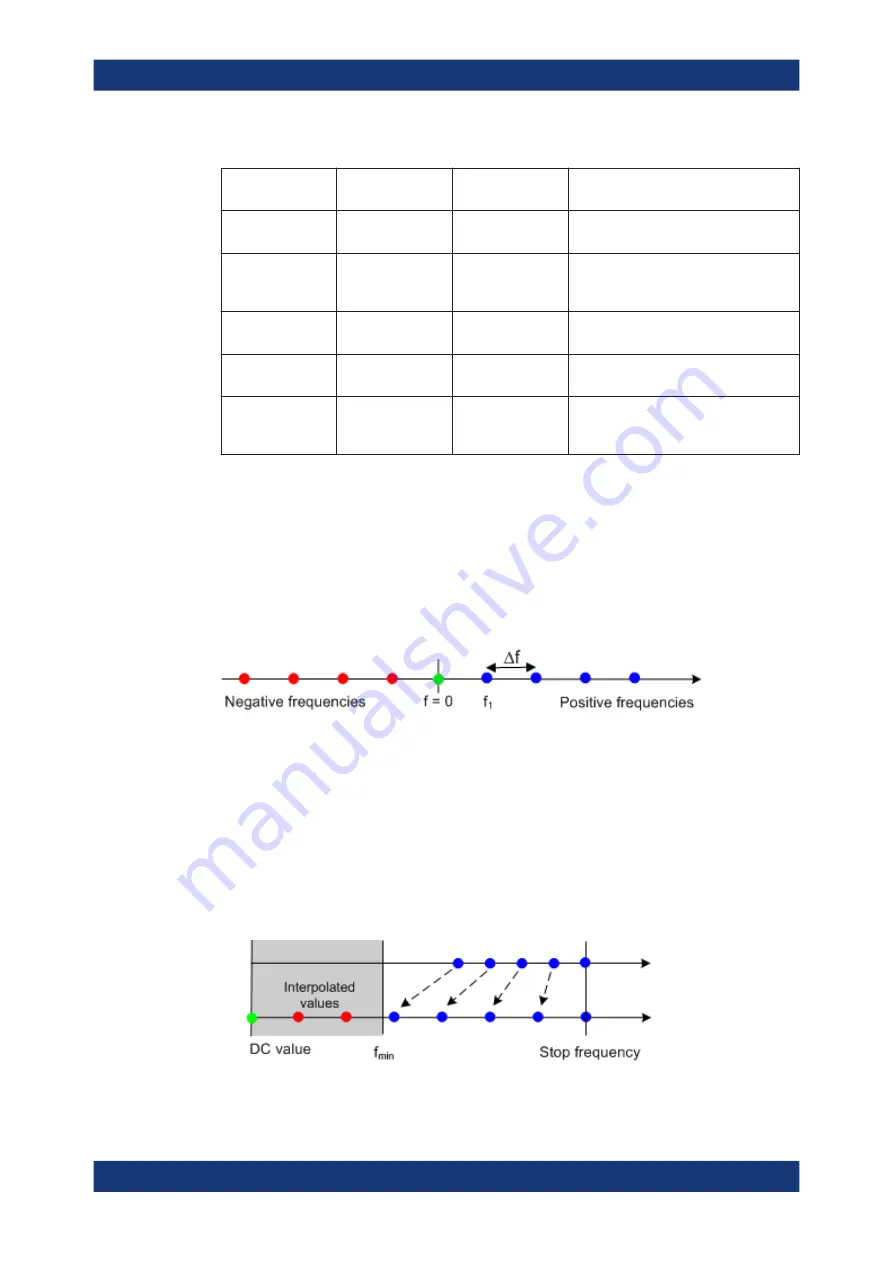
Harmonic grid

A harmonic grid is formed by a set of equidistant frequency points f

i

(i = 1...n) with

spacing Δf and the additional condition that f

1

= Δf. In other words, all frequencies f

i

are

set to harmonics of the start frequency f

1

.

If a harmonic grid, including the DC value (f = 0), is mirrored to the negative frequency
range, the result is again an equidistant grid.

The point symmetry with respect to the DC value makes harmonic grids suitable for
lowpass time domain transformations.

Visualization of the harmonic grid algorithms

The R&S

ZNA provides three different algorithms for harmonic grid calculation. The

three harmonic grids have the following characteristics:

●

Keep "Stop Frequency and Number of Points" means that the stop frequency and
the number of sweep points is maintained. The sweep points are redistributed
across the range between the minimum frequency of the analyzer and the stop fre-
quency; the step width can be increased.

●

Keep "Frequency Gap and Number of Points" means that the number of sweep
points and their relative spacing is maintained. If the start frequency of the sweep

Optional extensions and accessories

«
...
221
222
223
224
225
...
»

Содержание 1332.4500K22

Страница 1: ...R S ZNA Vector Network Analyzers User Manual 1178646202 Version 20 2...

Страница 2: ...S ZNA50 10 MHz to 50 GHz 2 test ports 2 4 mm order no 1332 4500K52 R S ZNA50 10 MHz to 50 GHz 4 test ports 2 4 mm order no 1332 4500K54 R S ZNA67 10 MHz to 67 GHz 2 test ports 1 85 mm order no 1332 4...

Страница 3: ...safety instructions 25 2 2 6 Data sheets and brochures 25 2 2 7 Release notes and open source acknowledgment OSA 25 2 2 8 Application notes application cards white papers etc 25 2 3 About this help 25...

Страница 4: ...ment 75 3 4 2 Reflection S parameter measurement 82 4 Concepts and features 84 4 1 Basic concepts 84 4 1 1 Global persistent settings 84 4 1 2 Softtools 85 4 1 3 Traces channels and diagrams 85 4 1 4...

Страница 5: ...arameters and de embedding 201 4 6 1 Offset parameters 201 4 6 2 Embedding and deembedding 207 4 7 Optional extensions and accessories 219 4 7 1 Spectrum analyzer mode 220 4 7 2 Time domain analysis 2...

Страница 6: ...r receiver access 277 4 7 27 Additional removable system drive 278 4 7 28 Source step attenuators 278 4 7 29 Direct IF access 278 4 7 30 Receiver step attenuators 279 4 7 31 Internal pulse modulators...

Страница 7: ...b frequency converting DUT 370 5 3 Format softtool 374 5 4 Scale softtool 380 5 4 1 Scale Values tab 380 5 4 2 Scale Coupling tab 383 5 4 3 Zoom tab 384 5 5 Traces softtool 386 5 5 1 Traces tab 386 5...

Страница 8: ...d tab 471 5 7 9 Marker Coupling tab 472 5 8 Stimulus softtool 473 5 8 1 Stimulus tab 473 5 8 2 Power tab 476 5 8 3 Time Domain X Axis tab 482 5 9 Pwr Bw Avg softtool 484 5 9 1 Power tab 484 5 9 2 Band...

Страница 9: ...67 5 14 4 Single Ended tab 669 5 14 5 Fixture modeling dialog 672 5 14 6 Port Sets tab 687 5 14 7 Balanced tab 690 5 14 8 Ground Loop tab 693 5 14 9 Differential Match tab 695 5 14 10 Config tab 697 5...

Страница 10: ...etup tab 835 5 19 8 Generic Device tab 836 5 20 Help softtool 841 5 21 Preset softtool 842 5 21 1 Select Preset tab 842 6 Remote control 845 6 1 Introduction to remote control 845 6 1 1 Remote control...

Страница 11: ...atus register 867 6 5 3 Contents of the status registers 869 6 5 4 Application of the status reporting system 875 6 5 5 Reset values of the status reporting system 878 7 Command reference 880 7 1 Spec...

Страница 12: ...ilization commands 1559 7 4 5 Service date commands 1562 7 5 R S ZVR ZVABT compatible commands 1564 8 Programming examples 1590 8 1 Basic tasks 1590 8 1 1 Typical stages of a remote control program 15...

Страница 13: ...and disposal 1638 11 1 Cleaning 1638 11 2 Storage 1638 11 2 1 Disposal 1638 12 Annexes 1639 12 1 Administrative tasks 1639 12 1 1 Firmware installation 1639 12 1 2 Remote operation in a LAN 1640 12 2...

Страница 14: ...Contents R S ZNA 14 User Manual 1178 6462 02 20...

Страница 15: ...roducts from the Rohde Schwarz group of companies are manufactured according to the highest technical standards To use the products safely follow the instructions provided here and in the product docu...

Страница 16: ...ays fold the feet completely in or out to ensure sta bility The feet can collapse if they are not folded out completely or if the product is moved without lifting it The foldable feet are designed to...

Страница 17: ...essible If the product is integrated into a system that does not meet these requirements provide an easily accessible circuit breaker at the system level Cleaning the product Use a dry lint free cloth...

Страница 18: ...he sig nal word indicates the severity of the safety hazard and how likely it will occur if you do not follow the safety precautions WARNING Potentially hazardous situation Could result in death or se...

Страница 19: ...K210 new Port Order setting See Port Order on page 686 New remote control features New command SENSe Ch CORRection COLLect AUTO REPeat to repeat an automatic calibration New remote commands DISPlay LA...

Страница 20: ...f r mixer measurements R S ZNA K4 Spectrum measurements R S ZNA K1 Noise power ratio trace statistic See Power Ratio on page 413 Increased Level Accuracy zero padding measurement option See Increased...

Страница 21: ...ration types P One Path Two Ports and PUOSM if one receiver pair is used for both IMD tones at the source side P Refl OSM if two receiver pairs are used Trace data export The number of decimal places...

Страница 22: ...A K5 Measurement results varied with VUOSM calibration sequence The visibility of the Track LO popup was not setup specific Pulse profile measurements R S ZNA K7 Popup hint Measurement not fast enough...

Страница 23: ...ts R S ZNA K5 Command SENSe Ch CORRection COLLect AUTO TYPE did not work for cal types VUOSm and CPUosm Bad zip compression performance of Create R S Support Information could lead to GUI freezes and...

Страница 24: ...ement examples and general informa tion e g safety instructions etc A printed version is delivered with the instrument A PDF version is available for down load on the Internet 2 2 2 User manual and he...

Страница 25: ...numbers and optional accessories The brochure provides an overview of the instrument and deals with the specific char acteristics See https www rohde schwarz com brochure datasheet ZNA 2 2 7 Release...

Страница 26: ...y text strings The buttons below the title bar provide further navigation support Toolbar But tons Name Purpose Previous Next Browse the topics you visited before Back Forward Go to the previous next...

Страница 27: ...operator For advanced search consider the following To find a defined string of several words enclose it in quotation marks For example a search for sweep settings finds all topics with exactly sweep...

Страница 28: ...to the handles See Lifting and carrying the product on page 15 3 1 2 Unpacking and checking 1 Unpack the R S ZNA carefully 2 Retain the original packing material Use it when transporting or shipping t...

Страница 29: ...rbances It is therefore not suitable for class B environments If class A equipment causes radio disturbances take appropriate measures to eliminate them 3 1 4 Setting up the product See also Setting u...

Страница 30: ...ns in Setting up the product on page 16 2 NOTICE Insufficient airflow can cause overheating and damage the product Design and implement an efficient ventilation concept for the rack To mount the R S Z...

Страница 31: ...ternal devices comply with EMC regulations Regarding length and quality the following requirements have to be met for cable that are directly connected to the R S ZNA Table 3 1 Cable Requirements Cabl...

Страница 32: ...nformation see Connecting to power on page 16 The network analyzer is automatically adapted to the AC supply voltage which must be in the range of 100 V to 240 V at 50 Hz to 60 Hz A line frequency of...

Страница 33: ...y Windows ses sion 2 If desired set the AC power switch to position O Off To disconnect from power The R S ZNA is in standby state 1 NOTICE Risk of data loss If you disconnect the product from power w...

Страница 34: ...o open the Start menu press the Windows key in the tool bar or on an external keyboard User accounts and password protection The analyzer uses a user name and password as credentials for remote access...

Страница 35: ...de Schwarz does NOT recommend running anti virus software in the background on access mode on Windows based instruments due to potentially degrading instrument performance However Rohde Schwarz does r...

Страница 36: ...r manual GUI operation of the VNA application A printer can be used to create hard copies of the measurement diagrams and traces from within the VNA application A LAN connection can be established to...

Страница 37: ...Windows use the button in the toolbar of the application window 3 1 11 3 Connecting a mouse A USB mouse can be connected to any of the USB connectors After being auto detec ted by the operating syste...

Страница 38: ...anagement to configure the printer properties and printing preferences 3 1 11 5 Connecting a LAN cable A LAN cable can be connected to the LAN connector on the rear panel of the analyzer To establish...

Страница 39: ...ve touchscreen and the test port area below it Brief explanations on the controls and connectors can be found on the next pages 3 2 1 1 Touchscreen The touchscreen is split into two parts the applicat...

Страница 40: ...tion to touchscreen operation refer to Chapter 3 3 1 Manual operation on page 48 The following sections contain further useful information about manual control of the instrument Refer to Chapter 3 3 O...

Страница 41: ...sweep type and the num ber of measured sweeps Cal functions that are necessary to perform and manage calibrations Channel Config functions for channel management Mode set up channels for particular n...

Страница 42: ...eys switch between the numeric and the string keyboard The wheel control at the right of the numerical data input panel increases and decreases numerical values and scrolls within lists Step Size open...

Страница 43: ...ed electrical short the RF interlock mode is inac tive and the LED is off see picture below If the connector is open the RF interlock state is active and the LED is on red Figure 3 2 RF Off Control te...

Страница 44: ...usage Two LEDs above each test port indicate the connector usage Tx on connector is used as a source port Rx on connector is used as a receive port both LEDs on connector is used as a bidirectional s...

Страница 45: ...ch Out In loop must be closed using a jumper See also Chapter 3 1 5 Considerations for test setup on page 31 USB connectors The front panel offers three USB connectors of type A master USB The USB por...

Страница 46: ...only External monitor connector DVI D see Chapter 3 1 11 1 Connecting a monitor on page 36 4 LAN RJ 45 connector to integrate the instrument to a Local Area Network primarily for remote control purpo...

Страница 47: ...rotection see Preventing electrostatic discharge ESD on page 32 Table 3 3 Optional rear panel elements Index Label Description 16 Converter LO Hardware option R S ZNA B8 provides a local oscillator ou...

Страница 48: ...r related remote commands are linked bidirectionally Background information is provided in the Concepts and features chapter 3 3 1 Manual operation The analyzer functions are accessible via several ta...

Страница 49: ...o access an instrument function 1 Press a virtual key e g the Meas key in the Trace section The corresponding softtool expands at the current docking position 2 Make sure the S Params radio button is...

Страница 50: ...ram immediately reflects your selection The active trace shows the mea surement results for the selected measured quantity A control element with three dots e g System Setup Setup System Con fig opens...

Страница 51: ...arams Wave a1 Src Port 1 The diagram immediately reflects your selection The active trace shows the mea surement results for the selected measured quantity At the same time the related softtool tab is...

Страница 52: ...e several alternative ways for accessing an instrument function Using a function key on the virtual hardkey panel to open the related softtool rec ommended provides all settings Using the menus and su...

Страница 53: ...Title bar By default the analyzer GUI is shown in full screen mode covering the whole screen and hiding the Windows taskbar However you can toggle the full screen mode using System Display View Bar Ti...

Страница 54: ...ese icons provide the following actions from left to right Print the current diagrams to a bitmap file same as System File Print Print To File By default all diagrams are printed no matter if displaye...

Страница 55: ...ad and modify settings e g Ref Value in the screenshot above some perform actions e g Auto Scale Trace while others open additional dialogs button label ends with 3 3 2 4 Menu bar You can also access...

Страница 56: ...ng and arranging different diagrams The Application menu gives access to applications and tools that extend the func tionality of the analyzer firmware The System menu provides functions that can be u...

Страница 57: ...control window s function keys inside the main application window For a short description of the function keys refer to section Control window function keys on page 40 The Hard Key panel is particula...

Страница 58: ...redefined S parameters if the physical ports have been redefined see Chapter 5 19 7 2 Define Physical Ports dialog on page 835 the current date and time Figure 3 5 R S ZNA with frequency converters Th...

Страница 59: ...yed area of the table or diagram to show results that were previously out of view Figure 3 7 Dragging Spreading and pinching two fingers Move two fingers apart on the display spread or move two finger...

Страница 60: ...touch actions correspond to mouse actions Table 3 4 Correlation of mouse and touch actions Mouse operation Touch operation Click Tap Double click Double tap Click and hold Touch and hold Right click...

Страница 61: ...e convenience functions Use the Dialog Transparency function to make the display elements behind the dialog visible Note The Dialog Transparency is a global setting i e it applies to all dialogs Use t...

Страница 62: ...ments 3 3 5 1 Adding new traces and diagrams A new trace is required if you want to measure and display an additional quantity Typical scenario The transmission coefficient S21 is measured as describe...

Страница 63: ...1178 6462 02 20 3 In the dialog box that is opened when you release the New Trace icon select the S parameter to be measured For a four port analyzer The R S ZNA generates a new trace for the selecte...

Страница 64: ...ons Select System Display to access more diagram handling functions 3 3 5 2 Adding new markers A marker is needed for instance to read a particular numerical trace value To add a new marker Drag and d...

Страница 65: ...n Note however that the last remaining trace cannot be deleted To delete a diagram drag and drop its diagram number label onto the Delete icon Note however that the last remaining diagram cannot be de...

Страница 66: ...te icon see Chapter 3 3 5 3 Deleting dis play elements on page 65 Marker info field Add See Chapter 3 3 5 2 Adding new markers on page 64 Move within diagram Marker info field move to one of several p...

Страница 67: ...8 3 3 6 2 Using the numeric editor The Numeric Editor is a tool for convenient entry and modification of numeric values It is available for all numeric input fields in the analyzer GUI The numeric edi...

Страница 68: ...ens a dialog containing the Step Size panel of the numeric editor Select the adequate step size for efficient operation of the on screen wheel and mouse wheel 3 3 6 3 Using the analyzer s on screen ke...

Страница 69: ...pose the input string 4 Select Enter to apply your selection and close the keyboard 3 3 6 4 Using the Windows on screen keyboard The Windows on screen keyboard allows you to enter characters in partic...

Страница 70: ...oom win dow to fill the entire diagram area The sweep points are not affected The graphical zoom function is only supported for cartesian trace formats For inver ted Smith and polar diagrams it is not...

Страница 71: ...ive settings The Trace Scale Zoom softtool tab allows you to define the displayed zoom range numerically It can also be opened from the menu of the Zoom Config toolbar icon If the active diagram is gr...

Страница 72: ...age 73 3 3 7 3 Reference value and position The analyzer provides three parameters for changing the scale of the vertical response axis Changing the Ref Value or Ref Pos shifts the trace in vertical d...

Страница 73: ...Increasing the Ref Value scales down the polar diagram Decreasing the Ref Value magnifies the polar diagram The Ref Value is indicated in the scale section of the trace info To change the Ref Value s...

Страница 74: ...le Delta Mode for this marker The analyzer automatically creates an additional reference marker 3 Place the reference marker to the start value of the desired sweep range 4 Set the value of the delta...

Страница 75: ...gram the System Display key or the Display menu to access the display settings 3 4 Performing measurements This chapter takes you through a sample session with a R S ZNA network analyzer and describes...

Страница 76: ...that you preset the R S ZNA to start from a well defined instrument state DUT 1 2 VNA 1 2 1 Connect the DUT between test ports 1 and 2 of the network analyzer as shown above 2 Use the Preset key to re...

Страница 77: ...ws you how to configure a smaller sweep range 1 Select Stimulus Start and set the Start Frequency to the lowest frequency you want to measure e g 1 77 GHz 2 In the Stop Frequency input field enter the...

Страница 78: ...to the R S ZNA s calibration wizard calibration is a straightforward guided proc ess 1 Replace the DUT by the Through standard of your calibration kit Make sure to dis connect all calibration units 2...

Страница 79: ...s 1 and 2 is optional Skip it for now The analyzer performs a calibration sweep for the measured quantity S21 The magnitude and phase of the result is displayed in two diagrams together with the expec...

Страница 80: ...ce a marker info field in the upper right corner of the diagram The marker info field displays the stimulus value frequency and response value magnitude of the transmission coefficient converted to a...

Страница 81: ...r to Chapter 3 1 11 Connecting external accessories on page 36 and Chapter 12 1 2 Remote operation in a LAN on page 1640 to obtain information about the necessary steps 1 Activate the System File Prin...

Страница 82: ...to learn from the data Only one analyzer test port is required for reflection measure ments In principle a reflection measurement involves the same steps as a transmission mea surement Note the follow...

Страница 83: ...ted for reflection measurements For instance you can display the measured reflection coefficient S11 in a Smith chart to obtain the complex input impedance at port 1 Proceed as described in Chapter 3...

Страница 84: ...mize the evaluation of results To ensure that the instrument resour ces are easily accessible and that user defined configurations can be conveniently implemented stored and reused the instrument uses...

Страница 85: ...ll sets A recall set only contains setup instructions i e information on how to measure how to process the measurement results and how to display the processed results It does not contain any trace or...

Страница 86: ...settings can be accessed via the keys in the Trace section of the virtual key panel They complement the Channel settings accessible via the Stimulus and Chan nel sections Each trace is assigned to a...

Страница 87: ...active channel is highlighted Channels with no traces are not indicated in the diagrams but can be accessed via the Channel Manager dialog In manual control there is always exactly one active trace ir...

Страница 88: ...at fixed hardware settings In particular a fixed source port and receive port is used Each sweep point requires a single partial measurement See also Chapter 4 3 1 S parameters on page 122 For a comp...

Страница 89: ...ll sweep points Trigger event starts m complete measurements at consecutive sweep points Sweep Segment Trigger event starts n partial sweeps over the next segment Trigger event starts complete mea sur...

Страница 90: ...ed at 102 MHz In the 101st measurement step measurements for port group 1 will be performed at 199 MHz measurements for port group 2 will be performed at 200 MHz Finally in the 102nd measurement step...

Страница 91: ...power are both entered in absolute units dBm Start and stop power must be different the stop power must be larger than the start power If a stop power smaller than the start power is set then the star...

Страница 92: ...become unstable If it is too low the ALC possibly does not respond sufficiently to errors and become too slow The integration time Ti controls the change of the controller output based on the integral...

Страница 93: ...erent source signals ALC optimization To reduce the overall measurement time the ALC pre measurements can also be per formed in a speed optimized way By default this optimization is done if a single s...

Страница 94: ...ION User RAW WAVE QUANTITIES SYST ERR CORRECTION DATA POWER CORRECTION DATA OFFSET Alternative 1 MIXED MODE S PARAMS CUSTOM DLLs COMPLEX CONVERSION AVERAGE S parameters Wave quantities with wave de em...

Страница 95: ...S parameters were transformed to conductance wave quantities and vice versa afterwards The new wave de embedding remains in the wave quantity conductance domain and calculates all de embedding steps...

Страница 96: ...port complex data with trace math 6 Export formatted data SHIFT RESPONSE TIME DOMAIN GATE TRACE FORMAT SMOOTH SHIFT HOLD Formatted TRACE MATH DISPLAYED QUANTITY 6 5 Requires Time Domain option Before...

Страница 97: ...practi cally unlimited number of traces which can be assigned to different channels Most diagram settings are arranged in the Display softtool key System Display To assign traces and channels to diagr...

Страница 98: ...to display or hide it 4 2 1 2 Traces A trace is a set of data points displayed together in the diagram The individual data points are connected so that each trace forms a continuous line The trace ca...

Страница 99: ...ll set A mathematical trace that is based on the active data trace is dynamic It is possible to generate an unlimited number of memory traces from a data trace and display them together Markers and ma...

Страница 100: ...le if a trace is hidden and Math if the trace is a mathematical trace Gat indicates that a time gate is active for the trace Use the Mem Math and Traces tabs of the Traces softtool to display and hide...

Страница 101: ...ed using the Marker menu functions Determine the coordinates of a measurement point on the trace In polar diagrams where no x axis is displayed markers can be used to retrieve the stimulus value of sp...

Страница 102: ...r as the associated trace The marker coordinates are expressed in one of the marker formats selected via Trace Marker Marker Props Marker Format The formats of the markers assigned to a trace are inde...

Страница 103: ...ntities and trace formats see Chapter 4 2 3 3 Measured quantities and trace formats on page 121 Essen tially a marker format is simply a conversion between points on a complex valued trace the raw mea...

Страница 104: ...alized series conductance susceptance and either inductance or capacitance in three lines Inverted Smith diagram Gs Bs Ls or Cs G j B parallel Parallel admittance Unnormalized parallel conductance sus...

Страница 105: ...related traces If the new stimulus value is outside a trace s sweep range the marker value is invalid for this trace The corresponding info field only displays the stimulus value Only one kind of mark...

Страница 106: ...actor the trace format must be dB Mag The info field contains the following search results Bandwidth is the n dB bandwidth of the bandpass bandstop region where n is a selectable bandwidth factor The...

Страница 107: ...automatically assigned number n If a time domain transform is active the R S ZNA displays an additional line to indicate the stimulus range of the displayed time domain trace Open the Channel Manager...

Страница 108: ...he Channel Sweep Sweep Params softtool tab and the Channel Pwr Bw Avg softtool 4 2 1 5 Context menus To provide access to the most common tasks and speed up the operation the analyzer offers context m...

Страница 109: ...ling as in Balanced Ports call up a dialog The dialogs of the analyzer have an analogous structure and several common control elements Dialogs are controlled in the usual way For an introduction refer...

Страница 110: ...tings provide both an OK button and a Cancel button Example Balanced Ports dialog Immediate settings can be undone using the Undo toolbar icon 4 2 2 2 Common dialogs Open Dialog The Open File dialog i...

Страница 111: ...layed with different caption default direc tory Traces in the above screenshot and file types Context specific options e g Output Format in the dialog above are accessible via controls in the section...

Страница 112: ...m top to bottom generator and receiver ports signal path direct access combiners etc sweep parameters measurement logic measurement specific channel settings The channel selector in the title bar and...

Страница 113: ...ormats dB Mag Phase SWR Unwr Phase Lin Mag Log Mag Real Imag and Delay Complex trace formats Polar Smith and Inv Smith The VNA firmware allows arbitrary combinations of trace formats and measured quan...

Страница 114: ...ithmically The resulting linear or lin log grid is plotted with the formatted trace The following examples show dB Mag Cartesian traces for the same measured quan tity and sweep range but with Lin Fre...

Страница 115: ...l direction Result values for consecutive stimulus values are interconnected by straight lines so the trace is represented as a polygonal chain in the complex plane The stimulus axis is not visible Ho...

Страница 116: ...ut The outer circumference Sii 1 represents a totally reflected signal Examples for definite magnitudes and phase angles The magnitude of the reflection coefficient of an open circuit Z infinity I 0 i...

Страница 117: ...e resist ance is mapped into a unit circle The basic properties of the Smith chart follow from this construction The central horizontal axis corresponds to zero reactance real impedance The center of...

Страница 118: ...ove the graphical representation in a Smith chart has the following properties Real reflection coefficients are mapped to real impedances resistances The center of the plane 0 is mapped to the referen...

Страница 119: ...e grid lines overlaid to a Smith trace correspond to points of equal conductance G and susceptance B Points with the same conductance are located on circles Points with the same susceptance produce ar...

Страница 120: ...ex reflection coefficient e g S11 S22 then the inverted Smith chart can be used to read the normalized admittance of the DUT The coordinates in the normalized admittance plane and in the reflection co...

Страница 121: ...meaning standing wave ratio normalized impedance or admittance if they are used for transmission S parame ters ratios and other quantities For complex Impedances Admittances Z parameters and Y parame...

Страница 122: ...e Meas softtool function key Trace Meas 4 3 1 S parameters S parameters are the basic measured quantities of a network analyzer They describe how the DUT modifies a signal that is transmitted or refle...

Страница 123: ...asurement with matched DUT port 1 S22 b2 a2 if a2 0 and a1 0 Meaning of squared amplitudes The squared amplitudes of the incident and outgoing waves and of the matrix elements have a simple meaning Ta...

Страница 124: ...ence the measured S parameters and other measured quantities are also redefined The receivers and generators can be freely assigned but without reusing the same original physical port in different red...

Страница 125: ...ation of the physical port impedances affects e g S parameters and wave quantities in Power representation Renormalization of the balanced port impedances affects all measured quantities that the anal...

Страница 126: ...t theories 1 Traveling waves In the model of Marks and Williams A General Waveguide Circuit Theory the wave quantities a and b are transformed as follows i i i i i i i i i i i i i i i i i b a Z Z Z Z...

Страница 127: ...onverts a single measured S parameter Sij to determine the corresponding converted impedance under the assumption that each of the other ports is terminated with its respective reference impedance Z0k...

Страница 128: ...lated Table 4 4 Calculation of Converted Reflection Impedances Traveling Waves Power Waves Series transmission impedance A two port transmission parameter Zij i j can describe a pure serial impedance...

Страница 129: ...en output ports impe dance 0 The analyzer provides the full set of Z parameters including the transfer impedances i e the complete nxn Z matrix for an n port DUT This means that Z parameters can be us...

Страница 130: ...tance parameters Converted admittances each admittance parameter is obtained from a single S parameter Y parameters complete description of the n port DUT 4 3 4 1 Converted admittances The converted a...

Страница 131: ...opagation 4 3 5 Wave quantities and ratios The elements of the S Z and Y matrices represent fixed ratios of complex wave amplitudes As long as the assumption of linearity holds the S Z and Y parameter...

Страница 132: ...DUT port i In a standard forward S parameter measurement a1 Src Port 1 is the incident wave and b1 Src Port 1 is the reflected wave at DUT port 1 4 3 5 2 Ratios A ratio measurement provides the compl...

Страница 133: ...port 1 ist stimulated This corresponds to the forward reflection coefficient S11 4 3 5 3 Detector settings The Detector settings select the algorithm that is used to calculate the displayed measuremen...

Страница 134: ...s The detector setting in the More Ratios menu applies to both the numerator and the denominator wave quantity To allow for different detector settings measure the numer ator and denominator wave quan...

Страница 135: ...olerances limited frequency range Calibration can be performed at the DUT s ports If necessary e g to compensate for the effect of a test fixture it is possible to shift the calibration plane using le...

Страница 136: ...t Bal port Differential mode Zref Z0d Common mode Zref Z0c Balanced port Log VNA port A balanced port configuration is defined in two steps First select the pairs of physical ports that you want to co...

Страница 137: ...nt defined as the ratio of the wave quantities b1 mode mout reverse measurement with matched input b1 in the figure above and a1 0 to a2 mode min S mout min 22 is the mixed mode output reflection coef...

Страница 138: ...it is a measure for the deviation of the balun from ideality The general definition of the transmission imbalance between two differ ent ports at least one of them balanced is given below For a DUT wi...

Страница 139: ...i j CMRRji Sddji Sccji Differential Imbalance A differential imbalance can also be calculated if the second balanced DUT port is connected to two single ended logical ports DUT Balanced port Balanced...

Страница 140: ...Sinsky A New Criterion for Linear 2 Port Stability Using a Single Geometrically Derived Parameter IEEE Trans MTT vol 40 No 12 pp 2303 2311 Dec 1992 4 3 8 Delay aperture electrical length The group de...

Страница 141: ...or the related stimulus range A ripple limit defines the maximum difference between the largest and the smallest response value for the related stimulus range A ripple limit test is suitable e g to ch...

Страница 142: ...nes can be partially or entirely outside the sweep range however the limits are only checked at the measurement points The following figure shows a limit line consisting of 3 upper and 2 lower limit l...

Страница 143: ...Limit Line is active the diagrams display all limit line segments Exception In a segmented frequency sweep with point based x axis gaps between the segments are minimized To facilitate the interpretat...

Страница 144: ...ot have to be smaller than the Start Stimu lus value of range no n 1 Overlapping ranges are allowed The limit check in the overlapping area is related to the tighter limit the pass test involves a log...

Страница 145: ...ion on page 141 4 4 1 3 Circle limits A circle limit is a special type of upper limit line which is defined by its center coordi nate in the diagram and its radius Depending on the diagram type circle...

Страница 146: ...146 User Manual 1178 6462 02 20 With a circle limit line adjusted to the right border of a Smith diagram Z infinity you can check whether the imaginary part of the impedance Im Z reactance falls below...

Страница 147: ...er Manual 1178 6462 02 20 With a circle limit line centered on the left border of an inverted Smith diagram Y infinity you can check whether the imaginary part of the admittance Im Y sus ceptance fall...

Страница 148: ...has the extension limit and is stored in the directory shown in the Save Limit Line and Recall Limit Line dialogs The file starts with a preamble containing the channel and trace name and the header o...

Страница 149: ...s with a preamble con taining the channel and trace name and the header of the range list The following lines contain the entries of all editable columns of the list Example of a ripple limit file The...

Страница 150: ...Format Specification Version 2 0 The R S ZNA supports Touchstone file versions 1 1 and 2 0 By convention Touchstone files use file extensions snp where n is the number of network ports The following...

Страница 151: ...the net work data given as a real positive resistance Its value is given in default 50 During export if the impedances of all involved ports are identical the analyzer sets the reference resistance to...

Страница 152: ...rt The following Port Impedance Renormalization has been used when saving the data PortZ Port1 100 j0 Port2 50 j0 Note The Port Impedances differ from the reference impedance of this file While readin...

Страница 153: ...1 1 and Version 2 0 For export format Version 1 1 ZNx the assignments are given in the Network data table header comment lines For export formats Version 1 1 and Version 2 0 they are declared in a ded...

Страница 154: ...f Frequencies Number of Frequencies 201 Number of exported frequency sweep points can also be derived from the Network parameter data block Reference Reference 50 75 If the exported S parameter data w...

Страница 155: ...cending order of frequency For segmented sweeps the R S ZNA makes an exception to the strict ascending order principle During export the segments are always kept together which can result in downward...

Страница 156: ...a single trace Typically Touchstone files contain a complete set of S parameter traces of an n port network The only exceptions are s1p files created from arbitrary S parameter traces using the s1p Ac...

Страница 157: ...file into standard applications including the R S ZNA itself the reference resistance of the Touchstone option line is used and the impedance system underlying the data is not interpreted correctly 4...

Страница 158: ...ascending order 4 4 2 3 Finding the best file format The file format depends on how you want to use the exported data Use a Touchstone file format to export S parameter data traces to a file that can...

Страница 159: ...system error correction data is used to correct the measurement results of a DUT that is measured instead of the standards Calibration is always channel specific because it depends on the hardware se...

Страница 160: ...d port de activation The analyzer fimware automatically activates deactivates ports during after a success ful calibration Calibrated ports that were previously disabled are automatically enabled as s...

Страница 161: ...d transmission mea surements classi cal 12 term error correction model Adapter Removal 2 port Open Short Match1 at each port Through All Reflection parame ters with and with out adapter Reflection tra...

Страница 162: ...eceiver cal ibration Scalar mixer mea surements if no phases delays are required OSM Vector Mixer Open Short Match1 at each port Calibration Mixer All except feed throughs Reflection tracking source m...

Страница 163: ...compensate for directivity or mismatch errors This limits the accuracy of a normalization Manual reflection normalizations offer Complementary Match standard measure ments Manual transmission normali...

Страница 164: ...connected instead of 7 for a full two port TOSM calibration It is suitable when only the forward e g S11 and S21 or reverse S parameters e g S22 and S12 are nee ded and if the DUT is well matched esp...

Страница 165: ...mplementary isolation measurement optional UOSM TOSM with unknown Through The analyzer can perform a TOSM calibration with any 2 port network serving as through connection as long as it fulfills the r...

Страница 166: ...ferent connector types must be made using adapters The problem here is that unlike Through standards adapters are usually not character ized i e their delay time transmission phase is unknown If the a...

Страница 167: ...reflection low loss Through standard with an electrical length that can be different from zero an Open and a Match The characteristics of all standards must be fully known the Match can have non idea...

Страница 168: ...at have to be solved for the error terms exhibits singularities whenever the length difference L between the Through and the Line is an integer multiple of half of the wave length 2 n L As a rule sing...

Страница 169: ...he calibrated range into segments The calibration data of the longest line is applied to the lowest segment the calibration data of the shortest line to the highest segment The calibration sweep segme...

Страница 170: ...st be below the minimum difference to the singularity points 0 deg or 180 deg multiplied by two Suppose that an approximately known Line standard causes a transmission phase 30 deg at the start freque...

Страница 171: ...t of Through connections have been measured However you can measure additional Through connections to improve the accuracy If Multiple port assignments are required an Automatic calibration always pro...

Страница 172: ...ion standards for a particular connector type The magnitude and phase response of the calibration standards i e their S parame ters must be known or predictable within a given frequency range The stan...

Страница 173: ...wn mismatched reflection symmetric stan dard two port Offset parameters The offset parameters have the following physical meaning The delay is the propagation time of a wave traveling through the stan...

Страница 174: ...nstead of a definite impedance value Moreover no loss or offset loss can be set Offset parameters and standard types Offset parameters are used to describe all types of standards except the Sliding Ma...

Страница 175: ...uffix Ideal Kit describe an idealized calibration kit for each connector type see below Make sure to use universal or individual parameter sets if you need to obtain high pre cision results The precis...

Страница 176: ...Smith chart The center of this circle corresponds to per fect match The network analyzer determines and further corrects this match point fol lowing I K sa s circle fitting algorithm To obtain the re...

Страница 177: ...ary to determine its magnitude and phase response By default cal kit files are stored in the C Users Public Documents Rohde Schwarz ZNA Calibration directory To export cal kit data the analyzer uses a...

Страница 178: ...system Cal kit files generated on an English operating system contain dots the ones generated on a German system contain commas The network analyzer expects the dot as a separator and displays an erro...

Страница 179: ...al Off The system error correction is no longer applied e g User Cal Active is disabled See also Cal Off label on page 160 4 5 5 Automatic calibration A calibration unit is an integrated solution for...

Страница 180: ...which is used to power supply and control the unit A USB cable for connection to the network analyzer is provided with the cali bration unit RF connectors which are connected to the test ports For all...

Страница 181: ...he network analyzer is operating Never connect or disconnect the unit while data is being transferred between the analyzer and the unit Never connect the unit during a firmware update 4 5 5 2 Performi...

Страница 182: ...m RF input power of the calibration unit quoted in the data sheet is never exceeded The available calibration types depend on the number of ports to be calibrated For a single calibrated port the refl...

Страница 183: ...libration unit 2 Connect the calibration unit to the network analyzer 3 Access the Characterize Cal Unit dialog Channel Cal Cal Devices Characterize Cal Unit and select Start Characterization 4 Step t...

Страница 184: ...it ICU is inserted between the corresponding VNA test port and the DUT The ICU remains within the signal path throughout the measurement low loss through path and on request of the VNA performs a full...

Страница 185: ...ion see the R S ZN Z3x product pages at https www rohde schwarz com product NetworkAnalyzer_Acc_ZNZ3 Firmware integration The control connection between R S ZNA and ICC is established via USB After th...

Страница 186: ...Embedding the Through into the ICU s OSM standards is necessary because the ICU remains in situ For this reason during ICU characterizations the Through measurement is mandatory Workflow 1 Configure t...

Страница 187: ...rements c Skip Through measurements 4 5 5 5 Calibration validation This feature allows you to validate the active channel calibration against the expected result from a stored characterization It requ...

Страница 188: ...is connected to a 4 port cali brated channel only the two connected instrument ports are validated against the cal unit The validation logic proceeds through the connected ports p 1 4 in ascending ord...

Страница 189: ...anced mode you can configure the validation settings reference characteriza tion deviation limits and run or rerun particular measurements reflection at port p with connected Open Short or Match 4 5 5...

Страница 190: ...d and complete solution can be described as fol lows Calibration type Minimal solution Default solution minimal Full One Port Each calibrated test port must appear in exactly one port assignment Subdi...

Страница 191: ...e 4 12 Full n port Line shaped optimum solution Test Port Assignment 1 Assignment 2 Assignment 3 1 Cal Unit Port 1 2 Cal Unit Port 2 3 Cal Unit Port 3 4 Cal Unit Port 4 Cal Unit Port 4 5 Cal Unit Port...

Страница 192: ...combination with SMARTerCal Yes Power sweep e g for com pression point measurement Yes Power source Yes Meas receiver not necessary Not necessary Frequency conversion mea surements on linear DUTs Not...

Страница 193: ...alibration procedure The source power calibration requires an external power meter to be connected via GPIB bus USB or LAN interface The power sensor can be connected directly at the calibration plane...

Страница 194: ...lyzer b waves agree with the source power level calibrated at an arbitrary calibration plane Typically the calibration plane is at the input of the receiver so that the calibration eliminates frequenc...

Страница 195: ...ected by a SMARTerCal Therefore a scalar power calibration is not applied to S parameters and derived quan tities no power calibration labels appear in the trace list Table 4 14 Power calibration labe...

Страница 196: ...st frequency A label PCax in the trace list indicates an extrapolated power calibration 4 5 6 4 Extended test setups The power calibration data can be modified to account for an additional two port de...

Страница 197: ...alibration methods serve different purposes The system error correction requires a set of calibration standards it provides vec tor error corrected S parameters For equal port frequencies the n port c...

Страница 198: ...quipment The R S ZNA also supports a SMARTerCal based on the calibration units The cali bration units provide the n port system error correction data TOSM or UOSM a sub sequent power calibration sweep...

Страница 199: ...ion step of the scalar source calibration ensures accurate input powers at the DUT A combined SMARTerCal and scalar power calibration is also appropriate for fre quency conversion measurements on non...

Страница 200: ...ibrate All supports all Chapter 4 5 1 Calibration types on page 160 calibra tion types excluding scalar power calibration Use a suitable SMARTerCal instead Calibrate multiple channels in one go using...

Страница 201: ...this section complements the calibration com pensating for the effect of known transmission lines or matching networks between the calibrated reference plane and the DUT 4 6 1 Offset parameters Offse...

Страница 202: ...loss at frequency f is approximated by If in addition the loss at a second frequency f2 is known or measured then the loss can be approximated by In practice the frequency dependent part ist often dom...

Страница 203: ...because it uses all sweep points For non dispersive cables aperture and evalua tion range effects are expected to vanish Use Trace Marker Set by Marker Zero Delay at Marker to set the delay at a spec...

Страница 204: ...n S parameter ratio wave quantity a converted impedance or a converted admittance The effect of Auto Length and Loss on S parameters wave quantities and ratios is to eliminate a linear phase response...

Страница 205: ...sate for the effect of the test fixture con nections The following features can further improve the accuracy of the fixture compensation Direct Compensation provides a frequency dependent transmission...

Страница 206: ...ally removing a perfectly matched transmission line at that port A negative offset moves the reference plane away from the DUT which is equiva lent to embedding the DUT by numerically adding a perfect...

Страница 207: ...can be applied to balanced ports two port networks can be applied to single ended ports A combination of four port and two port networks can be applied to any pair of sin gle ended ports Moreover it...

Страница 208: ...ally removed the real network must be described by a set of S parame ters or by an equivalent circuit of lumped elements Deembedding the DUT effectively extends the calibration plane towards the DUT p...

Страница 209: ...rial C Shunt C Serial L Shunt L The following networks are composed of a shunt C or L as seen from the analyzer port followed by a serial C or L They are named Shunt C Serial L Shunt L Serial C Shunt...

Страница 210: ...In the default setting the resistors are not effective since the serial Rs are set to 0 the shunt Rs are set to 10 M Moreover the serial elements can be replaced by imported 2 port S parameters or the...

Страница 211: ...allel with conduc tance G i circuit blocks 4 6 2 5 Port pair de embedding Port pair de embedding extends the functionality of balanced port de embedding to pairs of single ended physical ports The ana...

Страница 212: ...2 and 1 3 with appropriate sets of 4 port S parameters The R S ZNA FW handles Port Pair De Embedding as a special case of Port set de embedding 4 6 2 6 Port set de embedding The port set de embedding...

Страница 213: ...set deembed ding is calculated after single ended deembedding and the port set embedding step precedes single ended embedding It is possible to combine a sequence of port sets for deembedding embeddin...

Страница 214: ...ion of the DUT causing a difference in potential between the analyzer s and the DUT s ground reference A typ ical and often unavoidable source of ground loops is the parasitic inductance of the ground...

Страница 215: ...accurate deembedding algorithms to calculate and remove these effects from the mea surements leaving only the result for the area of interest Instead of asking the user to define the fixture by param...

Страница 216: ...third party tool to calculate the fixture deembedding files After a final confirmation the calculated deembedding files s2p for single ended ports s4p for balanced ports are used for deembedding the D...

Страница 217: ...xture modeling tool support assumes symmetrical lead ins and lead outs 4 6 2 10 Combining Several de embedding networks The R S ZNA allows you to select a combination of networks to be numerically add...

Страница 218: ...4 port network 4 Ground Loop Deembedding the DUT s ground connection can be deembedded from a single 1 port network 5 Ground Loop Embedding the DUT s ground connection can be embedded in a sin gle 1...

Страница 219: ...Single ended port embedding 4 7 Optional extensions and accessories The instrument can be upgraded with various software and hardware options provid ing enhanced flexibility and an extended measuremen...

Страница 220: ...le at all For more information on spectrum mode measurements and channel setups see the description of the Chapter 5 2 11 Spectrum tab on page 351 and the Chap ter 5 2 11 2 Spectrum Setup dialog on pa...

Страница 221: ...f is the spacing between two consecutive sweep points in the frequency domain For a sweep span of 4 GHz and 201 equidistant sweep points f 4 GHz 200 2 107 Hz so that t 50 ns t is termed measurement ra...

Страница 222: ...step response corresponds to the response of a DUT that is stimulated with a voltage waveform that transitions from zero to unity The two alternative responses are mathematically equivalent the step...

Страница 223: ...acing f and the additional condition that f1 f In other words all frequencies fi are set to harmonics of the start frequency f1 If a harmonic grid including the DC value f 0 is mirrored to the negativ...

Страница 224: ...c grids cannot be calculated for any set of sweep points If the minimum number of sweep points is smaller than 5 then the interpolation extrapolation algorithm for additional sweep points does not wor...

Страница 225: ...amplitude accu racy are low Steep Edges Hamming 43 dB 0 019 dB Good compromise between edge steepness and side lobe suppression Normal Gate Hann 32 dB 0 032 dB Good compromise between edge steepness...

Страница 226: ...option K2 supports TD site VSWR measurements For the frequency range 1 GHz to 18 GHz this method of SVSWR measurement is pro posed in standard ANSI C63 25 Hence a R S ZNA equipped with time domain opt...

Страница 227: ...response is related to the shortest distance the earliest impulse is the direct antenna response Reflections from the test site are from farther distance away thus come at later time It is therefore p...

Страница 228: ...the peaks to be considered as being caused by a fault The following example shows the reflection at the end of a faultless open cable con nected to test port 2 A full one port calibration ensures that...

Страница 229: ...sic Time Domain representation capabilities of option K2 by signal integrity testing functionality in the time domain Simulated Eye Diagram With the impulse response calculated from the measured S par...

Страница 230: ...p 2 a the eye diagram is simulated b the Eye Diagram Results are calculated c the Eye Mask Test is evaluated if enabled 4 In continuous sweep mode go back to step 2 The simulation progress is shown in...

Страница 231: ...enote the standard deviations of the observed high and low voltage levels respectively Eye Width This result indicates the effects of jitter in reducing the horizontal eye opening It is defined as Bit...

Страница 232: ...oise level Accordingly it is equal to Eye Amplitude Top Base Eye diagram results are only available for NRZ modulated signals Eye Mask Test Furthermore the eye diagram simulation allows to perform tes...

Страница 233: ...be used to evaluate the quality of a balanced transmission line intra pair skew Limit Test Testing against a user defined skew limit is also supported DUT centric setup As far as possible the require...

Страница 234: ...mixer VNA 1 2 RF LO IF fIF up fRF fLO fIF down fRF fLO In this example the transmission parameter S21 corresponds to the mixer s conversion gain Before measuring the port frequencies must be set appro...

Страница 235: ...icant improvement of the transmission S parameter measurements if the load ports are poorly matched Select the appropriate correction type according to your accuracy and speed require ments Notice tha...

Страница 236: ...one signal is generated at port 1 the upper tone is provided by the external generator Both signals are combined externally and fed to the DUT input The intermo dulation quantities can be measured at...

Страница 237: ...n measurement can be extended to frequency converting DUTs E g it is possible to feed the two tone source signal to the RF input of a mixer and measure the intermodulation distortion of the IF output...

Страница 238: ...enerator for 2 port R S ZNA For a R S ZNA that is not equipped with Internal combiner or with Direct generator receiver access R S ZNAxx B16 lower and upper tone must be combined from the RF ports on...

Страница 239: ...E REF O UT O UT IN IN MEAS SOURCE REF Figure 4 23 Direct access to lower tone port external combiner The lower tone is generated at a VNA port the upper tone is also provided by the ana lyzer 4 port m...

Страница 240: ...of results In the swept measurement the analyzer performs a frequency or power sweep of the two tone stimulus signal and displays the selected intermodulation quantities as a function of the lower ton...

Страница 241: ...of kth order IMk where k 3 5 7 9 at the lower tone frequency minus k 1 2 times the tone distance and at the upper tone frequency plus k 1 2 times the tone distance Intermodulation prod ucts can be me...

Страница 242: ...g nal is applied at the input is known as harmonic distortion Harmonic distortion measurements can be performed irrespective of the sweep type A frequency sweep yields the harmonics as a function of t...

Страница 243: ...ation including the group delay is meaningless The phase or group delay of mixers can be analyzed in a vector mixer measurement In the scalar mixer mode the analyzer provides the following functionali...

Страница 244: ...s defined in the Channel Stimulus menu The RF signal parameters can be changed in the Power and Frequencies dialogs The Local Oscillator LO signal is an additional RF signal that is either generated b...

Страница 245: ...rt number e g Port 2 frequency range sweep range LO or sweep range LO expected fixed power The following mixer signal diagram corresponds to a power sweep RF signal left side Analyzer port number e g...

Страница 246: ...er measurements Option R S ZNA K5 Option R S ZNA K5 enables measurement of the parameters of an external mixer including phase e g the complex conversion loss or reflection coefficients In contrast to...

Страница 247: ...rture f in and out are the phase differences of the two tones at the input and output of the DUT respectively The phase difference of the source signal in and the aperture f are known quanti ties out...

Страница 248: ...defined phases are established during a pre measurement per sweep point and source coherent port which significantly slows down the sweep 4 7 4 1 Amplitude Imbalance and Phase Imbalance Sweep Since fi...

Страница 249: ...rol option R S ZNA K6 is a prerequisite for option R S ZNA K61 The Prerequisites for accurate phase control also apply to true differential mode 4 7 5 1 Virtual vs true differential mode The analyzer...

Страница 250: ...of the differential output signal caused by unequal lengths of the balanced input line conductors This length asymmetry corresponds to a phase imbalance increasing over frequency To see the effect of...

Страница 251: ...of RF and microwave technology Pulsed signals are used in mobile phone applications and radar systems and amplifiers are typically designed for pulsed rather than continuous wave CW con ditions The a...

Страница 252: ...R S ZNA K7 Pulsed measurements R S VSE basic or enterprise edition licensed separately running on the R S ZNA or on a PC that is reachable via LAN V1 90 or higher with option R S VSE K6 Pulse measurem...

Страница 253: ...verters use frequency multi pliers to transform the RF source signal from one of the network analyzer ports into a high frequency stimulus signal A dual directional coupler separates the reference and...

Страница 254: ...is tool is no longer required and can no lon ger be used with the R S ZNA Best leveling results are obtained for frequency converters R S ZCxxx R S ZVA Z110 Var 03 and R S ZVA Z90 Other frequency conv...

Страница 255: ...al wizard For each frequency converter port the leveling procedure consists of the following steps 1 Source flatness calibration optional To improve the accuracy of PRF In it is recommended to perform...

Страница 256: ...ion This is the actual leveling step i e for each point on the leveling grid the RF out put power PRF Out PRF In i fj is determined However during this step it is sufficient to measure the reference r...

Страница 257: ...eveling dataset is applied to all channels in the current recall set A Preset does not affect the leveling dataset and whether or not it is used for power control 4 7 7 2 System error correction S par...

Страница 258: ...4 7 7 3 Measurement After power calibration and system error correction the mm wave measurement can be performed like any other network analyzer measurement The port configuration settings together w...

Страница 259: ...e option increases the maximum measurement bandwidth IF bandwidth of a R S ZNA from 1 5 MHz to 30 MHz 4 7 9 Frequency resolution 1 mHz Option R S ZNA K19 This software option improves the frequency re...

Страница 260: ...range Currently this option is only available for R S ZNA26 and R S ZNA43 Noise figure or noise factor if the linear representation is chosen is an important fig ure of merit for system designers and...

Страница 261: ...s then called the noise fig ure NFD 10 log FD Noise figure and noise factor are used interchangeably through out this manual 4 7 11 1 Algorithmic implementation Cold surce method Historically the nois...

Страница 262: ...he input of the DUT The input noise temperature TSource is derived from the internal temperature of the R S ZNA typically around 38 C and the ambient temperature Tamb of the measurement environment Th...

Страница 263: ...n constant 1 38064852e 23 J K and B is the equivalent noise bandwidth of the used IF filter The equivalent noise bandwidth represents the cumulated normalized filter response In other words it is the...

Страница 264: ...tion additional measurements are required to determine the gain GD IM and the source noise temperature Tsource IM Sideband correction Typically VNA receivers do not have any preselection filters withi...

Страница 265: ...uncertainty analysis of the METAS VNA Tools is limited to S parameter mea surements Uncertainty analysis of wave quantities is not supported The uncertainty calculations are in line with EURAMET Calib...

Страница 266: ...the magnitude and phase stability of the cables must be considered The VNA firm ware uses the cable models available in the local METAS VNA Tools installation You can add your cable models using its...

Страница 267: ...hey have different impedances They are charac terized in steps of 250 MHz and specified together with their measurement uncertain ties Characterization and uncertainties are accredited by the DAkks Ge...

Страница 268: ...mbedding EZD is an implementation of the IEEE algorithm for generating deembedding files from a symmetrical 2x thru measurement Option R S ZNA K210 allows you to model a test fixture using the EZD fix...

Страница 269: ...s dialog Figure 4 34 Fixture modeling EZD Balanced Ports dialog For general information about fixture de embedding see Chapter 4 6 2 9 Fixture modeling and deembedding on page 215 Option R S ZNA K210...

Страница 270: ...phase angle change in insertion loss or 8 points per wavelength 2 The 2x Thru s return loss must be smaller than its insertion loss 3 The 2x Thru s insertion loss must be relatively linear with a few...

Страница 271: ...tps www intel com content dam www public us en documents guides delta l plus methodology for electrical characterization guide pdf https ieeexplore ieee org document 7048423 The R S ZNA uses the Clear...

Страница 272: ...on the R S ZNA includes an SNMP agent and a REST service with HTTP endpoints So you can access the health and usage information via LAN using the SNMP protocol or the REST protocol Accessing the data...

Страница 273: ...urements can be performed without the need for additional external generators 4 7 20 Precision frequency reference Option R S ZNA B4 An optional OCXO improves the static frequency accuracy of the R S...

Страница 274: ...ct generator receiver access the upper tone port can serve as receiving port in intermodulation and two tone group delay measurements And with two independent internal RF sources the R S ZNA can gener...

Страница 275: ...nt of three wave quantities To retrofit the memory extension board the R S ZNA must be sent to Rohde Schwarz Service 4 7 24 LO Out R S ZNA B8 Hardware option R S ZNA B8 provides a dedicated Rear panel...

Страница 276: ...iants of the RFFE GPIO extension board are available R S ZNA B15 variant 02 order number 1332 4575 02 R S ZNA B15 variant 03 order number 1332 4575 03 including current and volt age measurements for a...

Страница 277: ...ly from Rohde Schwarz R S ZN Z25 order no 5202 9238 02 For a detailed pin description see the R S ZN Z25 data sheet 4 7 26 Direct generator receiver access R S ZNAxx B16 Hardware option R S ZNAxx B16...

Страница 278: ...removable system drive for the R S ZNA including operating system and VNA firmware 4 7 28 Source step attenuators Option R S ZNAxx B21 B22 B23 B24 Hardware option R S ZNAxx B2y provides a mechanical s...

Страница 279: ...or higher 4 7 32 Trigger board Option R S ZNA B91 Hardware option R S ZNA B91 adds the following BNC connectors to the Chap ter 3 2 2 Rear panel on page 45 of the R S ZNA 3 trigger in 4 trigger out 4...

Страница 280: ...ct source monitor access is only available for instruments with Direct generator receiver access The unattenuated source signal is available via monitor ports below the respective direct access connec...

Страница 281: ...161 U163 are available to retrofit direct source monitor access with the same logic and prerequisites as for options R S ZNAxx B161 163 The R S ZNA must be sent to Rohde Schwarz service though 4 7 34...

Страница 282: ...l to the receiver step attenuator The R S ZNA must be equipped with a receiver step attenuator at port 2 option R S ZNAxx B32 Currently internal LNAs are not available for R S ZNA50 and R S ZNA67 The...

Страница 283: ...igure Ref receiver Meas receiver Port 2 Source R S ZNAxx B32 R S ZNAxx B22 Source step att 0 70 dB 10 dB Receiver step att 0 35 dB 5 dB R S ZNAxx B302 Low Noise Amplifier 20 30 dB 5 dB 10 dB Figure 4...

Страница 284: ...To suppress the overall LO leakage at the port further it is recommended to use the Direct source monitor access at port 1 option R S ZNAxx B161 This configuration suppresses the LO leakage of the re...

Страница 285: ...nalyzer Connect the GPIB port of the adapter to the external device This hardware option is required if you want to use GPIB for two purposes at the same time to control the R S ZNA from an external c...

Страница 286: ...al example is a source power calibration for an arbitrary analyzer port VNA 1 2 From VNA source port USB ports Power meter sensor Control connection e g USB Power calibration plane External power mete...

Страница 287: ...stening the power meter to an RF connector at high temperature After several hours of operation When low power signals are to be measured e g less than 10 dB above the lower measurement limit A reset...

Страница 288: ...e PM5 control unit is equipped with a USB interface for the PM4 an additional USB to RS232 adapter is required The PM5 can be auto configured the PM4 has to be configured manually The required USB to...

Страница 289: ...g To increase calibration accuracy in particular for low measurement ranges zeroing the PMx power meter is recommended Zeroing is also recommended before a refer ence receiver calibration After mounti...

Страница 290: ...erators increase the number of RF input signals for the DUT A typical example is a mixer measurement with a 2 port analyzer where an external generator provides the LO input signal VNA 1 2 RF LO IF US...

Страница 291: ...and frequency stability in a test setup where different devices represent the signal sources and receivers it is advisable to use a common reference frequency Accurate frequencies are particularly im...

Страница 292: ...to switch the generator to the next point in the list Afterwards the analyzer waits for the next EXT GEN BLANK signal If the User Port connection is interrupted during the measurement the sweep is ha...

Страница 293: ...ce properties the ana lyzer firmware has to consider when interacting with the device Currently only its Settling Time key value pair is evaluated It describes the time number of seconds the firmware...

Страница 294: ...file If certain command sequences require a significantly longer shorter settling time it is recommended to declare them in a separate config file 4 7 41 External DLLs The R S ZNA firmware provides a...

Страница 295: ...n switched ON 2 At the beginning of each sweep point the plugin sets the power supply gate volt age to the next value 3 At the end of each sweep point the plugin reports the power supply reading of th...

Страница 296: ...the same way as for other calculation steps All internal measurement types can be used as input for the new calculation step The new calculation step is only enabled if a plugin is installed and activ...

Страница 297: ...based on the standard test setup for forward S parameter measurements on a 2 port DUT An additional measurement to determine the supplied power PDC is required DC power measurement For the R S ZNA an...

Страница 298: ...tion point to the related remote control commands For a general overview of the analyzer s capabilities and their use refer to Chapter 4 Concepts and features on page 84 5 1 Function keys and softtool...

Страница 299: ...input control corresponding to the start stop center or span of the active channel s current sweep type Activates the corresponding tab on the Stimulus softtool Stimulus Stop Alt Shift K Stimulus Cen...

Страница 300: ...result and display it as a trace you can simply drag and drop the corre sponding button into a diagram area See also Chapter 3 3 5 Handling diagrams traces and markers on page 62 Port activation on d...

Страница 301: ...2 S Params Non Frequency Converting DUTs The Meas softtool for non frequency converting DUTs provides the following tabs Table 5 2 Meas softtool for S Params Measurements Meas softtool tab Channel Mod...

Страница 302: ...n is not available the Mixer Params measurement type and the corresponding tabs are not available Table 5 3 Meas softtool for Mixer Params Measurements Meas softtool tab Channel Mode Required Software...

Страница 303: ...ork analyzer They describe how the DUT modifies a signal that is transmitted or reflected in forward or reverse direction S parameters and similarly Y and Z parameters fully characterize a linear DUT...

Страница 304: ...anced ports standard S parameters are defined in the form S m_out m_in out in where output mode m_out and input mode m_in can be one of d differential balanced c common balanced s single ended unbalan...

Страница 305: ...ients Sij i j occupy the other diagrams By default reflection coeffi cients are displayed in Smith diagrams transmission coefficients in Cartesian dia grams dB Mag scale See also Format softtool Remot...

Страница 306: ...background information see Chapter 4 3 4 1 Converted admittances on page 130 Remote command CALCulate Ch PARameter MEASure Trace_Name Y S11 Y S12 Y S12SER Y S12PAR CALCulate Ch PARameter SDEFine Trac...

Страница 307: ...if a balanced and two single ended logical ports are configured in the Balanced Ports dialog See Imbal ance Differential dialog Remote command CALCulate Ch PARameter MEASure Trace_Name IMB21 IMB12 CMR...

Страница 308: ...alanced in the active channel See Chapter 5 2 2 5 Balanced Ports dialog on page 311 Note If either multiple DUTs are configured see Chapter 5 12 2 3 Define Parallel Measurement dialog on page 627 or t...

Страница 309: ...efer to Chapter 4 3 5 3 Detector settings on page 133 Remote command CALCulate Ch PARameter MEASure CALCulate Ch PARameter SDEFine SENSe Ch SWEep DETector TIME 5 2 2 4 Imbalance Differential dialog Al...

Страница 310: ...o select the signal direction and hence the Imbalance Result to be calculated DUT Balanced port Balanced port Log NWA port Physical port c Logical ports j k single ended Physical port d Logical port i...

Страница 311: ...e following sections Chapter 4 3 6 Unbalance balance conversion on page 134 Chapter 4 3 6 1 Balanced port configurations on page 135 Chapter 4 3 2 Reference impedances on page 125 Chapter 4 7 5 True d...

Страница 312: ...t one balanced port is configured whose physical ports are fed by independent sources see Chapter 4 7 19 Internal 3rd and 4th source for 4 port R S ZNA on page 273 In case the balanced port is made up...

Страница 313: ...urthermore provides functions for renumbering the logical ports Remote command SOURce Ch LPORt LogPt SOURce Ch LPORt LogPt CLEar Reference Impedance tab The Reference Impedance tab of the Balanced Por...

Страница 314: ...ce Z0 50 of the single ended physical analyzer ports The default value for the differential mode is Z0d 100 2 Z0 The default value for the common mode is Z0c 25 Z0 2 Remote command SENSe Ch PORT PhyPt...

Страница 315: ...Quantities button opens the More Wave Quantities dialog which allows to select an arbitrary wave quantity e g for different source ports or higher port numbers a i Source Port i b j Source Port i Sele...

Страница 316: ...is fed to the output port port 2 of the DUT reverse measurement Tip In the trace list the source port is indicated in brackets For example a1 P1 denotes the wave a1 with source port 1 The analyzer ca...

Страница 317: ...a waves are the outgoing transmitted waves at the analyzer s test ports The b waves are the incoming measured waves The source port for the stimulus signal must be specified in addition The port numb...

Страница 318: ...easure ment data For details refer to Chapter 4 3 5 3 Detector settings on page 133 Remote command CALCulate Ch PARameter MEASure CALCulate Ch PARameter SDEFine SENSe Ch SWEep DETector TIME Display un...

Страница 319: ...connected between the analyzer ports 1 and 2 The stimulus signal is provided by the analyzer port 1 or 2 Source Port The predefined wave quantities correspond to the 2 port S parameters b1 a1 Source P...

Страница 320: ...rror corrected in the active channel If checked default the best available calibration is applied with the following prece dence Scalar power calibration User system error correction Factory system er...

Страница 321: ...he numerator of the ratio Remote command CALCulate Ch PARameter MEASure Trace_Name B2 A1 CALCulate Ch PARameter SDEFine Trace_Name B2 A1 Denominator Selects the type left pull down list and the port n...

Страница 322: ...on page 133 Remote command CALCulate Ch PARameter MEASure CALCulate Ch PARameter SDEFine SENSe Ch SWEep DETector TIME 5 2 5 Noise Figure tab Noise figure measurements are available for both non freque...

Страница 323: ...nnel Noise Figure Selects the calculated noise figure of the DUT Gain This button selects the measured gain of the DUT as used for the noise figure calculation Note that in NF channels the gain is cal...

Страница 324: ...you to set up a noise figure NF measurement Access Noise Figure tab S Params Noise Figure tool button The noise figure specific parameters in the lower part of the dialog are identical for non frequen...

Страница 325: ...inter nal noise of the VNA Remote command SENSe Ch NFIGure DEFine Source Attenuator For a precise noise figure measurement it is necessary to suppress to internal noise of the VNA To this end set the...

Страница 326: ...ing of 0 dB Remote command Receiver step attenuator see Receiver Step Att on page 477 Internal preamplifier see Receiver Step Att on page 477 Noise Bandwidth Sets the bandwidth used for the NF measure...

Страница 327: ...om the NF quickset dialog Use the checkbox to the right to enable the input field and set its value manually Remote command SENSe Ch NFIGure NTIMe Gain Det Meas Time Sets the detector time at each mea...

Страница 328: ...an check if NF calibration and measurement are set up properly without browsing through various dialogs Quickset Opens the NF quickset dialog that guides you through to setup of a noise figure NF meas...

Страница 329: ...physical temperature equal to the ambient temperature See Source noise temperature on page 262 for a detailed description Remote command SENSe Ch NFIGure TEATtenuator Sideband Correction Advanced Set...

Страница 330: ...n be decreased after calibration This allows you to inves tigate the effect of the parasitic sidebands and to increase the measurement speed since less additional measurements are required Remote comm...

Страница 331: ...te By default a linear frequency sweep is used for calculating the measurement fre quencies If a logarithmic frequency sweep is desired select this sweep type in the Noise figure setup dialog before u...

Страница 332: ...nsiders the achievable measurement time and the effort to reconfigure the R S ZNA The intention of the algorithm is to recommend a reasonably fast measurement with minimum effort in terms of changing...

Страница 333: ...g port to let the Quickset logic choose the suitable source powers for calibration and measurement Receiving Port For the receiving port you can activate or deactivate the Reverse Coupler Configura ti...

Страница 334: ...ly transferred into the corresponding measurement and calibration settings 5 2 6 Intermodulation tab Allows you to set up the channel for an intermodulation measurement and to select intermodulation p...

Страница 335: ...et up see Intermod on page 335 this button opens the intermodulation measurements dialog and activates the Intermod Product tab Intercept Point After the intermodulation measurement has been set up se...

Страница 336: ...The channel settings differ from the swept intermodulation measurement therefore a new channel is created when the spectrum measurement is activated Add CW Mode The following example shows an intermod...

Страница 337: ...the active intermodulation trace the center frequency of the related channel is used Max IM Order Defines the width of the spectrum measurement Remote command SENSe Ch FREQuency CW SENSe Ch FREQuency...

Страница 338: ...external generator as a source of the upper tone signal For a 2 port R S ZNA without Internal 2nd source and 2nd LO generator for 2 port R S ZNA the upper tone must be provided by an external generato...

Страница 339: ...biner This allows you to measure intermodulation products at the DUT output but not at the DUT input External Direct Access Use the signal from the source out direct access of the lower tone port and...

Страница 340: ...available at test port 1 The intermodulation quantities can be measured at the DUT input or at the DUT output Internal If the R S ZNA is equipped with an Internal combiner R S ZNAxx B213 4 port instru...

Страница 341: ...tandard parame ters can be set in the dialog see Start delta Freq Stop delta Freq Number of Points Base Power CW Frequency CW fixed to on page 342 The frequency distance between lower tone and upper t...

Страница 342: ...quency Overview dialog summarizes the resulting measured frequency ranges according to the current setup of the related channel Invalid frequency ranges that are not covered by the analyzer s frequenc...

Страница 343: ...ct the measured quantities that are relevant for intermodulation mea surements Access Trace Meas Intermodulation Intermod Product Intercept Point Main Tone Background information Refer to Intermodulat...

Страница 344: ...intermodulation product reveals the worst case performance of the DUT Currently intermodulation products are always measured at DUT Output Remote command CALCulate Ch PARameter MEASure CALCulate Ch P...

Страница 345: ...t point denotes the lower or upper intercept point whichever is smaller It reveals the worst case performance of the DUT DUT port defines the reference port for the intercept point calculation Possibl...

Страница 346: ...Value the compression point is calculated for each of these power sweeps The power sweep is stopped when the compression point at the current frequency is reached However the maximum number of measure...

Страница 347: ...on Value With a Compression Value of x the gain compression measurement searches for the x dB compression points of the power sweeps The x dB compression point is defined as the stimulus level where t...

Страница 348: ...equency grid the firmware performs a power sweep Start Frequency Stop Frequency See the corresponding controls on the Stimulus tab Number of Points See the corresponding controls on the Sweep Params t...

Страница 349: ...to the Amplifier Compression setup dialog but with additional configuration possibilities for frequency converting DUTs Access Meas softtool Mixer Params Gain Compression Setup Frequency Converting DU...

Страница 350: ...ailable diagrams or in a new one Remote command CALCulate Ch PARameter MEASure TraceName PmtrD1 CALCulate Ch PARameter SDEFine TraceName PmtrD1 Power Meter Shows a list of all power meters that have b...

Страница 351: ...e measured Voltage V or convert it to a Power according to the formula P V2 Re Z0 Z0 denotes the reference impedance of the source port The reference impedances are defined in the Balanced Ports dialo...

Страница 352: ...Spectrum Setup dialog on page 354 Spectrum Analysis Opens the Spectrum Setup dialog Measurement Selects the physical VNA port for the spectrum measurement Drag one of the Port buttons to the diagram...

Страница 353: ...g and Hold mode Trace mode Infinite averaging Hold mode Clear Write OFF Hold OFF AVG ON Hold OFF Min Hold OFF Min Hold Max Hold OFF Max Hold Use Reset History to reset the averaging minimization maxim...

Страница 354: ...crease the level accuracy Not using it can increase the measurement speed in particular for multi channel measurements at the possible cost of a small decrease in level accuracy Remote command SENSe C...

Страница 355: ...internal low noise amplifier LNA ON you can specify a Preamp Gain instead of a Step Att Receiving Port See Chapter 5 8 2 Power tab on page 476 Sweep Time Auto Allows you to set a sweep time or use the...

Страница 356: ...h is pre installed on instruments shipped with firmware V2 15 or higher and that can be installed on any instrument running firmware V2 15 or higher It opens the Power Added Efficiency PAE dialog 5 2...

Страница 357: ...external DLL This string is processed by the external DLL with DLL specific syntax and semantics If it is malformed or inappropriate for some reason the firmware logs and displays the error messages...

Страница 358: ...s Opens an additional dialog that allows you to specify the addresses of external devices the external DLL requires to complete its tasks For each task type a single device address can be configured T...

Страница 359: ...that is supported with FW V2 15 or higher The PAE measurement requires an external power supply R S HMP2030 that is connected to the R S ZNA via USB There are no dedicated remote commands for the PAE...

Страница 360: ...d observe the result in the measurement diagram The power supply R S HMP2030 applies a configurable DC voltage U0 between the DUT ports and measures the resulting current Im With the resulting DC powe...

Страница 361: ...ger You can also use it to specify the corresponding Result in remote commands CALCulate Ch PARameter MEASure CALCulate Ch PARameter SDEFine Define Trace Requests the external DLL for which the Trace...

Страница 362: ...dditional informa tion about the source and receive impedance recimp 50 srcimp 50 to calculate the PAE trace correctly The Additional Input is the last part of the trace identifier Cu P Src Port lower...

Страница 363: ...eters for scalar mixer measurements 5 2 13 1 Scalar Mixer Meas setup dialog The Scalar Mixer Meas dialog is an example of a Multi channel setup dialog It allows you to prepare one or more channels for...

Страница 364: ...lists in the graphical part of the dialog contain all analyzer ports or external generators which can provide the RF signal and local oscillator LO signals Generators must be configured explicitly in...

Страница 365: ...alyz er s channel base power Base Pwr the signal is swept if a power sweep is active it is at fixed power CW for the other sweep types or at the Fixed power The same applies to the IF signal The conve...

Страница 366: ...g A Mix label in the channel list indicates that a scalar mixer measurement is active Remote command SENSe Ch FREQuency CONVersion 5 2 13 2 Frequency Converting Measurements dialog The Frequency Conve...

Страница 367: ...tab scalar vector mixer measurements Offers the full set of gain measurement parameters for frequency converting DUTs Conversion Phase tab vector mixer measurements Offers the full set of phase measu...

Страница 368: ...p the channel for phase measurements on a frequency converting DUT a k a vector mixer measurements and lets you select the quantities to be measured These measurements require software option R S ZNA...

Страница 369: ...parameters for mixer measurements More Reflection Feedthru opens the vector mixer measurements variant of the dia log More Waves opens thescalar mixer measurements variant 5 2 14 1 Vector Mixer Meas...

Страница 370: ...oup Dly tab frequency converting DUT Sets up the channel for a two tone group delay measurement a k a mixer group delay measurement with embedded LO and selects the measured quantities These measureme...

Страница 371: ...2 15 1 Controls on the Two Tone Group Dly tab Setup Frequency Converting DUT Opens the Two Tone Group Delay setup dialog Measurement Allows you to select the quantities to be measured The Main Tone bu...

Страница 372: ...stant Cal Dly Variable Cal Dly Mixer Delay If Use Constant Cal Dly is checked you can enter a constant calibration delay Other wise the button is labeled Variable Cal Dly and you can click on it to lo...

Страница 373: ...e signal Remote command SENSe Ch FREQuency MDELay UTONe IF Select the receive port for the two tone group delay measurement Remote command SENSe Ch FREQuency MDELay RPORt Delta Frequency Selects the f...

Страница 374: ...the data it is important to select a display format which is appropriate to the analysis of a particular measured quantity An extended range of formats is available for markers To convert any point o...

Страница 375: ...s a trace with a typical sawtooth shape The alternative Unwr Phase format avoids this behavior Application Phase measurements e g phase distortion deviation from linearity Tip alternative formats The...

Страница 376: ...displays it in a Cartesian diagram Properties The SWR or voltage standing wave ratio VSWR is a measure of the power reflected at the input of the DUT It is calculated from the magnitude of the reflect...

Страница 377: ...complex quantity C i e C sqrt Re C 2 Im C 2 appears on the vertical axis also scaled linearly Application Real measurement data i e the stability factors and the DC voltages are always displayed in a...

Страница 378: ...inverted Smith chart to display a complex quantity primarily a reflection S parameter Properties The inverted Smith chart is a circular diagram obtained by mapping the positive complex semi plane into...

Страница 379: ...ct in the measurement results To compensate for a frequency dependent delay in the test setup a system error correction is required Note The delay for reflection factors corresponds to the transmissio...

Страница 380: ...logarithmic and segmented frequency sweeps it varies with the sweep point number m Application The aperture must be adjusted to the conditions of the measurement A small aperture increases the noise...

Страница 381: ...s no Scale Div no Ref Pos and no Max and Min values can be defined The default scale is activated automatically when a display format diagram type is selected Scale settings that are not compatible wi...

Страница 382: ...set to the value of the outer circumfer ence Auto scale does not affect the stimulus values and the horizontal axis Remote command DISPlay WINDow Wnd TRACe WndTr Y SCALe AUTO Auto Scale Diagram Adjust...

Страница 383: ...ion of the reference line in a Cartesian diagram The reference line is indicated by an arrowhead symbol at the right edge of the dia gram area The color of the symbol corresponds to the trace color Re...

Страница 384: ...e Remote command n a Decouple All Traces Decouple Trc Assigns independent scale settings to all traces to the active trace Remote command n a 5 4 3 Zoom tab Provides graphical and stimulus zoom functi...

Страница 385: ...utton to initiate the selection of a paraxial rectangular zoom area Its label changes according to the selected zoom mode Remote command n a Zoom Select Stim Zoom Select Prepares the analyzer GUI for...

Страница 386: ...diagram and the zoom area You can move the zoomed part of the trace by moving the zoom area or use the numerical input fields Max Min Start Stop to do so Remote command DISPlay WINDow Wnd TRACe WndTr...

Страница 387: ...in remote control on page 859 5 5 1 1 Controls on the Traces tab The Trace Manager button opens the Trace Manager dialog Active Trace Selects an arbitrary trace of the active recall set as the active...

Страница 388: ...m is also deleted Delete Trace is disabled if the active recall set contains only one trace In manual control each recall set must contain at least one diagram area with one channel and one trace Tips...

Страница 389: ...bar button allows you to create a trace in the active channel Tap click the Trc button to duplicate the active trace equivalent to Add Trace Drag the respective button onto the diagram area to create...

Страница 390: ...editable gray columns Table Area The table contains the following columns Name indicates name of the related trace Trace names must be unambiguous across all channels and diagram areas in a recall set...

Страница 391: ...used in addi tion Note The analyzer does not accept illegal or ambiguous trace names If an illegal or ambiguous name is specified the entry is denied Remote command CONFigure TRACe CATalog CONFigure...

Страница 392: ...me channel Remote command n a Couple All Scales Decouple All Scales Couple All Scales couples the scale settings of all traces to the scale settings of the active trace The scale settings of the other...

Страница 393: ...the sweep type the stimulus type of a data trace changes all its memory traces are deleted Active Trace vs Active Data Trace In the context of memory traces we distinguish between the active trace an...

Страница 394: ...the destination for the Data to Destination and Data Func to Destination operations The destination can be one of the following An existing memory trace of the active data trace The existing memory t...

Страница 395: ...tination stores the raw trace without the trace functions All Data Func to Destination stores the trace after it has been transformed using trace func tions Remote command CALCulate Chn MATH MEMorize...

Страница 396: ...et Hidden traces are not deleted Remote command DISPlay WINDow Wnd TRACe WndTr SHOW Delete All Mem Deletes all memory traces in the active recall set Tips Use the Trace Manager dialog to hide or delet...

Страница 397: ...e Complex Data section define math operations on raw complex trace data the controls in the Formatted Data section define math operations on for matted trace data Complex Data math is available in all...

Страница 398: ...th unchanged settings the divided subtracted curve is typically centered at 1 0 dB 0 It shows the variation of the results in subsequent sweeps The result of the division is a mathematical trace and r...

Страница 399: ...ted from a set of constant values c1 cn plus the trace points Trc1i Trcmi of all traces 1 to m in the mathematical relation Mathi Fct c1 cn Trc1i Trcmi i 1 no of points Different traces can be used in...

Страница 400: ...races of the active recall set Data and memory traces are identified by their trace names Data denotes the active data trace Mem is the memory trace associated with the active data trace or the first...

Страница 401: ...stimulus value of a power sweep If Show as Power is selected in the More Wave Quantities dialog the result is converted into a linear power before the selected trace format is applied Otherwise no con...

Страница 402: ...mathemati cal expression as it is written in the User Def Math dialog It is possible to change or create math string files using a text editor Remote command CALCulate Chn MATH WUNit STATe 5 5 5 Time...

Страница 403: ...n in a second line Trace settings in time domain representation While the time domain representation is active the trace settings behave as follows The Start and Stop settings in the Time Gate tab con...

Страница 404: ...main The drop down list shows the impulse response of a constant trace over a finite sweep range i e a rectangular function that was filtered using the different avail able window types The selected w...

Страница 405: ...6 Time domain SVSWR measurements on page 226 Remote command CALCulate TDVSwr STATe Gate Span Time domain SVSWR measurements relies on a time gate that is centered at the anten na s direct response plu...

Страница 406: ...age 489 This algorithm can increase the frequency gap i e the Freq Step Size Keep Frequency Gap and Number of Points calculates a harmonic grid based on the current Stop Frequency and the current freq...

Страница 407: ...nual Entry Examples At f 0 the reflection factor of an open ended cable is 1 It is 1 for a short circuited cable and 0 for a cable with matched termination If a cable with known termination is measure...

Страница 408: ...ime Domain tab and Time Gate tabs are hidden Background information Refer to Chapter 4 7 2 5 Time gates on page 225 Time Gate Enables or disables the time gate for the time domain and frequency domain...

Страница 409: ...to filter the trace in the time domain The drop down list visualizes how the time gate will affect a constant function after transformation back into the frequency domain The selected window is applie...

Страница 410: ...Range dialog see Chap ter 5 5 8 2 Evaluation Range dialog on page 415 The Decimal Places button opens the System Config dialog to define the maxi mum number of fractional digits for setting values and...

Страница 411: ...iation of a trace depend on the selected trace format In contrast the RMS calculation is based on linear unformatted values The physical unit for unformatted wave quantities is 1 Volt The RMS value ha...

Страница 412: ...to the group delay For more informa tion refer to Chapter 4 3 8 Delay aperture electrical length on page 140 Note To account for the propagation in both directions delay and electrical length of a ref...

Страница 413: ...where x is the selected compression value To obtain valid compression point results a power sweep must be active and the trace format must be dB or phase For dB traces the x dB compression point of an...

Страница 414: ...ear COMP PHASe CALCulate Chn STATistics NLINear COMP RESult CALCulate Chn STATistics STATe AREA Reference Value This control is only available if compression point statistics are calculated It selects...

Страница 415: ...OP CALCulate Chn STATistics NLINear COMP RDOMain USER SHOW Power ratio statistics spectrum analysis CALCulate Chn STATistics RDOMain USER on page 1040 CALCulate Chn STATistics RDOMain USER STARt on pa...

Страница 416: ...on ranges are defined similar to the marker search ranges For more infor mation see Chapter 5 7 3 2 Search Range dialog on page 458 Note A restricted evaluation range is indicated in the Trace Statist...

Страница 417: ...arker values may not behave as expected Smoothing Activates the smoothing function for the active trace which can be a data or a memory trace With smoothing active the trace value at each sweep point...

Страница 418: ...efinition above takes boder cases and rounding into account Tips Finding the appropriate aperture A large smoothing aperture enhances the smoothing effect but can hide quick varia tions of the measure...

Страница 419: ...shifted relative to the markers and the x axis In complex diagrams the trace is not affected Remote command DISPlay WINDow Wnd TRACe WndTr X OFFSet Mag Phase Real Imag Shift Trace Modifies the active...

Страница 420: ...z is calculated as follows Amplitude Dyn_Shift z z zr Phase Dyn_Shift z z e j zr Real Dyn_Shift z z Re zr Imag Dyn_Shift z z Im zr Complex Dyn_Shift z z zr Remote command n a 5 5 10 Infinite Averagin...

Страница 421: ...mation Refer to Chapter 4 4 2 Trace files on page 149 All buttons on the Trace Data tab serve as openers for related dialogs Import calls up a dialog to load a memory trace from a trace file see Chap...

Страница 422: ...the stimulus values of the imported data and of the active trace must be compatible Com patibility means that the Sweep Type of the two traces must match the position and number of the sweep points do...

Страница 423: ...g Trace Data Export snp Files Trace Trace Config Trace Data Export Data export can serve many purposes e g To process and evaluate measurement data in an external application To store measurement data...

Страница 424: ...e trace all traces of the active channel including memory traces or all traces in all channels For Touchstone file export it is possible to export the traces of the active channel or of all channels C...

Страница 425: ...ameters are always nor malized Remote command MMEMory STORe TRACe OPTion BALanced Decimal Places Opens a dialog that allows you to specify the number of decimal places for stimulus and data values in...

Страница 426: ...export the dB magnitude if trace format dB Mag is selected The trace file does not necessarily contain the full complex information about the trace For trace formats involving Cartesian diagrams dB M...

Страница 427: ...mple on pressing Open in the Import Complex Data dialog Select All Deselect All During trace data import selects deselects all traces contained in the opened trace file Auto Distribute Available for t...

Страница 428: ...signment in the created s n p file By default analyzer and s n p port numbers are identical You can interchange the port assignment to change the order of the S parameters in the created Touchstone fi...

Страница 429: ...are close to a particular target line Linearity Deviation is only available for cartesian trace formats On Activates deactivates the linearity deviation calculation using the current Constant and Slo...

Страница 430: ...2458 m s Hence the Slope determines the El Length and vice versa Remote command CALCulate Chn LDEViation ELENgth Constant Y intercept of the linear function to which the trace is compared Remote comma...

Страница 431: ...check Limit lines are available for all cartesian diagram types dB Mag limits can also be checked in complex diagrams Smith Polar Background information Refer to Chapter 4 4 1 Limit check on page 141...

Страница 432: ...t check are independent of each other Hiding the limit line does not switch off the limit check Remote command CALCulate Chn LIMit DISPlay STATe Limit Check Enables disables the limit check for the ac...

Страница 433: ...it violations are visualized on the trace if any Figure 5 15 Limit line violations in complex trace formats Remote command CALCulate Chn LIMit STATe CALCulate Chn LIMit LOWer STATe CALCulate Chn LIMit...

Страница 434: ...one of the limit checks fails then no TTL signal is generated Monitoring several traces If a channel contains several traces is possible to assign each of them to any TTL out put The assignment divide...

Страница 435: ...e Chn LIMit LOWer SHIFt 5 6 1 2 Define Limit Lines dialog The Define Limit Lines dialog defines the limit lines for the active trace on a segment by segment basis For each segment the limit is defined...

Страница 436: ...ot delete the segment but changes its screen color Start Stimulus is the stimulus x axis value of the first point of the segment Stop Stimulus is the stimulus x axis value of the last point of the seg...

Страница 437: ...tive segment Its response values are equal to the Start Response value of the active segment The segment numbers in the list are adap ted If no segment is active Insert is equivalent to Add Delete rem...

Страница 438: ...ams tab on page 488 The n 1 segments are appended to the current segment table for fur ther editing Existing limit line segments are not overwritten Both import dialogs contain the following file impo...

Страница 439: ...an enter the formula in the text field to the right of the Formula button or Tap and hold the text field to open the User Def Math dialog Remote command CALCulate Chn LIMit SEGMent Seg FORMula STATe C...

Страница 440: ...ociated with the active trace in a Cartesian dia gram area The vertical positions of the ripple lines are recalculated after each sweep only their stimulus range and distance the ripple limit are fixe...

Страница 441: ...d if no limit lines are defined In this case the info field displays No ripple defined and the limit check always passes For each trace ripple limits can only be set for a single cartesian trace forma...

Страница 442: ...page 434 5 6 2 2 Define Ripple Test dialog The Define Ripple Test dialog defines the ripple limits for the active trace on a range by range basis A separate ripple limit can be assigned to each range...

Страница 443: ...m Stop Stimulus Start Stimulus is the length of both lines if the range is within the sweep range Ripple Limit is their vertical distance See Chapter 4 4 1 2 Rules for ripple test definition on page 1...

Страница 444: ...nges Remote command CALCulate Chn RIPPle CONTrol DOMain CALCulate Chn RIPPle DATA CALCulate Chn RIPPle DELete ALL Recall Ripple Test Save Ripple Test The buttons open an Open Save File dialog to load...

Страница 445: ...er Color Scheme dialog see Chap ter 5 18 3 2 Define User Color Scheme dialog on page 778 You can choose between various options Assign the same color to traces and associated limit lines Assign differ...

Страница 446: ...You can choose between various options Change the trace color between failed measurement points Show or hide the colored squares Note Circle limit check and display of limit circles are independent of...

Страница 447: ...it check is switched off Note The R S ZNA does not have a built in audio device and loudspeaker To hear these sounds connect a USB audio device to the instrument or operate it via remote desktop Remot...

Страница 448: ...ay circle Most of the controls on this tab are only active if the active trace is displayed in a com plex format Show Border If enabled the border of the Display Circle is shown whenever the related t...

Страница 449: ...r X Center Y Defines the Display Circle either by drawing it in the diagram area or by providing its radius and center Remote command CALCulate Chn LIMit DCIRcle DATA 5 6 5 Horiz Line tab Shows or hid...

Страница 450: ...perties Markers are also convenient tools for searching special points on traces and for scaling diagrams Access Trace Marker Background information Refer to the following sections Chapter 4 2 1 3 Mar...

Страница 451: ...of an Arbitrary marker see Marker Mode on page 454 Remote command CALCulate Chn MARKer Mk Y CALCulate Chn MARKer Mk REFerence Y On Enables disables the active marker Markers remember their Marker Prop...

Страница 452: ...can be entered in the Mkr i Stimulus entry field The default position is the center of the sweep range You can also drag and drop markers in a diagram to change their X position If the Marker Mode is...

Страница 453: ...other traces then follow the movements of the tracked marker The same holds true for the reference marker Remote command CALCulate MARKer COUPled STATe 5 7 2 Marker Props tab Modifies the properties o...

Страница 454: ...nes if and how the marker s position is adjusted and if and how it can be moved in the diagram area Normal If Tracking is enabled the marker s stimulus value is updated automatically with every sweep...

Страница 455: ...er Ref Mkr Mkr is not active if the active marker is a reference marker Remote command n a Export Markers Calls up a Save As dialog to store the current marker values to a marker file The analyzer use...

Страница 456: ...r is enabled to indicate the search result Background information Refer to Basic marker search functions on page 105 5 7 3 1 Controls on the Marker Search tab Max Min Sets the active marker to the abs...

Страница 457: ...s set to the next maximum The next maximum is the maximum with the largest response value that is below the current marker response value If Min is active then the marker is set to the next minimum Th...

Страница 458: ...e marker on the active trace In this case it performs the following actions Create a channel Sets its center to the active marker s position Set its span to 10 times the previously active channel s Ba...

Страница 459: ...store up to 10 customized search ranges The Search Range s are bordered by the Start and Stop values Start must be smaller than Stop otherwise the second value is automatically adjusted Search Range p...

Страница 460: ...ge Limit Lines On Displays or hides the range limit lines in the diagram area Range limit lines are two vertical lines at the Start and Stop values of the active search range Range 1 to Range 10 The l...

Страница 461: ...Threshold to a format specific default value Remote command CALCulate Chn MARKer Mk THReshold CALCulate Chn MARKer Mk THReshold STATe Excursion Settings Defines a minimum excursion for peak searches...

Страница 462: ...e required markers are created deleted as needed Remote command CALCulate Chn MARKer Mk FUNCtion EXECute MMAXimum MMINimum CALCulate Chn MARKer Mk FUNCtion RESult Eval Range Opens the Evaluation Range...

Страница 463: ...race format and cannot be modified Changing the trace format resets the Threshold to a format specific default value Remote command CALCulate Chn MARKer MPEak THReshold CALCulate Chn MARKer MPEak THRe...

Страница 464: ...target value for the search The VNA software allows you to specify the target value in different formats see Tar get Format below For example you can search for a particular phase value in a Smith ch...

Страница 465: ...get is not found e g because the active trace does not contain the target value then the active marker is not moved away from its original position Remote command CALCulate Chn MARKer Mk FUNCtion EXEC...

Страница 466: ...ntity must be a transmission S parameter and a frequency sweep must be performed However for other formats measured quantities or sweep types the Bandfilter functions can still be useful to analyze ge...

Страница 467: ...s region M1 indicates the maximum of the peak Max M2 indicates the point on the left edge of the peak where the trace value is equal to the maximum minus the bandwidth factor Lower Edge M3 indicates t...

Страница 468: ...n on the active trace and activates Tracking A bandstop region is the lowest peak local minimum in the search range whose level is at least Bandwidth below the maximum passband value If Bandstop Ref t...

Страница 469: ...value at the active marker position plus minus the Bandwidth To be valid the peak must be above below the response value for the band edges Absolute Level The bandpass bandstop is the tallest lowest p...

Страница 470: ...ker appears in the center of the diagram Start Marker sets the beginning start of the sweep range equal to the stimulus value of the active marker leaving the end stop value unchanged The active marke...

Страница 471: ...that the delay at the marker position becomes zero Mathematically it modifies the measurement results by subtracting the delay at the current marker position The delay represents the propagation time...

Страница 472: ...the tracked marker The same holds true for the reference marker Remote command CALCulate MARKer COUPled STATe Coupling Type Determines the set of traces whose markers are coupled to the markers of the...

Страница 473: ...polar and Smith diagrams this direct relation is lost In any case a marker can be used to dis play the stimulus value of a given trace point For Time Domain traces points in the observation interval a...

Страница 474: ...e quency and Span Frequency are alternatives For a Log Freq only Start Frequency and Stop Frequency can be set Note For segmented frequency sweeps the start and stop frequencies and the num ber of swe...

Страница 475: ...ncy for Power CW Mode and Time sweeps The CW Frequency is also used as the channel base frequency for frequency con verting measurements see Chapter 4 7 3 Frequency conversion measurements on page 234...

Страница 476: ...Power tab The Power tab provides settings related to transmit and receive power It is also dis played on the Pwr Bw Avg softtool 5 8 2 1 Controls on the Power tab For power sweeps the Start Power Sto...

Страница 477: ...r Cal dialog Leveling Table to create a leveing dataset and Leveling Data sets dialog to activate it For background information see ALC with mmWave convert ers on page 93 Remote command SOURce Ch POWe...

Страница 478: ...ion amplifier b1 and the Internal low noise preamplifier b2 Remote command SENSe Ch PAMPlifier2 STATe SENSe Ch PAMPlifier2 VALue 5 8 2 2 Power Config dialog Except for the ALC settings which are cover...

Страница 479: ...ialog to activate it For background information see ALC with mmWave convert ers on page 93 Remote command SOURce Ch POWer ALC CSTate ALC On table area Enables or disables ALC for the respective port R...

Страница 480: ...not use the configured offset or the single ended deembedding network Remote command SOURce Ch POWer Pt ALC VNETwork ALC Meas Port ALC Meas Receiver Defines the received signal to be used for ALC i e...

Страница 481: ...STOLerance Show Advanced Settings Shows or hides advanced ALC settings ALC Optimize Show Advanced Settings Enables configures ALC optimization Auto default The firmware decides whether speed optimizat...

Страница 482: ...opor tional gain Kr the integration time Ti and the Control Time manually The latter specifies the maximum duration of the control loop phase per measurement point With Auto Settling enabled the firmw...

Страница 483: ...artesian diagram Time Center corresponds to the center of the Cartesian diagram i e Time Start Time Stop 2 Time Span corresponds to the diagram width i e Time Stop Time Start Time Start and Time Stop...

Страница 484: ...Time c0 Velocity Factor for transmission measurements Distance 1 2 Time c0 Velocity Factor for reflection measurements The factor 1 2 accounts for the return trip from the DUT to the receiver The velo...

Страница 485: ...an be selected independently for each segment see Chapter 5 10 2 2 Define Segments dialog on page 499 Bandwidth Bandwidth the measurement bandwidth of the IF filter Within the value range the entered...

Страница 486: ...frequency dependent dynamic factor The bandwidth reduction reduces the trace noise at small frequencies and improves the accuracy of the time domain transform Remote command SENSe Ch BANDwidth RESolu...

Страница 487: ...n Reduce Noise and Flatten Noise mode depending on the trace type Reduce Noise Cumulative moving averages of the real and imaginary parts of each measurement result provides the most effective noise s...

Страница 488: ...page 90 5 10 1 Sweep Params tab Allows you to define the scope and timing of the measurement in the active channel Segmented sweeps In Segmented linear frequency sweeps the sweep parameters can be se...

Страница 489: ...e increases roughly linearly with the number of points See also Chapter 4 1 4 Sweep control on page 87 Remote command SENSe Ch SWEep POINts Freq Step Size Sets the distance between two consecutive swe...

Страница 490: ...delay and thus the total sweep time it is possible to select Alternated driving mode see Driving Mode on page 632 Remote command SENSe Ch SWEep DWELl All Partial Meas ments First Partial Meas ment If...

Страница 491: ...ommand SENSe Ch SWEep CTIMing STIMe MODE Wait Time per Point For the User Def Sweep Time Mode you can specify the wait time between sweep point measurements If you select a wait time that is smaller t...

Страница 492: ...lus settings see Chapter 5 8 1 Stimu lus tab on page 473 The step width between two consecutive sweep points is con stant and given by Span n 1 where n is the specified Number of Points n 1 Lin Freq i...

Страница 493: ...1 with the condition that the step width is constant on the log arithmic scale Log Freq sweeps are suitable for the analysis of a DUT over a large frequency range e g over several octaves In a Cartesi...

Страница 494: ...of all segments The following example shows a segmented frequency sweep with 2 segments The forward transmission parameter S21 is mea sured and a dB Mag scaled y axis is used In the frequency range be...

Страница 495: ...nt triggers the first partial mea surement of a measurement point The time interval between two consecutive mea surements depends on the trigger settings and the sweep parameters especially the number...

Страница 496: ...zer is analogous to an oscilloscope In a Cartesian diagram the measure ment result is displayed as a trace over a linear time scale The following example shows a Time sweep with a DUT that does not ma...

Страница 497: ...ents dialog that allows to set up the channel for a Segmented frequency sweep Seg X Axis Scales the x axis for a segmented frequency sweep In Freq based mode the x axis covers the frequency ranges of...

Страница 498: ...ep REVerse Fast Power Sweep This control is only enabled if sweep type Power is selected The alternative power swep methods are most suitable for compression measurements on freqency converting DUTs w...

Страница 499: ...ment While this method does not make an assumption on the phase drift it assumes that the DUT can cope with rapid power level changes Remote command SENSe Ch SWEep FPOWer 5 10 2 2 Define Segments dia...

Страница 500: ...unexpected behavior when used in overlapping sweep segments The reason is that the assignment of markers to traces in overlap ping segments is ambiguous If you want to analyze a particular segment us...

Страница 501: ...Point List Import Export The buttons open standard dialogs to import export sweep segment settings to from an ASCII file SegList Import replaces the current segment list by a sweep segment list loade...

Страница 502: ...yed Columns dialog allows you to select the channel settings that can be defined per sweep segment These settings are displayed in the Define Segments dia log and in the Point List opened via Show Poi...

Страница 503: ...dth on page 485 Selectivity defines the selectivity of the IF filter used for each sweep segment See IF Filter digital on page 486 LO RF allows you to define segment specific Image Suppr settings see...

Страница 504: ...s switched off Remote command SENSe Ch SEGMent Seg POWer LEVel CONTrol SENSe Ch SEGMent Seg POWer LEVel SENSe Ch SEGMent Seg BWIDth RESolution SENSe Ch SEGMent Seg BWIDth RESolution CONTrol SENSe Ch S...

Страница 505: ...R S ZNA K91 it provides four configurable output trigger signals that can be used to synchronize external devi ces with the measurement These signals are available at the rear panel connectors Trigger...

Страница 506: ...External trigger mode can be configured using the Sequence Delay and Sig nal Type settings In Manual trigger mode the trigger signal is generated by the Manual Trigger button If Multiple Triggers is a...

Страница 507: ...nce If the analyzer receives a trigger event while the last sequence is still running the R S ZNA skips the trigger event and generates a message If it observes lost trigger events the analyzer increm...

Страница 508: ...ces and delays for the trig gered measurement sequences The dialog is available and its settings are valid if the analyzer is configured for Multiple Triggers see FreeRun External Manual Multi ple Tri...

Страница 509: ...r Out interfaces Access Channel Sweep Trigger Trigger Out Manager Trigger Out connectors A to D are provided by the optional Trigger board If this hard ware option is not installed it is not possible...

Страница 510: ...Ch AUXiliary n DELay Width If pulses are used as trigger out signals see Signal Type on page 509 this parame ter specifies the pulse width Remote command TRIGger CHANnel Ch AUXiliary n DURation on pa...

Страница 511: ...guage this setting applies to the active channel only Use All Channels Continuous All Channels on Hold to select a common sweep mode for all channels For remote languages other than DEFAULT this setti...

Страница 512: ...nnels are swept cyclically the Single sweep channels are on hold First the Single sweep mode channels are swept one after the other each with its channel spe cific number of Sweeps Then the Continuous...

Страница 513: ...duration sweeps that are executed in single sweep mode by observing the dialog it is easy to realize when the sweep is done The possible sweep stages and how they are displayed partly depend on the se...

Страница 514: ...mote languages other than DEFAULT the Sweeps setting above the separator line defines the common number of sweeps that are executed on each channel in sin gle sweep mode The table below the separator...

Страница 515: ...libration on page 179 Chapter 4 5 6 Scalar power calibration on page 191 Chapter 4 5 7 SMARTerCal on page 197 Chapter 4 1 6 Data flow on page 93 5 11 1 1 Measurement modes and default calibration setu...

Страница 516: ...ion setup Standard only one port OSM POSM at least one two port UOSM PUOSM Spectrum R S ZNA K1 required POSM Scalar mixer R S ZNA K4 required 1x PUOSM or 2x POSM see screenshot above IMD R S ZNA K4 re...

Страница 517: ...ctions Cal Active Channel section The main purpose of the buttons in this section is to calibrate the current channel Quick Start Calibration immediately starts the calibration of the current channel...

Страница 518: ...Cal Only section The Scalar Power Cal button opens the Power Cal wizard which allows you to perform Ref Receiver Meas Receiver and scalar source power Source Flat ness calibrations for the current ch...

Страница 519: ...corrections and or power corrections from the acquired data and applies the result to the related channels The Reduced Through logic and the powerful Calibrate All function help you to keep the number...

Страница 520: ...the remote control command SENSe Ch CORRection CDATa Manual calibrations If a manual calibration shall be performed the calibration wizard allows you to acquire error correction data for every require...

Страница 521: ...the buttons representing the calibration standards to start the corresponding cali bration sweeps If Show Cal Kit Label is enabled on the Calibration tab of the System Config dialog an additional Cal...

Страница 522: ...RRection COLLect ACQuire SELected See also SENSe Ch CORRection COLLect LOAD SELected Restart Sweep on Std Meas If this function is active a new standard measurement initiates a new sweep starting at t...

Страница 523: ...CORRection STIMulus SENSe Ch CORRection STIMulus PORT PhyPt Automatic calibrations The calibration wizard can also guide you through a calibration using a calibration unit plus a power meter for SMAR...

Страница 524: ...2 20 2 If a SMARterCal is performed a Replace the calibration unit by the power meter b Start the power calibration sweep The upper part of the screen displays power trace diagrams and the lower part...

Страница 525: ...rors If the correct standard type is measured and everything is properly connected then the measured trace is expected to be similar to the typical trace Power Sweep Diagrams Power sweep traces are di...

Страница 526: ...is enabled when sufficient data have been acquired for the calibrated ports The button starts the calculation of the calibration data and closes the calibration wiz ard The results are applied to the...

Страница 527: ...ct AUTO SAVE SENSe Ch CORRection COLLect AUTO POWer 5 11 1 4 Calibration setup dialog Allows you to configure the calibration setup of the available channels Access Channel Cal Start Cal Cal Active Ch...

Страница 528: ...fault setting and the selected power cals depend on the active channel mode E g for a standard S parameter channel a SMARTerCal is proposed if a power meter is available Source Leveling Src Lev Method...

Страница 529: ...e 5 23 Cal settings for inline calibration systems Note Currently for each channel either manual calibrations automatic calibrations or inline calibration systems are allowed Port i Cal Settings Tick...

Страница 530: ...appear in each port assignment and that are as the center of all Through measurements Remote command SENSe Ch CORRection COLLect AUTO CPORt Calibration Type Cal Settings Displays the calibration type...

Страница 531: ...n types Trans Norm One Path Two Ports the Source port must be specified in addition The full n port calibration types n 1 e g TOSM are symbolized by a closed square symbol The number of arrows increas...

Страница 532: ...the cal unit such as the connec tion of additional adapters you can generate modified sets of characterization data using the cal unit characterization wizard See Chapter 5 11 2 3 Characterize Cal Uni...

Страница 533: ...ally set according to the connector type of the selected calibration unit port Detect Port Assignment starts a procedure by which the R S ZNA with a little help from the attached calibration unit auto...

Страница 534: ...ndard applications To account for modifications of the cal unit such as the connec tion of additional adapters you can generate modified sets of characterization data using the cal unit characterizati...

Страница 535: ...a of the active calibration are reused if available which allows the calibration to be repeated without repeating all measurements Remote command SENSe Ch CORRection COLLect AUTO REPeat on page 1286 I...

Страница 536: ...you to specify the harmonic orders to be calibrated in the current channel By default the firmware selects all harmonic orders that are relevant for an existing harmonics trace in this channel Remote...

Страница 537: ...t does not increase the number of sweep points and uses interpolation if necessary Remote command SOURce Ch POWer CORRection IMODulation METHod Calibration Mixer Settings Defines the properties of the...

Страница 538: ...anced SOURce PhyPt ATTenuator on page 1242 SENSe Ch CORRection ADVanced SOURce PhyPt ATTenuator STATe on page 1243 ZNA Port Power Power Result as displayed in the port settings dialog Cal Power Offset...

Страница 539: ...emote command SENSe Ch CORRection ADVanced POWer PhyPt SENSe Ch CORRection ADVanced POWer PhyPt STATe Preamp Gain Preamplifier gain used during calibration if activated Only available if the R S ZNA i...

Страница 540: ...es carefully chosen power levels depending on the active receiver step attenuator and internal preamplifier configura tion For a noise figure measurement the power level at the test port input must no...

Страница 541: ...command SOURce Ch NFIGure CALibration TPOWer SOURce Ch NFIGure CALibration TPOWer STATe Noise Det Meas Time Defines the detector time of the RMS detector which is used for the noise measure ment durin...

Страница 542: ...ary according to the calibration stage While no calibration is performed or during a source power calibration Power the following traces are displayed A limit line double horizontal represents the tar...

Страница 543: ...calibration can be performed If a SMARTerCal is active additional receiver power calibrations are not allowed and the R S ZNA only offers the possibility to perform Source Flatness calibra tions of th...

Страница 544: ...545 Power Cal dialog Meas Receiver on page 546 Power Cal dialog Source Flatness on page 550 and Power Cal dialog Leveling Table on page 548 A green checkmark indicates that the calibration data has b...

Страница 545: ...Ch POWer CORRection DATA SOURce Ch POWer CORRection DATA PORT PhyPt SENSe Chn CORRection PSTate Power Cal dialog Ref Receiver In Ref Receiver mode the Power Cal dialog guides you through a reference...

Страница 546: ...by default the reference receivers are cali brated for all resulting frequencies even if they are currently not relevant for some ports Using this switch you can limit the calibrated frequencies to th...

Страница 547: ...alibration If the source port is equal to the calibrated port the measurement receiver is cali brated by the wave that is reflected back by a connected Open or Short standard Connect the Open or Short...

Страница 548: ...t and allows you to define the leveling grid for the current channel A circuit diagram visualizes the setup The leveling function and the Leveling Table variant of the Power Cal dialog are only availa...

Страница 549: ...sweep points of the leveling grid By default the R S ZNA uses 201 sweep points The higher the number of Sweep Points the more accurate the leveling but also the longer it takes to create the level in...

Страница 550: ...ee step 3 of the Leveling procedure Remote command SOURce POWer PhyPt CORRection SLEVeling ACQuire Power Cal dialog Source Flatness In Source Flatness mode the Power Cal dialog guides you through a so...

Страница 551: ...ration procedure Remote command SOURce Ch POWer PhyPt CORRection ACQuire SOURce Ch POWer PhyPt CORRection COLLect ACQuire 5 11 1 6 Calibrate All dialog The Calibrate All dialog guides you through the...

Страница 552: ...deleted Use the checkboxes in the Enable column to enable disable the related calibration definition Use the ellipsis button in the Channels column to assign the related cali bration definition also...

Страница 553: ...al is disabled if both manual and auto matic calibration definitions are enabled in the Calibration definition table Save Cancel Saves the channel calibration definitions to the current setup or disca...

Страница 554: ...es the overall progress of the guided calibration After all required calibration steps were performed the Guided Calibration wizards asks you to connect your DUT to the VNA as you have specified in th...

Страница 555: ...the current recall set you are prompted to select the channels to be calibrated Use Active Channel if you only want to calibrate the active channel All Channels if you want to calibrate all channels...

Страница 556: ...ation proceeds through the connected ports p 1 4 in ascending order measuring Spp for the Open Short and Match standard PASS all measured reflection parameters are within the specified Validation Lim...

Страница 557: ...ulate CALValidate CHARacteriza Calibration Standard Selects the calibration standard to be measured in advanced mode Validation Limits The Limits button opens the Cal Validation Limits dialog that all...

Страница 558: ...ter 4 5 2 Calibration standards and calibration kits on page 172 Chapter 4 5 5 3 Characterization of calibration units on page 183 The buttons in the Cal Devices tab open the following dialogs Cal Con...

Страница 559: ...r type you can still change its name offset model and reference impedance If you switch between sexed and sexless all kits assigned to the connector type are deleted Char Imp The characteristic impeda...

Страница 560: ...M type lines the relative permittivity r is needed for the conversion of a ZVR type Loss in units of dB sqrt GHz into a Keysight type Offset Loss in units of G s and vice versa see View Modify Cal Kit...

Страница 561: ...er types on page 175 Chapter 5 11 2 1 Cal Connector Types dialog on page 558 The contents of the Available Cal Kits table vary depending on the selected Connec tor Type The table can also contain kits...

Страница 562: ...Connector Type The Connector Type table displays the available cal kit connector types Select a row in this table to get the list of Available Cal Kits Remote command SENSe Ch CORRection CONNection C...

Страница 563: ...ibration kits SENSe CORRection CKIT ConnType SELect SENSe CORRection CKIT ConnType LSELect SENSe CORRection CKIT DMODe SENSe CORRection CKIT DELete Query connector types and calibration kits SENSe Ch...

Страница 564: ...alidity range of all measured standards and possibly generates a warning s1p File and s2p File define whether the characteristics of the standard are described by a Touchstone file rather than by a ci...

Страница 565: ...page 565 Remote command SENSe CORRection CKIT StandardType SENSe CORRection CKIT StandardType WLABel Read s1p File Read s2p File Opens a file selection dialog where you can select a Touchstone file c...

Страница 566: ...58 In a Keysight Model a calibration standard is characterized by its Delay in s its characteristic impedance Z0 in and its Offset Loss in G s Otherwise the standard is characterized by the R S ZVR co...

Страница 567: ...dent and approximated by the first four terms of the Taylor series around f 0 Hz See also description of the load parameters for the different standard types in Chap ter 4 5 2 1 Calibration standard t...

Страница 568: ...n Set Password opens a dia log to enter the password and activate password protection at the next time the Set Password dialog is opened Enter an empty string no password to deactivate pass word prote...

Страница 569: ...n wizard Start Characterization The guided characterization consists of the following steps 1 Characterization Select the characterized ports and cal unit standards to ini tiate the characterization s...

Страница 570: ...TO SENSe Ch CORRection COLLect AUTO PORTs SENSe CORRection COLLect AUTO PORTs CONNection SENSe CORRection COLLect AUTO CKIT PORTs Take OSM Take All OSM and Through Take OSM starts a calibration sweep...

Страница 571: ...S ZN Z15x they can also be saved to an SD card inserted at the calibration unit Activate this checkbox to save the characterization data to the SD card Tip If the characterized calibration unit does...

Страница 572: ...ons open the Start Cal tab and select Scalar Power Cal Power Cal to perform the necessary calibration sweeps with default power cali bration settings You do not need any of the buttons in the Pwr Cal...

Страница 573: ...e Remote command SOURce Ch POWer PhyPt CORRection COLLect AVERage NTOLerance Flatness Cal Convergence Modifies the amount of power correction for each of the flatness calibration sweeps The power corr...

Страница 574: ...Meter Shows a list of all power meters that have been properly configured and are available for the power calibration of a source port The last configured power meter is selected by default See Confi...

Страница 575: ...erter LO are included in the source port overview Remote command SOURce Ch POWer PhyPt LEVel IMMediate OFFSet SOURce Ch POWer PhyPt CORRection LEVel OFFSet Channel Base Power Port Cal Power Allows you...

Страница 576: ...he Cal Power Off set has no impact on the source power Example Use of an amplifier in the signal path Assume that a DUT requires a constant input power of 25 dBm and that the mea surement path contain...

Страница 577: ...mpli fier is connected between the calibrated test port and the power meter ensure that the Reference Receiver Cal Power does not exceed its maximum input power Remote command SOURce Ch POWer PhyPt CO...

Страница 578: ...nfiguration dialog The Two Port Configuration dialog defines the transmission characteristics of an additional two port in the calibrated frequency range Access Power Meter Transmission Coefficients d...

Страница 579: ...possibly has to interpolate or extrapolate the transmission coefficients Remote command SOURce Ch POWer PhyPt CORRection TCOefficient FEED Import File Imports the transmission coefficients from a tra...

Страница 580: ...nnel and trace appears in the setup information Setup Info Setup Remote command SENSe Ch CORRection STATe Load Match Correction Load match correction prevents a possible impairment of the transmission...

Страница 581: ...calibration status of each channel and trace appears in the setup information see Setup Tab on page 805 Remote command SENSe Ch CORRection PCAL Active Power Cals Opens the Active Power Cals dialog Pow...

Страница 582: ...ows all source ports together with their possible power calibrations Unavailable cali brations i e calibrations that have not been performed yet are grayed out If external generators are configured th...

Страница 583: ...flatness cals at the converter RF in or out ports are only used if the converter port is not configured to use leveling data Remote command SOURce Ch POWer PhyPt CORRection STATe SOURce Ch POWer PhyP...

Страница 584: ...pply Apply to All The buttons between the tables are used to modify the calibration pool and apply cali bration data sets cal groups to channels Add copies the correction data of the selected channel...

Страница 585: ...ding table cell Delete from Pool deletes a cal group file from the pool Note however that calibra tions being used in any of the opened recall sets cannot be deleted Remote command MMEMory DELete CORR...

Страница 586: ...the selected calibration Remote command SENSe Ch CORRection DATE SENSe Ch CORRection DATA PARameter Sfk SENSe Ch CORRection DATA PARameter Sfk PORT PhyPt SENSe Ch CORRection DATA PARameter COUNt SENSe...

Страница 587: ...rols on the METAS Cal tab option K50 METAS Cal Active After a successful METAS calibration this checkbox enables or disables the uncer tainty calculation for the related channel If enabled measurement...

Страница 588: ...ays appended If no measurement data are available the firmware displays a message box instead of the Measurement Filename dialog Export Data to METAS VNA Tools Saves the current state of the METAS VNA...

Страница 589: ...ration Manual dialog This dialog allows you to set up and perform a METAS Calibration of the active chan nel Access Channel Cal METAS Cal METAS Calibration Start Manual Only a single VNA channel can b...

Страница 590: ...ection COLLect METHod DEFine CalName MTOSm MUOSm MOSM MTRL Detector Selects the detector type the analyzer uses during the calibration AVG The average detector eliminates noise contributions which are...

Страница 591: ...es are connected to the related ports The cable models are read from the METAS VNA Tools database Additional cable models can be added on a project specific basis Remote command SENSe CORRection METas...

Страница 592: ...th Uncertainties can be one of the following a zip file containing calibration standard characterizations in a format supported by METAS VNA Tools sdatb sdatx sdatcv calstd a scolcv file See the docum...

Страница 593: ...Active is enabled automatically In parallel to the METAS Calibration a regular VNA calibration without uncertainties is created This calibration can be activated if METAS Cal Active is unchecked 5 11...

Страница 594: ...cation Kit to import your verification kit Import Verification Kit Opens the Import Verification Standards with Uncertainties dialog A Verification Kit with Uncertainties is a ZIP file containing veri...

Страница 595: ...rification By selecting a button representing a verification standard the required measurements are performed The raw measurement data are handed over to METAS VNA Tools that returns the calibrated re...

Страница 596: ...about addi tional reconnections and or cable movements The latter is only applicable if cables were configured during the setup of the METAS calibration Access main toolbar icon or Channel Cal METAS C...

Страница 597: ...etup of the METAS calibration Remote command SENSe Ch CORRection METas CABLe REConnection SENSe Ch CORRection METas CABLe MOVement Measurement Filename Allows you to configure the name of the measurem...

Страница 598: ...elected channel one of these traces becomes the active trace The order of all channels in a recall set is given by the channels creation time By default the channels are named Ch1 Ch2 so that Ch n 1 p...

Страница 599: ...Dow Wnd TRACe WndTr FEED CONFigure TRACe Trc CHANnel NAME CONFigure TRACe Trc CHANnel NAME ID New Channel Diagram Creates a channel and a trace with default settings and assigns the created trace to a...

Страница 600: ...log and the de embedding tabs and dock widgets are disabled Related remote commands generate an error When Fixture Simulator is reactivated the situation before the deactivation is restored Note The O...

Страница 601: ...AME ID CONFigure CHANnel Ch MEASure STATe INITiate Ch CONTinuous Add Delete The buttons below the channel table add and delete channels Add adds a new channel to the list The new channel is named Ch n...

Страница 602: ...he upper part gives access to the RFFE interface settings and allows manual command execution and result display see Basic RFFE interface settings and command execution on page 604 The lower part allo...

Страница 603: ...rea up to column Output Voltage allows you to define and apply the GPIO pin voltages see Basic GPIO configuration on page 607 The right part allows you to define and execute voltage and current measur...

Страница 604: ...es RFFE1 and RFFE2 If at least one of the RFFE commands reads back values an additional result area is shown below the table For each of these read back commands it displays the respec tive read count...

Страница 605: ...a dialog that allows to define the RFFE command to be sent In this dialog enter the following parameters according to the device to be controlled SA a slave address between 0 and 15 as 1 hex digit CMD...

Страница 606: ...e and current measurements on the RFFE and GPIO pins are only possible with Var 03 of the extension board R S ZN B15 Z15 part number 1323 9355 03 or 1325 5905 03 Output Voltage Defines the output volt...

Страница 607: ...unt settings of the current channel Use Set to activate them manually The measurement sampling time can only be set via remote command It is not channel specific and applies to RFFE and GPIO measureme...

Страница 608: ...Port STATe Voltage Output Voltage Sets the default voltage of the respective GPIO pin for R S ZN B15 Z15 Var 02 Var 03 Remote command CONTrol Ch GPIO Port VOLTage DEFault Apply Use the Apply button to...

Страница 609: ...dialog Range Shunt Range defines an upper bound of the current to be measured on the respective GPIO pin The analyzer firmware automatically selects a suitable shunt resistance Possible ranges for por...

Страница 610: ...t Make sure that the required RFFE bus interfaces and GPIO ports are enabled by setting the respective Seq flags in the RFFE Config dialog see Basic RFFE interface settings and command execution on pa...

Страница 611: ...ble Remote command Unsegmented sweeps CONTrol Ch SEQuence COUNt CONTrol Ch SEQuence CLEar ALL Segmented sweeps CONTrol Ch SEGMent Nr SEQuence COUNt CONTrol Ch SEGMent Nr SEQuence CLEar ALL RFFE column...

Страница 612: ...chan nel line The arbitrary port configuration is part of the Frequency Conversion Measurements option R S ZNA K4 If this option is not equipped the Port Config Tab is not availa ble Background inform...

Страница 613: ...isabled for the rela ted channel see Fixture Simulator on page 600 the Balanced Ports button is inactive grayed out Multiple DUTs Opens a dialog that allows you to set up the analyzer for parallel mea...

Страница 614: ...urements on page 234 If any of the port frequencies are changed from their preset values the frequency con version mode is activated on pressing Apply or OK in the dialog An Arb label appears in the c...

Страница 615: ...for the active channel The settings are restored when the RF power is switched on again While the RF power is switched off the port can still be used as a receive port Remote command SOURce Ch POWer...

Страница 616: ...settings and hence are read only The result is displayed in the Frequency Result column A red Freq Conversion cell indicates that certain hardware limits are exceeded Remote command SOURce Ch FREQuen...

Страница 617: ...s calculated according to the Receiver Freq Conver sion formula and either applies to the reference receiver and the measurement receiver a b or to the measurement receiver only only b Modify Frequenc...

Страница 618: ...sion formula is removed from the list all Freq Conversion definitions using this formula are replaced by the unlinked formula Frequency Conversion Formula The conversion formula has been modeled accor...

Страница 619: ...ource ports include all configured external generators Source Gen Each port is displayed with its port number and device type Info For each port the available power range and resulting port power are...

Страница 620: ...tor in the source path of each port You can either select one of the possible attenuation factors 0 dB 20 dB 40 dB or select Auto to let the firmware decide default Remote command SOURce Ch POWer Pt E...

Страница 621: ...SENSe Ch POWer ATTenuation Wb Nb IF Gain Controls the gain of the wideband and narrowband signal paths Same functionality as IF Gain Mode on the Mode tab Remote command SENSe Ch POWer GAINcontrol GLOB...

Страница 622: ...Via the direct source monitor access connector see Chapter 4 7 33 Direct source monitor access on page 279 Can only be selected for analyzer ports 1 and 3 and only if the optional source monitor acces...

Страница 623: ...ency input as it is for the mm con verter IF frequency Remote command SENSe Ch PATH Pt IFINpath SENSe Ch PATH Pt MEASurement IFINpath SENSe Ch PATH Pt REFerence IFINpath Rear IF Frequency Sets the fre...

Страница 624: ...ncy conversion arbitrary mode depending on the cur rent port configuration If individual port frequencies are selected the arbitrary mode is activated If individual port powers but no individual frequ...

Страница 625: ...and the tab s related parameter group Arbitrary Frequency Arbitrary Power Receiver Level or Input Output are available for selection Stimulus dialog The Stimulus dialog gives access to the parameters...

Страница 626: ...e Sweep Frequency Sweep Enter Dis play x x x Start x Stop x Power x x x Power Sweep Enter Dis play x x x Start x Stop x Frequency x x x Other params Enter Dis play x Number of Points x x Enter Display...

Страница 627: ...ngle DUT in parallel The Define Parallel Measurement dialog allows you to configure these DUTs and to declare the connec tions between physical VNA ports and DUT ports Furthermore it provides convenie...

Страница 628: ...eembedding network DUT Definition tab Modify an existing DUT The DUT table allows you to rename an existing DUT 1 Locate the related row in the DUT table 2 In the DUT column specify the DUT s name Con...

Страница 629: ...ect one of its constituent DUT ports b In the Balanced Ports tab select Single to dissolve all related balanced ports Reference Impedance tab The Reference Impedance tab of the Define Parallel Measure...

Страница 630: ...hs then the resulting frequency offsets are also differ ent Remote command SOURce Ch GROup Grp SIMultaneous FOFFset STATe Minimum Offset The Minimum Offset can be specified either as an absolute value...

Страница 631: ...ia remote control command SOURce Ch GROup SIMultaneous FOFFset CONDition The configuration problems listed above are indicated as error codes 8 invalid offset or frequency spac ing and 6 no simultaneo...

Страница 632: ...pped mode In Chopped mode the analyzer completes the necessary sequence of partial measurements at each sweep point and obtains the result measurement point before proceeding to the next sweep point A...

Страница 633: ...e of several spurious signals setting the Image Suppr parameter globally can be insufficient To improve the result perform a segmented frequency sweep and assign independent LO frequencies to the indi...

Страница 634: ...t and receiver see Drive port specific settings on page 636 Remote command SENSe Ch POWer GAINcontrol GLOBal Manual Config The Manual Config button opens the Wideband IF Gain Manual Configuration dia...

Страница 635: ...ncy Note The Track LO functionality is now implemented within the original channel Before V1 80 of the analyzer firmware each time Track LO was switched on a new independent channel was created In par...

Страница 636: ...h POWer GAINcontrol ALL 5 13 2 Pulse Mod tab Gives access to the pulse generation pulse modulation and pulse profile measure ment functionality of the R S ZNA As outlined in the table below the relate...

Страница 637: ...ettings part of the Pulse Mod tab allow you to set up con figure and run Chapter 4 7 6 1 Live pulse analysis with R S VSE on page 251 Configure VSE VSE Settings Opens the VSE Connection Configuration...

Страница 638: ...e subsequent sweeps Make sure that your DUT can handle the potentially doubled pulse sequence with out being damaged or changing its characteristics Remote command CONTrol VSE MODE Trace b wave VSE Se...

Страница 639: ...lled either via internal pulse generators or externally via the PuMo In Out connectors The latter requires the optional trig ger board R S ZNA B91 If the optional trigger board R S ZNA B91 is availabl...

Страница 640: ...he measurement Master pulse settings Pulse Gen Enables or disables pulse generation Remote command SENSe Ch PULSe GENerator Id STATe Master Pulse Clock Source Defines whether the Master Pulse is provi...

Страница 641: ...generators to keep the Duty Cycle at the specified percent age The same pulse width and the minimum possible pulse delay are used for all sources These settings are only enabled if Master Pulse Clock...

Страница 642: ...TRIGger CHANnel Ch AUXiliary n DURation Pulse Train Pulse trains can only be configured for the port related pulse generators The Pulse Train button opens the Define Pulse Train dialog on page 642 th...

Страница 643: ...ipped with the Trigger board the Internal pulse modulators can be controlled externally via the PuMo In connectors Remote command SENSe Ch PULSe INTernal STATe Port Activates or deactivates the respec...

Страница 644: ...igger Out Manager dialog Remote command TRIGger CHANnel Ch AUXiliary n INTerval PGENerator NONE TRIGger CHANnel Ch AUXiliary n STYPe POSitive NEGative Measurement settings Sets up the Pulse Profile me...

Страница 645: ...ound information see Chapter 4 7 6 1 Live pulse analysis with R S VSE on page 251 Access Pulse Mod tab Configure VSE Start Connect VSE Disconnect VSE Select your target R S VSE using Local VSE or the...

Страница 646: ...t Manually added addresses are stored in the active R S ZNA setup Remote command CONTrol VSE ADDRess on page 1133 Save VSE Configuration If a connection to a R S VSE instance is established you can do...

Страница 647: ...ool bar shows the Src Coh button with exactly the same func tionality as the Source Coherence button in the softtool tab Remote command SOURce Ch CMODe STATe True Differential Mode See True Differenti...

Страница 648: ...ontrol apply ing port match corrections and calibration checked default If checked the phase values are relative to a common zero Otherwise the phase rela tion between the different ports is undetermi...

Страница 649: ...alance Sweep Settings below it are only visible if option R S ZNA K6 True differential mode is available and is only enabled if Source Coher ence On is unchecked Remote command SOURce Ch TDIF STATe Im...

Страница 650: ...MBalance AMPLitude STOP Topology graphical button Visualizes the logical port configuration If the Balanced Ports dialog is not already in the background you can open it from here 5 13 4 Timer Setting...

Страница 651: ...loss of non dispersive and per fectly matched transmission lines between the calibrated reference plane and the DUT It also contains advanced functions for deembedding embedding the DUT from into mor...

Страница 652: ...set panel Allows you to activate or deactivate offset loss correction for selected physical ports and to set related parameters Access Overview panel Offset Figure 5 34 Offset Embed dock widget Offset...

Страница 653: ...unt for the propagation in both directions the magnitude shift of a reflection parameter due to a given loss is twice the magnitude shift of a transmission parameter See also Chapter 4 6 1 6 Applicati...

Страница 654: ...Touchstone file from the dock widget Access Overview panel Single Ended The Single Ended panel can also be activated by selecting the Single Ended tab soft tool tab Refer to its description for backgr...

Страница 655: ...uments Remote command MMEMory LOAD VNETworks Ch SENDed DEEMbedding PhyPt MMEMory LOAD VNETworks Ch SENDed EMBedding PhyPt 5 14 1 4 Port Sets panel The Port Sets panel allows you to configure the Port...

Страница 656: ...late Ch TRANsform VNETworks PPAir EMBedding ListId DELete Active The checkboxes in the Active column activate or deactivate the configured de embeding for the related port set i e adds or removes it t...

Страница 657: ...ding embedding of balanced ports Access Overview panel Balanced If the selected Balanced tab is defined using one or more touchstone files these files can be selected from the dock widget For network...

Страница 658: ...is also possible to instruct the analyzer to assume an Increasing Port Sequence i e that the low port numbers are on the VNA side Furthermore it is possible to Swap Gates i e to instruct the analyzer...

Страница 659: ...on flow without changing its parameters Remote command CALCulate Ch TRANsform VNETworks GLOop DEEMbedding group STATe CALCulate Ch TRANsform VNETworks GLOop EMBedding group STATe File Name 1 The ellip...

Страница 660: ...ivate differential match embedding Access Overview panel Differential Match For the 2 Port Data network type the required touchstone file can also be selected from here If necessary use the button on...

Страница 661: ...tive recall set Persisted recall sets contain the full embedding and deembedding data so that they can be trans ferred to other instruments Remote command MMEMory LOAD VNETworks Ch DIFFerential EMBedd...

Страница 662: ...ength Mech Length Defines the length offset at the selected port as a delay an electrical length or a mechanical length The three quantities are related by and overwrite each other See also Chapter 4...

Страница 663: ...Time Gate Activates the operating mode where the time gate is moved in the opposite direction when the Delay setting or any other length offset parameter is changed The button is available if a time...

Страница 664: ...uto Length corrected trace shows the devia tion from linear phase Note that in order to calculate the electrical length offset of port i you have to select a trace that uses port i as one of its recei...

Страница 665: ...ted see Auto Length and Loss vs Direct Compensation on page 205 Remote command SENSe CORRection COLLect FIXTure LMParameter STATe SENSe Ch CORRection OFFSet PhyPt DFComp STATe Prompt for Each Port Det...

Страница 666: ...ports in one go Otherwise sweeps are taken port by port For Direct Compensation it is also possible to save the acquired data to file In future measurements you can load these files instead of repeati...

Страница 667: ...Short standards Refer to the program example for SENSe Ch CORRection COLLect FIXTure ACQuire Remote command SENSe Ch CORRection COLLect FIXTure STARt SENSe Ch CORRection COLLect FIXTure ACQuire SENSe...

Страница 668: ...4 6 1 4 Auto Length and Loss on page 204 Note If Auto Length and Loss is used with a line connected to a test port the end of the line should be left open To calculate the electrical length offset and...

Страница 669: ...physical port Such a network is either defined via its S parameters stored in a two port Touchstone file s2p or by selecting a predefined lumped element model and specifying the available parameters r...

Страница 670: ...Overview on page 662 Type Switches between Deembedding and Embedding network definition Port Physical port The transformation networks are defined such that the analyzer is con nected to the left of...

Страница 671: ...ate Ch TRANsform VNETworks SENDed DEEMbedding PhyPt STATe CALCulate Ch TRANsform VNETworks SENDed DEEMbedding PhyPt TNDefinition CALCulate Ch TRANsform VNETworks SENDed DEEMbedding PhyPt PARameters C...

Страница 672: ...select and run a third party fixture modeling tool see Chap ter 4 6 2 9 Fixture modeling and deembedding on page 215 and to use its results for single ended deembedding If the selected fixture modelin...

Страница 673: ...3 User Manual 1178 6462 02 20 Figure 5 38 Fixture modeling dialog SFD Single Ended Ports Figure 5 39 Fixture modeling dialog ISD Balanced Ports Figure 5 40 Fixture modeling dialog EZD Single Ended Por...

Страница 674: ...implementation assumes symmetrical lead ins and lead outs 5 14 5 1 Common controls on the dialog The following controls are located at the bottom of each dialog ISD SFD EZD single ended balanced Outpu...

Страница 675: ...te command CALCulate FMODel ISD Ph_pt PRESet CALCulate FMODel SFD Ph_pt PRESet CALCulate FMODel EZD Ph_pt PRESet Apply This button is only active if the selected deembedding tool has been successfully...

Страница 676: ...ows all the S parameter measurements being made The resulting Touchstone file is written to C Users Public Documents Rohde Schwarz ZNA Embed ding For Coupon Type 1x Open 1x Short ISD only the measurem...

Страница 677: ...COUPon MEASure FILename CALCulate FMODel EZD Ph_pt DUT MEASure FILename CLEar Active Before starting to measure the test coupon use these checkboxes to indicate the physical ports to which it is conne...

Страница 678: ...t the Active ports The display area shows all the S parameter measurements being made The resulting Touchstone file is written to C Users Public Documents Rohde Schwarz ZNA Embed ding Remote command C...

Страница 679: ...nished successfully or the test coupon data were successfully loaded from file see Chapter 5 14 5 2 Measure Coupon on page 675 the measurement of DUT test fixture finished successfully see Chapter 5 1...

Страница 680: ...when the dialog is closed using Apply Note For a left deembedding file the original port order is used for a right de embedding files the swap gates ports function is used Remote command CALCulate FMO...

Страница 681: ...GUI reference R S ZNA 681 User Manual 1178 6462 02 20 Figure 5 43 Delta L error messages 5 14 5 6 ISD Advanced Settings For details see the ISD User Guide Offset Embed softtool...

Страница 682: ...lit and used directly for deembedding This option can be more accurate if the fixture and the 2x Thru have the same impedance at every location Remote command CALCulate FMODel ISD Ph_pt ATTenuation BE...

Страница 683: ...IMe DUT DUT With Fixture Defines how the ISD tool interprets the DUT Test Fixture data Port Sequence DUT With Fixture Tells the ISD tool about the arrangement of DUT Fixture ports 1 to N on left ports...

Страница 684: ...alculations Tells the ISD tool about coupling among lead in traces None no coupling The ISD tool optimizes odd and single ended insertion losses Weak coupling is extracted even if there are 2 ports en...

Страница 685: ...data for f 0 Remote command CALCulate FMODel ISD Ph_pt DCEXtrapolat Small Fixture Calculations Enables disables the ISD tool s small fixture mode Remote command CALCulate FMODel ISD Ph_pt SMALlfixture...

Страница 686: ...ol performs automatic impedance adjustments Remote command CALCulate FMODel SFD Ph_pt AUTO on page 955 5 14 5 8 EZD Advanced Settings Allows you to specify advanced parameters for the EZD tool Port Or...

Страница 687: ...Remote command CALCulate FMODel EZD Ph_pt IMPedance on page 942 CALCulate FMODel EZD Ph_pt IMPedance REFerence on page 943 CALCulate FMODel EZD Ph_pt IMPedance BWIDlimit on page 942 5 14 6 Port Sets t...

Страница 688: ...if any This control is only visible if Type Deembedding Network Depending on the size m of the selected port set the graphical list contains all availa ble 2m port deembedding embedding networks Note...

Страница 689: ...PAir EMBedding ListId PARameters R 1 2 3 CALCulate Ch TRANsform VNETworks PPAir EMBedding ListId PARameters G 1 2 3 D1 D2 The D1 and D2 buttons are enabled as long as the selected deembedding embed di...

Страница 690: ...ected balanced ports see Chapter 5 14 1 5 Bal anced panel on page 657 Background information Refer to the section Chapter 4 6 2 10 Combining Several de embedding networks on page 217 Figure 5 44 Offse...

Страница 691: ...0 The symbol selects no network and disables deembedding embedding for the selected balanced port The 4 port data network symbol D1 only is defined by means of an s4p file see D1 D2 For deembedding th...

Страница 692: ...struct the analyzer to assume an Increasing Port Sequence i e that the low port numbers are on the VNA side Furthermore it is possible to Swap Gates i e to instruct the analyzer to swap the VNA and DU...

Страница 693: ...eters resistance inductance or resistance capacitance Use the complementary dock widget to activate or deactivate ground loop dembedding and embedding see Chapter 5 14 1 6 Ground Loop panel on page 65...

Страница 694: ...resistances in Capacitor in parallel with resistor model to conductances and vice versa Remote command CALCulate Ch TRANsform VNETworks GLOop DEEMbedding group TNDefinition CALCulate Ch TRANsform VNE...

Страница 695: ...networks Otherwise the same de embedding network is used for all active ports The checkbox is only visible if the instrument supports multiple DUTs which is the case for 4 port R S ZNA Remote command...

Страница 696: ...cal analyzer test ports are connected to the left of the circuit the DUT ports are on the right side You can define independ ent embedding networks for all balanced ports Remote command The LogPt nume...

Страница 697: ...erential EMBedding LogPt PARameters G Cmp Select File Select File is enabled as long as the 2 Port Data network is selected This net work is defined by its S parameters stored in a two port Touchstone...

Страница 698: ...ding calculation default Otherwise the legacy S parameter based calculation is used See Chap ter 4 1 6 Data flow on page 93 Remote command CALCulate Ch TRANsform VNETworks WAVes 5 14 11 Delta L tab Th...

Страница 699: ...s you to configure a Delta L measurement The Delta L Measurement button runs the config ured measurement 5 14 11 1 Delta L Settings dialog From the Detal L Settings dialog you can set up the Delta L m...

Страница 700: ...ASurement Port Order Declares how the DUT is connected to the measurement system Choosing the wrong port ordering for your setup can result in erroneous results Sequential Ports 1 2 and 3 4 form input...

Страница 701: ...ing OK Furthermore the VNA collects data for the LPI computation a the Mini mum and Maximum frequencies in addition to the other specified Frequencies on page 700 The default Minimum is the standard D...

Страница 702: ...elta L settings and starts the Delta L measurement 5 14 11 2 Delta L measurement When the Delta L measurement starts the analyzer firmware prepares the Delta L measurement channel populates the diagra...

Страница 703: ...FILename CALCulate FMODel DELT Ph_pt M2L FILename CALCulate FMODel DELT Ph_pt M3L FILename Measure 1L Measurement 2L Measurement 3L Measurement Measures the full set of S parameters at the selected p...

Страница 704: ...Measurements If Method 1L is selected in the Delta L Settings dialog the VNA firmware builds up a cache of coupon measurements Touchstone files in the DeltaL 1LMeasurementCache subfolder of the user d...

Страница 705: ...which analysis pair it belongs and the computed Delta L uncertainty Method 1L For Method 1L the Delta L diagram displays the following traces An insertion loss trace L i for each touchstone file L i s...

Страница 706: ...L1 are added to the StdDev trace at the analysis frequencies The marker names indicate the computed Delta L uncertainties 5 15 File Print softtool The File Print softtool allows you to work with recal...

Страница 707: ...call sets tab A recall set comprises a set of diagrams together with the underlying system channel trace and display settings It can be stored to a VNA recall set file znxml znx For background informa...

Страница 708: ...use Save Remote command MEMory DEFine Open Recall Loads a recall set from a file znxml znx Opens the Open Dialog with suitable file filters Note In case the recall set could only be loaded after adjus...

Страница 709: ...ites New favorites are always created at the topmost spare position in the favorites list If the list is complete i e if there are no more spare positions new favorites can only be added after existin...

Страница 710: ...1165 HCOPy IMMediate initiates printing Print Opens a dialog that allows to define the content printer and page settings see Chap ter 5 15 4 Printer Setup dialog on page 711 To File Opens the Save Im...

Страница 711: ...all then To Clipboard is disabled 5 15 4 Printer Setup dialog The Printer Setup dialog specifies how the diagram content of and or other informa tion on the active recall set is printed Printer optio...

Страница 712: ...see Info Table Show Position on page 777 and or Setup Info see Setup Tab on page 805 is printed on additional pages 5 15 4 2 Printer tab The Printer settings select one of the installed printers and...

Страница 713: ...ia log box to select the file from all trace files s p csv dat stored on the file system see Open Dialog on page 110 The opened trace replaces the active trace Exit Persists the current state of all l...

Страница 714: ...Select the DUT Type Currently this can be either Mixer or Amplifier where Mixer measurements require option R S ZNA K4 see Chapter 4 7 3 Frequency conversion measure ments on page 234 Other DUT types...

Страница 715: ...figures the required channels for you If you have decided to proceed with calibra tion the wizards selects the suitable calibration types and guides you through the channel calibrations see Chapter 5...

Страница 716: ...n you proceed to the next step of the DUT Centric Wizard If a DUT with the same name exists you are asked whether you want to overwrite the existing DUT con figuration or to create a DUT with a differ...

Страница 717: ...20 Figure 5 46 Mixer VNA connections Figure 5 47 Amplifier VNA connections Which connections are actually possible depends on the number of analyzer ports and the number of independent sources on the...

Страница 718: ...he DUT Centric Wizard allows you to select the mixer properties to be measured Channel Settings The Channel Settings page allows you to change the measurement channel configu ration proposed by the DU...

Страница 719: ...page of the DUT Centric Wizard summarizes the channels to be created and the parameters to be measured allows you where to define these channels new setup append to current setup replace current setu...

Страница 720: ...specify basic properties of an amplifier or load them from the DUT Manager Similar to the Amplifier Configuration dialog of the DUT Manager Load from DUT Manager Use Load from DUT Manager to load a pr...

Страница 721: ...n you proceed to the next step of the DUT Centric Wizard If a DUT with the same name exists you are asked whether you want to overwrite the existing DUT con figuration or to create a DUT with a differ...

Страница 722: ...20 Figure 5 48 Mixer VNA connections Figure 5 49 Amplifier VNA connections Which connections are actually possible depends on the number of analyzer ports and the number of independent sources on the...

Страница 723: ...o be measured Channel Settings The Channel Settings page allows you to change the measurement channel configu ration proposed by the DUT Centric Wizard based on the properties of the DUT the RF topolo...

Страница 724: ...they will mea sure allows you where to define these channels new setup append to current setup replace current setup allows you to decide whether to proceed with a suitable calibration Calibrate Newly...

Страница 725: ...om the DUT Centric Wizard the filter is set to the DUT type that is being configured Edit This button is only active if exactly one row is selected in the table area It allows you to edit the selected...

Страница 726: ...you to delete the selected DUTs Export DUTs Import DUTs Export DUTs allows you to save the currently configured DUTs to a file Import DUTs allows you to import a set of user defined DUTs either in ad...

Страница 727: ...uration dialog The Mixer Configuration dialog allows you to set up the fundamental properties of a mixer Access DUT Manager dialog Edit with a DUT of type Mixer selected The DUT type Mixer is only ava...

Страница 728: ...elay measurements requires option R S ZNA K9 Use Fixed Freq Uncheck Use Fixed Freq if you want the LO signal to vary in frequency which will enable LO Freq Min and LO Freq Max Otherwise only LO Fixed...

Страница 729: ...ined external tools Access System Applic External Tools GPIB Explorer Opens a tool that allows you to connect to the analyzer obtain an overview of all imple mented remote control programs test progra...

Страница 730: ...oard This tool allows you to enter characters in particular letters if an input field cannot call up the analyzer s own on screen key board and if no external keyboard is connected See also Chapter 3...

Страница 731: ...up tab allows you to set up the measurement for the time domain trans formation which is then used to analyze the time domain behavior of the DUT eye diagram rise time skew Access System Applic TDR TD...

Страница 732: ...apter 5 11 1 Start Cal tab on page 515 Stimulus The Stimulus section provides quick access to settings that are also available in the TDR Stimulus Settings dialog These settings are only available in...

Страница 733: ...ons are the same as the ones offered on the Predefined Config tab of the Balanced Ports dialog Step 2 Stimulus Settings The Stimulus Settings step gives access to the basic settings of the TDR Stimulu...

Страница 734: ...ings dialog This dialog allows you to configure the frequency sweep whose results are then used for the time domain transformation and subsequent TDR simulation Access System Applic TDR TDR Setup Stim...

Страница 735: ...specified as delay electrical length or mechanical length Given the DUT s permittivity r these alternative length parameters are related by i e setting one of them determines the other two Note The le...

Страница 736: ...Time Definition Selects between rise time definitions 10 90 default and 20 80 Remote command SENSe Ch HARMonic RTIMe THReshold Rise Time The minimum rise time according to the selected Rise Time Defi...

Страница 737: ...re down to 0 Hz These parameters are the same settings as for the standard time domain option R S ZNA K2 For a description and related remote control commands see DC Value on page 407 5 17 2 4 Eye Dia...

Страница 738: ...mprises two separate info fields for Basic and Time results see Measurements on page 738 By default they are stacked below each other however they can be moved independ ently like any other info field...

Страница 739: ...on page 230 Furthermore it is possible to change the Rise and Fall Time definition for the eye measurement from the standard 10 90 90 10 step to any other Start Value Stop Value pair Remote command C...

Страница 740: ...select an S Parameter i e the transmission or reflec tion whose time domain properties shall be analyzed The Topology to the right is updated accordingly Remote command CALCulate Ch PARameter MEASure...

Страница 741: ...bit ASCII compatible text file the Byte Order Mark BOM that is common with UTF encodings is not allowed Only the ASCII codes 0x30 0 and 0x31 1 will be interpreted all other codes including whitespace...

Страница 742: ...a more realistic shape Low Pass enabled If enabled a single pole low pass filter is inserted into the simulated signal path which is defined using its rise time see Rise Time Rise Time Definition on p...

Страница 743: ...lic TDR Eye Diag Advanced Settings This dialog is available with Extended time domain analysis option R S ZNA K20 only Controls in the dialog Generator Emphasis Jitter and Noise allow you to specify t...

Страница 744: ...ettings dialog This dialog is available with Extended time domain analysis option R S ZNA K20 only Simulated Bit Pattern Simulated Rise Time see Chapter 5 17 2 5 Eye Diagram dialog on page 740 Modulat...

Страница 745: ...es since the channels show typically larger losses for high fre quencies This type of equalization is done using finite impulse response FIR filters using between 2 and 4 taps The filter s sampling ti...

Страница 746: ...ive value in dB for increasing the amplitude of the first bit after a transition Below an example for Post 1 Cursor 3dB The FIR filter coefficients are found by solving the equations c 1 c0 c1 c2 Va c...

Страница 747: ...only Four types of jitter can be configured and selectively enabled random periodic Dirac and user defined If enabled the jitter is added at the start of each symbol period and its magnitude depends o...

Страница 748: ...tter is specified by the amplitude in seconds Dirac Delta positive or negative as well as the probability of the jitter occurring at each symbol period Dirac Probability Remote command CALCulate Chn E...

Страница 749: ...y LOAD EYE JITTer Noise The Define Noise dialog allows you to add Gaussian noise to the digital signal simu lated for the eye diagram Access Noise button in the Advanced Settings dialog This dialog is...

Страница 750: ...Mode Allows you to switch temporarily between the real DUT with measured frequency response and an ideal one with flat frequency response Remote command CALCulate Chn EYE DUT MODE Equalization The Def...

Страница 751: ...specify the equalizer s frequency response using a gain factor plus poles and zeros in the frequency domain Active Enables disables equalization Remote command CALCulate Chn EYE EQUalization STATe CTL...

Страница 752: ...her than NRZ display of eye mask and eye mask test is not available i e the buttons in the Eye Mask Test tab are grayed out Define Mask Opens the Define Mask Configuration dialog Show Mask This checkb...

Страница 753: ...ote The R S ZNA does not have a built in audio device and loudspeaker To hear these sounds connect a USB audio device to the instrument or operate it via remote desktop Remote command CALCulate Chn EY...

Страница 754: ...eye diagram test center poly gon top rectangle and bottom rectangle Testing against these mask areas can be selectively enabled Polygon Setup Allows you to define the center polygon and to activate i...

Страница 755: ...ter and also their vertical off sets are specified relative to this point Remote command CALCulate Chn EYE MASK SHAPe TOP STATe CALCulate Chn EYE MASK SHAPe TOP HORizontal CALCulate Chn EYE MASK SHAPe...

Страница 756: ...shown below Mask Top Active true Width Top 9e 009 Offset Top 6 Mask Bottom Active true Width Bottom 9e 009 Offset Bottom 6 Mask Polygon Active true Shape Octagon Main Width 9e 009 Minor Width 8e 009 M...

Страница 757: ...turn requires the stimulus grid to be harmonic If the rise time measurement cannot be enabled a message is displayed Instead of performing the required configuration changes manually you may also use...

Страница 758: ...asurement can only be performed if the following conditions are met for both the active trace and the reference trace the trace format is Real Time Domain is enabled the Low Pass Step time domain tran...

Страница 759: ...ALCulate Chn DTIMe POSition Extended Info Defines whether the info field for the skew measurement shall only provide basic infor mation default or extended information Figure 5 59 Skew info basic vs e...

Страница 760: ...ofttool see Chapter 5 5 6 Time Gate tab on page 407 Access System Applic TDR Time Gate Since for the eye diagram the time axis always equals two times the symbol time the display of the time gate rang...

Страница 761: ...ust the center span or start stop no matter if the X axis rep resents Time or Distance see Time Distance on page 484 5 17 3 Distance to Fault application With option K2 the R S ZNA can locate faults a...

Страница 762: ...63 3 Adjust the number of sweep points and if necessary the frequency span see Auto Number of Points 4 Select or define and select a suitable cable type see Cable Type on page 764 5 Perform a full one...

Страница 763: ...Define the distance window for the Distance to Fault measurement Start Distance and Stop Distance correspond to the left and right edge of the diagram area and are displayed in the Distance Domain tra...

Страница 764: ...ep points would be higher than 100001 the maximum number of sweep points of the R S ZNA the analyzer sets N to 100001 and displays the following message box OK reduces the frequency span f to f v c0 1...

Страница 765: ...he peak response value for total reflection after proper calibration Note that the fault limit is handled like any other limit line When Fault Limit Check is enabled the current Fault Limit forms a ne...

Страница 766: ...y factor of 1 and zero attenuation and a wide range of standard cable types Cable types are defined by their relative permittivity r or equivalently by their velocity factor 1 sqrt r and a frequency d...

Страница 767: ...y list If the cable attenuation is specified at several frequency points the R S ZNA cal culates the Attenuation fcenter by linear interpolation If fcenter is below above the specified frequency range...

Страница 768: ...peaks that violate the active Fault Limit Unless tracking is dis abled see Tracking Enabled on page 769 the table is updated with every sweep Remote command CALCulate Chn TRANsform DTFault PEAK COUNt...

Страница 769: ...mm 8 56 dB 2 Trc1 1 539 m 9 24 dB For an explanation of the possible filters see Contents on page 768 Tracking Enabled If checked default the Fault Table on page 768 is cleared at every sweep start a...

Страница 770: ...gram Each recall set screen can display several diagrams simultaneously each with a vari able number of traces One of these diagrams and traces is active at each time The diagram number or name in the...

Страница 771: ...guous numbers Delete Diag Area is disabled if the recall set contains only one diagram area In manual control each recall set must contain at least one diagram area with one channel and one trace Tip...

Страница 772: ...of the trace format and the channel set tings it is even possible to overlay Cartesian and complex diagrams The active trace and active channel is highlighted The scaling of the axes corresponds to t...

Страница 773: ...s drag and drop the separating frames or use the functions in the Split tab Remote command DISPlay LAYout SPLit 5 18 2 Split tab Arranges multiple diagrams on the screen Some of the Split settings are...

Страница 774: ...of the diagrams drag and drop the separating frames or use the functions in the Diagram tab Remote command No command display configuration only Split Type The R S ZNA provides the following split typ...

Страница 775: ...r than Rows Cols only the total number of Diagrams can be specified Remote command DISPlay LAYout GRID Additional Functionality SCPI Commands The analyzer provides remote control commands for efficien...

Страница 776: ...ng results on the analyzer screen Light Background sets a light background color The traces and information ele ments in the diagram areas are displayed in different colors This setting is suitable fo...

Страница 777: ...he next time someone tries to uncheck Hide Sensitive Information to unmask all stimulus values for this recall set Both checked state and password are stored in and loaded from the active recall set R...

Страница 778: ...f checked default the relative position of the application window and the control win dow is locked if you drag one of them then both windows move together Single Window Mode If enabled default the co...

Страница 779: ...element See DISPlay CMAP DispEl RGB Properties Configures the selected screen element Color opens a standard color dialog where you can assign a color to the selected element Trace Line Style and Trac...

Страница 780: ...r Diagram Controls the color of traces that are created in a diagram or moved to another diagram If Trace Colors per Diagram is enabled factory preset all diagrams use the same trace colors The first...

Страница 781: ...are non editable files with the extension ColorScheme the default directory is C Users Public Documents Rohde Schwarz ZNA ColorSchemes Remote command MMEMory STORe CMAP MMEMory LOAD CMAP 5 18 3 3 Inf...

Страница 782: ...info field whose contents you want to display in parts or in total It contains all info fields of the current recall set that are currently not displayed in the info table see Info Table Show Posi ti...

Страница 783: ...er interface Hiding the information elements leaves more space for the diagrams All elements can be shown or hidden simultaneously Menu Bar Toggles the visibility of the Menu Bar For background inform...

Страница 784: ...3 3 2 1 Title bar on page 53 Remote command SYSTem DISPlay BAR TITLe STATe Tool Bar Toggles the visibility of the Tool Bar For background information see Chapter 3 3 2 2 Toolbar on page 53 Remote com...

Страница 785: ...xecute service functions Access System Setup Persistent vs session settings The settings in the Setup softtool and the configuration dialogs are global settings and not affected by a Preset or shutdow...

Страница 786: ...m the Rohde Schwarz Internet site Remote command n a Remote Encoding Selects the character encoding used at the remote interface The selected encoding applies to directory and file names calibration k...

Страница 787: ...t Scope applies to the GUI and to the SYSTem PRESet DUMMy com mand The RST command always resets all open recall sets Remote command SYSTem PRESet SCOPe Remote Preset Configuration Align RST to User D...

Страница 788: ...ted or moved System Preset ini tiates a factory preset The preset configuration applies to the System Preset hardkey if Immediate Preset on page 843 is selected the Preset button the remote commands R...

Страница 789: ...tion STATe Auto Averaging Activates automatic averaging which means that the VNA performs multiple calibration sweeps and applies averaging to reduce trace noise In contrast to regular averaging see C...

Страница 790: ...evice under test this calibration method is potentially more accurate than the previous method If the sweeps are relatively slow or if many ports are calibrated it causes longer calibration times Remo...

Страница 791: ...on whenever possible Remote command SENSe CORRection IMEThod on page 1272 User Interface tab Provides general user interface configurations Sounds Switches the sounds generated by the analyzer GUI on...

Страница 792: ...ion see Chapter 5 1 Function keys and softtools on page 298 Remote command n a Conductance in Embedding Networks Changes the presentation of capacitance C i in parallel with resistance R i circuit blo...

Страница 793: ...it Hz to kilo k mega M giga G or tera T or lets the R S ZNA select the appropriate prefix Auto default setting Remote command n a Reset Colors Reset Dialogs Reset Decimal Places Reset Units Prefix Res...

Страница 794: ...er information popups are displayed whenever a remote control com mand error occurs The displayed information can be useful for program development and optimization it does not necessarily indicate th...

Страница 795: ...tput signal at pin 8 The signal is switched on while a measure ment sweep is running in the selected channel All other signals are inactive 2 enables the output signal at pin 9 3 enables the output si...

Страница 796: ...l of detail Minimal Normal Large Full and hence the size of the dump files that are created if a firmware exception occurs None disables dump file creation The latest 5 exception dumps can be retrieve...

Страница 797: ...hitespace Touchstone Export Options If checked whitespace at the beginning of each line is removed Remote command MMEMory STORe TRACe OPTion TRIM Positive Number Prefix Touchstone Export Options Posit...

Страница 798: ...ep mode power sweep Note By default at sweep end the output power of the first measurement point is restored Auto mode After a small settling time 10 ms the sweep can be restar ted Selecting a differe...

Страница 799: ...ming a Time or CW Mode sweep In triggered mode the analyzer always uses the settings of the first measurement point while waiting for the trigger signal Remote command SOURce POWer SWEepend MODE Settl...

Страница 800: ...two AGC algorithms Memory AGC factory default Optimized gain control pre measurement only if required and for as few sweep points as possible Point AGC Legacy AGC algorithm gain control pre measureme...

Страница 801: ...service The Status LED indicates the current state of the HUMS service grey inactive green up and runnning If HUMS has been used before turning on restores the previous protocol settings Remote comman...

Страница 802: ...c HUMS DELete ALL on page 1549 Protocol subtab Gives access to SNMP configuration options for protocol version 2c and 3 SNMPv2c Configuration Allows you to grant read or read write access via SNMPv2c...

Страница 803: ...ALL Device Tags subtab Allows you to manage key value pairs for HUMS device tagging A device tag is a label to assign to your instrument You can create any device tag for your instrument and define it...

Страница 804: ...ay softkeys for printing the contents or saving them to a file Access System Setup Setup Info Common controls in the Info dialog The Save Print and Save Report buttons at the bottom of the Info dialog...

Страница 805: ...le you can send to R S Support for fault diagnosis see Chapter 9 3 Collecting information for technical support on page 1634 Remote command DIAGnostic DEVice STATe SYSTem DFPRint Setup Tab Displays th...

Страница 806: ...oftware and hardware options You can also enable additional software options using the option key supplied with the option Proceed according to the instructions in the dialog For an overview of option...

Страница 807: ...sted with their name and description the option key and key type and the activation and expiration date if applicable Remote command DIAGnostic PRODuct OPTion INFO Hardware Tab Gives an overview of th...

Страница 808: ...ed test results Error Log Tab Contains a chronological record of errors that occurred in the current and in previous sessions see Chapter 9 1 Errors during firmware operation on page 1632 The Error ta...

Страница 809: ...s the end time of the related sweep Reload Lost Trigger Log While the Lost Trigger tab is open the display is not refreshed automatically Use Reload Lost Trigger Log to reload the lost trigger log fil...

Страница 810: ...nded Calibration Interval Queries the recommended calibration interval set by Rohde Schwarz service Remote command DIAGnostic SERVice CALibration INTerval Last Service The last date and time the instr...

Страница 811: ...to activate the required service level Remote command SYSTem PASSword CENable Service Function Identifier of the service function in dotted textual example sw common mem ory_usage or dotted decimal ex...

Страница 812: ...ernal reference signal has to meet the specifications of the data sheet its frequency must be specified in the Ext Frequency field The synchronized internal 10 MHz reference clock is also made avail a...

Страница 813: ...gnal is output to the Reference In Out SMA connector Ineffective and disabled if this connector is used as reference clock source Remote command SENSe ROSCillator SMA OUTPut Reference I O SMA In Speci...

Страница 814: ...SUPPress Remote Language Selects the syntax of the R S ZNA s instrument control commands The DEFAULT language corresponds to the commands reported in this documen tation see Chapter 7 3 SCPI command r...

Страница 815: ...software and hardware options The bit order for transferred binary data is swapped FORMat BORDer SWAPped If the PNA language is activated Keysight compatible ID and option strings are set The bit orde...

Страница 816: ...em COMMunicate GPIB SELF LPORt ALIGn SYSTem COMMunicate GPIB SELF DCLear SUPPress Instrument Messages Opens the System Configuration dialog with the Messages tab selected From there you can configure...

Страница 817: ...line physically connected switched on ready to be used Log Errors Enables the transfer of error messages for external devices e g connection errors to the error log The error log appears in the Info d...

Страница 818: ...erface type and address The R S ZNA can auto detect the instrument type driver and the serial number of the connected power meter To control external devices via GPIB the pre installed RS Visa library...

Страница 819: ...sed There is a problem with the device This state can be caused by different problems General communication error In this case check whether the device is properly connected to the configured interfac...

Страница 820: ...ce supported by the installed VISA library GPIB VXI 11 and SOCKET is applicable for devices connected to the GPIB Bus or LAN connectors on the rear panel of the analyzer respectively Raw Serial is use...

Страница 821: ...12 13 50000 LAN connection with pure TCP IP protocol refer to your VISA user documentation GPIB GPIB0 GPIB9 Address for example 20 Full VISA resource string GPIB board Address INSTR USB Raw Serial PM...

Страница 822: ...z com appnotes 1GP70 Remote command SYSTem COMMunicate RDEVice PMETer Pmtr SPCorrection STATe 5 19 5 Generator tab Allows you to set up and configure external generators and to enable error logging fo...

Страница 823: ...they can be used for measurements and power calibrations Access System Setup Generator Generators Background information Refer to section Chapter 4 7 39 External generators on page 290 The configurati...

Страница 824: ...e here Ext Ref switches the generator to either external box checked or internal fre quency reference The setting does not affect the frequency reference settings of the analyzer System Setup Freq Ref...

Страница 825: ...switchover to remote control When using the NI VISA library ensure that the network analyzer itself is not listed as a network device in the Measurement Automation Explorer Otherwise Scan Instru ments...

Страница 826: ...cted to the R S ZNA the entries in the Driver for New Instrument panel can be auto detected for the specified interface type and address Identify sends an identification query IDN to the specified dev...

Страница 827: ...the Frequency Converter tab open the following dialogs Frequency Converter opens a dialog that allows you to define the Converter Topology i e which converters are used an how they are connected to t...

Страница 828: ...r Frequency Converter Converter Type Selects the type of the converter that is connected to the related VNA port None No converter connected to this port ZCxxx Serial If a converter R S ZCxxx is conne...

Страница 829: ...er Port RFPort Local Defines the source for the LO In port of the converter In general if two or more converters are used in a measurement it is recommended to distribute a single LO signal to all con...

Страница 830: ...ble LO source here and set Local to Splitter i Splitter 2 is only useful if 2 independent 2 port converter measurements shall be per formed If compatible with the overall converter setup the source is...

Страница 831: ...currently defined pre defined and user defined Remote command SENSe CONVerter DEFinition CATalog SENSe CONVerter DEFinition COUNt Type The name of the converter type Remote command SENSe CONVerter DE...

Страница 832: ...Verter DEFinition LPOWer Add Copy Use Add to create a converter type with default properties or use Copy to duplicate the properties of the converter type whose tab is currently selected Remote comman...

Страница 833: ...setups or after a Preset these values are written to the Slope of the respective ports Remote command SENSe CONVerter Port CSSLope Apply to all channels in all setups Use Apply to all channels in all...

Страница 834: ...ection SLEVeling STATe Available Shows the availability of a leveling data set Remote command SOURce POWer PhyPt CORRection SLEVeling STATe Load Save Use Save to persist the leveling dataset of the re...

Страница 835: ...Port Settings dialog Combiner Tells the analyzer if and how signals from two ports are combined For descriptions and visualizations of the supported combiner configurations see Combiner Configuration...

Страница 836: ...not natively supported by the analyzer firmware Background information Refer to Chapter 4 7 40 Generic devices on page 292 1 Use Generic Device to open the External Generic Devices dialog which allows...

Страница 837: ...ect to copy the device to the list of configured devices If the R S ZNA fails to detect a connected external device click Add Device to define the interface type and address manually To control extern...

Страница 838: ...n Devices on the supported interfaces Note Unintentional switchover to remote control When using the NI VISA library ensure that the network analyzer itself is not listed as a network device in the Me...

Страница 839: ...to 30 GPIB and IP addresses must agree with the entries in the VISA library The remaining interface types require composite address formats see Table 5 11 Identify sends an identification query IDN t...

Страница 840: ...onfigured generic devices during channel initialization Access System Setup Generic Device Generic Device Config If the previously measured channel uses the same command to control a generic device th...

Страница 841: ...assignment for all devices Remote command SENSe Ch GDEVice DELete 5 20 Help softtool The Help softtool presents the functions of the menu bar s Help menu except call ing context sensitive help Access...

Страница 842: ...softtool Access System Preset Menu bar Channel Preset Select Preset various menu items 5 21 1 Select Preset tab The controls on the Select Preset tab allow you to customize the function of the Sys te...

Страница 843: ...lors Reset Dia logs Reset Decimal Places Reset Units Prefix reset RF connec tion configuration between VNA and external devices Currently the latter only resets the frequency converter configuration F...

Страница 844: ...ne go Opens a standard Save File dialog Remote command MMEMory STORe STATe 1 RecallSetFile SYSTem PRESet USER NAME RecallSetFile Select Preset File Lets you select a recall set file to be used as user...

Страница 845: ...S ZNA offers a GPIB bus interface according to standard IEC 625 1 IEEE 488 1 The GPIB bus connector is on the rear panel of the instrument see Chap ter 3 2 Instrument tour on page 39 The R S ZNA can b...

Страница 846: ...2 92 mm connectors 0x019A 4 port R S ZNA43 with 2 92 mm connectors 0x019B 2 port R S ZNA43 with 2 4 mm connectors 0x023F 4 port R S ZNA43 with 2 4 mm connectors 0x0240 2 port R S ZNB50 0x0251 4 port...

Страница 847: ...ew LabWindows CVI IVI VXIplug play for different programming languages The drivers are available free of charge on the product pages in the R S internet along with instal lation information 6 1 3 GPIB...

Страница 848: ...nded for remote test on the instrument EB200 currently not supported Select SETUP Setup Info to look up the IP address information of your ana lyzer If you run the GPIB explorer on the analyzer the lo...

Страница 849: ...display is switched off If the Remote softtool is not visible tap click on the main window to restore it The softkeys in the remote screen are used to modify or quit the remote state Go to Local switc...

Страница 850: ...ted when triggered by the remote control command SYSTem DISPlay UPDate ONCE The instrument remains in the remote state until it is reset to the manual state via the GUI or via remote control see Chapt...

Страница 851: ...disabled e g by the Local Lockout Message via GPIB LLO see Chapter 12 3 3 2 Interface mes sages on page 1648 The lockout prevents unintentional switch over i e return to manual control is possible via...

Страница 852: ...the instrument Setting commands cause instrument settings such as a reset of the instrument or setting the output level to some value Queries cause data to be provided for output on the GPIB bus e g...

Страница 853: ...utomati cally adapted Therefore GPIB bus control programs should always define an initial device status e g with the command RST and then implement the required settings 6 2 2 1 Common commands Common...

Страница 854: ...s the trigger source external trigger The following command has the same effect TRIGger SOURce EXTernal Note The short form is marked by uppercase letters the long form corresponds to the complete wor...

Страница 855: ...n When abbreviating the command line the second command begins with the level below TRIG The colon after the semicolon is omitted The abbreviated form of the command line reads as follows TRIG SOUR EX...

Страница 856: ...ermissible K kilo M milli U micro and N nano If the unit is missing the default unit is used Example SOUR RFG FREQ 1 5GHz is equivalent to SOUR RFG FREQ 1 5E9 Special numeric values The texts MINimum...

Страница 857: ...ngs Strings must always be entered within single or double quotation marks or Example CONFigure CHANnel NAME Channel 4 or CONFigure CHANnel NAME Channel 4 6 2 3 5 Block data format Block data is a tra...

Страница 858: ...manual control this generally means that the control elements are easy to find and intuitive to handle and that the effect of each operation is easy to verify on the screen Convenient remote control...

Страница 859: ...exactly one active trace irrespective of the number of channels and traces defined The active channel contains the active trace see Chap ter 4 1 3 1 Trace settings on page 86 In remote control each c...

Страница 860: ...e active trace in channel 1 In the SCPI command description the numeric suffix Ch is used for channel settings it denotes the configured channel whereas Chn is used for trace settings it refers to the...

Страница 861: ...6 1 Addressing channels Method Commands Example Channel number Ch as a numeric suffix CONFigure CHANnel Ch STATe ON Query all channel names CONFigure CHANnel CATalog returns the names of all channels...

Страница 862: ...for a trace referenced by its trace name CONFigure TRACe Trc CHANnel NAME ID ABCD returns the actual channel number for trace ABCD the trace suffix is ignored 6 4 Command processing The block diagram...

Страница 863: ...tings Subsequently it is immediately prepared to process further commands This means that new commands can already be serviced while the hardware is still being set overlapping execution 6 4 3 Data ba...

Страница 864: ...o data is sent on the GPIB bus or via the Ethernet the controller waits until it has reached its time limit This behavior is specified by SCPI 6 4 6 Command sequence and command synchronization IEEE 4...

Страница 865: ...te DUMMy takes longer to execute than OPC sending the command sequence INIT OPC results in initiating a sweep and after some time setting the OPC bit in the ESR Sending the commands INIT OPC CLS still...

Страница 866: ...erarchy of status registers As shown in section Overview of status registers the status information is of hierarchi cal structure STB SRE The STatus Byte STB register and its associated mask register...

Страница 867: ...mit trace no 11 Limit trace no 10 Limit trace no 9 Limit trace no 8 Limit trace no 7 Limit trace no 6 Limit trace no 5 Limit trace no 4 Limit trace no 3 Limit trace no 2 Limit trace no 1 LIMit2 summar...

Страница 868: ...n The CONDition part is permanently overwritten by the hardware or the sum bit of the next lower register Its contents always reflect the current instrument state This register part can only be read b...

Страница 869: ...to the sum bit ENAB bit 1 If the associated EVENT bit is 1 the sum bit is set to 1 as well This part can be overwritten and read by the user at will Its contents are not affected by reading The sum bi...

Страница 870: ...n error can be recognized and further pinned down by polling the error queue The poll provides an informative error message 3 QUEStionable status summary bit This bit is set if an EVENt bit is set in...

Страница 871: ...gister on a bit by bit basis The ESR corresponds to the CONDition part of a SCPI register indicating the cur rent instrument state although reading is destructive The ESE corresponds to the ENABle par...

Страница 872: ...able ESE register can be set using the command ESE and read using ESE See also Common commands 6 5 3 4 STATus OPERation The STATus OPERation register contains conditions which are part of the instrume...

Страница 873: ...the limit check 2 Failed limit check for trace no 16 This bit is set if any point on trace no 16 fails the limit check Numbering of traces The traces numbers 1 to 16 are assigned as follows Traces as...

Страница 874: ...ency and level of the supplied reference signal 2 Output power unleveled This bit is set if the level control at one of the ports is unsettled or unstable possibly due to an external disturbing signal...

Страница 875: ...weep are too close so that the analyzer can not process the measurement data until the next sweep point starts Increase stop time reduce no of points increase IF bandwidth If possible reduce number of...

Страница 876: ...Set bit 5 in the SRE ESB 3 Insert OPC in the command sequence e g at the end of a sweep When all commands preceding OPC have been completed the instrument generates an SRQ Example Generate an SRQ whe...

Страница 877: ...ents are simultaneously requested by the controller with a single command to transmit 1 bit of information each on the data lines i e to set the data line allocated to each instrument to a logical 0 o...

Страница 878: ...ntries of the error queue are detailed plain text error messages that can be queried via remote con trol using SYSTem ERRor NEXT or SYSTem ERRor ALL Each call of SYSTem ERRor NEXT provides one entry f...

Страница 879: ...ENABle parts of all OPERation and QUESTiona ble registers Fill ENABle parts of all other registers with 1 yes yes Fill PTRansition parts with 1 Clear NTRansition parts yes yes Clear error queue yes ye...

Страница 880: ...of the R S ZNA features but do not need R S ZVR com patibility you should use the commands listed in Chapter 7 3 SCPI command refer ence on page 883 7 1 Special terms and notation This section explai...

Страница 881: ...ded zero or more times 7 1 3 Parameters Many commands are supplemented by a parameter or a list of parameters Parame ters either provide alternative options setting a or setting b or setting c see spe...

Страница 882: ...atus byte STB the standard event register ESR and the EVENt part of the QUEStionable and the OPERation register to zero The command does not alter the mask and transition parts of the registers It cle...

Страница 883: ...contents of the power on status clear flag The response can be 0 or 1 RST Reset no query Sets the instrument to a defined default status The command is equivalent to SYSTem PRESet DUMMy The RST value...

Страница 884: ...a global composite limit check on several traces has failed Since V2 20 of the R S ZNA FW the result is automatically recalculated whenever a relevant setting is changed i e a subsequent query will re...

Страница 885: ...er port no Value RST 0 2 LOWer 0 2 UPPer Default unit dB Manual operation See Validation Limits on page 557 CALCulate CALValidate RESPonse PHASe LOWer Port Value CALCulate CALValidate RESPonse PHASe U...

Страница 886: ...89 CALCulate Ch DATA CHANnel ALL 889 CALCulate Ch DATA CHANnel DALL 889 CALCulate Ch DATA MDATa INTerpolate 890 CALCulate Ch DATA SGRoup 890 CALCulate Chn DATA 890 CALCulate Chn DATA NSWeep LAST 893 C...

Страница 887: ...atted trace data SDATa can only be imported to memory traces Formatted trace data FDATa can only be imported to live traces if the related channel is in single sweep mode INITiate Ch CONTinuous OFF Be...

Страница 888: ...ny request for new data from the hardware Restart Sweep in single sweep mode or switching to continuous sweep mode discards imported data and switches back the display to measured data Suffix Ch Chann...

Страница 889: ...el number Query parameters Format FDATa SDATa MDATa Output format for the S parameter data see CALCulate Chn DATA Return values Data Trace data either in ASCII or block data format depending on the cu...

Страница 890: ...response values of the selected channel s active trace or reads error terms of the selected channel The set command either imports formatted or unformatted trace data to the selected channel s active...

Страница 891: ...a separated ASCII values are returned CALC2 PAR SDEF Trc2 S11 Create a second trace in a new channel no 2 The second trace does not become the active trace and is not displayed CALC DATA TRAC Trc2 FDA...

Страница 892: ...l output val ues of the A D converter acquired at a fixed sampling rate The number of val ues in the returned array is not correlated with the number of sweep points NCData Factory calibrated trace da...

Страница 893: ...DATA NSWeep LAST Format RvCount Reads the response values of a trace acquired in single sweep mode INITiate Ch CONTinuous OFF The trace can be any of the traces acquired dur ing the single sweep cycl...

Страница 894: ...n be any of the traces acquired during the single sweep cycle Tip This command can only be used for SENSe Ch SWEep COUNt 1 Suffix Chn Channel number used to identify the active trace Example See CALCu...

Страница 895: ...INIT Activate single sweep mode and start a single sweep sequence in channel no 1 No synchronization is necessary if CALC DATA NSW COUN 4 CALC DATA NSW FIRS SDAT 5 Wait until 5 sweeps have been measu...

Страница 896: ...ameters Boolean ON OFF horizontal line on or off RST OFF Example See CALCulate Chn DLINe Manual operation See Show Horiz Line on page 450 7 3 1 5 CALCulate DTIMe Defines the properties and retrieves t...

Страница 897: ...oint on current trace Y value of ref point on current trace X value of ref point on reference trace Y value of ref point on reference trace Stimulus axis distance skew distance skew distance skew time...

Страница 898: ...Chn DTIMe LIMit STATe Boolean Activates deactivates the skew limit check Use CALCulate Chn DTIMe LIMit LIMit to set the applicable limit Suffix Chn Channel number used to identify the active trace Pa...

Страница 899: ...ects the reference trace for the skew measurement See CALCulate Chn DTIMe STATe for conditions on both the active and the refer ence trace Suffix Chn Channel number used to identify the active trace P...

Страница 900: ...K DATA 912 CALCulate Chn EYE MASK FAIL 913 CALCulate Chn EYE MASK FAIL BEEP 914 CALCulate Chn EYE MASK FAIL CONDition 914 CALCulate Chn EYE MASK SHAPe BOTTom STATe 915 CALCulate Chn EYE MASK SHAPe POL...

Страница 901: ...ve trace in the selected channel is not an eye diagram Emphasis in the generator simulation for eye diagram measurements is enabled using CALCulate Chn EYE EMPHasis STATe Suffix Chn Channel number use...

Страница 902: ...n CTLE DC DC Gain Specifies the DC gain of the CTLE a two pole filter with single zero at the receiver simulation of the related eye diagram This command will raise an execution error if the active tr...

Страница 903: ...ed using CALCulate Chn EYE EQUalization STATe Suffix Chn Channel number used to identify the active trace Parameters CTLE Zero Default unit Hz Options R S ZNA K20 Manual operation See CTLE Equalizer o...

Страница 904: ...Options R S ZNA K20 Manual operation See Bit Stream on page 740 CALCulate Chn EYE INPut DRATe DataRate Defines the data trate of the bit stream generator for the related eye diagram This command will...

Страница 905: ...e trace in the selected channel is not an eye diagram Before executing this command the pattern type must be set to PRBS see CALCulate Chn EYE INPut BPATtern TYPE Suffix Chn Channel number used to ide...

Страница 906: ...is command will raise an execution error if the active trace in the selected channel is not an eye diagram The low pass is enabled using CALCulate Chn EYE STIMulus LOWPass The rise time definition can...

Страница 907: ...using CALCulate Chn EYE JITTer TYPE PERiodic Suffix Chn Channel number used to identify the active trace Parameters DiracDelta RST 1 ns Default unit s Options R S ZNA K20 Manual operation See Dirac on...

Страница 908: ...agnitude Defines the magnitude of the periodic jitter in the generator simulation of the relaed eye diagram This command will raise an execution error if the active trace in the selected channel is no...

Страница 909: ...d to identify the active trace Parameters StdDeviation Default unit s Options R S ZNA K20 Manual operation See Random on page 747 CALCulate Chn EYE JITTer STATe Boolean Activates the jitter functional...

Страница 910: ...tify the active trace Parameters Boolean RST OFF Options R S ZNA K20 Manual operation See Dirac on page 748 CALCulate Chn EYE JITTer TYPE PERiodic Boolean Enables disables periodic jitter insertion in...

Страница 911: ...7 CALCulate Chn EYE JITTer TYPE USER Boolean Enables disables user defined jitter insertion in the generator simulation of the related eye diagram This command will raise an execution error if the act...

Страница 912: ...er of the eye mask in the related eye diagram This command will raise an execution error if the active trace in the selected channel is not an eye diagram Suffix Chn Channel number used to identify th...

Страница 913: ...CALCulate Chn EYE MASK FAIL Returns Pass or Fail to indicate the result of the limit check in the related eye diagram This command will raise an execution error if the active trace in the selected ch...

Страница 914: ...e Chn EYE MASK FAIL CONDition FailCondition Defines whether the fail condition for the eye mask test of the related eye diagram is specified in absolute or relative terms This command will raise an ex...

Страница 915: ...le in the eye mask of the related eye dia gram This command will raise an execution error if the active trace in the selected channel is not an eye diagram Use CALCulate Chn EYE MASK SHAPe BOTTom STAT...

Страница 916: ...te the area in the eye mask The eye mask test is enabled disabled using CALCulate Chn EYE MASK STATe Suffix Chn Channel number used to identify the active trace Parameters PolygonType OCTogon HEXagon...

Страница 917: ...STATe Suffix Chn Channel number used to identify the active trace Parameters Main Default unit V Minor Default unit V Options R S ZNA K20 Manual operation See Polygon Setup on page 754 CALCulate Chn E...

Страница 918: ...onRate Default unit percent Options R S ZNA K20 Manual operation See Test Settings on page 756 CALCulate Chn EYE MASK VIOLation TOLerance ViolationTolerance Defines the violation tolerance i e the num...

Страница 919: ...generator signals CALCulate EYE MEASurement DATA returns something like Eye Measurements Eye Minimum 102 344 mV Eye Maximum 2 699 V Eye Base 277 189 V Eye Top 2 597 V Eye Mean 1 298 V Eye Amplitude 2...

Страница 920: ...e thresholds can either be specified by enum constants for the standard 10 90 or 20 80 rise times or numerically Suffix Chn Channel number used to identify the active trace Parameters ThresholdEnum Lo...

Страница 921: ...n See Active on page 749 CALCulate Chn EYE STATe Boolean Defines whether the active trace in the selected channel shall be represented as an eye diagram Suffix Chn Channel number used to identify the...

Страница 922: ...the active trace Parameters Boolean RST OFF Options R S ZNA K20 Manual operation See Low Pass on page 742 CALCulate Chn EYE STIMulus SCRambler Boolean Enables disables the scrambler in the bit stream...

Страница 923: ...FILTer GATE The CALCulate FILTer GATE commands define the properties of the time gate which is used to optimize the time domain response CALCulate FILTer GATE TIME AOFFset 923 CALCulate Chn FILTer GA...

Страница 924: ...just Time Gate on page 663 CALCulate Chn FILTer GATE TIME TYPE TimeGateFilter Selects the time gate filter type defining what occurs to the data in the specific time region Suffix Chn Channel number u...

Страница 925: ...nd is only available if a Dolph Chebyshev time gate is active CALCulate Chn FILTer GATE TIME WINDow DCHebyshev Suffix Chn Channel number used to identify the active trace Parameters SidebandSupp Sideb...

Страница 926: ...HOW Boolean Enables or disables permanent display of the gate limits Suffix Chn Channel number used to identify the active trace Parameters Boolean ON time gate permanently displayed OFF time gate hid...

Страница 927: ...e gate permanently Manual operation See Axis Pair on page 408 Note If the start frequency entered is greater than the current stop frequency the stop frequency is set to the start frequency plus the m...

Страница 928: ...and balanced fixture modeling is not supported CALCulate FMODel DELT Commands for Delta L PCB characterization see Chapter 4 7 17 Delta L 4 0 PCB characterization on page 271 CALCulate FMODel DELT Ph_...

Страница 929: ...BWIDth RESolution DREDuction 937 CALCulate FMODel DELT Ph_pt SWEep CONTrol IFBW 937 CALCulate FMODel DELT Ph_pt SWEep CONTrol STEP 938 CALCulate FMODel DELT Ph_pt SWEep FREQuency MAXimum 938 CALCulate...

Страница 930: ...ALCulate FMODel DELT Ph_pt FREQuencies CURRent to query the list of frequencies Suffix Ph_pt This suffix is ignored Usage Query only Options R S ZNA K231 Manual operation See Frequencies on page 700 C...

Страница 931: ...efined using CALCulate FMODel DELT Ph_pt FRE Quencies USER commands Suffix Ph_pt This suffix is ignored Parameters Boolean ON 1 Use default frequencies OFF 0 Use custom frequencies Options R S ZNA K23...

Страница 932: ...ithm can calculate the loss per inch uncertainties either at default or at custom frequencies see CALCulate FMODel DELT Ph_pt FREQuencies USEDefault CALCulate FMODel DELT Ph_pt FREQuencies USER ADD DE...

Страница 933: ...he 1 length method is used CALCulate FMODel DELT Ph_pt METHod M1L the VNA firmware builds up a cache of coupon measurements Touchstone files in the DeltaL 1LMeasurementCache subfolder of the user data...

Страница 934: ...l operation See Length on page 704 CALCulate FMODel DELT Ph_pt M1L MEASure CALCulate FMODel DELT Ph_pt M2L MEASure CALCulate FMODel DELT Ph_pt M3L MEASure Measures the full set of S parameters at the...

Страница 935: ...ults differently This command selects between the two modes Parameters Boolean OFF 0 Single ended mode ON 1 Differential mode RST OFF 0 Options R S ZNA K231 Manual operation See Method on page 700 CAL...

Страница 936: ...form input output pairs Options R S ZNA K231 Manual operation See Port Order on page 700 CALCulate FMODel DELT Ph_pt RUN Runs the AITT DL tool with the collected measurement data and the configured D...

Страница 937: ...Delta L measure ments see SENSe Ch BANDwidth RESolution DREDuction Suffix Ph_pt This suffix is ignored Parameters Boolean ON 1 Dynamic bandwidth at low frequencies is active default OFF 0 Dynamic ban...

Страница 938: ...and MINimum values are set as SENSe Ch FREQuency STOP and SENSe Ch FREQuency STARt respectively Furthermore the VNA collects data for the LPI computation a the MAXimum and MINi mum frequencies in addi...

Страница 939: ...ge 701 CALCulate FMODel EZD Commands for Eazy Deembedding see Chapter 4 7 14 Eazy de embedding based on IEEE 370 on page 268 CALCulate FMODel EZD Ph_pt COUPon MEASure For a fixture modeling with the E...

Страница 940: ...ODel EZD Ph_pt COUPon STATe Boolean For a fixture modeling with the EZD tool this command allows to specify the ports to which the test coupon is connected This has to be defined before measuring the...

Страница 941: ...Culate FMODel EZD Ph_pt DUT MEASure FILename String For a fixture modeling with the EZD tool this command loads the DUT Test Fixture properties from a Touchstone file Suffix Ph_pt This suffix is ignor...

Страница 942: ...xture deembedding data at the specified side 1 2 OFF 0 Do not create fixture deembedding data at the specified side 1 2 Options R S ZNA K210 Manual operation See Generate Side 1 Generate Side2 on page...

Страница 943: ...2 N are on the right Options R S ZNA K210 Manual operation See Port Order on page 686 CALCulate FMODel EZD Ph_pt PRESet Restores the default settings of the EZD tool Suffix Ph_pt This suffix is ignor...

Страница 944: ...0 otherwise Options R S ZNA K210 Manual operation See Apply on page 680 CALCulate FMODel ISD Commands for AtaiTec s In Situ De Embedding ISD tool see http ataitec com prod ucts isd CALCulate FMODel IS...

Страница 945: ...Del ISD Ph_pt TRACe COUPling 954 CALCulate FMODel ISD Ph_pt ATTenuation BEHavior AttenuationBehavior Sets gets the linear_2x batch mode parameter of the ISD tool LINear parameter value 1 Used for line...

Страница 946: ...Del ISD Ph_pt DUT MEASure FILename CALCulate FMODel ISD Ph_pt COUPon MEASure OPEN FILename or CALCulate FMODel ISD Ph_pt COUPon MEASure SHORt FILename Suffix Ph_pt This suffix is ignored Usage Event M...

Страница 947: ...easure Short on page 676 CALCulate FMODel ISD Ph_pt COUPon MEASure SHORt FILename String For a fixture modeling with the ISD tool and coupon type OPSHort1x see CALCulate FMODel ISD Ph_pt COUPon TYPE t...

Страница 948: ...CEXtrapolat Boolean Defines whether the de embedding file generated by the ISD tool contains extrapola ted DC data Can only be set to ON if a recent version of the ISD tool from 2019 12 or later is us...

Страница 949: ...Lename Suffix Ph_pt This suffix is ignored Usage Event Manual operation See Load File on page 678 CALCulate FMODel ISD Ph_pt DUT TYPE DUTType Defines whether the DUT is active or passive Corresponds t...

Страница 950: ...LCulate FMODel ISD Ph_pt FTIMe OVERride Boolean If set to true the flight time for DUT lead in is specified using CALCulate FMODel ISD Ph_pt FTIMe DUT Otherwise it is calculated automatically True is...

Страница 951: ...are on the left and ports N 1 to 2 N are on the right ODD ports 1 3 5 etc are on the left and ports 2 4 6 etc are on the right ALL use this to tell the ISD tool that all ports are on the left i e assu...

Страница 952: ...P to define an arbitrary set of ports to be skipped Suffix Ph_pt This suffix is ignored Usage Event Manual operation See Ports to Skip on page 683 CALCulate FMODel ISD Ph_pt PRESet Restores the defaul...

Страница 953: ...ber for single ended deembedding logical port number for balanced deembedding Parameters Boolean 1 ON if a deembedding file shall be assinged to port Pt 0 OFF otherwise Example See Chapter 8 2 8 Fixtu...

Страница 954: ...nual operation See Small Fixture on page 685 CALCulate FMODel ISD Ph_pt SMALlfixture Boolean Enables disables the ISD tool s small fixture mode Parameters Boolean Manual operation See Small Fixture on...

Страница 955: ...TATe 958 CALCulate FMODel SFD Ph_pt RUN RUN 958 CALCulate FMODel SFD Ph_pt RUN STATe 959 CALCulate FMODel SFD Ph_pt TOTaldiffcfg 959 CALCulate FMODel SFD Ph_pt AUTO Boolean Defines whether the SFD too...

Страница 956: ...peration See Load File 1x Open Preset 1x Short Preset on page 677 CALCulate FMODel SFD Ph_pt COUPon STATe Boolean For a fixture modeling with the SFD tool this command allows to specify the ports to w...

Страница 957: ...s see CALCulate FMODel SFD Ph_pt DUT STATe Suffix Ph_pt This suffix is ignored Usage Event Manual operation See Measure on page 678 CALCulate FMODel SFD Ph_pt DUT MEASure FILename String For a fixture...

Страница 958: ...d to port Ph_pt 0 OFF false otherwise Manual operation See Active on page 678 CALCulate FMODel SFD Ph_pt RUN RUN Runs the SFD tool Before executing this command make sure that The test coupon measurem...

Страница 959: ...fix Ph_pt This suffix is ignored Parameters SFDPortConfig ODD NON ODD odd ports are on the left and even ports are on the right NON ports 1 to N are on the left and ports N 1 to 2 N are on the right M...

Страница 960: ...ented in the graphical display Note The analyzer allows arbitrary combinations of display formats and measured quantities see Chapter 5 3 Format softtool on page 374 and CALCulate Ch PARameter command...

Страница 961: ...ys it in a Cartesian diagram UPHase Calculates Phase z unwrapped and displays it in a Cartesian diagram POLar COMPlex for compatibility with R S ZVR ana lyzers Displays z in a polar diagram SMITh Disp...

Страница 962: ...measure ment CALCulate Chn GDAPerture SCOunt Steps Defines an aperture for the calculation of the group delay as an integer number of fre quency sweep steps Suffix Chn Channel number used to identify...

Страница 963: ...ue Manual operation See Mode on page 420 7 3 1 12 CALCulate LDEViation CALCulate Chn LDEViation AUTO Activate Initiates a re calculation of the linearity deviation correction factors and applies them...

Страница 964: ...ength for the linearity deviation calculation This command is available if the active trace format is Phase or Unwrapped Phase or if a mixer delay is measured Suffix Chn Channel number used to identif...

Страница 965: ...3 1 13 CALCulate LIMit The CALCulate LIMit commands define the limit lines and control the limit check CALCulate LIMit CIRCle FAIL ALL 966 CALCulate LIMit FAIL ALL 966 CALCulate LIMit FAIL DATA 966 C...

Страница 966: ...IMit CIRCle FAIL ALL RecallSet Returns a 0 or 1 to indicate whether or not the circle limit check has failed for at least one channel in the referenced recall set 0 represents pass and 1 represents fa...

Страница 967: ...ets the circle test for the active trace of channel Chn Suffix Chn Channel number used to identify the active trace Usage Event Manual operation See Clear Test on page 447 CALCulate Chn LIMit CIRCle D...

Страница 968: ...LIMit CIRCle FAIL Returns a 0 or 1 to indicate whether or not the circle limit check has failed 0 repre sents pass and 1 represents fail Tip Use CALCulate CLIMits FAIL to perform a composite global l...

Страница 969: ...stimulus values of the limit line segments no 1 to n are updated the limit line segments n 1 k are generated with default response values see CALCulate Chn LIMit UPPer DATA CALCulate Chn LIMit LOWer D...

Страница 970: ...the stimulus range between 1 GHz and 2 GHz using default response values CALC LIM CONT SHIF 1 CALC LIM CONT Shift the segment by 1 Hz The modified limit line segment ranges from 1000000001 Hz to 20000...

Страница 971: ...limit line segment in the active diagram Manual operation See Segment List on page 436 CALCulate Chn LIMit DCIRcle STATe Boolean Sets queries the state of the display circle for the active trace of c...

Страница 972: ...whenever the related trace is displayed in complex format Manual operation See Show Border on page 448 CALCulate Chn LIMit DELete ALL Deletes all limit line segments Suffix Chn Channel number used to...

Страница 973: ...Example RST CALC LIM CONT 1 GHZ 2 GHZ Define an upper limit line segment in the stimulus range between 1 GHz and 2 GHz using default response values CALC LIM STAT ON FAIL Switch the limit check on and...

Страница 974: ...it line segments 2k 1 2s updates odd limit line segments 1 3 2s 1 with type upper and the given response values creates upper limit line segments 2s 1 2s 3 2k 1 with type upper and the given response...

Страница 975: ...ce TraceName is specified Setting parameters StimulusOffset Stimulus offset value used to shift all imported limit line seg ments in horizontal direction Default unit NN ResponseOffset Response offset...

Страница 976: ...it SEGMent Seg AMPLitude STARt Response CALCulate Chn LIMit SEGMent Seg AMPLitude STOP Response These commands change the start or the stop response values i e the response val ues assigned to the sta...

Страница 977: ...ents including enabled and disabled segments Suffix Chn Channel number used to identify the active trace Example CALC LIM DATA 1 1500000000 2000000000 2 3 Define an upper limit line segment segment no...

Страница 978: ...stom formula for a segment use CALCulate Chn LIMit CONTrol DATA to create it Parameters State OFF 0 The line segment is a straight line connecting the endpoints specified in the segment definition ON...

Страница 979: ...s for time sweeps For CW mode sweeps stimulus values are dimensionless Default unit NN Example CALC LIM DATA 1 1500000000 2000000000 2 3 Define an upper limit line segment segment no 1 in the stimu l...

Страница 980: ...late Chn LIMit SOUNd STATe Boolean Switches the acoustic signal fail beep on or off The fail beep is generated each time the analyzer detects an exceeded limit Suffix Chn Channel number used to identi...

Страница 981: ...Example See CALCulate Chn LIMit FAIL Manual operation See Limit Check on page 432 CALCulate Chn LIMit TTLout Pt STATe Boolean Switches the TTL pass fail signals on or off The signals are applied to th...

Страница 982: ...r Mk FORMat 991 CALCulate Chn MARKer Mk FUNCtion BWIDth MODE 992 CALCulate Chn MARKer Mk FUNCtion CENTer 992 CALCulate Chn MARKer Mk FUNCtion DOMain USER RANGe 993 CALCulate Chn MARKer Mk FUNCtion DOM...

Страница 983: ...ate mark ers 1 and 2 for it The default position for both markers is the center of the sweep range INSTrument NSELect 2 Make channel 2 the active channel CALCulate MARKer COUPled TYPE ALL Select coupl...

Страница 984: ...zontalPos VerticalPos Moves the marker info field for the active trace Chn to one of nine predefined posi tions in the active diagram Suffix Chn Channel number used to identify the active trace Parame...

Страница 985: ...arker Frmt on page 380 SCPI GUI MLINear Lin Mag MLOGarithmic MDB for R S ZVR compatibility dB Mag PHASe Phase POLar COMPlex for R S ZVR compatibility Real Imag GDELay Delay REAL Real IMAGinary Imag SW...

Страница 986: ...Chn s active trace this command does the following Create a channel Set its center to marker Mk s position Set its span to 10 times channel Chn s BW Switch to spectrum mode Suffix Chn Channel number u...

Страница 987: ...Channel number used to identify the active trace Parameters Value Threshold value The unit is derived from the active trace format and cannot be modified Changing the trace format resets the threshol...

Страница 988: ...tion VerticalPos TOP MID BOTTom Vertical position Example See CALCulate Chn MARKer Mk BWIDth Manual operation See Bandpass Ref to Max on page 467 CALCulate Chn MARKer Mk AOFF Removes all markers from...

Страница 989: ...nter frequency and the 3 dB bandwidth Loss loss at the center of the bandpass bandstop region the response value of marker M4 at the time of the bandfilter search LowerEdge lower band edge UpperEdge u...

Страница 990: ...Boolean Switches the delta mode for marker Mk on trace no Chn on or off The marker must be created before using CALCulate Chn MARKer Mk STATe ON If the active trace contains no reference marker the c...

Страница 991: ...e Excursion Settings on page 461 CALCulate Chn MARKer Mk FORMat OutFormat Sets queries the output format for the complex value of the related marker Suffix Chn Channel number used to identify the acti...

Страница 992: ...x BPRMarker Bandpass Search Ref to Marker BSRMarker Bandstop Search Ref to Marker BPABsolute Bandpass Absolute Level BSABsolute Bandstop Absolute Level NONE deactivate bandfilter search result off RST...

Страница 993: ...ll span search range Example CALC1 MARK1 FUNC DOM USER 2 Select the search range no 2 assigned to marker no 1 and trace no 1 CALC MARK FUNC DOM USER STARt 1GHz Set the start frequency of the search ra...

Страница 994: ...de for marker no Mk and initiates the search The marker must be created before using CALCulate Chn MARKer Mk STATe ON exception bandfilter search Suffix Chn Channel number used to identify the active...

Страница 995: ...MARKer Mk BWIDth MMAXimum or MMINimum Multiple peak search SPRogress Sweep progress CALCulate Chn MARKer Mk FUNCtion RESult Returns the result stimulus and response value of a search started by means...

Страница 996: ...the sweep range equal to the stimulus value of the marker Mk on trace no Chn Suffix Chn Channel number used to identify the active trace Mk Marker number Example RST CALC MARK ON Create marker 1 in t...

Страница 997: ...Mk The marker doesn t have to be created before CALCulate Chn MARKer Mk STATe ON the name can be assigned in advance Suffix Chn Channel number used to identify the active trace Mk Marker number Parame...

Страница 998: ...ance PADMittance MADMittance MPIMpedance MPADmittance INDX NOISe DEFault means the reference marker is formatted according the related trace s default marker format see CALCulate Chn MARKer DEFault FO...

Страница 999: ...ce Mk This numeric suffix is ignored and may be set to any value Parameters MarkerName Marker name string parameter RST R Example See CALCulate Chn MARKer Mk NAME Manual operation See Marker Name on p...

Страница 1000: ...or power sweeps 0 s to 127500 s for time sweeps and 1 to 100001 for CW mode Setting parameters Seg For a segmented frequency sweep with overlapping segments you can assign the reference marker to a pa...

Страница 1001: ...Ker Mk FUNCtion EXECute It enables or disables tracking which causes the search logic of the related marker s to be repeated after each sweep Tip If the current search mode is a bandfilter or multiple...

Страница 1002: ...e of z in dB Mag z 20 log z dB PHASe Phase of z z arctan y x UPHase Unwrapped phase of z comprising the complete number of 360 phase rotations z z 2k 360 REAL Real part of z Re z x IMAGinary Imaginary...

Страница 1003: ...MARKer1 FUNCtion EXECute Execute the target search for marker 1 CALCulate1 MARKer1 FUNCtion RESult Query for the results Manual operation See Target Format on page 464 CALCulate Chn MARKer Mk STATe B...

Страница 1004: ...e Defines a marker specific threshold value for single peak searches Use CALCulate Chn MARKer Mk THReshold STATe to activate it Suffix Chn Channel number used to identify the active trace Mk Marker nu...

Страница 1005: ...sequent sweeps Tracking is disabled Stimulus and response value are stored with the marker they are not adjusted to subsequent sweeps and trace format changes The marker stimulus can be set using CALC...

Страница 1006: ...se 9 9E 11 Hz to 9 9E 11 Hz for frequency sweeps 999 dBm to 999 dBm for power sweeps 0 s to 127500 s for time sweeps and 1 to 100001 for CW mode Setting parameters Seg For a segmented frequency sweep...

Страница 1007: ...race format and marker format requires a concrete stimulus value Default unit NN Example Suppose that the active recall set contains an active trace no 1 CALC MARK ON Create marker no 1 and display it...

Страница 1008: ...CALC MATH MEM Copy the current state of the default trace Trc1 to a memory trace named Mem2 Trc1 The memory trace is not dis played CALC MATH SDEF Trc1 Mem2 Trc1 Define a mathematical trace dividing t...

Страница 1009: ...Chn MATH FORMatted EXPRession SDEFine to define general math traces Suffix Chn Channel number used to identify the active trace Parameters Mode NORMal ADD SUBTract MULTiply DIVide NORMal Math trace a...

Страница 1010: ...TraceName DataTraceName To copy an active mathematical trace use TRACe COPY MATH MemTraceName DataTraceName Suffix Chn Channel number used to identify the active trace Example RST CALC MATH MEM Copy t...

Страница 1011: ...umes that the result of the mathematical expression represents a volt age OFF Result is Wave Quantity disabled the analyzer assumes that the result of the mathematical expression is dimensionless RST...

Страница 1012: ...the default trace Trc1 in channel 1 The manual control screen shows No Trace Example See CALCulate Ch PARameter DELete Usage Event Manual operation See Delete Trace on page 388 CALCulate PARameter DEL...

Страница 1013: ...Creates the traces for all S parameters associated with a group of logical ports S parameter group The traces can be queried using CALCulate Ch DATA SGRoup Traces must be selected to become active tr...

Страница 1014: ...e names are important for referencing the generated traces see program example below LogicalPort2 Example CALC2 PAR DEF SGR 1 2 Create channel 2 and four traces to measure the two port S parameters S1...

Страница 1015: ...meters CALCulate4 PARameter DELete CH4TR1 Delete the first created trace CALCulate4 PARameter DELete CALL Delete the remaining three traces in channel 4 CALCulate PARameter DELete ALL Delete all trace...

Страница 1016: ...defined traces You can open the Trace Manager dialog to obtain an overview of all channels and traces including the traces that are not displayed Suffix Ch Channel number of an existing channel conta...

Страница 1017: ...parame ter to it The trace becomes the active trace in the channel but is not displayed Note To display the trace defined via CALCulate Ch PARameter SDEFine cre ate a diagram DISPlay WINDow Wnd STATe...

Страница 1018: ...rs are determined by the channel s logical port configuration For details about the measurement results see Chapter 4 3 Measurement results on page 122 S11 S12 S0101 Single ended S parameters S i j fo...

Страница 1019: ...ies denote a normal sample RMS peak or average detector AP1G2 AP01G02 BP1G2 BP01G02 AP1G1SAM AP1G1RMS AP1G1PEAK AP1G1AVG Primed wave quantities with port numbers and external generator providing the s...

Страница 1020: ...D no PAE21 PAE12 Power added efficiency referring to logical ports IM3UI IM3UO IM3LI IM3LO IM3MI IM3MO IM5UI IM9MO Intermodulation product IM order side at DUT where order 3 5 7 9 side U L M for uppe...

Страница 1021: ...displayed Similar to CALCulate Ch PARameter SDEFine but in presence of balanced ports this command distinguishes between single ended S parameters for logical ports SSS Li Lj raw single ended S param...

Страница 1022: ...te Chn PHOLd HoldFunc Enables disables or restarts the max hold and the min hold functions Suffix Chn Channel number used to identify the active trace Parameters HoldFunc MIN MAX OFF MIN Enable the mi...

Страница 1023: ...active recall set Parameters Enable ON Info fields are displayed for all traces for which a limit check is enabled OFF Only the info field for the active trace is displayed if the ripple check is ena...

Страница 1024: ...r sweep CALC RIPP CONT DOM PLIN Delete all existing ripple limit ranges and select level units for the domain of the active trace CALC RIPP DATA 1 10 5 3 Define and enable a ripple limit range in the...

Страница 1025: ...00000 2000000000 3 1 2000000000 3000000000 5 Define and enable a ripple limit range in the stimulus range between 1 5 GHz and 2 GHz assigning a ripple limit of 3 dB Define and enable a second ripple l...

Страница 1026: ...ck has failed Tip Use CALCulate Chn RIPPle SEGMent Seg RESult to query the result for a single ripple limit range Suffix Chn Channel number used to identify the active trace Example RST CALC RIPP DATA...

Страница 1027: ...sage Setting only CALCulate Chn RIPPle SEGMent COUNt Queries the number of ripple limit ranges The response is an integer number Suffix Chn Channel number used to identify the active trace Example RST...

Страница 1028: ...Chn Channel number used to identify the active trace Seg Number of the ripple limit range Parameters Limit Ripple limit in the range The unit is adjusted to the format of the active trace CALCulate C...

Страница 1029: ...d ripple limit range with disabled limit check no limit check results are displayed in the diagram area CALC RIPP SEGM2 RES Query the result for the second range Possible response 0 1 149071925 Usage...

Страница 1030: ...mit Suffix Chn Channel number used to identify the active trace Parameters Boolean ON OFF fail beep on or off RST OFF Example CALC RIPP STAT ON SOUN ON Switch the limit check on and activate the fail...

Страница 1031: ...r trace no Chn Suffix Chn Channel number used to identify the active trace Parameters Boolean ON OFF smoothing on or off RST OFF Example See CALCulate Chn SMOothing APERture Manual operation See Smoot...

Страница 1032: ...in USER 1036 CALCulate Chn STATistics NLINear COMP RDOMain USER SHOW 1037 CALCulate Chn STATistics NLINear COMP RDOMain USER STARt 1037 CALCulate Chn STATistics NLINear COMP RDOMain USER STOP 1037 CAL...

Страница 1033: ...e El Length results in addition CALC STAT SFL ON Display the Flatness Gain Slope results in addition CALC STAT STAT AREA LEFT TOP For a subsequent check at the GUI or a hardcopy move the info field to...

Страница 1034: ...AT DOM USER STARt 1GHZ STOP 2GHZ SHOW ON Select evaluation range no 1 and define the evaluation range between 1 GHz and 2 GHz Display the range limit lines Manual operation See Evaluation Range on pag...

Страница 1035: ...info field on or off RST OFF Example See CALCulate Chn STATistics STATe Manual operation See Min Max Peak Peak Mean Std Dev RMS on page 410 CALCulate Chn STATistics FORMat Format For complex valued t...

Страница 1036: ...Parameters PhaseValue Compression value Default unit deg Manual operation See Compr Point Compr Val on page 413 CALCulate Chn STATistics NLINear COMP RDOMain USER RefRange Selects one of the 10 channe...

Страница 1037: ...top value of the related range Manual operation See Ref Range on page 415 CALCulate Chn STATistics NLINear COMP REFerence ReferenceValue Defines how the reference value small signal value for the comp...

Страница 1038: ...fy the active trace Example RST SWE TYPE POW Select a power sweep with default CW frequency and sweep range CALC STAT NLIN COMP LEV 2 Define a compression value of 2 dB CALC STAT NLIN COMP RES Query t...

Страница 1039: ...defines the reference phase for compression point calculation on phase for matted traces Only applies if CALCulate Chn STATistics NLINear COMP REFerence is set to DVALue For dB formatted traces the re...

Страница 1040: ...LCulate Chn STATistics RDOMain USER EvalRange Selects one of the 10 channel specific user defined ranges as the reference range for spectrum power ratio calculations The selected range can then be con...

Страница 1041: ...page 415 CALCulate Chn STATistics PRATio STATe Boolean Displays or hides the power ratio statistics in the diagram area of spectrum analysis channel Chn s active trace Use CALCulate Chn STATistics PRA...

Страница 1042: ...tandard deviation of all response values MAX return the maximum of all response values MIN return the minimum of all response values RMS return the root mean square of all response values PTPeak retur...

Страница 1043: ...esults in the diagram area of trace no Chn Suffix Chn Channel number used to identify the active trace Parameters Boolean ON OFF statistical info field on or off RST OFF Example See CALCulate Chn STAT...

Страница 1044: ...CALCulate Chn TRANsform TIME DCHebyshev 1047 CALCulate Chn TRANsform TIME LPASs 1047 CALCulate Chn TRANsform TIME LPASs DCSParam 1047 CALCulate Chn TRANsform TIME LPASs DCSParam CONTinuous 1048 CALCul...

Страница 1045: ...ce RNORmal Model Selects the theory for the renormalization of port impedances The selection has an impact on the conversion formulas for wave quantities and S parameters Suffix Chn Channel number use...

Страница 1046: ...f points Manual operation See Type on page 404 CALCulate Chn TRANsform TIME CENTer CenterTime Defines the center time of the diagram in time domain Suffix Chn Channel number used to identify the activ...

Страница 1047: ...obe Level on page 404 CALCulate Chn TRANsform TIME LPASs Algorithm Calculates the harmonic grid for low pass time domain transforms according to one of the three alternative algorithms Suffix Chn Chan...

Страница 1048: ...ed trace overwrite the defined DC value and query the new value CALC TRAN TIME LPAS DCSP CONT ON Switch over to continuous extrapolation e g because you noticed a discrepancy between the manually ente...

Страница 1049: ...ulate Chn TRANsform TIME LPASs if you wish to use one of the other algorithms for calculating the grid Suffix Chn Channel number used to identify the active trace Example See CALCulate Chn TRANsform T...

Страница 1050: ...ve trace Parameters StartTime Start time of the diagram Range 100 s to 99 999999999998 s Increment 0 1 ns RST 1E 009 s Default unit s Example RST CALC TRAN TIME STAT ON Reset the instrument activating...

Страница 1051: ...ass mode STEP step response only in lowpass mode a bandpass mode setting CALCulate Chn TRANsform TIME TYPE BPASs is automatically changed to lowpass RST IMP Example See CALCulate Chn TRANsform TIME TY...

Страница 1052: ...ng in time units or distance units Suffix Chn Channel number used to identify the active trace Parameters Unit TIME DISTance TIME x axis scaled in time units DISTance x axis scaled in distance units D...

Страница 1053: ...airs CblAtt1 CblFreq1 CblAtt2 CblFreq2 At least one pair has to be specified Suffix Chn Channel number This suffix is ignored cable types are defined for all channels Setting parameters CblName Name o...

Страница 1054: ...tes the user defined cable type My cable type Usage Setting only Options R S ZNA K2 Manual operation See Add Delete on page 767 CALCulate Chn TRANsform DTFault SELect DtfSelectCable Selects one of the...

Страница 1055: ...e DtF limit checking and CALCulate Chn TRANsform DTFault PEAK THReshold to set the fault limit Suffix Chn Channel number used to identify the active trace Usage Query only Options R S ZNA K2 Manual op...

Страница 1056: ...the active trace of channel 1 a DtF trace CALCulate1 TRANsform DTFault PEAK STATe ON enables DtF limit checking Options R S ZNA K2 Manual operation See Fault Limit Check on page 765 CALCulate Chn TRAN...

Страница 1057: ...DTFault STATe ON makes the active trace of channel 1 a DtF trace SENSe1 SWEep POINts returns the default number of sweep points 201 CALCulate1 TRANsform DTFault STARt 0 STOP 100 widens the DtF distanc...

Страница 1058: ...ive trace of channel 1 ON Options R S ZNA K2 Manual operation See Distance to Fault on page 762 7 3 1 25 CALCulate TRANsform VNETworks The CALCulate TRANsform VNETworks commands define and activate th...

Страница 1059: ...1178 6462 02 20 Parameter Circuit model Pictogram CSC Serial C shunt C LSL Serial L shunt L SLC Shunt L serial C SCL Shunt C serial L SCC Shunt C serial C SLL Shunt L serial L SHLC Shunt L shunt C GS...

Страница 1060: ...nt C SGL Shunt C serial L SLG Shunt L serial C SGG Shunt C serial C Table 7 7 Circuit models for balanced port and port pair de embedding Parameter Circuit model Pictogram FIMPort File import generic...

Страница 1061: ...r Circuit model Pictogram SLST Shunt L serial Touchstone s2p data SCST Shunt C serial Touchstone s2p data CSSL Serial Cs shunt L LSSC Serial Ls shunt C CSSC Serial Cs shunt C LSSL Serial Ls shunt L SL...

Страница 1062: ...r Circuit model Pictogram SCCS Shunt C serial Cs SLLS Shunt L serial Ls STSG Serial Touchstone s2p data shunt C SGST Shunt C serial Touchstone s2p data GSSL Serial Cs shunt L LSSG Serial Ls shunt C GS...

Страница 1063: ...t set de embedding Parameter Circuit model Pictogram FIMPort File import no circuit model STSL SGGS The models for port pairs i e port sets with two ports are the same as the ones for balanced ports S...

Страница 1064: ...gPt PARameters DATA Port 1068 CALCulate Ch TRANsform VNETworks BALanced DEEMbedding LogPt PARameters G Cmp 1069 CALCulate Ch TRANsform VNETworks BALanced DEEMbedding LogPt PARameters L Cmp 1069 CALCul...

Страница 1065: ...VNETworks GLOop EMBedding group PARameters C 1084 CALCulate Ch TRANsform VNETworks GLOop EMBedding group PARameters G 1085 CALCulate Ch TRANsform VNETworks GLOop EMBedding group PARameters L 1085 CALC...

Страница 1066: ...PhyPt TNDefinition 1102 CALCulate Ch TRANsform VNETworks SENDed EMBedding PhyPt STATe 1102 CALCulate Ch TRANsform VNETworks SENDed EMBedding PhyPt PARameters DATA 1103 CALCulate Ch TRANsform VNETwork...

Страница 1067: ...apacitance parameter must be omitted The command returns the capacitance value for the specified circuit model Suffix Ch Channel number LogPt Logical port number balanced port Cmp Number of capacitanc...

Страница 1068: ...data from a Touchstone file located at the R S ZNA s file system Suffix Ch Channel number LogPt Logical port number Port Port assignment for two 2 port networks 1 Port 1 2 Port 2 This parameter is ig...

Страница 1069: ...specified circuit model Range 1kS to 1 kS Increment 1 nS 1E 9 S RST 0 S Default unit Siemens SI unit symbol S Parameters for setting and query CircuitModel STSG SGST GSSL LSSG GSSG SLGS SGLS SGGS Pos...

Страница 1070: ...Culate Ch TRANsform VNETworks BALanced DEEMbedding LogPt PARameters R Cmp CiruitModel Resistance CALCulate Ch TRANsform VNETworks BALanced DEEMbedding LogPt PARameters R Cmp CiruitModel Specifies the...

Страница 1071: ...TNDefinition CircuitModel Selects the circuit model for balanced port deembedding Suffix Ch Channel number LogPt Logical port number balanced port Parameters CircuitModel FIMPort STSL STSC SLST SCST...

Страница 1072: ...ance parameter must be omitted The command returns the capacitance value for the specified circuit model Suffix Ch Channel number LogPt Logical port number balanced port Cmp Number of capacitance in c...

Страница 1073: ...Touchstone file located at the R S ZNA s file system Suffix Ch Channel number LogPt Logical port number Port Port assignment for two 2 port networks 1 Port 1 2 Port 2 This parameter is ignored for 4 p...

Страница 1074: ...ecified circuit model Range 1kS to 1 kS Increment 1 pS 1E 12 S RST 0 S Default unit Siemens SI unit symbol S Parameters for setting and query CircuitModel STSG SGST GSSL LSSG GSSG SLGS SGLS SGGS Possi...

Страница 1075: ...ulate Ch TRANsform VNETworks BALanced EMBedding LogPt PARameters R Cmp CircuitModel Resistance CALCulate Ch TRANsform VNETworks BALanced EMBedding LogPt PARameters R Cmp CircuitModel Specifies the res...

Страница 1076: ...d EMBedding LogPt TNDefinition CircuitModel Selects the circuit model for balanced port embedding Suffix Ch Channel number LogPt Logical port number balanced port Parameters CircuitModel FIMPort STSL...

Страница 1077: ...ion See Network on page 696 CALCulate Ch TRANsform VNETworks DIFFerential EMBedding LogPt PARameters DATA Interchange SParamTrcs Defines a Differential match embedding network for a balanced port base...

Страница 1078: ...uctance Range 10 MS to 10 MS Increment 1 S 1E 6 F RST 0 S Default unit S iemens Manual operation See Network on page 696 CALCulate Ch TRANsform VNETworks DIFFerential EMBedding LogPt PARameters L Cmp...

Страница 1079: ...M to 10 M Increment 1 m 1E 3 RST 0 Default unit Ohm Manual operation See Network on page 696 CALCulate Ch TRANsform VNETworks DIFFerential EMBedding LogPt TNDefinition CircuitModel Selects the circui...

Страница 1080: ...ion See Active on page 659 CALCulate Ch TRANsform VNETworks GLOop DEEMbedding group PARameters C CircuitModel Capacitance CALCulate Ch TRANsform VNETworks GLOop DEEMbedding group PARameters C CircuitM...

Страница 1081: ...roup DUT number If multiple port groups are configured see SOURce Ch GROup Grp PPORts and CALCulate Ch TRANsform VNETworks GLOop GROup is ON then each port group can have its own de embedding models P...

Страница 1082: ...L SL 2 2E 9 Increase the inductance to 2 2 nH Manual operation See Network on page 694 CALCulate Ch TRANsform VNETworks GLOop DEEMbedding group PARameters R CircuitModel Resistance CALCulate Ch TRANs...

Страница 1083: ...URce Ch GROup Grp PPORts and CALCulate Ch TRANsform VNETworks GLOop GROup is ON then each port group can have its own de embedding models Parameters CircuitModel FIMPort SL SC SG Possible circuit mode...

Страница 1084: ...ue C in the different circuit models for ground loop embedding Suffix Ch Channel number group Port group DUT number If multiple port groups are configured see SOURce Ch GROup Grp PPORts and CALCulate...

Страница 1085: ...ance G for the specified circuit model Range 1kS to 1 kS Increment 1 pS 1E 12 S RST 0 S Default unit Siemens SI unit symbol S Parameters for setting and query CircuitModel SG Possible circuit models c...

Страница 1086: ...cuitModel Resistance CALCulate Ch TRANsform VNETworks GLOop EMBedding group PARameters R CircuitModel Specifies the resistance value R in the different circuit models for ground loop embed ding Suffix...

Страница 1087: ...have its own de embedding models Parameters CircuitModel FIMPort SL SC SG Possible circuit models character data see Circuit models for ground loop de embedding RST FIMPort Example See CALCulate Ch T...

Страница 1088: ...n See Add Delete on page 655 CALCulate Ch TRANsform VNETworks PPAir DEEMbedding ListId STATe arg0 Enables or disables the deembedding function for port set or port pair ListId It is allowed to change...

Страница 1089: ...r to be created Parameters PP_First PP_Second PP_First Sequence of port pairs each one consisting of two different ports The port pairs don t have to be disjoint Example See CALCulate Ch TRANsform VNE...

Страница 1090: ...Model Specifies the conductance value G i in the different lumped circuit models for port pair deembedding In the query form the Conductance parameter must be omitted The command returns the conductan...

Страница 1091: ...umeric suffix is set to 1 Parameters Inductance Inductance L i Range 1 H to 1 H Increment 1 pH 1E 12 H RST 1 nH 1E 9 H Default unit H Parameters for setting and query CircuitModel STSL SLST CSSL LSSC...

Страница 1092: ...nual operation See Network on page 688 CALCulate Ch TRANsform VNETworks PPAir DEEMbedding ListId TNDefinition arg0 Selects the circuit model for port pair deembedding Suffix Ch Channel number ListId I...

Страница 1093: ...Bedding ListId DEFine PP_First PP_Second PP_First Creates one or more port pairs for port set embedding The command can be used repeatedly to extend or partially overwrite the list of port sets for em...

Страница 1094: ...or port pair embedding In the query form the Capacitance parameter must be omitted The command returns the capacitance value for the specified circuit model Suffix Ch Channel number ListId Index of th...

Страница 1095: ...pecified the numeric suffix is set to 1 Parameters Inductance Inductance L i Range 1 H to 1 H Increment 1 pH 1E 12 H RST 1 nH 1E 9 H Default unit H Parameters for setting and query arg0 STSL SLST CSSL...

Страница 1096: ...ate Ch TRANsform VNETworks PPAir EMBedding ListId STATe Manual operation See Network on page 688 CALCulate Ch TRANsform VNETworks PPAir EMBedding ListId PARameters G 1 2 3 arg0 Conductance CALCulate C...

Страница 1097: ...see Table 7 7 Example See CALCulate Ch TRANsform VNETworks PPAir EMBedding ListId STATe Manual operation See Network on page 688 CALCulate Ch TRANsform VNETworks PSET DEEMbedding ListId DEFine Port1 P...

Страница 1098: ...l C shunt L circuit model for deembedding CALC TRAN VNET SEND DEEM PAR R2 CSL 2 2E 3 CALC TRAN VNET SEND DEEM ON Increase the resistance R2 for the Serial C shunt L circuit model to 2 2 k and enable d...

Страница 1099: ...tance parameter must be omitted The command returns the capacitance value for the specified circuit model Suffix Ch Channel number PhyPt Physical port number Cmp Number of capacitance in circuit model...

Страница 1100: ...1E 9 S RST 0 S Default unit Siemens SI unit symbol S Parameters for setting and query CircuitModel SHLC Circuit model whose conductance G Cmp shall be set see Table 7 6 Example RST CALC TRAN VNET SEN...

Страница 1101: ...Manual operation See Network on page 671 CALCulate Ch TRANsform VNETworks SENDed DEEMbedding PhyPt PARameters R Cmp CircuitModel Resistance CALCulate Ch TRANsform VNETworks SENDed DEEMbedding PhyPt PA...

Страница 1102: ...tModel Selects the circuit model for single ended port deembedding Suffix Ch Channel number PhyPt Physical port number Parameters CircuitModel FIMPort CSL LSC CSC LSL SLC SCL SCC SLL SHLC GSL LSG GSG...

Страница 1103: ...executing this com mand MMEMory LOAD VNETworks Ch SENDed EMBedding PhyPt to load circuit data from a Touchstone file located at the R S ZNA s file system Suffix Ch Channel number PhyPt Physical port n...

Страница 1104: ...RAN VNET SEND EMB PAR C2 CSC Query the default capacitance C2 for the Serial C shunt C cir cuit model The response is 1E 012 1 pF CALC TRAN VNET SEND EMB PAR C2 CSC 2 2E 12 Increase the capacitance to...

Страница 1105: ...NDed EMBedding PhyPt PARameters L Cmp CircuitModel Inductance CALCulate Ch TRANsform VNETworks SENDed EMBedding PhyPt PARameters L Cmp CircuitModel Specifies the inductance value L Cmp in the differen...

Страница 1106: ...lue for the specified circuit model Suffix Ch Channel number PhyPt Physical port number Cmp Number of resistance in circuit model The total number of resis tances depends on the selected circuit model...

Страница 1107: ...tion See Network on page 671 CALCulate Ch TRANsform VNETworks WAVes Boolean Defines whether the wave based or the classical S parameter based de embedding calculation is used in channel Ch Suffix Ch C...

Страница 1108: ...e stop threshold crossing distance stop threshold crossing voltage CALCulate Chn TTIMe STATe Boolean Enables disables the Rise Time Measurement Note The rise time measurement can only be enabled if th...

Страница 1109: ...vice spe cific CONFigure CHANnel CATalog 1109 CONFigure CHANnel MEASure ALL STATe 1110 CONFigure CHANnel MEASure OPTimized 1110 CONFigure CHANnel Ch STATe 1111 CONFigure CHANnel Ch MEASure STATe 1112...

Страница 1110: ...ed Parameters Boolean ON OFF RST ON Example See CONFigure CHANnel Ch MEASure STATe Manual operation See Continuous Single on page 511 CONFigure CHANnel MEASure OPTimized Order Ch1 Ch2 This setting tel...

Страница 1111: ...NF CHAN MEAS OPT MAN 2 3 1 4 INIT2 INIT3 INIT1 INIT4 optimize the switching times for channel order 2 3 1 4 and restart the sweeps in the same order Usage Setting only CONFigure CHANnel Ch STATe Boole...

Страница 1112: ...three channels CONFigure CHANnel MEASure ALL OFF Disable the measurement in all channels CONFigure CHANnel2 MEASure ON Re enable the measurement in channel no 2 The analyzer measures in channel 2 the...

Страница 1113: ...ffix Ch Channel number Setting parameters TraceName Trace name e g Trace 4 Example RST CONF CHAN TRAC REN Testtrace_1 Reset the analyzer to create a default trace in channel 1 and set this trace as th...

Страница 1114: ...Trc2 CONF CHAN1 TRAC CAT Query the channels in channel no 1 The response is 1 Trc1 CONF TRAC NAME ID Ch2Trc2 Query the trace number for the trace named Ch2Trc2 The response is 2 CONF TRAC2 NAME Query...

Страница 1115: ...Create a diagram no 2 DISP WIND2 TRAC3 FEED Trc2 Display the trace in the new diagram no 2 assigning the trace number 3 CONF TRAC WIND Trc2 Query the diagram number for Trc2 The response is 2 CONF TRA...

Страница 1116: ...xisting trace Parameters TraceName Trace name e g Ch2Trc2 RST Trc1 Example See CONFigure TRACe CATalog CONFigure TRACe Trc NAME ID TraceName Queries the trace number numeric suffix of a trace with kno...

Страница 1117: ...Trol Ch GPIO TIME 1121 CONTrol Ch GPIO VOLTage OUTPut 1121 CONTrol Ch GPIO Port STATe 1122 CONTrol Ch GPIO Port RANGe 1122 CONTrol Ch GPIO Port SHUNt 1123 CONTrol Ch GPIO Port VOLTage DEFault 1124 CON...

Страница 1118: ...ue Sets or queries a channel dependent eight bit decimal value to control eight independ ent output signals at the User Port connector lines 8 9 10 11 and lines 16 17 18 19 The output signals are 3 3...

Страница 1119: ...lues correspond to the following states of the User Port connector 0 no signal at any of the no signal at any of the eight pins 8 9 10 11 16 17 18 19 1 output signal at pin 8 2 output signal at pin 9...

Страница 1120: ...easurement on the related GPIO pin or ALL GPIO pins Suffix Port 1 10 GPIO port number If ALL currents are queried this suffix is ignored Query parameters ALL ALL Use ALL to measure the currents at all...

Страница 1121: ...03 Manual operation See Meas on page 609 CONTrol Ch GPIO TIME MeasTime Sets the measurement time for the voltage current measurements on the RFFE and GPIO pins Same functionality as CONTrol Ch RFFE T...

Страница 1122: ...O pin The analyzer firmware automatically selects a suitable shunt resistance which can be queried using CONTrol Ch GPIO Port SHUNt Suffix Ch Channel number Port GPIO port number 1 10 Parameters Curre...

Страница 1123: ...d 10 have the same current range as pins 1 to 8 Suffix Ch Channel number Port GPIO port 1 10 Example CONTrol1 GPIO1 RANGe 20mA CONTrol1 GPIO1 SHUNt Returns 10 CONTrol1 GPIO1 RANGe 2mA CONTrol GPIO1 TE...

Страница 1124: ...unt settings to the RFFE GPIO pins Same function as CONTrol Ch GPIO VOLTage OUTPut Suffix Ch Channel number Usage Event Options R S ZN B15 Z15 Var 03 Manual operation See Set on page 607 CONTrol Ch RF...

Страница 1125: ...interface number Parameters Command 3 5 35 or 37 hexadecimal digits 0 F defining the com mand to be executed digit 1 is the slave address digits 2 and 3 specify the command number and the remaining d...

Страница 1126: ...COMMand DATA Suffix Ch Channel number Bus RFFE bus interface number Parameters EnableInSequence Enabled state Manual operation See Seq on page 604 CONTrol Ch RFFE Bus SETTings FREQuency ClockFrequenc...

Страница 1127: ...on the RFFE pins Note The voltages for DATA and CLOCk are always identical their values cannot be set independently The output voltages are applied using CONTrol Ch RFFE TEST OUTPut the volt age curre...

Страница 1128: ...ns R S ZN B15 Z15 Var 03 Manual operation See Range Shunt on page 606 CONTrol Ch RFFE Bus TEST CLOCk SHUNt CONTrol Ch RFFE Bus TEST DATA SHUNt CONTrol Ch RFFE Bus TEST VIO SHUNt Returns the shunt resi...

Страница 1129: ...RFFE Bus TEST DATA CURRent CONTrol RFFE Bus TEST CLOCk CURRent CONTrol RFFE Bus TEST VIO CURRent Returns the results of the current measurement on the related RFFE pin Suffix Bus RFFE bus Return valu...

Страница 1130: ...sequence steps and between the final sequence step and the sweep segment start The command switch sequences are defined using CONTrol Ch SEGMent Nr RFFE Bus COMMand DATA and CONTrol Ch SEGMent Nr GPIO...

Страница 1131: ...and 3 to 37 hexadecimal digits 0 F defining the command to be executed digit 1 is the slave address digits 2 and 3 specify the command number and the remaining digits represent the data part up to 17...

Страница 1132: ...rameters Delay Delay time Default unit s Manual operation See Wait Sweep Sequencer Table on page 612 CONTrol Ch SEQuence Nr GPIO Port VOLTage Voltage For unsegmented sweeps this command allows to defi...

Страница 1133: ...ve starting at 1 reusing a sequence number overwrites a previously defined command Delays between subsequent commands and finally the start of the respective sweep can be introduced using CONTrol Ch S...

Страница 1134: ...E Configuration on page 646 CONTrol VSE CONNect If CONTrol VSE LOCal on page 1134 is set to 1 the setting tries to connect to a local R S VSE Otherwise it tries to connect to a remote R S VSE whose VI...

Страница 1135: ...ce b wave on page 638 7 3 4 DIAGnostic commands The DIAGnostic commands provide access to service and diagnostic routines used in service maintenance and repair In accordance with the SCPI standard al...

Страница 1136: ...DumpSize NONE MINI NORMal LARGe FULL Either disables dump file creation NONE or determines the level of detail Manual operation See Error Dump Type on page 796 DIAGnostic PRODuct OPTion INFO Option D...

Страница 1137: ...ion See Service Function on page 811 7 3 5 DISPlay commands The DISPlay commands control the selection and presentation of graphical and trace information on the screen Trace display Traces are identi...

Страница 1138: ...Play WINDow Wnd TRACe WndTr LABel SHOW 1145 DISPlay WINDow Wnd TRACe WndTr SHOW 1145 DISPlay WINDow Wnd TRACe WndTr X OFFSet 1146 DISPlay WINDow Wnd TRACe WndTr Y SCALe AUTO 1146 DISPlay WINDow Wnd TR...

Страница 1139: ...indow Manual operation See Info Window on page 778 DISPlay IWINdow BFILter STATe TraceName BandfilterItem Boolean Defines the bandfilter search results to be displayed in the Info Window Parameters Bo...

Страница 1140: ...d Ch4Tr1 to measure the input reflection coefficient S11 DISP WIND2 STAT ON Create diagram area no 2 DISP WIND2 TRAC9 FEED CH4TR1 Display the generated trace in diagram area no 2 assigning the trace n...

Страница 1141: ...Manual operation See Title on page 771 DISPlay WINDow Wnd MAXimize Boolean Maximizes all diagram areas in the active recall set or restores the previous display configuration Suffix Wnd Number of the...

Страница 1142: ...name Example See DISPlay WINDow Wnd CATalog Manual operation See Title on page 771 DISPlay WINDow Wnd TITLe STATe Boolean Displays or hides the title for area number Wnd defined by means of DISPlay WI...

Страница 1143: ...agram on page 770 DISPlay WINDow Wnd TRACe WndTr DELete Releases the assignment between a trace and a diagram area as defined by means of DISPlay WINDow Wnd TRACe WndTr FEED TraceName and expressed by...

Страница 1144: ...DISP WIND2 STAT ON Create diagram area no 2 DISP WIND2 TRAC EFE CH4TR1 Display the generated trace in diagram area no 2 No trace number is assigned Usage Setting only DISPlay WINDow Wnd TRACe WndTr FE...

Страница 1145: ...ace Example RST DISP TRAC LAB SHOW Trc1 Reset the analyzer creating the default trace Trc1 The trace label is displayed the query returns 1 DISP TRAC LAB SHOW Trc1 OFF Hide the label of trace Trc1 Man...

Страница 1146: ...390 DISPlay WINDow Wnd TRACe WndTr X OFFSet StimulusOffset Shifts the trace WndTr in horizontal direction leaving the positions of all markers unchanged Suffix Wnd Number of an existing diagram area...

Страница 1147: ...lue between two grid lines and the reference value for the default trace The response is 10 0 DISP WIND TRAC Y AUTO ONCE PDIV RLEV or DISP WIND TRAC Y AUTO ONCE Trc1 PDIV RLEV Autoscale the default tr...

Страница 1148: ...0 or DISP WIND2 TRAC Y BOTT 40 CH4TR1 TOP 10 CH4TR1 Scale the diagram between 40 dB and 10 dB Manual operation See Max Min on page 383 DISPlay WINDow Wnd TRACe WndTr Y SCALe PDIVision VerticalDiv Trac...

Страница 1149: ...level or reference value for a particular displayed trace Setting a new reference level does not affect the value of PDIVision The trace can be refer enced either by its number WndTr or by its name Tr...

Страница 1150: ...he reference position is the point on the y axis which should equal the RLEVel Suffix Wnd Number of an existing diagram area defined by means of DISPlay WINDow Wnd STATe ON This suffix is ignored if t...

Страница 1151: ...aginaryPart Modifies all points of the trace WndTr by means of an added and or a multiplied complex constant The response values M of the trace are transformed according to Suffix Wnd Number of an exi...

Страница 1152: ...if the optional TraceName parameter is used Parameters Boolean Enable or disable the zoom OFF also restores the original dia gram size after a zoom function was applied OFF TraceName Optional string p...

Страница 1153: ...right border of the zoom window Range See description above RST Start or stop of the analyzer s sweep range Default unit NN TraceName Optional string parameter for the trace name e g Trc4 If this opti...

Страница 1154: ...de information element s RST ON Example RST DISP ANN TRAC OFF CHAN ON FREQ OFF Create diagram area no 1 with default trace and hide the trace list Keep the channel list but hide the swept frequency ra...

Страница 1155: ...colors are defined via DISPlay CMAP DispEl RGB where DispEl 9 12 The limit line colors are independent of the trace colors OFF all limit lines have the color of the associated trace RST n a a RST doe...

Страница 1156: ...DispEl Number of the display element as described in the table below Parameters Red Red content of the defined color Range 0 zero intensity corresponding to a 0 in the 24 bit color model to 1 full in...

Страница 1157: ...e marker color depends on the set tings made in previous sessions it is not reset A possible response is 0 0 0 for black markers DISP CMAP MARK OFF Change the marker colors M1 turns red M2 turns green...

Страница 1158: ...FEED Trc3 Create a new trace named Trc3 and display the trace in a new diagram area no 3 The new trace is not red Manual operation See General Trace Colors per Diagram on page 780 DISPlay CMAP TRACe R...

Страница 1159: ...diagrams are arranged one on top of the other HORizontal the diagrams are arranged in horizontal rows VERTical the diagrams are arranged in vertical rows GRID the diagrams are arranged as a rectangula...

Страница 1160: ...DISPlay LAYout EXECute to create more compli cated nested layouts Note The maximum number of diagrams in a layout is 256 Parameters LayoutFormatMode HORizontal VERTical Horizontal or vertical layout...

Страница 1161: ...Parameters LayoutData String parameter defining the number of diagrams and their position executable format see above Example See Creating diagrams Manual operation See Additional Functionality SCPI...

Страница 1162: ...in a single diagram which is maximized to occupy the whole screen Usage Event Manual operation See Overlay All on page 772 DISPlay LAYout SPLit Creates a separate diagram for each trace in the active...

Страница 1163: ...able identifiers Note that no error is generated if a valid MenuKey is specified but the tab cannot be activated for any other reason Setting parameters MenuKey Identifier of a softtool tab Example RS...

Страница 1164: ...bytes The numbers are separated by commas as specified in IEEE 488 2 REAL Data is transferred in a definite length block as IEEE floating point numbers of the specified Length See Chap ter 6 2 3 5 Blo...

Страница 1165: ...mands control the output of screen information to an external device Part of the functionality of this system is included in the File menu HCOPy IMMediate 1165 HCOPy DESTination 1166 HCOPy DEVice LANG...

Страница 1166: ...ile format see HCOPy DEVice LANGuage HCOP DEST MMEM HCOP Select Print to file and create the printer file specified before Manual operation See To File on page 710 HCOPy DEVice LANGuage Format Selects...

Страница 1167: ...not affect the printer configuration Example HCOP ITEM ALL Select the complete information to be printed HCOP ITEM LOGO ON HCOP Include the logo in the printed output and start printing HCOPy ITEM ML...

Страница 1168: ...previously configured using DISPlay CMAP commands DBACkground Dark background LBACkground Light background BWLStyles Black and white with white background and different line styles BWSolid Black and...

Страница 1169: ...FT 10 RIGHT 10 Set an left and a right margin of 1 cm HCOPy PAGE MARGin RIGHt RightMargin Defines the distance between the right edge of the page and the right edge of the prin ted information Paramet...

Страница 1170: ...am per page ACTive print only active diagram HARDcopy print a screenshot of the diagram area preserving layout and colors real screenshot NONE print no diagram at all RST n a RST does not affect the p...

Страница 1171: ...eps defined via SENSe Ch SWEep COUNt INITiate Ch IMMediate DUMMy initiates a new measurement cycle RST ON Example See INITiate Ch CONTinuous Manual operation See All Channels Continuous All Channels o...

Страница 1172: ...all channels or in the referenced channel Ch only Example See INITiate Ch CONTinuous Manual operation See Sweep All Channels Sweeps on page 514 INITiate Ch CONTinuous Boolean Qualifies whether the ana...

Страница 1173: ...s also stopped after the current sweep INIT ALL Re start a single sweep in both channels Example Alternative settings using the R S ZVAB compatibility mode RST SYSTem LANGuage ZVABT RST INIT CONT OFF...

Страница 1174: ...el no 2 and select it as the active channel The query returns 2 INSTrument PORT COUNt Returns the number of test ports Port 1 Port 2 of the analyzer Example INST PORT COUN Return the number of ports o...

Страница 1175: ...he active recall set Set_2 to a file renaming it Set_2 znxml Close the setup Usage Query only MEMory CATalog COUNt Returns the number of loaded recall sets Usage Query only MEMory DEFine Name Creates...

Страница 1176: ...11 MMEMory commands The MMEMory commands provide mass storage capabilities for the analyzer Internal and external mass storage The mass storage of the analyzer can be internal or external The interna...

Страница 1177: ...R S ZVA B default path C Rohde Schwarz Nwa is replaced by C Users Public Documents Rohde Schwarz ZNA MMEMory AKAL FACTory CONVersion 1178 MMEMory AKAL USER CONVersion 1179 MMEMory CATalog 1179 MMEMor...

Страница 1178: ...MMEMory STORe EYE MASK 1207 MMEMory STORe EYE MASK RESults 1208 MMEMory STORe EYE MEASurements 1208 MMEMory STORe LIMit 1209 MMEMory STORe MARKer 1209 MMEMory STORe RIPPle 1210 MMEMory STORe SEGMent...

Страница 1179: ...cal kit file If an empty string is specified the factory cal kit file stored in the active calibration unit SYSTem COMMunicate RDEVice AKAL ADDRess is used By default this file is also used in manual...

Страница 1180: ...ctory Returns the contents of the current or of a specified directory and all subdirectories The information is returned in the following format Directory of Directory Used Size FreeDiskSpace FileEntr...

Страница 1181: ...CalKitFile Detail Queries connector type name label and gender of a cal kit defined in the specified cal kit file Query parameters CalKitFile Path to the cal kit file either absolute or relative to t...

Страница 1182: ...r Example MMEM DATA C Users Public TEST01 HCP Query the block data contained in file TEST01 HCP MMEMory DELete File Force Removes a file from the specified directory Setting parameters File Mandatory...

Страница 1183: ...EMory CDIRectory The empty string represents an empty position in the favorites list Note that when a non empty favorite is set the target recall set must exist Example MMEMory CDIRectory DEFault MMEM...

Страница 1184: ...ple MMEM LOAD CKIT C Users Public Documents Rohde Schwarz ZNA Calibration Kits New_kit calkit Load the previously created cal kit file New_kit calkit from the default cal kit directory MMEM STOR CKIT...

Страница 1185: ...ry RST FirstPort First port number sufficient for one port standards If the port numbers are omitted the cal kit data is valid for all ports SecondPort Second port number for two port standards If the...

Страница 1186: ...tandard types for a description refer to table Standard types and their parameters StandardLabel String parameter addressing a particular calibration standard by its label An empty string means that n...

Страница 1187: ...parately MMEMory MDIRectory An empty string means that no cal kit files will be loaded Example MMEM LOAD CKIT UDIR C Users Public Documents Rohde Schwarz ZNA Calibration Kits Autoload Specifiy the dir...

Страница 1188: ...existing channel ALL applies the selected data set to all channels CalGroupFile String parameter to specify the name of the cal group file to be loaded Cal group files must have the extension cal The...

Страница 1189: ...lt path C Users Public Documents Rohde Schwarz ZNA CALibration DATA Example SENS1 CORR COLL METH DEF Test FRTRans 1 3 Select a bidirectional transmission normalization between ports 1 and 3 as a calib...

Страница 1190: ...rec tory must not be specified the analyzer always uses the default path C Users Public Documents Rohde Schwarz ZNA Calibration Data If there is no link between Ch and the specified file the command h...

Страница 1191: ...ace Optional string parameter For multiport Touchstone files snp n 1 the parameter refers to a particular S parame ter trace S11 S12 For ASCII csv files the parameter references a trace name in the fi...

Страница 1192: ...ame of the jitter file If the path is omitted the current directory is used see MMEMory CDIRectory Usage Setting only Options R S ZNA K20 Manual operation See User Specific on page 748 MMEMory LOAD EY...

Страница 1193: ...hes the current directory to be queried with MMEMory CDIRectory See also note on Touchstone files above TouchstoneFile String parameter selects an S parameter from a Touchstone file The parameter must...

Страница 1194: ...created Touchstone limit line file and assign the limit lines to Trc1 applying a response offset of 2 dB CALC LIMit DISPlay ON Show the limit line in the diagram Usage Setting only Manual operation Se...

Страница 1195: ...ep segment definition loaded from the specified ASCII file Setting parameters Channel Channel number SweepSegFile String parameter to specify the name and directory of the sweep segment file to be loa...

Страница 1196: ...e Loads trace data from a specified trace file and assigns it to a trace with a specified name Traces are created using the CALCulate Ch PARameter SDEFine com mand Setting parameters Destination Trace...

Страница 1197: ...ce assigned to Trc1 with the S11 trace Usage Setting only Manual operation See Import Data to New Mem on page 422 MMEMory LOAD TRACe AUTO TraceFile Loads the specified trace file and automatically dis...

Страница 1198: ...y Port PMAin PSECondary Assigns a 2 port s2p file to the appropriate port PMAin cor responds to D1 PSECondary to D2 in the circuit model picto grams For an s4p file this parameter is ignored However i...

Страница 1199: ...EMBedding LogPt PARameters DATA Port to load circuit data from the remote client instead The query returns the name of the loaded file Suffix Ch Channel number LogPt Logical port number of a balanced...

Страница 1200: ...O TND FIMP Select the 1 Port Data s1p circuit model MMEM LOAD VNET GLO EMB C Users Public Documents Rohde Schwarz ZNA Embedding Test s1p Load a Touchstone file Manual operation See File Name 1 on page...

Страница 1201: ...the Touchstone file in the context of the deembedding network FPORts or omitted Standard interpretation odd port numbers towards VNA even port numbers towards DUT IPORts For s2p files IPORts means in...

Страница 1202: ...e and directory of the loaded Touchstone file If no path is specified the analyzer searches the current direc tory which can be set and queried using MMEMory CDIRectory Interchange FPORts IPORts SGATe...

Страница 1203: ...ithout mov ing it Setting parameters SourceFile NewFile String parameters to specify the name and the path of the file to be copied and the name and the path of the new file Example MMEM MOVE C Users...

Страница 1204: ...ecified directory must exist otherwise no file can be generated If no path is specified the analyzer uses the current directory to be queried with MMEMory CDIRectory RST Hardcopy Example MMEM NAME C U...

Страница 1205: ...CalKitFile String parameter to specify the name and directory of the cal kit file to be created The file is a network analyzer specific cal kit file with the extension calkit If no path is specified...

Страница 1206: ...cheme file to be created If no path is specified the analyzer uses the current directory to be queried with MMEMory CDIRectory The default extension manual control for color scheme files is ColorSchem...

Страница 1207: ...operation See Load Save on page 834 MMEMory STORe CORRection TCOefficient Ch PmclFile Saves the power meter two port transmission coefficients of channel Ch to a power meter correction list file Suff...

Страница 1208: ...e file If the path is omitted the current directory is used see MMEMory CDIRectory Usage Setting only Options R S ZNA K20 Manual operation See Export Test Results on page 753 MMEMory STORe EYE MEASure...

Страница 1209: ...cify the name and directory of the cre ated limit line file The default extension manual control for limit line files is limit although other extensions are allowed If no path is specified the analyze...

Страница 1210: ...are created using the CALCulate Chn RIPPle commands Setting parameters TraceName Name of an existing trace in the active setup string parameter for which a ripple limit definition exists RippleLimFil...

Страница 1211: ...ters File String parameter containing the path and file name of the lev file If the path is omitted the current directory is used see MMEMory CDIRectory Usage Setting only Options R S ZNA K8 Manual op...

Страница 1212: ...ctory of the cre ated trace file Several file formats for trace files are supported The file extensions s n p csv and dat for Touch stone ASCII and Matlab files are mandatory To generate a multiport T...

Страница 1213: ...ate file is created for each channel in the active recall set TraceFile String parameter to specify the name and directory of the cre ated trace file Several file formats for trace files are supported...

Страница 1214: ...arz ZNA Traces Chn1 csv Store all trace data of channel 1 to a trace file MMEM STOR TRAC CHAN 1 C Users Public Documents Rohde Schwarz ZNA Traces Chn1 s2p Store the four S parameter traces to a two po...

Страница 1215: ...rmat to be generated during Touchstone export Parameters TS_Frmt F11 F20 L11 F11 Trace output according to Touchstone standard 1 1 new library F20 Trace output according to Touchstone standard 2 0 new...

Страница 1216: ...rts If the specified physical ports comprise at least one balanced port and MMEMory STORe TRACe OPTion BALanced is ON the Touchstone file contains the full set of mixed mode S parameters Otherwise it...

Страница 1217: ...to the common target impedance and store them to a two port Touchstone file MMEM STOR TRAC PORT 1 Test_PIMP s2p COMPlex PIMPedance 1 2 Calculate all single ended S parameters renormalize them to to th...

Страница 1218: ...internal source power at all ports and the power of all external generators on or off Suffix Ch Channel number This suffix is ignored the setting is valid for all channels Parameters Boolean ON OFF s...

Страница 1219: ...ween up to 16 inde pendent analyzer states for each channel The command is valid for segmented fre quency sweeps It is analogous to the channel dependent command OUTPut Ch UPORt VALue The bits for the...

Страница 1220: ...l at pin 17 B0011 output signal at pin 16 and 17 B1111 output signal at pin 16 17 18 and 19 Range B0000 to B1111 for setting command 0 to 15 query RST B0000 0 Example RST SEGM INS 1MHZ 1 5MHZ 111 21DB...

Страница 1221: ...on zero channel bits is started They are changed whenever a channel with differ ent channel bits becomes the measuring channel The signals at the USER PORT connector are maintained after the analyzer...

Страница 1222: ...nual operation See Channel Bits Decimal on page 795 7 3 13 PROGram commands The PROGram commands control external application programs that can be run on the analyzer PROGram SELected EXECute 1222 PRO...

Страница 1223: ...script Exit42 bat PROGram SELected WAIT Lock command execution and manual control of the analyzer until the batch job has finished This is required for PROGram SELected RETVal Get the return value Th...

Страница 1224: ...by PROGram SELected EXECute The query must be sent with a single Key value It reads the value associated with the key from the PARAMETER section of the preferences file specified by file_path If the...

Страница 1225: ...preceded by a PROGram SELected WAIT query see PROGram SELected WAIT on page 1225 Example See PROGram SELected EXECute Usage Query only PROGram SELected WAIT Locks command execution from the current co...

Страница 1226: ...pecified number of sweeps SENSe Ch AVERage COUNt RST ON Example See SENSe Ch AVERage CLEar Manual operation See Factor On Reset on page 487 SENSe Ch AVERage CLEar Starts a new average cycle clearing a...

Страница 1227: ...moving averages of the real and imaginary parts of each measurement result provides the most effective noise sup pression for the Real and Imag formats and for complex trace formats MOVing Simple mov...

Страница 1228: ...e RST 10 kHz Default unit Hz Example BAND 1 1 Set a IF bandwidth of approx 1 1 Hz for channel 1 BAND The analyzer returns the rounded bandwidth of 1 5 Hz Manual operation See Bandwidth on page 485 SEN...

Страница 1229: ...asks and traces SENSe CDLL ADD DllPath Tells the analyzer firmware to load a custom external DLL The DLL and its accompanying files must be packed in a zip archive It will be loaded on every subsequen...

Страница 1230: ...DllName Name of a loaded DLL see SENSe CDLL LIST Lowercase string without dll extension Usage Query only SENSe Ch CDLL PERManent ADDitional DllName Additional Input SENSe Ch CDLL PERManent ADDitional...

Страница 1231: ...NSe Ch CDLL PERManent STATe DllName Boolean SENSe Ch CDLL PERManent STATe DllName Defines whether DLL DllName shall operate in permanent mode in channel Ch Use SENSe Ch CDLL PERManent TASK and SENSe C...

Страница 1232: ...provide traces or operate in permanent mode see SENSe Ch CDLL PERManent STATe Suffix Ch Channel number Parameters Boolean ON 1 DLL is active OFF 0 DLL is inactive Parameters for setting and query Dll...

Страница 1233: ...s Type The name of the new converter type Must be unique among the existing ones see SENSe CONVerter DEFinition CATalog Example SENSe CONVerter DEFinition DEFine My converter type creates a new conver...

Страница 1234: ...he fixed intermediate frequency of the related converter type Parameters IF Intermediate frequency Default unit Hz Parameters for setting and query Type Name of the converter type whose IF is set quer...

Страница 1235: ...Nerator Gen LEVel OFFSet Parameters LocalNomPowerCalOffset Default unit dBm Parameters for setting and query Type Name of the converter type whose cal power offset is set quer ied Options R S ZNA K8 S...

Страница 1236: ...Se CONVerter DEFinition SPOWer OFFSet Type SourceNomPowerCalOffset SENSe CONVerter DEFinition SPOWer OFFSet Type Sets queries the cal power offset of the source port that is connected to the RF In por...

Страница 1237: ...tter i on page 830 SENSe CONVerter SPLitter Port LOSS Loss Defines the cable and splitter loss for splitter no Port Suffix Port Splitter number Parameters Loss Loss value Default unit dB Options R S Z...

Страница 1238: ...ptional direct IF access connectors IF Reference i and IF Meas i at the rear panel of the R S ZNA Options R S ZNA K8 Manual operation See IF Input on page 829 SENSe CONVerter Port IFRequency IF Sets q...

Страница 1239: ...nal LO Out port at the rear panel of the R S ZNA PORT A VNA port GENerator An external generator port SPLitter A splitter is used to distribute the original LO signal to the LO In ports of the involve...

Страница 1240: ...er number VNA port number Parameters Boolean Options R S ZNA K8 Manual operation See Active on page 829 7 3 14 5 SENSe Ch CORRection ADVanced Remote control commands for advanced calibration features...

Страница 1241: ...ion POWer PhyPt HARMonic ACQuire to calibrate Suffix Ch Channel number Setting parameters Order Order number Usage Setting only Options R S ZNA K4 Manual operation See Harmonic Orders to Calibrate on...

Страница 1242: ...POWer PhyPt STATe Boolean Suffix Ch PhyPt Parameters Boolean Manual operation See Cal Power on page 539 SENSe Ch CORRection ADVanced SENSe PhyPt ATTenautor Attenuation Suffix Ch PhyPt Parameters Atten...

Страница 1243: ...l operation See Preamp Gain on page 539 SENSe Ch CORRection ADVanced SENSe PhyPt PREamp STATe Boolean Suffix Ch PhyPt Parameters Boolean Manual operation See Preamp Gain on page 539 SENSe Ch CORRectio...

Страница 1244: ...AVE SELected DEFault For an overview of calibration methods and error terms refer to Chapter 4 5 1 Calibra tion types on page 160 G and H matrices The 7 term calibration types named Txx e g TOM TRM TR...

Страница 1245: ...urce port SourcePort LOADMATCH Load match at load port LoadPort TRANSTRACK Transmission tracking between source port SourcePort and load port LoadPort G11 G22 G matrix elements at source port SourcePo...

Страница 1246: ...MATCH REFLTRACK TRANSTRACK TOSM TOSM DIRECTIVITY SRCMATCH REFLTRACK LOADMATCH TRANSTRACK TOM TSM TRM TRL TNA TOM TSM TRM TRL TNA DIRECTIVITY SRCMATCH REFLTRACK LOADMATCH TRANSTRACK for reading and wri...

Страница 1247: ...e See SENSe CORRection CKIT LABel Usage Setting only Manual operation See Add Copy Delete Standards on page 563 SENSe CORRection CKIT DMODe ConnectorType CalKitName KitLabel Mode Sets gets the delay m...

Страница 1248: ...t 1 0 4000000000 0 0 50 99 2 3 0 22 0 0 0 0 0 Create a new cal kit New Kit 1 and assign an open f standard for the N 50 connector type with specific properties CORR CKIT LAB New Kit 1 Test kit created...

Страница 1249: ...ATalog to query cal kit names Example See SENSe Ch CORRection CONNection CATalog Usage Query only SENSe CORRection CKIT STANdard DATA ConnectorType CalKitName KitLabel StandardType DelayMode Port1 Por...

Страница 1250: ...ed by its name Tip For connector types with arbitrary user defined names you can use the command SENSe CORRection CKIT SELect Cal kit naming conventions Calibration kit names must be entered as string...

Страница 1251: ...g on the standard type only a subset of the parameters is used see Table 7 13 Note As the query does not reflect the delay mode specified using SENSe CORRection CKIT DMODe it is deprecated and only av...

Страница 1252: ...ample CORR CKIT FOP N 50 Ohm New Kit 1 0 4000000000 0 0 50 99 2 3 0 22 0 0 0 0 0 Define the properties of the Open f standard for a N 50 con nector type in cal kit New Kit 1 CORR CKIT FOP N 50 Ohm Que...

Страница 1253: ...ame of the calibration kit Use SENSe CORRection CKIT CATalog to query cal kit names String parameter CalKitLabel Label e g the serial number of the calibration kit for WLABel com mand only String para...

Страница 1254: ...rameters Port restrictions are indicated in brackets Table 7 15 Standard types and their parameters std_type Meaning MOPen FOPen Open male m or female f MSHort FSHort Short m or f OSHort 1 2 3 MOSHort...

Страница 1255: ...Example See SENSe Ch CORRection CONNection CATalog Usage Query only SENSe CORRection CKIT LDELete CalKitName KitLabel Deletes an imported or user defined cal kit which is identified by its cal kit na...

Страница 1256: ...or the labelled cal kit to the cal kit file New Kit 1 2012 05 25 calkit CORR CKIT LDEL New Kit 1 2012 05 25 Delete the kit from the internal memory MMEMory LOAD CKIT C Users Public Documents Rohde Sch...

Страница 1257: ...IT ConnType LSELect CalKitName Label Selects the calibration kit to be used for a specified connector type ConnType The kit is identified by its name and label Tip For connector types with arbitrary u...

Страница 1258: ...a non ideal 1 port or 2 port calibration standard StandardType where a particular cal kit can be addressed by name and label Apart from the additional CalKitLabel parameter the syntax and semantics of...

Страница 1259: ...l kit standard previously loaded from Touchstone file using MMEMory LOAD CKIT SDATa or MMEMory LOAD CKIT SDATa WLABel The cal kit is identified by its name and label Query parameters TwoPortStandard M...

Страница 1260: ...e on page 591 SENSe Ch CORRection METas CABLe MOVement Ports Ports While a METAS calibration is active for the related channel see SENSe Ch CORRection METas STATe on page 1261 this command notifies th...

Страница 1261: ...CABLe CATalog to list the available cable types Suffix Ch Channel number The related channel must be prepared for a METAS calibration using SENSe Ch CORRection COLLect METHod DEFine CalName MTOSm MUO...

Страница 1262: ...ection COLLect DETector 1271 SENSe CORRection COLLect FIXTure LMParameter STATe 1271 SENSe CORRection COLLect FIXTure LMParameter LOSS STATe 1271 SENSe CORRection COLLect PMETer ID 1272 SENSe CORRecti...

Страница 1263: ...ND 1300 SENSe Ch CORRection COLLect NFIGure SAVE 1300 SENSe Ch CORRection COLLect NFIGure STARt 1301 SENSe Ch CORRection COLLect NFIGure ACQuire 1301 SENSe Ch CORRection DELete 1302 SENSe Ch CORRectio...

Страница 1264: ...unit if available Note that this command can only be executed if the number of cal unit ports is less or equal to the number of test ports Furthermore the command assumes the canonical assignment of...

Страница 1265: ...cal unit characterization Setting parameters Password Password string parameter as defined in manual control Example SENSe CORRection COLLect AUTO CKIT PASSword My_password Enter a password My_passwor...

Страница 1266: ...nly Manual operation See Test Port Assignment on page 570 SENSe CORRection COLLect AUTO CKIT PORTs ADD Characterization TestPort1 CalUnitPort1 TestPort2 Extends or modifies an existing characterizatio...

Страница 1267: ...nnect Enables or disables the Reduced Through logic for every port assignment of an auto matic calibration Note that for this to work the port assignments must have a star topology with a Common Port...

Страница 1268: ...ic averaging which means that the VNA may perform multiple cali bration sweeps and apply averaging to reduce trace noise In contrast to manual aver aging see SENSe Ch AVERage STATe the number of calib...

Страница 1269: ...f the available channels using channel specific ports and calibration types see Chap ter 4 5 8 Parallel calibration of multiple channels on page 200 Enable the MCTYpes mode before defining the calibra...

Страница 1270: ...ion in channel 1 Then connect the Open standard to port 2 SENSe CORRection COLLect ACQuire SELected OPEN 2 Acquire calibration data for the open on port 2 needed for cali bration in channel 2 Then con...

Страница 1271: ...ample detector recommended for all other applica tions RST NORMal Manual operation See Detector on page 590 SENSe CORRection COLLect FIXTure LMParameter STATe Boolean Selects an Auto Length and Loss c...

Страница 1272: ...of the analyzer TestPort Test port number During power calibration the power meter is connected to this port RST 1 to the number of test ports Example See SENSe Ch CORRection COLLect AUTO POWer Manual...

Страница 1273: ...sition until position 20 has been recorded Subsequent calls will start over at position 1 overwriting the previously acquired data Suffix Ch Channel number of the calibrated channel Setting parameters...

Страница 1274: ...is entered the analyzer uses the calculated value which is closest to the estimate Automatic determination of the phase The UOSM algorithm provides the transmission factor of the unknown through stan...

Страница 1275: ...rization Location of the characterization cal kit file to be used for the automatic calibration string parameter If an empty string is specified the factory cal kit file stored in the active calibrati...

Страница 1276: ...signments were established using SENSe Ch CORRection COLLect AUTO ASSignment DEFine DEFault See Chapter 4 5 5 6 Multiple port assignments on page 189 for background infor mation Note In MultiCal scena...

Страница 1277: ...page 1278 Usage Setting only Manual operation See CalUnit Con on page 533 SENSe Ch CORRection COLLect AUTO ASSignment DEFine TPORt DEFault TestPort1 TestPort2 Similar logic as SENSe Ch CORRection COL...

Страница 1278: ...Event Manual operation See Start Cal Sweep Abort Sweep on page 525 SENSe Ch CORRection COLLect AUTO ASSignment Asg DEFine TestPort1 CalUnitPort1 TestPort2 Manually defines port assignment no Asg for c...

Страница 1279: ...zation SENSe1 CORRection COLLect AUTO ASSignment1 DEFine 2 1 3 2 Creates port assignment 1 explicitely no auto detection SENSe1 CORRection COLLect AUTO ASSignment2 DEFine TPORt 3 4 Creates port assign...

Страница 1280: ...the corresponding calibration sweep Suffix Ch Number of the channel Asg Number of the port assignment Parameters TestPort1 First test port number TestPort2 Second test port number Example See SENSe C...

Страница 1281: ...l n port FNPort calibration with additional power flatness calibration for SMARTerCal VUOSm FNPort UOSM Vector Mixer calibration VPUOSm PFNPort PUOSM Vector Mixer calibration CPUosm PFNPort PUOSM Scal...

Страница 1282: ...PORt DEFault on page 1277 If SENSe CORRection COLLect AUTO MCONnect is ON this port will be used as the center of all Through measurements If you set up the port assignments manually make sure that ev...

Страница 1283: ...n the active calibration unit is used A VNA cal kit file name calkit without path refers to a specific cal kit file stored in the internal memory of the active cal ibration unit A VNA cal kit file nam...

Страница 1284: ...M calibration TOSM Full n port calibration with characterized Through If the selected cal unit characterization does not contain a Through characterization the command silently falls back to a FNPort...

Страница 1285: ...rs must be specified For a one path two port calibration OPTPort the first port no denotes the source port fully calibrated port CalUnitPort1 Port numbers of the cal unit For an n port automatic calib...

Страница 1286: ...ration at Port 2 This completes the Usage Setting only Manual operation See Start Cal Sweep Abort Sweep on page 525 SENSe Ch CORRection COLLect AUTO REPeat Use this command to reuse measurement data o...

Страница 1287: ...ual operation See Temperature Compensation on page 534 SENSe Ch CORRection COLLect AUTO TYPE CalType Characterization TestPort1 TestPort2 Selects and initiates an automatic calibration at arbitrary an...

Страница 1288: ...PFNPort Full n port FNPort calibration with additional power flatness calibration for SMARTerCal VUOSm FNPort UOSM Scalar Vector Mixer calibration CPUosm PFNPort PUOSM Scalar Vector Mixer calibration...

Страница 1289: ...ected using SYSTem COMMunicate RDEVice AKAL ADDRess is an inline calibration sys tem ICS Suffix Ch Channel number Setting parameters TestPort1 TestPort2 VNA test port numbers Example See Chapter 8 2 5...

Страница 1290: ...or ALL optional Usage Setting only SENSe Ch CORRection COLLect CKIT PORT PhyPt Detail Queries the cal kit data assigned to the given port via SENSe Ch CORRection COLLect CKIT LOAD or SENSe Ch CORRecti...

Страница 1291: ...user defined connector type UserConn2 The user defined connector types must be defined before being addressed by SENSe Ch CORRection COLLect CONNection PhyPt RST N50FEMALE for all ports Example RST CO...

Страница 1292: ...or types at the ports RST ALL Example CORR COLL CONN PORTS SING Select independent connector types at the ports CORR COLL CONN1 N50MALE CONN4 N75FEMALE CONN2 Select independent connector types at port...

Страница 1293: ...OLL SEL SHOR 1 Measure a short standard connected to port 1 and store the measurement results of this standard CORR COLL SAVE SEL CORR COLL DEL ALL Calculate the system error correction data and apply...

Страница 1294: ...at port 2 terminated with an open standard and at port 4 terminated with a short CORR COLL FIXT SAVE Save and apply the compensation data CORR COLL FIXT STAR Prepare a new fixture compensation measure...

Страница 1295: ...nsation data files are standard Trace files containing reflection parame ter traces for the related port s and standard Table 7 16 Direct Compensation data TestPorts File Type 1 s1p 1 csv For 1 port T...

Страница 1296: ...n See Measure Fixture wizard on page 665 SENSe Ch CORRection COLLect FIXTure SAVE Completes a fixture compensation storing and applying the acquired data Suffix Ch Channel number of the corrected chan...

Страница 1297: ...Lect LOAD SELected CalGroupFile SLIDe TestPort for the same cal group file and test port implicitly increases the position until position 20 has been recorded Subsequent calls will start over at posit...

Страница 1298: ...Fine CalName CalType TestPort1 TestPort2 TestPort3orAdapterCalKit TestPorts Defines the calibration to be performed for channel Ch If multiple channels shall be calibrated in parallel use SENSe CORRec...

Страница 1299: ...Ch CORRection COLLect SAVE SELected DUMMy TestPorts More ports to be calibrated Example SENSE1 CORRECTION COLLECT METHOD DEFine Test ARTosm 1 2 Adapter s2p Initiate an Adapter Removal calibration for...

Страница 1300: ...he same channel number Example See Chapter 8 2 Condensed programming examples on page 1599 Usage Event Options R S ZNA K30 SENSe Ch CORRection COLLect NFIGure SAVE Completes a noise figure calibration...

Страница 1301: ...se calibra tion arg4 Attenuation of the source level during the measurement i e after calibration Example See Chapter 8 2 Condensed programming examples on page 1599 Usage Setting only Options R S ZNA...

Страница 1302: ...Channel number Usage Event Manual operation See Manage Cals Remove all Cal Data on page 581 SENSe Ch CORRection COLLect SAVE SELected DEFault Generates a set of default system error correction data f...

Страница 1303: ...Usage Event Manual operation See Apply on page 522 SENSe Ch CORRection COLLect SAVE SELected DUMMy Calculates the system error correction data from the acquired measurement results SENSe Ch CORRection...

Страница 1304: ...ng conventions under SENSe CORRection CKIT ConnType SELect RST N 50 Ohm FEM for all ports Gender MALE FEMale Gender of the connectors The gender designation is not neces sary and ignored for sexless c...

Страница 1305: ...Hz to 1000 GHz RST Example CORR CONN USERCON TEM GEND 1 00000 50 Define a TEM type connector type named USERCON CORR CONN USERCON Query the properties of the configured connector type CORR CONN DEL U...

Страница 1306: ...012 05 25 A sequence of two commas means that the preceding cal kit has no label assigned CORRection CKIT STANdard CATalog 85032B E Query standards in cal kit named 85032B E Possible response MOP FOP...

Страница 1307: ...option R S ZNA K4 Query parameters SfkSettingType ACAL STARt STOP POINts SPOWer STYPe BANDwidth PDLY RATTenuation TYPE PORTs SPORt THRoughs TSTamp LTSTamp TVNA MVNA MTESt CKIT FSMode The requested se...

Страница 1308: ...cal kit name string if available and unique Multiple if cal kit names are available but not unique i e if PhyPt was not specified and multiple cal kits were used multi port cals with different connect...

Страница 1309: ...f this standard CORR COLL SAVE Calculate the system error correction data and apply it to the active channel CORR DATE Query the time when the system error correction became active The analyzer return...

Страница 1310: ...ty Permittivity Range 1 to 1E 6 RST 1 00062 Example See SENSe Ch CORRection EDELay PhyPt ELENgth Manual operation See Permittivity Velocity Factor on page 663 SENSe Ch CORRection EDELay PhyPt DISTance...

Страница 1311: ...29990704322 1 00062 CORR EDEL2 Query the value of the delay at port 2 The delay is equal to the electrical length divided by the speed of light in the vacuum so the response is 1 0006922856E 009 CORR...

Страница 1312: ...ave Correction on page 316 SENSe Ch CORRection LOSS PhyPt LossAtFrequency SENSe Ch CORRection LOSS PhyPt SECond LossAtFrequency These commands define the offset loss at the reference frequencies f1 an...

Страница 1313: ...nding losses can be defined using SENSe Ch CORRection LOSS PhyPt OFFSet Suffix Ch Channel number PhyPt Physical port number Parameters RefFreq Reference frequency Range Frequency range of the analyzer...

Страница 1314: ...sical port number Parameters Boolean OFF 0 f2 not used default ON 1 f2 used Manual operation See Loss at DC Loss at 1st Freq 1st Freq for Loss Use 2nd Freq Loss at 2nd Freq 2nd Freq for Loss on page 6...

Страница 1315: ...SENSe Ch CORRection OFFSet PhyPt COMPensation STATe Boolean Toggles length loss fixture compensation for physical port PhyPt ON OFF Suffix Ch Channel number PhyPt Physical port number Parameters Boole...

Страница 1316: ...bration is applied the response is 0 Usage Setting only Manual operation See Scalar Power Cal All Power Cals On All Power Cals Off on page 581 SENSe Ch CORRection POWer DATA Wave CorrData SENSe Ch CO...

Страница 1317: ...tted source power a1 is correct No external cabling is needed CORR POW DATA AWAV1 Query the correction values The analyzer returns 10 comma separated real numbers CORR POW DATA AWAV1 1 2 3 4 5 6 7 8 9...

Страница 1318: ...ave CalPort SourceType SourcePort CalOnlyPortFreq Selects the wave quantity and the source for the receiver power calibration starts the calibration sweep and applies the receiver power correction Suf...

Страница 1319: ...whether the calibration is applied the response is 1 CALC PAR MEAS TRC1 A1 Select a1 as a measured quantity for the default trace CALC MARK ON MARK Y SOUR POW Create marker no Mk in the center of the...

Страница 1320: ...re Suffix Ch Channel number PhyPt Port number Example See SOURce Ch POWer PhyPt CORRection HARMonic ACQuire on page 1443 Usage Event Manual operation See Harmonic Orders to Calibrate on page 536 SENSe...

Страница 1321: ...es the stimulus values of the active calibration A calibration must be selected before the command is executed see example Suffix Ch Channel number of the calibrated channel PhyPt Physical port number...

Страница 1322: ...11 SENSe COUPle The SENSe COUPle commands select the sweep mode SENSe Ch COUPle Mode Determines the order of partial measurements and sweeps see Chapter 4 1 4 1 Par tial measurements and driving mode...

Страница 1323: ...EQuency COMPression RECeiver 1326 SENSe Ch FREQuency CENTer 1326 SENSe Ch FREQuency CONVersion 1327 SENSe Ch FREQuency Pt CONVersion ARBitrary 1327 SENSe Ch FREQuency CONVersion ARBitrary PMETer Pmtr...

Страница 1324: ...FREQuency on page 1323 Note SENSe Ch FREQuency CW FIXed is equivalent to SOURce Ch FREQuency PhyPt CW FIXed Source and receiver frequency are always equal the four commands overwrite each other With...

Страница 1325: ...trace named Trc1 in diagram 1 CALCulate PARameter SDEFine Trc2 CmpPtPout Define trace Trc2 as the compression point output DISPlay WINDow1 TRACe2 FEED Trc2 Show trace Trc2 as trace no 2 in diagram 1...

Страница 1326: ...ual operation See Driving Port Receiving Port on page 348 SENSe Ch FREQuency CENTer CenterFreq Defines the center of the measurement and display range for a frequency sweep sweep range The default cen...

Страница 1327: ...see example ARBitrary Frequency conversion mode arbitrary port frequencies This mode is automatically activated when a port specific frequency is defined MIXer Scalar mixer mode The mixer mode is con...

Страница 1328: ...cified numerator is rounded to the next higher integer zero is not allowed RST 1 Denominator The specified denominator must be positive It is rounded to the next higher integer RST 1 Offset The specif...

Страница 1329: ...tor and Offset values are rounded to positive or negative integer numbers zero is not allowed Note The default frequency or frequency range corresponds to the sweep range or CW frequency of the analyz...

Страница 1330: ...ormula Defines the frequencies of the a wave receivers i e the frequencies to which the receivers of port Pt are set while this port is the source port Suffix Ch Channel number Pt Port number Paramete...

Страница 1331: ...rs Boolean RST ON Example See SOURce Ch FREQuency PhyPt CONVersion ARBitrary IFRequency Manual operation See Load Match Correction on page 580 SENSe Ch FREQuency CONVersion MIXer FIXed Stg InputOutput...

Страница 1332: ...RF Example See SENSe Ch FREQuency CONVersion MIXer MFFixed Manual operation See RF IF and Mixer Stages on page 365 SENSe Ch FREQuency CONVersion MIXer IFPort PortNumber Selects an analyzer port as rec...

Страница 1333: ...GENerator NONE NONE LO signal is not controlled by the analyzer PORT analyzer port GENerator external generator RST NONE SourceNumber Number of analyzer port or generator Example See SENSe Ch FREQuenc...

Страница 1334: ...ort for the local oscillator LO1 port 3 as a source for LO 2 FREQ CONV MIX RFMultiplier 2 1 LOMultiplier1 2 1 LOMultiplier2 2 1 Define frequency conversion factors 2 for the RF signals and the LO sign...

Страница 1335: ...factors on page 364 SENSe Ch FREQuency CONVersion MIXer RFPort Port Selects a test port as source port for the RF signal Suffix Ch Channel number Parameters Port Test port number Range 1 to number of...

Страница 1336: ...xample RST FREQ CONV MIX LOF 3 GHz Reset the analyzer and specify a fixed frequency of 1 GHz to be assigned to the LO signal FREQ STAR 1 GHz STOP 2 GHz Define a sweep range between 3 and 4 GHz to be a...

Страница 1337: ...IMOD PORT COMBINER Select Extenal Device as a source port for the two tone signal FREQ IMOD REC 2 Select port 2 as the receiver port for the intermodulation mea surement FREQ IMOD TDIS 2E6 Select a to...

Страница 1338: ...iver PortNumber Selects the receiving port for the intermodulation measurement Suffix Ch Channel number Parameters PortNumber Analyzer port number Range 1 to the number of analyzer ports RST 2 Example...

Страница 1339: ...IMOD LTON PORT 1 UTON GEN 1 Reset the instrument to create the default channel no 1 Select port 1 as a source port for the lower tone a previously config ured external generator no 1 as a source port...

Страница 1340: ...modulation spectrum measurement for the new channel no 2 CALC2 PAR CAT Query the traces in channel no 2 The response is Trc2 B2D1SAM Manual operation See CW Mode Spectrum on page 336 SENSe Ch FREQuenc...

Страница 1341: ...to set the sideband SBANd parameter individually for each segment see SENSe Ch SEGMent Seg DEFine The SENSe Ch FREQuency SBANd setting is global and not valid for segmented sweeps The two sideband set...

Страница 1342: ...operation See Seg X Axis on page 497 SENSe Ch FREQuency SPAN Span Defines the width span of the measurement and display range for a frequency sweep sweep range The default span equals to the maximum...

Страница 1343: ...Span Frequency on page 474 Note If the start frequency entered is greater than the current stop frequency the stop frequency is set to the start frequency plus the minimum frequency span SENSe Ch FRE...

Страница 1344: ...configuration of channel Ch Suffix Ch Channel number Parameters Address Use SENSe GDEVice ADDRess to get the address strings of the configured generic devices If omitted as a parameter the query retur...

Страница 1345: ...ured generic device addresses The result is 192 168 0 1 192 168 0 2 SENSe1 GDEVice SELect 192 168 0 1 test json MySetting1 SENSe1 GDEVice SELect 192 168 0 2 test json MySetting2 Configure the commands...

Страница 1346: ...407 SENSe Ch HARMonic DLENgth DATA DUTLength Sets gets the expected maximum time delay through the DUT The set command automatically activates the Automatic Harmonic Grid function i e it sets SENSe C...

Страница 1347: ...El Length Max Mech Length on page 735 SENSe Ch HARMonic PERMittivity DATA DUTPermittivity Sets gets the relative permittivity of the DUT The set command automatically activates the Automatic Harmonic...

Страница 1348: ...sets SENSe Ch HARMonic AUTO to ON Suffix Ch Channel number Parameters DUTRiseThreshold T1_9 T2_8 Rise time definition T1_9 10 90 T2_8 20 80 RST T2_8 Options R S ZNA K20 Manual operation See Rise Time...

Страница 1349: ...STATe 1350 SENSe Ch LPORt LogPt ZCOMmon RealPart ImaginaryPart SENSe Ch LPORt LogPt ZDIFferent RealPart ImaginaryPart These commands specify the complex common mode and differential mode reference im...

Страница 1350: ...between default and renormalized reference impedance s for logical port LogPt Suffix Ch Channel number LogPt Logical port number Parameters arg0 ON 1 Use default impedance s OFF 0 Use the redefined im...

Страница 1351: ...al operation See Noise Det Meas Time on page 541 SENSe Ch NFIGure CALibration GTIMe DetectorTime Defines the detector time of the AVG detector which is used for the gain measurement during a NF calibr...

Страница 1352: ...it s Example SENSe1 NFIGure GTIMe 10ms Options R S ZNA K30 Manual operation See Gain Det Meas Time on page 327 SENSe Ch NFIGure HARMonic STATe State Activates or deactivates the sideband correction fo...

Страница 1353: ...which are measured and corrected during calibration and measurement RST 3 Options R S ZNA K30 Manual operation See Sideband Correction on page 329 SENSe Ch NFIGure IFConst IFFreq If manual selection i...

Страница 1354: ...ns R S ZNA K30 Manual operation See Noise Det Meas Time on page 327 SENSe Ch NFIGure RFICorr Boolean Activates or deactivates the image band correction at the DUT during a frequency con verting noise...

Страница 1355: ...9 SENSe Ch PATH Pt REFerence IFINpath 1359 SENSe Ch PATH Pt MEASurement IFOutauto 1359 SENSe Ch PATH Pt REFerence IFOutauto 1359 SENSe Ch PATH Pt MEASurement IFRequency 1359 SENSe Ch PATH Pt REFerence...

Страница 1356: ...ameters Direct IF Access InPath DIRect LP_60m BP_279m DIRect Frequency range 5kHz to 1 GHz LP_60m Low pass frequency range 5 kHz to 60 MHz BP_279m Band pass frequency range 260 MHz to 290 MHz Usage Se...

Страница 1357: ...re the converted frequency or frequency range is within the Direct IF Access Input Output ports frequency range If the resulting frequencies are not completely within the allowed range the analyzer re...

Страница 1358: ...on Ref and Meas path OFF IN OUT OFF The ports are unused IN The ports are for IF input but not for IF output Note that direct generator receiver access cannot be used in this case OUT The ports are f...

Страница 1359: ...SENSe Ch PATH Pt MEASurement IFOutauto SENSe Ch PATH Pt REFerence IFOutauto Queries how the outgoing frequencies at IF Reference Pt and IF Meas Pt are determined in channel Ch if SENSe Ch PATH Pt IFSW...

Страница 1360: ...l number Pt Port number Usage Query only Options R S ZNA B26 Manual operation See Rear Out on page 622 7 3 14 19 SENSe PHASe SENSe Ch PHASe MODE True Phase Mode Defines the phase mode of channel Ch i...

Страница 1361: ...the port impedance This part may be omitted to define a real impedance Range 10 M to 10 M RST Default reference impedance of the connector fam ily assigned to the port real impedance e g 50 Default un...

Страница 1362: ...port number of the analyzer Parameters AGCMode LDIStortion LNOise LDIStortion 0 dB gain for high input levels LNOise 10 dB gain for low input levels RST LDIS Example RST SENSe POWer IFG2 MEAS LNO SEN...

Страница 1363: ...t affected Manual operation See Receiver Step Att on page 477 SENSe Ch POWer GAINcontrol ReceiverName Mode SENSe Ch POWer GAINcontrol ReceiverName Defines port specific gain settings for the related c...

Страница 1364: ...control GC Mode to all a and b wave receivers Note This applies to the wideband signal path only If you also want to distinguish between different sweep segments use SENSe Ch SEGMent Seg POWer GAINcon...

Страница 1365: ...t STATe Boolean Enables or disables the internal preamplifier located in the measurement receiver path of port Pt 1 or 2 Suffix Ch Channel number Pt 1 2 Port number Pt 1 addresses the Chapter 4 7 36 I...

Страница 1366: ...it s Manual operation See Acq Time Fixed on page 644 SENSe Ch PULSe COUPled STATe Boolean Enforces identical pulse generator settings for all ports Suffix Ch Channel number Parameters Boolean RST OFF...

Страница 1367: ...ON 1 Fixed duty cycle for all pulse generators in channel Ch The pulses are defined using SENSe Ch PULSe PERiod and SENSe Ch PULSe DUTYcycle OFF 0 The generator specific pulses are defined using SENS...

Страница 1368: ...d DELay Delay for the generated pulse Suffix Ch Id Parameters Delay for the generated pulse Manual operation See Pulse Delay Pulse Width on page 642 SENSe Ch PULSe GENerator Id TRAin DATA Set pulse tr...

Страница 1369: ...t on page 642 SENSe Ch PULSe GENerator Id TRAin SEGMent Seg STARt Start time of the segment of the pulse train Suffix Ch Id Seg Parameters Start time of the segment of the pulse train Manual operation...

Страница 1370: ...tor Id TRAin STATe Set pulse train active inactive Suffix Ch Id Parameters Set pulse train active inactive Manual operation See Enable Pulse Train on page 642 SENSe Ch PULSe GENerator Id WIDTh Width f...

Страница 1371: ...lay relative to the pulse start Suffix Ch Channel number Parameters Value Measurement delay s Manual operation See Acq Delay Fixed on page 644 SENSe Ch PULSe PERiod pulse period for pulse modulators a...

Страница 1372: ...NPut INVerted STATe Boolean If External Pulse Control is used this defines the polarity of the incoming generator pulses at PuMo In Out Pt Suffix Ch Channel number Pt Port number Parameters Boolean RS...

Страница 1373: ...pulses generated by the respective internal pulse generator This setting only takes effect if Internal Pulse Control is enabled SENSe Ch PULSe INTernal STATe ON Fixed Duty Cycle is disabled SENSe Ch...

Страница 1374: ...l SENSe Ch ROSCillator SOURce Source Selects the source of the reference oscillator signal Parameters Source INTernal EXTernal ESMA INTernal internal 10 MHz reference oscillator EXTernal external refe...

Страница 1375: ...ference clock signal at the Reference In Out SMA connector see SENSe Ch ROSCillator SOURce Parameters SupportedFreqs F100mhz F1GHz 100 MHz or 1 GHz RST F100mhz Manual operation See Reference I O SMA I...

Страница 1376: ...Seg DELete DUMMy 1383 SENSe Ch SEGMent Seg FREQuency CENTer 1383 SENSe Ch SEGMent Seg FREQuency SPAN 1383 SENSe Ch SEGMent Seg FREQuency STARt 1384 SENSe Ch SEGMent Seg FREQuency STOP 1384 SENSe Ch SE...

Страница 1377: ...surement in sweep segment Seg RST ON Example SEGM ADD Create a new sweep segment no 1 in channel no 1 using default settings SEGM OFF Disable the measurement in the created sweep segment Manual operat...

Страница 1378: ...andwidth 30 MHz on page 259 Bandwidths can be set in 1 1 5 2 3 5 7 steps The analyzer rounds up any entered value between these steps and rounds down values exceeding the maximum bandwidth At the same...

Страница 1379: ...segment no 1 to the initial value Manual operation See Optional Columns on page 503 SENSe Ch SEGMent Seg BWIDth RESolution SELect Selectivity Defines the Selectivity of the IF filter used in sweep se...

Страница 1380: ...HIGH Change the selectivity to HIGH BWID SEL Query the default selectivity for unsegmented sweeps The response is NORM SEGM BWID SEL CONT OFF Couple the selectivities in all segments and reset the sel...

Страница 1381: ...Ment Seg SWEep TIME In the setting SENSe Ch SEGMent Seg INSert SELect DWELl this parameter is replaced by MeasDelay Range Depending on other channel settings AUTO acti vates automatic sweep time setti...

Страница 1382: ...s Delay Suffix Ch Channel number Seg Sweep segment number Parameters TimeRef SWTime DWELl SWTime use segment sweep time DWELl use measurement delay Example SEGM1 DEF SEL DWEL Select the measurement de...

Страница 1383: ...lt settings SEGM DEL Delete the created segment Manual operation See Add Insert Delete Delete All on page 500 SENSe Ch SEGMent Seg FREQuency CENTer SENSe Ch SEGMent Seg FREQuency SPAN These commands r...

Страница 1384: ...Table Columns on page 500 Note If the start frequency entered is greater than the current stop frequency the stop frequency is set to the start frequency If the stop frequency entered is smaller than...

Страница 1385: ...ints Number of sweep points in the segment See SENSe Ch SEGMent Seg SWEep POINts Power Internal source power in the segment See SENSe Ch SEGMent Seg POWer LEVel Default unit dBm SegmentTime Duration o...

Страница 1386: ...of the analyzer Manual operation See Add Insert Delete Delete All on page 500 SENSe Ch SEGMent Seg INSert SELect TimeRef Defines whether the sweep time of a new segment i e numeric parameter no 5 of t...

Страница 1387: ...ult settings and thus 10 dBm internal source power SEGM POW 20 Decrease the power to 20 dBm Manual operation See Optional Columns on page 503 SENSe Ch SEGMent Seg PORT PortId POWer LEVel IntSourcePort...

Страница 1388: ...eg of channel Ch RST OFF Manual operation See Optional Columns on page 503 SENSe Ch SEGMent Seg POWer GAINcontrol ReceiverName Mode SENSe Ch SEGMent Seg POWer GAINcontrol ReceiverName Defines port and...

Страница 1389: ...sweep segment no 1 to Low Noise The setting applies while port 2 is the drive port SENSe SEGMent1 POWer GAINcontrol B1D2 Query the ACG setting for the wave b1 in sweep segment no 1 while port 2 is th...

Страница 1390: ...eration See Range on page 636 SENSe Ch SEGMent Seg POWer GAINcontrol CONTrol Boolean Defines whether common or independent gain control GC settings shall be used for the individual sweep segments Suff...

Страница 1391: ...fault settings and thus 10 dBm internal source power SEGM POW 20 Decrease the power to 20 dBm SEGM POW CONT OFF Couple the powers in all segments and reset the power in seg ment no 1 to the initial va...

Страница 1392: ...controls the whole segmented sweep Parameters Boolean ON use independent delay settings to be defined via SENSe Ch SEGMent Seg SWEep DWELl OFF reset the delay in all sweep segments to the delay for u...

Страница 1393: ...s SegSweepTime Segment time The minimum segment time depends on the other channel settings in particular on the number of points SENSe Ch SEGMent Seg SWEep POINts the IF bandwidth SENSe Ch SEGMent Seg...

Страница 1394: ...sing SENSe Ch SEGMent Seg SWEep TIME or if the channel settings in a sweep segment require a sweep time larger than the unsegmented sweep time RST OFF Example SEGM ADD Create a new sweep segment no 1...

Страница 1395: ...ues MINPeak Take the smallest of all sample values RMS Take the root mean square of all sample values AVERage Take the arithmetic mean of all sample values Options R S ZNA K1 Manual operation See Dete...

Страница 1396: ...commands provide general settings to control the sweep Most of the settings have an impact on the sweep time SENSe SWEep COUNt ALL 1397 SENSe Ch SWEep AXIS FREQuency 1397 SENSe Ch SWEep AXIS POWer 13...

Страница 1397: ...nly Manual operation See Sweeps Memory Size on page 512 SENSe Ch SWEep AXIS FREQuency Scale Selects either the channel base frequency or one of the port frequencies as the stimu lus axis in all diagra...

Страница 1398: ...he source signal at port 2 as the reference signal for fre quency definitions FREQ STAR STOP Query the frequency range at test port 2 The response is 2000000000 3000000000 Manual operation See Stimulu...

Страница 1399: ...SENSe Ch SWEep COUNt Sweep Defines the channel specific number of sweeps to be measured and buffered in single sweep mode INITiate Ch CONTinuous OFF Use SENSe SWEep COUNt ALL to define the sweep coun...

Страница 1400: ...Options R S ZNA K28 Manual operation See Sweep Time Mode on page 490 SENSe Ch SWEep DETector TIME DetectorTime Defines the observation time per sweep point if a detector other than the Normal SAMple d...

Страница 1401: ...vating a frequency sweep with the S parameter S21 as a measurement result for channel and trace no 1 SWEep TIME Query total sweep time SWEep DWEL 1 SWEep DWEL IPOint ALL Set a delay of 1 s for each pa...

Страница 1402: ...WEep GENeration mode Sets gets the sweep mode for linear frequency sweeps Suffix Ch Channel number Parameters mode STEPped The R S ZNA only supports the stepped mode i e the fre quency is changed step...

Страница 1403: ...SWEep STEP StepSize Sets the distance between two consecutive sweep points in a non segmented linear frequency sweep Suffix Ch Channel number Parameters StepSize Frequency step size The step size is...

Страница 1404: ...CW Mode SENSe Ch SWEep TYPE Suffix Ch Channel number Parameters SweepDuration Sweep time The minimum possible sweep time depends on the other channel settings in particular on the number of points SEN...

Страница 1405: ...mple SWE TIME 1 Set a total sweep time of 1 s SWE TIME AUTO A query returns the value 1 Manual operation See Sweep Time Auto on page 490 SENSe Ch SWEep TIME MODE Algorithm Defines how the firmware cal...

Страница 1406: ...arithmic frequency axis POWer Power sweep The measurement is performed at constant fre quency SOURce Ch FREQuency PhyPt FIXed but with variable generator power that is swept in linear equidistant step...

Страница 1407: ...ci ated to the active trace If an S parameter S out in is measured the second port number index in input port of the DUT drive port of the analyzer is set equal to the selected drive port Drive port s...

Страница 1408: ...causes a factory reset Suffix Pt Number of the redefined physical port Parameters PortString String representation Source a wave b wave where Source i refers to original VNA ports Source Gi refers to...

Страница 1409: ...K6 Manual operation See Source Coh On on page 647 SOURce Ch CMODe OPTimized STATe Boolean Allows to select between optimized and raw phase control in source coherence mode see SOURce Ch CMODe STATe on...

Страница 1410: ...t the phase span Suffix Ch Channel number If unspecified the numeric suffix is set to 1 Pt Physical port number Parameters Value Port specific phase value Increment 1 deg RST 0 deg Example See SOURce...

Страница 1411: ...EQuency PhyPt FIXed 1412 SOURce Ch FREQuency PhyPt CONVersion ARBitrary EFRequency Gen 1413 SOURce Ch FREQuency PhyPt CONVersion ARBitrary IFRequency 1414 SOURce Ch FREQuency CONVersion MIXer PMFixed...

Страница 1412: ...nimum of the analyzer s frequency range Example See SENSe Ch FREQuency CONVersion MIXer MFFixed Manual operation See RF IF and Mixer Stages on page 365 SOURce Ch FREQuency PhyPt CW FixedFreq SOURce Ch...

Страница 1413: ...r frequency sweeps or a CW fre quency for power time and CW Mode sweeps Suffix Ch Channel number PhyPt Test port number of the analyzer This suffix is ignored because the generator is referenced via G...

Страница 1414: ...R 1E 9 STOP 1 1E 9 Reset the analyzer activating a frequency sweep and set the sweep range between 1 GHz and 1 1 GHz SYST COMM RDEV GEN1 DEF Ext Gen 1 SME02 gpib0 21 Configure an R S SME02 generator a...

Страница 1415: ...range or CW frequency of the ana lyzer i e Numerator Denominator 1 Off set 0 Hz Default unit 1 Denominator See above Numerator Range The converted frequency or frequency range must be within the analy...

Страница 1416: ...LO Out port R S ZNA B8 SOURce Ch RLO FREQuency 1416 SOURce Ch RLO PABSolut 1417 SOURce Ch RLO PERMenable 1417 SOURce Ch RLO POFFset 1418 SOURce Ch RLO FREQuency Numerator Denominator Offset SweepType...

Страница 1417: ...the optional rear LO Out port for chan nel Ch Suffix Ch Channel number Parameters Mode OFF 0 Use the channel base power Pb ON 1 Use a fixed value of 0 dBm Options R S ZNA B8 Manual operation See Chann...

Страница 1418: ...port PhysicalPort1 or to a pair of physical ports PhysicalPort1 PhysicalPort2 at the same time defining them as balanced port Important All required logical ports balanced and single ended must be cr...

Страница 1419: ...efine logical ports no 1 and 2 as used group 1 port 3 as unused SOUR GRO Query the port group no 1 The response is 1 2 SOUR GRO CLE ALL SOUR GRO Dissolve all port groups and repeat the query The respo...

Страница 1420: ...1420 SOURce Ch GROup Grp 1420 SOURce Ch GROup Grp CLEar 1421 SOURce Ch GROup Grp NAME 1421 SOURce Ch GROup Grp PORTs 1422 SOURce Ch GROup Grp PPORts 1422 SOURce Ch GROup Grp SIMultaneous FOFFset STATe...

Страница 1421: ...number Grp Port group number This suffix is ignored only Grp 1 is supported Setting parameters arg0 ALL If ALL is specified all port groups are dissolved and a default port group 1 consisting of all a...

Страница 1422: ...e physical VNA ports making up group Grp Note Port groups must be created in ascending order i e first create port group 1 then port group 2 etc By default there is a single port group which contains...

Страница 1423: ...et may not be active if not all preconditions are met For each precondi tion there is a unique return value for the query SOURce Ch GROup Grp SIMultaneous FOFFset CONDition which is returned when the...

Страница 1424: ...3 DPORt 2 SOUR GRO2 PPORT4 DPORt 1 INIT IMM WAI Assign DUT Ports to VNA Ports CALCulate1 PARameter SDEFine Trc2 S34 DISPlay WINDow1 TRACe2 FEED Trc2 WAI Add and display a second trace for the second D...

Страница 1425: ...is actually used may be larger than the minimum offset as the firm ware chooses the smallest multiple of the frequency step size between the points in a segment or in a linear frequency sweep respect...

Страница 1426: ...um frequency offset To get the resulting frequency offset the minimum frequency off set is rounded to a multiple of the current frequency step size Range 0 Hz to 17 MHz RST 1 MHz Default unit Hz Examp...

Страница 1427: ...ormed so that no information about Simultaneous Measurement with Frequency Offset is available This query waits until all previous commands are fully executed because these com mands may affect the op...

Страница 1428: ...actual frequency offset of several MHz can be used Please note however that a small minimum fre quency offset combined with a large frequency step size may lead to a large actual frequency offset 9 ex...

Страница 1429: ...eter 1431 SOURce Ch POWer ALC PIParameter ASETtling 1432 SOURce Ch POWer ALC PIParameter CTIMe 1432 SOURce Ch POWer Pt ALC PIParameter GAIN 1432 SOURce Ch POWer Pt ALC PIParameter ITIMe 1433 SOURce Ch...

Страница 1430: ...F Bandwidth on page 481 SOURce Ch POWer ALC BANDwidth Bandwidth Selects the bandwidth in the ALC control loop in channel Ch Suffix Ch Channel number Parameters Bandwidth Bandwidth Hz in the ALC contro...

Страница 1431: ...timize Suffix Ch Pt Parameters Alc optimize AUTO ON OFF Manual operation See ALC Optimize on page 481 SOURce Ch POWer Pt ALC PIParameter Mode Enables automatic or manual setting of the ALC PI controll...

Страница 1432: ...imum settling time of the ALC control loop per sweep point Set SOURce Ch POWer ALC PIParameter ASETtling ON to exit the control loop as soon as the convergence criteria are met Suffix Ch Channel numbe...

Страница 1433: ...OWer Pt ALC RANGe CRange Defines the maximum change of the source signal level due to the ALC for physical port Pt in channel Ch Suffix Ch Channel number Pt Port number Parameters CRange Control range...

Страница 1434: ...been reversed in firmware version 2 20 i e before FW V2 20 a positive negative offset decreased increased the source signal level before the ALC loop is started Range 3 to 3 Increment 0 1 RST 0 Manua...

Страница 1435: ...Ceiver Only applies if the target power isn t taken from the port configuration SOURce Ch POWer Pt ALC TPOWer DEFault on page 1435 OFF Suffix Ch Channel number Pt Port number Parameters Value Default...

Страница 1436: ...SLEVeling The following commands define and control the source leveling function that is enabled with option R S ZNA K8 See Chapter 4 7 7 1 Leveling on page 254 SOURce POWer PhyPt CORRection SLEVelin...

Страница 1437: ...ce POWer PhyPt CORRection SLEVeling FREQuency POINts NrOfPts Defines the number of frequency sweep points of the leveling grid for physical port PhyPt After defining the leveling grid using SOURce Ch...

Страница 1438: ...ical port number Parameters FreqValue Start stop value Default unit Hz Example See SOURce POWer PhyPt CORRection SLEVeling ACQuire Options R S ZNA K8 Manual operation See Start Frequency Stop Frequeny...

Страница 1439: ...ptions R S ZNA K8 Manual operation See Start Power Stop Power on page 549 SOURce POWer PhyPt CORRection SLEVeling POWer STEP Size Defines the source power step size of the leveling grid for physical p...

Страница 1440: ...OWer CORRection SLEVeling POWer STOP 0 SOURce POWer CORRection SLEVeling POWer STEP 0 5 DB SOURce POWer CORRection SLEVeling POWer ALC ON SOURce POWer1 CORRection SLEVeling ACQuire Usage Event Options...

Страница 1441: ...STATe 1457 SOURce Ch POWer PhyPt CORRection TCOefficient CALibration 1458 SOURce Ch POWer PhyPt CORRection TCOefficient COUNt 1459 SOURce Ch POWer PhyPt CORRection TCOefficient DEFine ListNo 1459 SOU...

Страница 1442: ...is not equal to the number of sweep points Suffix Ch Calibrated channel number PhyPt Physical port number used to select a specific frequency axis in arbitrary mode with option R S ZNA K4 Parameters...

Страница 1443: ...librated port and calibration type Gen Source Power Rec Measurement Receiver STARt Start Frequency or Power Stop Frequency or Power POINts Nr of sweep points STYPe Sweep type or grid LIN LOG SEGM ATTe...

Страница 1444: ...ORR POW HARM ACQ Start receiver power calibration at receive port 2 Usage Event Options R S ZNA K4 Manual operation See Harmonic Orders to Calibrate on page 536 SOURce Ch POWer CORRection IMODulation...

Страница 1445: ...GENerator external generator is the source The port and generator numbers are specified by means of the SourcePort parameter A1 A2 A3 A4 direct analyzer port selection The parame ters A1 and A2 are ZV...

Страница 1446: ...R POW CORR ACQ GEN 1 Perform a source power calibration using the external generator no 1 as a source SOUR POW CORR GEN Check whether the calibration is applied the response is 1 Usage Setting only Ma...

Страница 1447: ...e command is valid for all channels ports and external generators Equivalent command SOURce Ch POWer PhyPt CORRection NREadings Suffix Ch Calibrated channel number This parameter is ignored the limit...

Страница 1448: ...gnored the conver gence factor is valid for all sources Parameters Convergence Convergence factor Range 0 to 2 Increment 0 1 RST 1 Example See SOURce Ch POWer PhyPt CORRection ACQuire Manual operation...

Страница 1449: ...is ignored the method is valid for all channels PhyPt Calibrated port number This parameter is ignored the selected calibration method is used for all sources Parameters PowerCalMethod PMONly RRAFter...

Страница 1450: ...ers arg0 Enables ON or disables OFF reference receiver calibration RST ON Example See SOURce Ch POWer PhyPt CORRection COLLect FLATness on page 1448 SOURce POWer CORRection COLLect VNETworks DeEmbNwTy...

Страница 1451: ...e default calibration data set is to provide a dummy power cali bration which you can replace with your own external power calibration data You may have acquired the external data in a previous sessio...

Страница 1452: ...he previous actions SOURce Ch POWer PhyPt CORRection FAST arg0 Enables or disables a fast source power calibration where the external power meter is used for the first calibration sweep only It is rec...

Страница 1453: ...ation Suffix Ch Channel number Parameters FastOrNot ON 1 Fast method enabled OFF 0 Fast method disabled RST ON 1 Options R S ZNA K4 Manual operation See IMD Cal Method Fast Cal Interp Meas on page 537...

Страница 1454: ...at the previ ously selected source port 3 CORR POW IMOD ACQ Start receiver power calibration at the previously selected receive port 2 CALC PAR SDEF IM_Meas IP3UI Create a new channel no 2 and a trac...

Страница 1455: ...imit applies to all sources PhyPt Calibrated port number This parameter is ignored the limit applies to all sources Parameters NoCalSweeps Number of readings Range 1 to 100 RST 2 Example See SOURce Ch...

Страница 1456: ...fines the source step attenuation the R S ZNA uses during the first calibration sweep of the source power calibration Reference Receiver Cal Power This attenu ation value is ignored if the R S ZNA is...

Страница 1457: ...PhyPt CORRection GENerator Gen STATe Suffix Ch Calibrated channel number PhyPt Calibrated port number Parameters Boolean Enables ON or disables OFF the source power calibration for port number PhyPt R...

Страница 1458: ...tup with the additional two port in front of the DUT SOUR POW CORR TCO DEF 1GHz 5 DEF 2GHz 10 DEF2 Define two points in the power loss list query the second point SOUR POW CORR TCO INS2 1 5 GHz 7 5 In...

Страница 1459: ...ent settings are valid for all channels PhyPt Calibrated port number This parameter is ignored the transmis sion coefficient settings are valid for all sources Example See SOURce Ch POWer PhyPt CORRec...

Страница 1460: ...channel number This parameter is ignored the trans mission coefficient settings are valid for all channels PhyPt Calibrated port number This parameter is ignored the transmis sion coefficient setting...

Страница 1461: ...Example See SOURce Ch POWer PhyPt CORRection TCOefficient CALibration Usage Setting only Manual operation See Get Trace on page 579 SOURce Ch POWer PhyPt CORRection TCOefficient INSert ListNo Frequen...

Страница 1462: ...ion LEVel OFFSet 1467 SOURce Ch POWer Pt EATTenuation 1468 SOURce Ch POWer Pt EATTenuation MODE 1468 SOURce Ch POWer PhyPt GENerator Gen OFFSet 1468 SOURce Ch POWer PhyPt GENerator Gen PERManent STATe...

Страница 1463: ...SWEepend MODE SweepEndModes Selects the power mode at sweep end This is a global setting Parameters SweepEndModes AUTO REDuce KEEP AUTO at sweep end restore the power at sweep start REDuce at sweep en...

Страница 1464: ...efine a port specific slope factor for the source power SOURce Ch POWer PhyPt LEVel IMMediate SLOPe and a port specific power offset SOURce Ch POWer PhyPt LEVel IMMediate OFFSet Parameters IntSourcePo...

Страница 1465: ...R POW1 OFFS 6 ONLY SOUR POW2 OFFS 6 CPAD Replace the source power at port 1 by 6 dBm the source power at port 2 by 0 dBm The powers at the remaining ports if available are not affected Manual operatio...

Страница 1466: ...to 6 dBm SOUR POW2 ATT 10 Set the mechanical step attenuator for the wave transmitted at port 2 to 10 dB The internal source power and the waves at the other test ports are not affected Options Manual...

Страница 1467: ...values or an attenuation negative values in the signal path between the source port and the calibrated reference plane The value has no impact on the source power Suffix Ch Calibrated channel number...

Страница 1468: ...SourceAttMode Defines how the electronic source attenuation of port Pt is selected in channel Ch Suffix Ch Channel number Pt Port number Parameters ElSourceAttMode AUTO MANual AUTO The firmware select...

Страница 1469: ...erator as external generator no 1 assigning the name Ext Gen 1 and a GPIB address 21 Configure a second R S SME02 generator assigning the name Ext Gen 2 and a GPIB address 22 SOUR POW GEN1 OFFS 6 ONLY...

Страница 1470: ...en 1 SME02 gpib0 21 Configure an R S SME02 generator as external generator no 1 assigning the name Ext Gen 1 and a GPIB address 21 SOUR POW GEN STAT OFF Turn the external generator off In the Port Con...

Страница 1471: ...generator model Increment 0 1 dB RST The power limit is not changed after a RST The factory setting is 0 dBm Default unit dBm Example See SOURce Ch POWer PhyPt LEVel IMMediate LLIMit VALue on page 14...

Страница 1472: ...source power at port 2 to 10 dBm and enable the source power limit SOUR POW2 LLIM DGR ON Optimize the automatic level control ALC for additional connec tors option R S ZVA B16 SYST COMM RDEV GEN1 DEF...

Страница 1473: ...end the output power of the sweep start is restored ON at sweep end the output power of the driving port is reduced as if the channel base power was set to its minimum possible value Example See SOUR...

Страница 1474: ...ower Default unit dBm Example SWE TYPE POW Activate a power sweep SOUR POW STAR 6 STOP 10 Select a power sweep range between 6 dBm and 10 dBm Manual operation See Start Power Stop Power on page 474 No...

Страница 1475: ...ferential received wave quantity bd1 using the single ended analyzer port no 2 as a source port Options R S ZNA K61 Manual operation See True Differential Mode on page 312 SOURce Ch TDIF IMBalance AMP...

Страница 1476: ...ace named Trc1 to measure the mixed mode S parameter Sds12 DISP TRAC FEED Trc1 Display the result Options R S ZNA K61 Manual operation See Amplitude Port Start Power Stop Power on page 650 SOURce Ch T...

Страница 1477: ...ivates the true differential mode the query returns 1 SOUR TDIF IMB PHAS LPOR 1 STAR 20 STOP 0 Configure the phase imbalance sweep selecting the logical port no 1 and a swept phase range between 20 de...

Страница 1478: ...two tone sig nal is available at the output of the combiner B16external The source out signal direct access of the lower tone port and the signal of the upper tone test port are combined externally T...

Страница 1479: ...eturn values freq_diff Frequency difference Usage Query only Options R S ZNA K9 Manual operation See Track LO on page 635 SOURce Ch NFIGure CALibration TPOWer SourcePowNfigCalExtAtt Suffix Ch Paramete...

Страница 1480: ...1481 STATus QUEStionable INTegrity HARDware ENABle 1482 STATus QUEStionable LIMit Lev ENABle 1482 STATus QUEStionable NTRansition 1482 STATus QUEStionable INTegrity NTRansition 1482 STATus QUEStionabl...

Страница 1481: ...rs see STATus QUEStionable LIMit 1 2 on page 872 Example STAT QUES LIM1 Query the EVENt part of the QUEStionable LIMit1 register to check whether an event has occurred since the last reading Usage Que...

Страница 1482: ...tern STATus QUEStionable INTegrity NTRansition BitPattern STATus QUEStionable INTegrity HARDware NTRansition BitPattern STATus QUEStionable LIMit Lev NTRansition BitPattern Sets the negative transitio...

Страница 1483: ...sages and troubleshooting on page 1632 Example STAT QUE Query the oldest entry in the error queue 0 No error is returned if the error queue is empty Usage Query only 7 3 17 SYSTem commands The SYSTem...

Страница 1484: ...STem COMMunicate RDEVice GENerator Gen SEPower 1498 SYSTem COMMunicate RDEVice PMETer CATalog 1498 SYSTem COMMunicate RDEVice PMETer CONFigure AUTO STATe 1498 SYSTem COMMunicate RDEVice PMETer COUNt 1...

Страница 1485: ...ing only SYSTem COMMunicate CODec Codec Selects the character encoding used at the remote interface The selected encoding applies to directory and file names CalKit names CalUnit characterizations and...

Страница 1486: ...B SELF LPORt ALIGn Boolean Configures queries the logical port creation If set to ON logical ports are aligned and must be set from low to high port which was the only possibility prior to firmware V1...

Страница 1487: ...erminator Example SYST COMM GPIB RTER EOI Set the terminator to EOI SYSTem COMMunicate NET HOSTname HostName Sets or gets the host name of the instrument Parameters HostName Host name RST A preset of...

Страница 1488: ...ne Calibration Controller ICC R S ZN Z30 serial number 100010 SYSTem COMMunicate RDEVice AKAL ADDRess SUBModule ALL Returns something like 1 ZN Z33 02 101325 2 ZN Z33 02 101325 3 ZN Z33 02 101339 i e...

Страница 1489: ...Query the active characterization SYSTem COMMunicate RDEVice AKAL CKIT ZN Z33 02 101336 afterAwhile Manual operation See Characterization on page 532 SYSTem COMMunicate RDEVice AKAL CKIT CATalog Queri...

Страница 1490: ...Factory Query the port assignment of the factory calibration SYSTem COMMunicate RDEVice AKAL CKIT STANdard CATalog Factory Query the standards of the factory calibration Possible response MOP P1 MSH P...

Страница 1491: ...the calibration unit Example See SYSTem COMMunicate RDEVice AKAL CKIT CATalog Usage Query only Manual operation See Characterization on page 532 SYSTem COMMunicate RDEVice AKAL FRANge CalKitName Quer...

Страница 1492: ...Usage Query only Manual operation See Characterization on page 532 SYSTem COMMunicate RDEVice AKAL PREDuction STATe Boolean Enables or disables automatic power reduction at all test ports while an au...

Страница 1493: ...KIT SDATa SecondPort Second port number for two port standards Port numbers can be omitted if the cal kit data is valid for all ports Example See SYSTem COMMunicate RDEVice AKAL CKIT CATalog Usage Que...

Страница 1494: ...Address Interface address string parameter depending on the interface type See Table 5 11 Example SYST COMM RDEV GDEV1 DEF VXI 11 192 168 0 1 SYST COMM RDEV GDEV2 DEF VXI 11 192 168 0 2 SYST COMM RDE...

Страница 1495: ...COMMunicate RDEVice GDEVice Gdev DEFine on page 1494 Usage Event Manual operation See Configured Devices on page 838 SYSTem COMMunicate RDEVice GENerator Gen CATalog Queries the numbers of all configu...

Страница 1496: ...t no particular name is assigned to the genera tor Driver Generator type string parameter The generator type is identi cal with the name of the generator driver file gen stored in the resources extdev...

Страница 1497: ...o longer configured Manual operation See Configured Devices on page 824 SYSTem COMMunicate RDEVice GENerator DELete Clears the configuration table for external generators Example See SYSTem COMMunicat...

Страница 1498: ...d external power meters The response is a string containing a comma separated list of power meter numbers Example SYST COMM RDEV PMET1 DEF USB Power Meter 1 NRP Z55 usb 100045 SYST COMM RDEV PMET2 DEF...

Страница 1499: ...auto zeroing of the external power meter Suffix Pmtr Number of the configured power meter Power meters must be numbered in ascending order starting with 1 If a number is re used the previous power met...

Страница 1500: ...ower meters PMx other Address Interface address string parameter depending on the interface type See Table 5 9 SensorId This optional string parameter is used for power meters that can control several...

Страница 1501: ...The following SYSTem commands provide general instrument configurations SYSTem AGC GLOBal METHod 1502 SYSTem CORRection JOINcal 1502 SYSTem CORRection WIZard IMMediate 1503 SYSTem DATE 1503 SYSTem DFP...

Страница 1502: ...em SHUTdown 1515 SYSTem SOUNd ALARm STATe 1516 SYSTem SOUNd STATus STATe 1516 SYSTem TIME 1516 SYSTem TRESet STATe 1517 SYSTem TSLock 1517 SYSTem TTLout Pt STATus STATe 1517 SYSTem USER DISPlay TITLe...

Страница 1503: ...tCal POOL of the resulting calibration Note If OutputCal LocationOutputCal matches one of the input calibrations this input calibration is replaced LocationOutputCal CHANnel POOL Return values Joinabl...

Страница 1504: ...AR TITLe STATe Boolean SYSTem DISPlay BAR TOOLs STATe Boolean Displays or hides the hardkey panel HKEY the menu bar below the diagram area MENU the status bar below the diagram area STATus the softtoo...

Страница 1505: ...eters ColorCount Range 1 to 100 Manual operation See Number of Trace Colors on page 792 SYSTem DISPlay UPDate Activate Switches the display on or off while the analyzer is in the remote state The comm...

Страница 1506: ...me time deletes all entries in the error queue The entries consist of an error number and a short description of the error Positive error numbers are instrument dependent Negative error numbers are re...

Страница 1507: ...enable disable display of information popups for the related event type RST ON Example SYST ERR DISP STATe ON ERR ON WARN OFF INFO OFF Display information popups for system errors but not for warn in...

Страница 1508: ...hannel 1 in the active recall set As a result the active recall set contains no chan nels traces and diagram areas Example SYST PRES SCOP SING Define the scope of a preset the active recall set is res...

Страница 1509: ...query Example SYST HELP SYNT SYST ERR Returns the full syntax In this case SYSTem ERRor NEXT Usage Query only SYSTem HELP SYNTax ALL Queries the implemented SCPI commands and their parameters Return...

Страница 1510: ...KLOCk ON Lock the local keys SYSTem LANGuage Language Specifies the remote language for the analyzer Parameters Language Command syntax for the analyzer string variable SCPI R S ZNA specific command...

Страница 1511: ...ory setting The analyzer returns a comma separated list of software and hardware options Usage Event Manual operation See Define IDN OPT on page 815 SYSTem OPTions STRing Options Defines an option str...

Страница 1512: ...ws you to select between different preset modes GLOBal and RFOFf presets can only be performed from the analyzer GUI FILE pre sets can also be performed via remote commands if SYSTem PRESet REMote STA...

Страница 1513: ...ESet MODE on page 1512 SINGle The active setup is reset according to the current preset mode the name of the active setup and the parameters of all other set ups remain unchanged Example See SYSTem PR...

Страница 1514: ...t Configuration on page 788 SYSTem PRESet USER CAL PresetUserCal Selects a calibration from the calibration pool that shall be restored during a user defined preset The corresponding cal group file Pr...

Страница 1515: ...ly The analyzer waits until the end of the current sweep sequence and changes all settings made during the last sweep period when the next single sweep sequence is initiated Several settings can be ma...

Страница 1516: ...Nd ALARm STATe Boolean SYSTem SOUNd STATus STATe Boolean These commands switch alarm or status sounds on or off Parameters Boolean ON OFF RST ON Example SYST SOUN ALAR OFF STAT OFF Switch alarm and st...

Страница 1517: ...ote screen remains active You can use the buttons in Remote softtool panels If you switch back to manual control the drag and drop functions e g for markers are still available DIAGrams lock the drag...

Страница 1518: ...display is switched off Parameters String Title string A n in the string starts a new line see example RST empty string Example SYST USER DISP TITL DON T TOUCH nRemote test running Define a title for...

Страница 1519: ...ult keys SYSTem VERSion Returns the SCPI version number to which the analyzer complies The analyzer com plies to the final SCPI version 1999 0 Example SYST VERS Query the SCPI version The response is...

Страница 1520: ...COPY TRACe DATA STIMulus ALL TRACe DATA RESPonse ALL CALCulate Chn MATH EXPRession DEFine TRACe COPY MemTraceName DataTraceName Copies a data trace to a memory trace The trace to be copied can be spec...

Страница 1521: ...Mem_Pt20 CH1DATA Copy the current state of the created trace to a memory trace named Mem_Pt20 The memory trace is not displayed DISP WIND TRAC2 FEED MEM_PT20 Display the created memory trace in the a...

Страница 1522: ...onal mnemonic SENSe1 CALC MATH SDEF Trc1 2 CALC MATH STAT ON Define a mathematical trace dividing the data trace by 2 Acti vate the mathematical mode and display the mathematical trace instead of the...

Страница 1523: ...f the available external trigger sources Suffix Ch Parameters TrigExtInput EXTA EXTB EXTC EXTD UPORt2 EXTA Trigger In A or Pin 2 of User Port EXTB EXTC EXTD Trigger In B Trigger In D requires Trigger...

Страница 1524: ...the current or at the next sweep point RST SWEep Example SEGM ADD SWE TYPE SEGM Select segmented frequency sweep TRIG LINK SEGM Select a trigger segment as triggered measurement sequence TRIG LINK Que...

Страница 1525: ...ment see TRIGger Ch SEQuence LINK DelayTime Delay time Range 0 s to 13680 s Increment 10 ms RST 0 s Default unit s Example See TRIGger Ch SEQuence MULTiple SOURce inp Manual operation See Logic on pag...

Страница 1526: ...events occur on the rising or on the falling edge or on the beginning of the high low level periods of the external TTL trigger signal Num Suffix Ch Channel number Num Number of trigger signal 1 or 2...

Страница 1527: ...Trigger In D requires Trigger board hardware option R S ZNA B91 UPORt2 Trigger event at pin 25 of User Port MANual Trigger event generated by pressing the Manual Trigger soft key Example See TRIGger...

Страница 1528: ...r mode The analyzer starts the next mea surement sequence when the Manual Trigger button is pressed Manual operation See FreeRun External Manual Multiple Triggers on page 506 7 3 19 2 TRIGger CHANnel...

Страница 1529: ...Delay on page 510 TRIGger CHANnel Ch AUXiliary n DURation TrigOutDuration If pulses are used as trigger out signals see TRIGger CHANnel Ch AUXiliary n STYPe this setting specifies the width of the ou...

Страница 1530: ...ite polarity can be generated as trigger out signal RST SWEep Example TRIGger CHANnel1 AUXiliary1 STYPe POSitive Generate pulses with positive polarity at Trigger Out A TRIGger CHANnel AUXiliary INTer...

Страница 1531: ...n STYPe Type Defines how the selected sweep sequence is indicated at the related Trigger Out con nector Use TRIGger CHANnel Ch AUXiliary n INTerval to select the sweep sequence Suffix Ch Channel numb...

Страница 1532: ...ay measurements Mixer phase calibration R S ZNA K5 SENSe Ch CORRection POWer MIXer IF ACQuire 1532 SENSe Ch FREQuency CONVersion MIXer PRFimage 1533 SOURce Ch POWer CORRection MIXer IF ACQuire 1533 SO...

Страница 1533: ...ription of the mixer calibration procedure Suffix Ch Calibrated channel number Example FREQ CONV VMIX Select a vector mixer measurement set frequencies see e g example for SENSe Ch FREQuency CONVersio...

Страница 1534: ...he command initiates two calibration steps the first over the RF frequency range the second over the IF frequency range for a subse quent receiver power calibration SENSe Ch CORRection POWer PhyPt MIX...

Страница 1535: ...EQuency MDELay UTONe 1538 SENSe Chn FREQuency MDELay MSTate 1539 MMEMory LOAD MDAData 1539 MMEMory LOAD MDCData 1539 MMEMory STORe MDCData 1540 SENSe Ch FREQuency MDELay ACQuire Starts a calibration s...

Страница 1536: ...ual operation See 2 Tone Delta Freq on page 372 SENSe Ch FREQuency MDELay CDELay const delay value for ref mixer Defines a constant mixer delay value to be used as a reference for a mixer delay mea su...

Страница 1537: ...ENSe Ch FREQuency MDELay CORRection STATe arg0 Qualifies whether the analyzer applies the current mixer delay measurement calibra tion data Tip The analyzer automatically applies the calibration data...

Страница 1538: ...IF on page 373 SENSe Ch FREQuency MDELay UTONe Upper tone source signal port number Selects the source for the upper tone signal that is used for the mixer delay measure ment Suffix Ch Channel number...

Страница 1539: ...analyzer searches the current direc tory to be queried with MMEMory CDIRectory Example See MMEMory LOAD MDCData on page 1539 Usage Setting only Manual operation See Constant Cal Dly Variable Cal Dly...

Страница 1540: ...ata to the current mixer delay measure ment Usage Setting only MMEMory STORe MDCData Channel Id Filename Stores the correction data for a mixer delay measurement calibration to a specified file Mixer...

Страница 1541: ...common receiver frequency Suffix Ch Channel number Pt Physical port number Parameters FreqOffset Frequency offset Range 0 Hz to 25 MHz Increment 1 Hz RST 0 Hz Default unit Hz Example FREQ STAR 2GHz S...

Страница 1542: ...Port to LO Assignment Mode OPTimized DEFault OPTimized Use both LOs if available DEFault Use only one LO RST DEFault Manual operation See LO Usage on page 634 7 3 20 4 Other SENSe Ch PPORt PPort BSHif...

Страница 1543: ...thm Example SYST CORR FMP ON Enable fast multiport correction 7 3 20 5 List of Commands SENSe Ch CORRection POWer MIXer IF ACQuire 1532 SENSe Ch FREQuency CONVersion MIXer PRFimage 1533 SENSe Ch FREQu...

Страница 1544: ...formation see the R S HUMS User Manual SNMP REST commands 1544 System information commands 1548 Device tags commands 1558 Utilization commands 1559 Service date commands 1562 7 4 1 SNMP REST commands...

Страница 1545: ...cate SNMP VERSion on page 1547 Setting parameters CommunityString String containing the community name Example Set read write access SYST COMM SNMP VERS V12 SYST COMM SNMP COMM RW ABC Usage Setting on...

Страница 1546: ...level Auth_pwd String containing the authentication password Priv_pwd String containing the privacy password Example Create user profile SYST COMM SNMP VERS V123 SYST COMM SNMP USM USER Peter RO PRIV...

Страница 1547: ...SNMP user profiles SYST COMM SNMP USM USER DEL ALL Usage Event Manual operation See SNMPv3 Configuration on page 802 SYSTem COMMunicate SNMP VERSion SnmpVersion This command selects the SNMP version P...

Страница 1548: ...1553 DIAGnostic HUMS STORage 1554 DIAGnostic HUMS SW 1555 DIAGnostic HUMS SYSTem INFO 1556 DIAGnostic HUMS SYSTem STATus 1556 DIAGnostic HUMS SYSTem STATus SUMMary 1557 DIAGnostic HUMS ALL This comma...

Страница 1549: ...data in a comma separated list manufacturer serialNumber version releaseDate caption isPresent Binary block data with the following syntax Digits Length Binarydata Indicates the start of the binary bl...

Страница 1550: ...ntSeverity Binary block data with the following syntax Digits Length Binarydata Indicates the start of the binary block Digits Decimal value Gives the number of decimal digits used for the Length valu...

Страница 1551: ...rydata Indicates the start of the binary block Digits Decimal value Gives the number of decimal digits used for the Length value Length Decimal value Number of bytes the follow in the Binary data part...

Страница 1552: ...values SecurityInfo block_data Security information of the connected instrument as block data in a comma separated list blockdata antimalware name enabled upToDate timestamp firewallEnabled Binary bl...

Страница 1553: ...llowing syntax Digits Length Binarydata Indicates the start of the binary block Digits Decimal value Gives the number of decimal digits used for the Length value Length Decimal value Number of bytes t...

Страница 1554: ...device1 storagedevice2 Binary block data with the following syntax Digits Length Binarydata Indicates the start of the binary block Digits Decimal value Gives the number of decimal digits used for the...

Страница 1555: ...100 worst 100 threshold 0 type Old_age updated Always whenFailed null rawValue 16856 Usage Query only DIAGnostic HUMS SW This command returns the installed software information of the instrument Depe...

Страница 1556: ...tes the start of the binary block Digits Decimal value Gives the number of decimal digits used for the Length value Length Decimal value Number of bytes the follow in the Binary data part Binary data...

Страница 1557: ...imit reference severity Example Return system status information DIAG HUMS SYST STAT 41874 globalStatus 3 values id 31522816 description RF Overload descriptionExtended null value null unit null upper...

Страница 1558: ...all tags DIAG HUMS TAGS ALL 1 location building_11 2 time zone CET Usage Query only Manual operation See Add Delete Trash Delete All on page 803 DIAGnostic HUMS TAGS VALue ID Key Value DIAGnostic HUMS...

Страница 1559: ...ge Event Manual operation See Add Delete Trash Delete All on page 803 7 4 4 Utilization commands Using these commands you can retrieve the utilization information provided by the R S HUMS They all req...

Страница 1560: ...1719936 scope DEVICE_SPECIFIC reference null name Self alignments unit counter description Report number of self alignments value 0 startupValue 0 activityTracking true Usage Query only DIAGnostic HUM...

Страница 1561: ...wing format blockdata timestamps timestamp1 timestamp2 activity activity1 activity2 Pair of values timestamp1 activity1 timestamp2 activity2 Binary block data with the following syntax Digits Length B...

Страница 1562: ...SERVice CALibration DATE CalibrationDate This command sets last date and time the instrument was calibrated in ISO 8601 for mat Parameters CalibrationDate String containing calibration date of the in...

Страница 1563: ...et by Rohde Schwarz service Parameters Duration String indicating the calibration interval ISO 8601 duration Example DIAG SERV CAL INT returns P1Y if the recommended calibration interval is 1 year Usa...

Страница 1564: ...Mit UPPer STATe 1567 CALCulate Ch PARameter DEFine 1567 CALCulate Chn MARKer Mk FUNCtion BWIDth 1569 CALCulate Chn MARKer Mk FUNCtion DELTa STATe 1569 CALCulate Chn MARKer Mk FUNCtion TARGet 1569 CALC...

Страница 1565: ...the y axis can be defined via CALCulate Ch LIMit RDOMain Suffix Chn Channel number used to identify the active trace Setting parameters SweepType FLIN FLOG FSEG FSINgle TLIN TLOG PLIN PLOG PSINgle Key...

Страница 1566: ...g limit line and re defines the physical units of the response values of the limit line The units of the stimulus values are defined via CALCulate Chn LIMit CONTrol DOMain Tip This command is compleme...

Страница 1567: ...mbers Note Use CALCulate Chn LIMit STATe to switch on or off the entire limit check including upper and lower limit lines Suffix Chn Channel number used to identify the active trace Parameters Boolean...

Страница 1568: ...C R2D R3A R3B R3C R3D R4A R4B R4C R4D R1R2 R1R3 R1R4 R2R1 R2R3 R2R4 R3R1 R3R2 R3R4 R4R1 R4R2 R4R3 Measurement parameter see list of parameters below TestPortNum Test port number drive port for wave qu...

Страница 1569: ...used to identify the active trace Mk This numeric suffix is ignored and may be set to any value because the bandfilter search functions always use markers M1 to M4 Parameters Bandwidth Range 100 dB t...

Страница 1570: ...Ction EXECute to select a search mode and at the same time initiate the search The CALCulate Ch MARKer Mk SEARch CALCulate Ch MARKer Mk MAXimum or CALCulate Ch MARKer Mk MINimum functions also select...

Страница 1571: ...Mk Marker number Usage Event CALCulate Chn MARKer Mk SEARch IMMediate Initiates a search according to the search function selected with CALCulate Chn MARKer Mk FUNCtion SELect The marker must be crea...

Страница 1572: ...MATH CH1DATA MDATA2 Define a mathematical trace dividing the data trace by the stored memory trace The mathematical trace is not displayed CALC MATH STAT ON Display the mathematical trace instead of...

Страница 1573: ...tenuation Sets the attenuation for the received waves This command is available if at least one of the Receiver step attenuators is installed The generated wave is attenuated via SOURce Ch POWer PhyPt...

Страница 1574: ...t Chn DPORt Port Selects a source port for the stimulus signal drive port The setting acts on the active trace The effect of the drive port selection depends on the measurement parameter associated to...

Страница 1575: ...connector type SMA Note This command only supports ZVR compatible connector types For general definitions use SENSe CORRection CKIT StandardType StandardType Standard type For reflection standards th...

Страница 1576: ...the previously created R S ZVR cal kit file ZCAN ck from the default cal kit directory MMEM STOR CKIT ZCAN C Users Public Documents Rohde Schwarz ZNA Calibration Kits ZCAN calkit Store the imported ca...

Страница 1577: ...he second number denotes the number of the standard 1 to 3 RST ON Dispersion Optional status parameter for UTHRough standard OFF unknown through standard is non dispersive ON unknown through standard...

Страница 1578: ...h does not introduce any systematic errors none of the measurement results acquired previously SENSe Ch CORRection COLLect ACQuire is taken into account Tip The main purpose of the default correction...

Страница 1579: ...continuous mode Usage Event SENSe Ch CORRection COLLect SAVE DUMMy Calculates the system error correction data from the acquired one or two port mea surement results SENSe Ch CORRection COLLect ACQuir...

Страница 1580: ...e Ch CORRection COLLect SAVE DEFault G and H matrices The 7 term calibration types named Txx e g TOM TSM TRM TRL TNA are based on a network analyzer with two ports i and j each equipped with a test re...

Страница 1581: ...ns SCORR11 load match at port 1 SCORR12 reverse transmission tracking between port 2 and port 1 G11 G22 G matrix elements see above H22 H matrix elements see above The error terms are dimensionless co...

Страница 1582: ...NSe Ch CORRection LOSS PhyPt commands to define the complete set of loss offset parameters SENSe Ch CORRection OFFSet PhyPt MAGNitude is equivalent to SENSe Ch CORRection LOSS PhyPt OFFSet Suffix Ch C...

Страница 1583: ...ONV MIX LOF 1 GHz Reset the analyzer and specify a fixed frequency of 1 GHz to be assigned to the LO signal FREQ CONV MIX FFIX FIX Query the fixed frequency and the signal assignment using the alterna...

Страница 1584: ...y MODE FreqSweep Selects the sweep type and defines which set of commands controls the stimulus fre quency Tip The command SENSe Ch SWEep TYPE provides a complete list of sweep types Suffix Ch Channel...

Страница 1585: ...req Defines the frequency vs time characteristics of a frequency sweep Lin Frequency or Log Frequency The command has no effect on segmented frequency power or time sweeps Note Use SENSe Ch SWEep TYPE...

Страница 1586: ...ed Ch4Tr1 to measure the input reflection coefficient S11 The trace automatically becomes the active trace SENS4 FUNC Check query the sweep type and measurement parameter of the active trace The resul...

Страница 1587: ...er calibration for arbitrary power meters use the alternative commands listed in the program example below Note The command cannot be used unless a power meter is connected via GPIB bus USB or LAN int...

Страница 1588: ...mory trace use CALCulate Chn DATA To read the response values of a trace acquired in single sweep mode INITiate Ch CONTinuous OFF use CALCulate Chn DATA NSWeep FIRSt Query parameters Response CH1Data...

Страница 1589: ...H3Mem CH4Mem MDATa1 MDATa2 MDATa3 MDATa4 MDATa5 MDATa6 MDATa7 MDATa8 Stimulus data of the selected trace see Table 7 18 The data is transferred in the data format defined via FORMat DATA Example SWE P...

Страница 1590: ...amples for programming tasks that almost every user will encounter when working with the R S ZNA 8 1 1 Typical stages of a remote control program A typical remote control program comprises the followi...

Страница 1591: ...itch off the measurement after one sweep reduce the number of sweep points RST INITiate1 CONTinuous OFF SENSe1 SWEep POINts 2 Avoid a delay time between different partial measurements and before the s...

Страница 1592: ...f the sweep INITiate1 IMMediate WAI Continue program sequence 2 Start single sweep use OPC If OPC follows INITiate Ch IMMediate it places a 1 into the output queue when the sweep is terminated An appr...

Страница 1593: ...Markers and Limit Lines 2 Read complete trace Select a trace format and read formatted trace data CALCulate1 FORMat MLINear Calculate the linear magnitude of z CALCulate1 DATA FDATa Read the formatted...

Страница 1594: ...ne channel two traces one diagram Reset the instrument creating the default trace Trc1 in channel 1 The default measured quantity is the forward transmission S parameter S21 The default format is dB M...

Страница 1595: ...ED Trc2 DISPlay WINDow3 TRACe3 FEED Trc3 DISPlay WINDow4 TRACe4 FEED Trc4 Check the result on the local screen Go to local SYSTem DISPlay UPDate ONCE 8 1 2 2 Several traces with different channel sett...

Страница 1596: ...race B1 B2 CALCulate3 PARameter SDEFine Z_trace Z21 CALCulate3 PARameter SDEFine Y_trace Y21 CALCulate3 PARameter SELect Z_trace the trace created previously becomes the active trace for channel 3 So...

Страница 1597: ...com mands for marker settings and for the limit check 2 Diagrams are referenced by a window suffix Wnd An additional suffix WndTr in the DISPlay WINDow Wnd TRACe WndTr commands numbers the dif ferent...

Страница 1598: ...ulate1 MARKer1 REFerence X 4 5 GHz Use the delta marker to search for the minimum of the trace and query the result the query returns the stimulus and the response value at the marker position CALCula...

Страница 1599: ...you are looking for is not in this section we suggest you to refer to the short command sequences in the reference chapter Proceed as follows 1 Find your subject in the help system preferably using c...

Страница 1600: ...DEFault MMEMory STORe TRACe Trc1 Traces S21 s1p You may also read the default path MMEMory CDIRectory and use the external RC program to build the complete paths 8 2 2 Trace and diagram handling The f...

Страница 1601: ...Create trace CALCULATE1 PARAMETER SDEFINE Trc2 S21 CALCULATE1 PARAMETER SDEFINE Trc3 S31 CALCULATE1 PARAMETER CATALOG Delete trace CALCULATE1 PARAMETER DELETE Trc2 CALCULATE1 PARAMETER CATALOG Assign...

Страница 1602: ...parameters String Char DISPLAY WINDOW1 TRACE3 FEED Mem3x Trc1 TRACE COPY MDATA4 CH1DATA DISPLAY WINDOW1 TRACE4 FEED Mem4 Trc1 Create new normal trace on channel 1 assign it to a window CALCULATE1 PAR...

Страница 1603: ...TE3 PARAMETER SELECT Trace_Name CALCULATE3 PARAMETER SELECT TRACE COPY XYZ Ch2Trc1 DISPLAY WINDOW2 TRACE3 FEED XYZ CALCULATE2 PARAMETER SELECT XYZ CALCULATE2 PARAMETER SELECT TRACE COPY MDATA4 Ch3Trc1...

Страница 1604: ...to a diagram same for TRC3 TRACE COPY MDATA7 CH1DATA DISPLAY WINDOW1 TRACE3 FEED Mem7 Trc1 TRACE COPY MDATA8 CH2DATA DISPLAY WINDOW2 TRACE2 FEED Mem8 Trc3 Examples for Trace Mathematics Special operan...

Страница 1605: ...uation range and retrieve statistical results Reset the analyzer RST SYSTEM DISPLAY UPDATE ON Create new channel and trace CALCULATE2 PARAMETER SDEFINE Trc2 S11 CALCULATE2 PARAMETER SELECT Trc2 DISPLA...

Страница 1606: ...max CALCulate1 MARKer FUNCtion BWIDth MODE BPASs CALCulate1 MARKer FUNCtion BWIDth MODE BPAS Measure single sweep wait until complete sweep is finished INITiate CONTinuous OFF INITiate WAI CALCulate1...

Страница 1607: ...commands are used to position several dia grams on the screen The remote control commands presented here extend the func tionality of the Display Diagram and Display Split softtool tabs Reset the ana...

Страница 1608: ...0 1 00 DISPlay LAYout DEFine 2 1 1 0 00 0 00 2 1 1 00 1 00 1 00 0 50 1 00 0 50 Join the 2 layouts display the nested layout DISPlay LAYout JOIN 1 2 2 DISPlay LAYout APPLy 1 Check the last applied i e...

Страница 1609: ...Reset the analyzer RST SYSTEM DISPLAY UPDATE ON Initiate a single sweep INITiate1 CONTinuous OFF INITiate1 IMMediate WAI The following marker commands have the channel no as a numeric suffix The para...

Страница 1610: ...example shows how to search for particular measurement points using markers Reset the analyzer RST SYSTEM DISPLAY UPDATE ON Define marker and stimulus range CALCULATE1 MARKER1 ON SENSE1 FREQUENCY STAR...

Страница 1611: ...ATE1 MARKER1 X CALCULATE1 MARKER1 X 1 5GHZ CALCULATE1 MARKER MAX CALCULATE1 MARKER1 X Minimum peak search functions CALCULATE1 MARKER1 FUNCTION SELECT MINIMUM CALCULATE1 MARKER1 FUNCTION SELECT NEXT P...

Страница 1612: ...CT Trc2 DISPLAY WINDOW2 STATE ON DISPLAY WINDOW2 TRACE1 FEED Trc2 CALCULATE3 PARAMETER SDEFINE Trc3 S11 CALCULATE3 PARAMETER SELECT Trc3 DISPLAY WINDOW3 STATE ON DISPLAY WINDOW3 TRACE1 FEED Trc3 Selec...

Страница 1613: ...race with the current extremum This is the last extremum for the next sweep INITIATE IMMEDIATE WAI TRACe COPY MATH LastExtr Trc1 Loop over these 2 commands INITIATE IMMEDIATE WAI TRACe COPY MATH LastE...

Страница 1614: ...lternative Reverse reading with command synchronization Select single sweep mode and measure a single sweep group for channels no 1 and 2 INITIATE CONTinuous ALL OFF INITIATE IMMEDIATE ALL WAI Read tr...

Страница 1615: ...te WAI MMEMory STORe TRACe PORTs 1 ParserTouchstonePorts s1p COMPlex 2 MMEMory STORe TRACe PORTs 1 ParserTouchstonePorts s2p COMPlex 3 2 MMEMory STORe TRACe PORTs 1 ParserTouchstonePorts s4p COMPlex 1...

Страница 1616: ...1 2 SENSe1 CORRection COLLect ACQuire SELected OPEN 1 SENSe1 CORRection COLLect ACQuire SELected SHORT 1 SENSe1 CORRection COLLect ACQuire SELected MATCH 1 SENSe1 CORRection COLLect ACQuire SELected O...

Страница 1617: ...pected result 2 2 3 3 4 4 SENSE1 CORRECTION COLLECT AUTO ASSignment1 DEFine create the second calibration full one port and factory calkit SENSe1 CORRection COLLect AUTO CONFigure FOPort the second ca...

Страница 1618: ...ection COLLect METHod DEFine Test SFK TOSM 12 TOSM 1 2 Measure Standards SENSe1 CORRection COLLect ACQuire SELected THROUGH 1 2 SENSe1 CORRection COLLect ACQuire SELected OPEN 1 SENSe1 CORRection COLL...

Страница 1619: ...ms Reset the analyzer RST Set frequency range SENSe1 FREQuency1 STARt 1GHz SENSe1 FREQuency1 STOP 4GHz SENSe1 SWEep POINts 6 Select calibration type TOSM at ports 1 and 2 SENSe1 CORRection COLLect MET...

Страница 1620: ...transfer FORMAT REAL 32 Important Stop sweep when loading error terms INITiate CONTinuous OFF Recall error terms CALCulate DATA is suitable for 2 port terms use SENSe CORRection CDATa for more than 2...

Страница 1621: ...SFK ARTosm 1 2 Start with calkit 1 e g 3 5 mm ideal Kit adapter side port 1 SENSE1 CORRECTION COLLECT ACQuire SELected OPEN 1 ON SENSE1 CORRECTION COLLECT ACQuire SELected SHORT 1 ON SENSE1 CORRECTIO...

Страница 1622: ...ed to VNA port i SENSe1 CORRection COLLect AUTO ASSignment1 DEFine 1 1 2 2 Step 1 OSM SENSe1 CORRection COLLect AUTO ASSignment1 ACQUire Step 2 Unknown Through SENSe1 CORRection COLLect AUTO UTHRough...

Страница 1623: ...mixer delete all pre existing port assignments SENSE1 CORRECTION COLLECT AUTO ASSignment DELete ALL UOSM vector mixer cal with calu s factory characterization SENSe1 CORRection COLLect AUTO CONFigure...

Страница 1624: ...step SENSe1 CORRection COLLect AUTO POWer 1 CPUOSM calibration A SMARTer UOSM scalar vector mixer calibration using a calibration unit a power meter and a calibration mixer Same procedure as describe...

Страница 1625: ...LO SOURce1 POWer1 CORRection ACQuire PORT 3 calibration of RF port1 Mixer connected SOURce1 POWer1 CORRection ACQuire PORT 1 calibration of IF port2 Mixer connected SOURce1 POWer1 CORRection ACQuire...

Страница 1626: ...et LO frequencies SENSe3 FREQuency1 CONVersion FUNDamental LO fix Start Stop sweep points 1 SENSe3 SWEep POINts 1 SENSe3 FREQuency1 STARt 200 MHz SENSe3 FREQuency1 STOP 200 MHz 8 2 7 RFFE GPIO interfa...

Страница 1627: ...E1 COMMAND DATA B5C38 CONTROL1 RFFE1 COMMAND SEND Read Product ID Register address of product ID is noted in the DUT specification This Example Product ID is stored at address 0x1D Therefore SA 0xB CM...

Страница 1628: ...GPIO 4 and RFFE interfaces 1 for Sweep Sequencer CONTROL GPIO4 STATe ON CONTrol RFFE1 SETTings STATe ON Set default voltage for GPIO Sweep Sequencer list CONTROL GPIO4 VOLTAGE DEF 0 5V Define command...

Страница 1629: ...ulate FMODel ISD1 RUN STATe 1 CALCulate FMODel ISD2 RUN STATe 1 CALCulate FMODel ISD3 RUN STATe 0 CALCulate FMODel ISD4 RUN STATe 0 CALCulate FMODel ISD RUN RUN Measure the DUT together with the test...

Страница 1630: ...er2 VALue 25 noise detector measurement time for measurement SENSe1 NFIGure NTIMe 50ms gain detector measurement time for measurement SENSe1 NFIGure GTIMe 10ms noise detector measurement time for cali...

Страница 1631: ...ds SENSe1 CORRection COLLect ACQuire SELected OPEN 1 SENSe1 CORRection COLLect ACQuire SELected SHORT 1 SENSe1 CORRection COLLect ACQuire SELected MATCh 1 through SENSe1 CORRection COLLect ACQuire SEL...

Страница 1632: ...tched off to avoid damage Hardware errors with possible causes and remedies are listed in the following sections Exceptions indicate anomalous or exceptional events that were not properly han dled by...

Страница 1633: ...op PLL fails For external reference check fre quency and level of the supplied ref erence signal 1 Converter clock fre quency lock failure The clock generator for the AD converter clock is phase locke...

Страница 1634: ...cess the measurement data until the next sweep point starts Increase stop time reduce no of points increase IF bandwidth If pos sible reduce number of partial mea surements e g by restricting the numb...

Страница 1635: ...ou to detect the cause of an error and find a remedy Error message types are described in Chap ter 9 Error messages and troubleshooting on page 1632 In addition the system Info dialog offers valuable...

Страница 1636: ...customer support Technical support where and when you need it For quick expert help with any Rohde Schwarz product contact our customer sup port center A team of highly qualified engineers provides s...

Страница 1637: ...tection and packing material designed for the product If you do not have the original packaging use similar materials that provide the same level of protection Securing When moving the product in a v...

Страница 1638: ...posal Rohde Schwarz is committed to making careful ecologically sound use of natural resources and minimizing the environmental footprint of our products Help us by dis posing of waste in a way that c...

Страница 1639: ...storage drive an external storage medium USB mem ory stick external CD ROM drive or a network connection LAN The default name of the internal drive is C External storage devices are automati cally map...

Страница 1640: ...setting 12 1 2 1 Assigning an IP address Depending on the network capacities the TCP IP address information for the analyzer can be obtained in different ways If the network supports dynamic TCP IP c...

Страница 1641: ...board to access Windows 3 Open the Control Panel Network and Sharing Center Change adapter set tings dialog and right click the local connection 4 Select Properties and confirm the user account contro...

Страница 1642: ...me the instrument is restarted Each instrument is delivered with an assigned computer name but this name can be changed The default instrument name is a case insensitive string with the following synt...

Страница 1643: ...firewall settings A firewall protects an instrument by preventing unauthorized users from gaining access to it through a network In the default configuration of the R S ZNA the firewall is enabled A...

Страница 1644: ...s to complete 3 Reboot the instrument After the restore upgrade to the desired firmware version see Chapter 12 1 1 Firm ware installation on page 1639 12 3 Interfaces and connectors This chapter provi...

Страница 1645: ...e TTL1 Pass TTL2 Pass 14 UC_PASS2 O Pass fail result of limit check 2 TTL Out Pass 2 15 AGND Ground 16 UC_DRV_PORT1 O Used as drive ports OUTPut UPORt ECBits OFF or channel bits OUTPut UPORt ECBits ON...

Страница 1646: ...n RJ 45 connector a network interface card and protocols The network interface card supports IEEE 802 3 for a 10 Mbps Ethernet and IEEE 802 3u for a 100 Mbps Ethernet Instrument access is possible via...

Страница 1647: ...e GPIB bus interface is intended for remote control of the R S ZNA from a controller Characteristics of the interface 8 bit parallel data transfer Bidirectional data transfer Three line handshake High...

Страница 1648: ...the data bus The R S ZNA provides several functions to communicate via GPIB bus They are described in the following sections 12 3 3 1 Interface functions Instruments which can be controlled via GPIB b...

Страница 1649: ...d of the parallel poll state Addressed commands Addressed commands are encoded in the range 00 through 0F hex They are only effective for instruments addressed as listeners Command QuickBASIC com mand...

Страница 1650: ...tandard 25 pin female D sub connector pro viding 2 independent RF Front End RFFE interfaces according to the MIPI Alliance System Power Management Interface Specification and 10 General Purpose Input...

Страница 1651: ...age 0 2 5 0 001 High voltage 0 2 5 0 001 MAX mA Current 20 MIN kHz MAX kHz Possible values kHz Clock frequency 31 25 26000 52000 n with n 1664 2 All remaining data e g rise time are according to the s...

Страница 1652: ...the current values are valid if the GPIO voltages are within 5 V to 9 V 12 4 Showroom mode In Showroom Mode the R S ZNA uses a configurable recall set whenever the device is re started or the Preset...

Страница 1653: ...ding PhyPt STATe CALCulate 1 4 FSIMulator SENDed PMCir cuit PORT Pt TYPE NONE PCSC PCSL PLPC PLSC PLSL SCPC SCPL SLPC SLPL USER CALCulate Ch TRANsform VNETworks SENDed EMBedding PhyPt TNDefinition CAL...

Страница 1654: ...TA CALCulate Chn LIMit DATA CALCulate 1 4 SELected MARKer 1 10 COU Ple ON OFF 1 0 CALCulate MARKer COUPled STATe CALCulate 1 4 SELected MARKer 1 10 FUNC tion TYPE MAXimum MINimum PEAK LPEak RPEak TARG...

Страница 1655: ...Tem DISPlay UPDate DISPlay WINDow 1 4 ACTivate numeric_value INSTrument NSELect DISPlay WINDow 1 4 MAXimize ON OFF 1 0 DISPlay WINDow Wnd MAXimize DISPlay WINDow SPLit DISPlay WINDow 1 4 TITLe DATA st...

Страница 1656: ...MEMory STORe TRACe PORTs MMEMory STORe SNP TYPE S4P numeric3 numeric2 numeric3 numeric4 MMEMory STORe TRACe PORTs MMEMory STORe STYPe STATe CSTate DSTate CDSTate MMEMory STORe STATe Always saves measu...

Страница 1657: ...SOLT1 numeric SENSe 1 4 CORRection COL Lect METHod SOLT2 numeric1 numeric2 SENSe 1 4 CORRection COL Lect METHod SOLT3 numeric1 numeric2 numeric3 SENSe 1 4 CORRection COL Lect METHod SOLT4 1 2 3 4 SENS...

Страница 1658: ...INear SEGMent SENSe Ch SWEep TYPE SERVice CHANnel COUNt n a no limit SERVice CHANnel TRACe COUNt n a no limit SOURce 1 4 POWer LEVel IMMediate AMPLi tude numeric SOURce Ch POWer PhyPt LEVel IMMediate...

Страница 1659: ...TATus QUEStionable LIMit EVENt STATus QUEStionable LIMit Lev EVENt STATus QUEStionable LIMit NTRansition numeric STATus QUEStionable LIMit Lev NTRansition STATus QUEStionable LIMit PTRansition numeric...

Страница 1660: ...channel that has been selected as the active trace CALCulate Ch PARameter SELect trace name Many commands e g TRACE act on the active trace It can be different from the active trace in manual control...

Страница 1661: ...screen used to display traces Diagram areas are arranged in windows they are independent of trace and channel settings Directivity error Measurement error caused by a coupler or bridge in the analyze...

Страница 1662: ...ches 0 dB Intermodulation measurement Measurement where the DUT is supplied with two RF signals of equal power but different frequencies termed the upper and lower tone The analyzer measures the frequ...

Страница 1663: ...on between constants and the data or memory traces of the active recall set Measurement point Result of the measurement at a specified stimulus value fre quency power time Measurement result Set of al...

Страница 1664: ...ion Stimulus value Value of the sweep variable frequency power time point number where a measurement is taken Also termed sweep point Sweep Series of consecutive measurements taken at a specified sequ...

Страница 1665: ...assumed to be ideally matched Beyond that the through must be lossless and its length must be exactly known Especially useful for DUTs in planar line technology TRM A calibration type which requires a...

Страница 1666: ...NVerter Port CSSLope 1237 SENSe CONVerter Port IFPort 1238 SENSe CONVerter Port IFRequency 1238 SENSe CONVerter Port LOLoss 1238 SENSe CONVerter Port LOPort 1239 SENSe CONVerter Port RFLoss 1239 SENSe...

Страница 1667: ...408 SENSe UDSParams Pt PARam 1408 SENSe Ch AVERage CLEar 1226 SENSe Ch AVERage COUNt 1226 SENSe Ch AVERage MODE 1227 SENSe Ch AVERage STATe 1226 SENSe Ch BANDwidth RESolution 1228 SENSe Ch BANDwidth R...

Страница 1668: ...Se Ch CORRection COLLect AUTO TYPE 1287 SENSe Ch CORRection COLLect AUTO UTHRough 1289 SENSe Ch CORRection COLLect CHANnels CSETup 1269 SENSe Ch CORRection COLLect CKIT INSTall 1289 SENSe Ch CORRectio...

Страница 1669: ...OSS PhyPt SECond 1312 SENSe Ch CORRection LOSS PhyPt SECond FREQuency 1313 SENSe Ch CORRection LOSS PhyPt SECond STATe 1314 SENSe Ch CORRection METas CABLe MOVement 1260 SENSe Ch CORRection METas CABL...

Страница 1670: ...er STAGes 1335 SENSe Ch FREQuency CONVersion MIXer TFRequency Stg 1336 SENSe Ch FREQuency FIXed 1324 SENSe Ch FREQuency IMODulation CONVersion 1336 SENSe Ch FREQuency IMODulation LTONe 1337 SENSe Ch F...

Страница 1671: ...IGure CALibration GTIMe 1351 SENSe Ch NFIGure CALibration NTIMe 1351 SENSe Ch NFIGure DEFine 1351 SENSe Ch NFIGure GTIMe 1352 SENSe Ch NFIGure HARMonic MAXFrequency 1352 SENSe Ch NFIGure HARMonic MAXi...

Страница 1672: ...NSe Ch PULSe GENerator Id TRAin SEGMent Seg STARt 1369 SENSe Ch PULSe GENerator Id TRAin SEGMent Seg STATe 1369 SENSe Ch PULSe GENerator Id TRAin SEGMent Seg STOP 1370 SENSe Ch PULSe GENerator Id TRAi...

Страница 1673: ...92 SENSe Ch SEGMent Seg SWEep POINts 1392 SENSe Ch SEGMent Seg SWEep TIME 1393 SENSe Ch SEGMent Seg SWEep TIME CONTrol 1394 SENSe Ch SEGMent Seg SWEep TIME SUM 1394 SENSe Ch SEGMent Seg STATe 1377 SEN...

Страница 1674: ...s USER COUNt 932 CALCulate FMODel DELT Ph_pt FREQuencies USER DELete 932 CALCulate FMODel DELT Ph_pt FREQuencies USER DELete ALL 932 CALCulate FMODel DELT Ph_pt FREQuencies USER 931 CALCulate FMODel D...

Страница 1675: ...pt COUPon MEASure 945 CALCulate FMODel ISD Ph_pt COUPon MEASure FILename 946 CALCulate FMODel ISD Ph_pt COUPon MEASure FILename CLEar 946 CALCulate FMODel ISD Ph_pt COUPon MEASure OPEN 946 CALCulate F...

Страница 1676: ...h_pt RUN STATe 959 CALCulate FMODel SFD Ph_pt TOTaldiffcfg 959 CALCulate LIMit CIRCle FAIL ALL 966 CALCulate LIMit FAIL ALL 966 CALCulate LIMit FAIL DATA 966 CALCulate MARKer COUPled TYPE 983 CALCulat...

Страница 1677: ...ential EMBedding LogPt STATe 1076 CALCulate Ch TRANsform VNETworks FSIMulator STATe 1088 CALCulate Ch TRANsform VNETworks GLOop DEEMbedding group PARameters C 1080 CALCulate Ch TRANsform VNETworks GLO...

Страница 1678: ...orm VNETworks SENDed EMBedding PhyPt PARameters L Cmp 1105 CALCulate Ch TRANsform VNETworks SENDed EMBedding PhyPt PARameters R Cmp 1106 CALCulate Ch TRANsform VNETworks SENDed EMBedding PhyPt TNDefin...

Страница 1679: ...TATe 915 CALCulate Chn EYE MASK SHAPe BOTTom VERTical 915 CALCulate Chn EYE MASK SHAPe POLYgon HORizontal 916 CALCulate Chn EYE MASK SHAPe POLYgon STATe 915 CALCulate Chn EYE MASK SHAPe POLYgon TYPE 9...

Страница 1680: ...1565 CALCulate Chn LIMit CONTrol SHIFt 970 CALCulate Chn LIMit CONTrol DATA 969 CALCulate Chn LIMit DATA 970 CALCulate Chn LIMit DCIRcle CLEar 971 CALCulate Chn LIMit DCIRcle DATA 971 CALCulate Chn LI...

Страница 1681: ...CALCulate Chn MARKer Mk FUNCtion CENTer 992 CALCulate Chn MARKer Mk FUNCtion DELTa STATe 1569 CALCulate Chn MARKer Mk FUNCtion DOMain USER SHOW 993 CALCulate Chn MARKer Mk FUNCtion DOMain USER STARt 9...

Страница 1682: ...ate Chn RIPPle DATA 1024 CALCulate Chn RIPPle DELete ALL 1025 CALCulate Chn RIPPle DISPlay STATe 1026 CALCulate Chn RIPPle FAIL 1026 CALCulate Chn RIPPle RDOMain FORMat 1026 CALCulate Chn RIPPle SEGMe...

Страница 1683: ...m COMPlex 1044 CALCulate Chn TRANsform DTFault CENTer 1054 CALCulate Chn TRANsform DTFault DEFine 1053 CALCulate Chn TRANsform DTFault DELete 1054 CALCulate Chn TRANsform DTFault PEAK COUNt 1055 CALCu...

Страница 1684: ...ACe Trc NAME ID 1116 CONFigure TRACe Trc REName 1116 CONTrol AUXiliary C DATA 1118 CONTrol GPIO SENSe SUMCurrent 1120 CONTrol GPIO Port SENSe CURRent 1120 CONTrol GPIO Port SENSe VOLTage 1119 CONTrol...

Страница 1685: ...TA 1131 CONTrol Ch SEGMent Nr SEQuence CLEar ALL 1130 CONTrol Ch SEGMent Nr SEQuence COUNt 1129 CONTrol Ch SEQuence CLEar ALL 1131 CONTrol Ch SEQuence COUNt 1131 CONTrol Ch SEQuence Nr DELay 1132 CONT...

Страница 1686: ...1155 DISPlay CMAP TRACe COLor STATe 1157 DISPlay CMAP TRACe RGB 1158 DISPlay CMAP DispEl RGB 1156 DISPlay IWINdow BFILter STATe 1139 DISPlay IWINdow MARKer Mk STATe 1139 DISPlay IWINdow STATe 1139 DI...

Страница 1687: ...DISPlay WINDow Wnd STATe 1140 FORMat BORDer 1165 FORMat DEXPort SOURce 1572 FORMat DATA 1164 HCOPy DESTination 1166 HCOPy DEVice LANGuage 1166 HCOPy ITEM ALL 1167 HCOPy ITEM LOGO STATe 1167 HCOPy ITE...

Страница 1688: ...OAD EYE MASK 1192 MMEMory LOAD LIMit 1192 MMEMory LOAD MDAData 1539 MMEMory LOAD MDCData 1539 MMEMory LOAD RIPPle 1194 MMEMory LOAD SEGMent 1195 MMEMory LOAD STATe 1196 MMEMory LOAD TRACe 1196 MMEMory...

Страница 1689: ...LUS 1215 MMEMory STORe TRACe OPTion SSEParator 1215 MMEMory STORe TRACe OPTion SYMMetric 1214 MMEMory STORe TRACe OPTion TABS 1216 MMEMory STORe TRACe OPTion TRIM 1216 MMEMory STORe TRACe PORTs 1216 O...

Страница 1690: ...13 SOURce Ch FREQuency PhyPt CONVersion ARBitrary IFRequency 1414 SOURce Ch FREQuency PhyPt FIXed 1412 SOURce Ch FREQuency PhyPt CW 1412 SOURce Ch GROup COUNt 1420 SOURce Ch GROup Grp 1420 SOURce Ch G...

Страница 1691: ...r PhyPt CORRection NREadings 1455 SOURce Ch POWer PhyPt CORRection OSOurces STATe 1455 SOURce Ch POWer PhyPt CORRection PSELect 1456 SOURce Ch POWer PhyPt CORRection SLEVeling FREQuency STARt 1438 SOU...

Страница 1692: ...PORt 1475 SOURce Ch TDIF IMBalance AMPLitude STARt 1476 SOURce Ch TDIF IMBalance AMPLitude STOP 1476 SOURce Ch TDIF IMBalance PHASe LPORt 1477 SOURce Ch TDIF IMBalance PHASe STARt 1477 SOURce Ch TDIF...

Страница 1693: ...m COMMunicate RDEVice GDEV Gdev DELete 1495 SYSTem COMMunicate RDEVice GDEVice CATalog 1493 SYSTem COMMunicate RDEVice GDEVice DELete ALL 1494 SYSTem COMMunicate RDEVice GDEVice Gdev DEFine 1494 SYSTe...

Страница 1694: ...INFO 1507 SYSTem ERRor DISPlay STATe 1507 SYSTem ERRor DISPlay WARNings 1507 SYSTem ERRor DISPlay REMote 1506 SYSTem ERRor NEXT 1506 SYSTem FIRMware UPDate 1508 SYSTem FPReset 1508 SYSTem FREQuency 15...

Страница 1695: ...CHANnel Ch AUXiliary n INTerval 1530 TRIGger CHANnel Ch AUXiliary n POSition 1530 TRIGger CHANnel Ch AUXiliary n STYPe 1531 TRIGger STATus READy 1531 TRIGger WAIT READy 1532 TRIGger SEQuence LTRigger...

Страница 1696: ...g tab 737 Eye Mask Test tab 752 Rise Time tab 757 Skew tab 758 TDR application 730 TDR Setup tab 731 Time Gate tab 760 X Axis tab 761 Y Axis tab 760 Application cards 25 Application notes 25 Apply cal...

Страница 1697: ...l Kit 563 Standards 563 Calibration labels scalar power calibration 195 SMARTerCal 199 system error correction 178 Calibration Manager dialog 583 584 Add 584 Apply 584 Apply to All 584 Channel and cal...

Страница 1698: ...n 393 Data base 862 Data bus GPIB 1647 Data entry 66 Data Entry panel 42 Data flow 93 Data sheets 25 Data Streaming 46 Data to Destination 393 Data Valid 1647 DAV 1647 dB Mag 374 DC Value 407 DCL 1646...

Страница 1699: ...rors 1632 Asynchronous 1633 During firmware update 1634 During measurement 1633 FPGA update 1634 ESB 870 ESE 866 871 ESR 871 Evaluation of data 80 Evaluation Range Dialog 415 EVENt register 867 Execut...

Страница 1700: ...R S ZNA K19 259 Front panel 39 Front panel keys 48 Fullscreen mode 784 Function keys 40 Channel section 41 Stimulus section 41 System section 41 Trace section 41 G Gain Compression tab 346 Generator e...

Страница 1701: ...Internal second LO generator 274 Internal second source for 2 port R S ZNA 274 Inv Smith 378 Inverted Smith chart 119 IP Address 814 IP address setting 1640 ISD Advanced settings 681 IST flag 866 871...

Страница 1702: ...x softkey trace scale 383 Max Marker 471 MAXimum 856 Meas Power Sensor 350 S Params 303 Meas Delay 490 Meas softtool 300 Gain Compression tab 346 Intermodulation tab 334 Power Sensor tab 350 Ratio tab...

Страница 1703: ...l 660 Ground Loop panel 658 Impedance Renormalization panel 659 Offset 652 Overview 651 Port Sets panel 655 Single Ended panel 654 Offset Embed softtool 651 Balanced tab 690 Delta L tab 698 Differenti...

Страница 1704: ...ts 542 Power calibration 191 measurement receiver 194 Power Meter 574 Procedure 515 reference receiver 193 Settings 572 source 193 Switch Off Other Sources 573 Power Config dialog 478 Power Meter Auto...

Страница 1705: ...ith manual control 851 Remote desktop 1642 Remote Language 814 Remote Preset Configuration 787 Remote Settings 813 Advanced 815 Define IDN OPT 815 GPIB Address 814 Instrument Messages 816 IP Address 8...

Страница 1706: ...ect Parameter dialog 427 Select Ports dialog 427 Selectivity 486 Selftest 808 Selftest info 808 Sequential command execution 862 Serial poll 877 Service Function 810 811 Password 811 Service Function...

Страница 1707: ...weep Active Channel 514 Sweep All Channels 514 Sweep Control Restart Manager dialog 513 Sweep Control tab 510 Sweep History 1613 Sweep mode Continuous 511 Single 511 Sweep range selection 77 Sweep ran...

Страница 1708: ...75 Polar 376 Real 377 Smith 375 SWR 376 Unwr Phase 376 Trace formats 113 Trace Info 777 Trace keys 41 Trace list 99 Trace Manager dialog 390 Add 391 Couple All Channels 392 Couple All Scales 392 Decou...

Страница 1709: ...Prefix 793 Show Sweep Symbols 792 Sounds 791 Transparent Info Fields 792 Units Prefix 793 Use Default Tab for Hardkey 792 User Port 1644 Connector 46 TTL Pass output 797 UTF 8 786 V Vector mixer cali...

Отзывы:

Нет отзывов

Похожие инструкции для 1332.4500K22

Blancett B3100 Series

Бренд: Badger Meter Страницы: 16

Бренд: Badger Meter Страницы: 26

Бренд: Badger Meter Страницы: 48

Бренд: F&F Страницы: 2

Бренд: R.M. Young Страницы: 2

Бренд: janitza Страницы: 6

Бренд: janitza Страницы: 36

Бренд: Raycus Страницы: 40

Бренд: RaycoWylie Страницы: 31

Бренд: X-Rite Страницы: 45

Бренд: X-Rite Страницы: 22

Бренд: Yamaha Страницы: 10

Бренд: Yamaha Страницы: 38

Бренд: Yamaha Страницы: 45

Бренд: Orange Electronic Страницы: 21

Бренд: Yamaha Страницы: 12

Бренд: Hanna Instruments Страницы: 2

Бренд: Toro Страницы: 60

Бренды по названию

0 1 2 3 4 5 6 7 8 9 A B C D E F G H I J K L M N O P Q R S T U V W X Y Z

Популярные бренды

Загрузить еще бренды