Miller SRL2-M Series
Rodless Cylinders
26
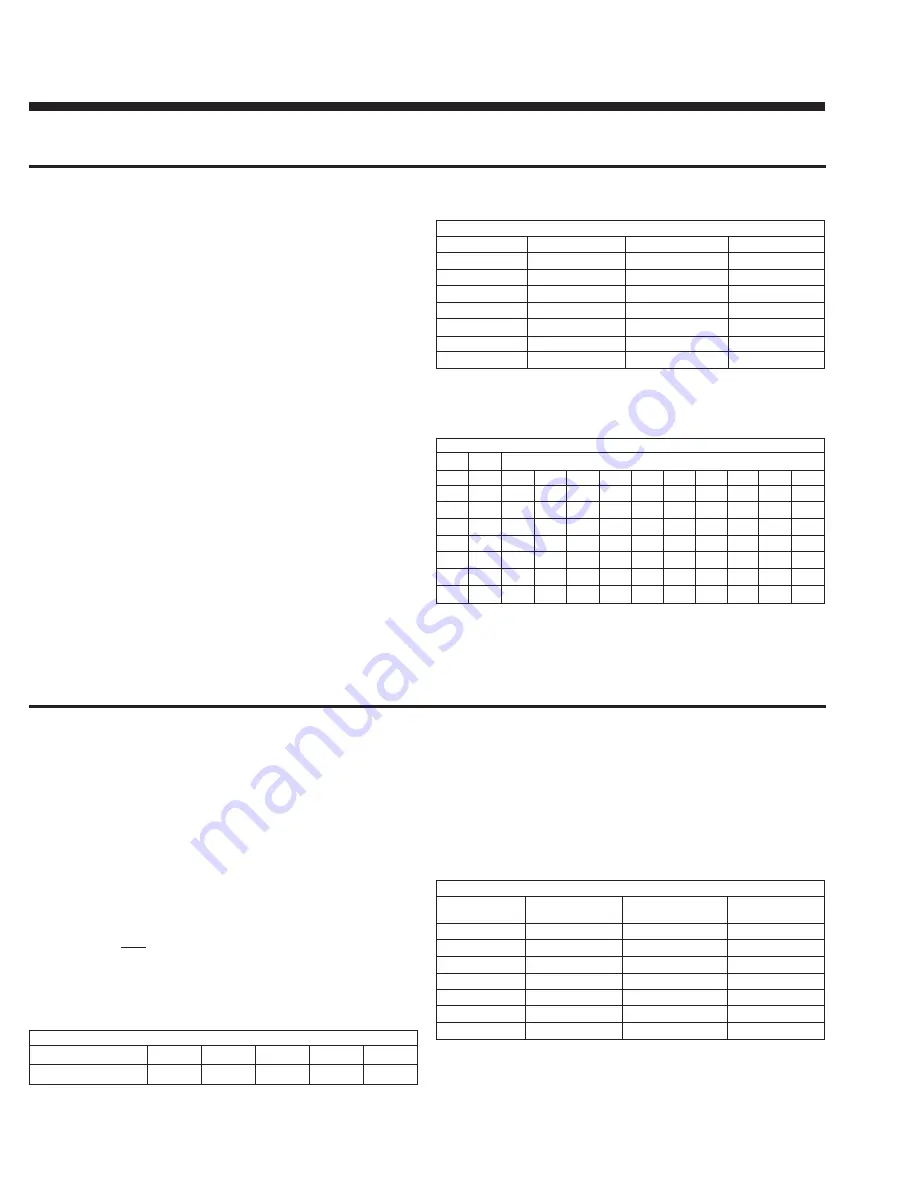
Moment Friction Factor
Bore mm
C1
C2
C3
16
.18
.61
.18
20
.15
.53
.15
25
.13
.41
.13
32
.10
.33
.10
40
.10
.28
.10
50
.10
.23
.10
63
.08
.20
.08
Figure 12
Maximum Kinetic Energy [E] Absorption
Bore
Cushion
With Cushion
W/out Cushion
length in.
in.- lbs.
in.-lbs.
16
0.75
1.95
0.06
20
0.87
5.22
0.09
25
0.82
12.39
0.13
32
0.92
22.75
0.27
40
0.94
37.79
0.44
50
0.98
80.81
0.64
63
1.16
154.01
1.22
Figure 15
Figure 14
Figure 13
Load Factor and Forces
The Load Factor must be determined to ensure that the cylinder force
available is adequate to overcome the total resistive force plus the
additional friction caused by the load moments. This is done by
calculating the frictional forces (Ff) created by the moments and the
weight, using the formulas and the chart in Figure 12, and adding the
resistive force (Fx), which is determined by the actual application.
Ff 1 = M1 x C1
Ff 2 = M2 x C2
Ff 3 = M3 x C3
Fwf (Weight Frictional Force) = .2 x (W
T
)
The sum of all resistive forces (F) including friction is determined as
follows:
Horizontal Mountings:
F = Ff1 + Ff2 + Ff3 + Fwf + Fx
Vertical Mountings:
F = Ff1 + Ff2 + Ff3 + W
T
+ Fx
Actual Cylinder Force (Fa) available is shown in Figure 13.
Load Factor (LF) is determined as follows:
%LF = F/Fa x 100
Load Factor (LF) should never exceed 50%. If LF does exceed 50%, it
will be necessary to select the next larger bore size and calculate its
load factor.
Deceleration and Energy Absorption
Actual Cylinder Force (Fa) in Lbs.
Effective
Pressure PSI
Area
Bore Sq.In. 15
20
30
40
50
60
70
80
90
100
16
0.33
—
—
6
10
13
16
19
22
25
28
20
0.48
—
7
11
15
20
24
28
33
37
41
25
0.84
—
11
19
26
34
41
49
56
64
71
32
1.26
—
17
28
39
50
62
73
84
95
107
40
1.96
—
27
44
61
78
97
114
131
148
166
50
3.08
35
42
69
96
123
152
179
206
233
260
63
4.86
55
66
109
152
194
239
283
325
368
411
Load Factor Coefficient
Load Factor
10%
20%
30%
40%
50%
K
1.15
1.30
1.45
1.60
1.75
The kinetic energy (E) to be absorbed during deceleration must be
calculated to determine whether the cushion can absorb the load or an
external shock absorber may be required. To calculate this, first
determine the actual speed (V) at which the piston enters the cushion by
multiplying the load factor coefficient (K), from Figure 14, times the
average speed (V1) that the piston travels. V1 is derived from actual
cycle time determined by the application requirement.
The formula is as follows:
V (In. per Sec.) = KV1
E (In.-Lbs.)
= W
T
V
2
772
The following chart, Figure 15 shows the maximum energy absorption
for each size cylinder, both with cushions and without cushions. If the
requirements for your application fall below the number shown for the
size of the cylinder you have selected, then no external shock absorber
may be required, but inertia forces and moments should be considered.
www.comoso.com







































