- 8 -
faster surface propagation velocity of HP
diaphragms. Combining properties of enforcement
material A, B and C, sound quality giving the
sensation of the fastest response, which has not
been available, is now an actuality.
E) Super damping fiber: Cellgaia pulp
F) Matrix material:bio-cellulose
This material elevates bonding strength of each pulp
and enforcement materials, and permits diaphragms
of high airtightness to be formed due to the fiber
filament diameter being as fine as only 30nm,
which no other pulp cones have ever materialized.
G) Secondary impregnant
Smooth roll-off response can only be achieved with
an appropriate thickness-stiffness parameter.
Previous techniques do not allow for compatibility
of thickness-stiffness parameter and propagation
velocity, requiring that propagation velocity be
sacrificed to a certain extent to attain the desired
thickness-stiffness parameter. Fostex has succeeded
in development of new materials which will allow
us to achieve ideal internal loss without impairing
propagation velocity. The fruit of our labor is the
use of Cellgaia pulp resulting in an increase in
internal loss without resorting to reduction in
propagation velocity of materials.
Sound quality of wood pulp cones is said to
fluctuate depending on ambient humidity.
The woofer for NF-1 is engineered to be humidity-
resistant to an extent incomparable to previous
wood pulp-based vibratory systems, by using
special nitrocellulose materials capable of
0.001
0.002
0.003 0.004 0.005
0.008 0.01
0.02
0.03 0.04 0.05 0.06 0.08 0.1
0.2
0.1
0.2
0.3
0.4
0.5
0.6
0.7
0.8
0.9
1.0
2.0
3.0
4.0
5.0
6.0
7.0
8.0
9.0
10.0
20.0
30.0
40.0
50.0
60.0
70.0
80.0
90.0
100.0
200.0
300.0
Polystyrene (3)
Hard urethane foam (6)
Polyethylene foam (25)
Polyethylene (62)
Polypropylene
PP (148)
Wood pulp
(178)
Aluminum (19)
Titanium (11)
Magnesium
(91)
Carbon fiber cone (754)
Carbon cloth (167)
Aramid cloth/epoxy (660)
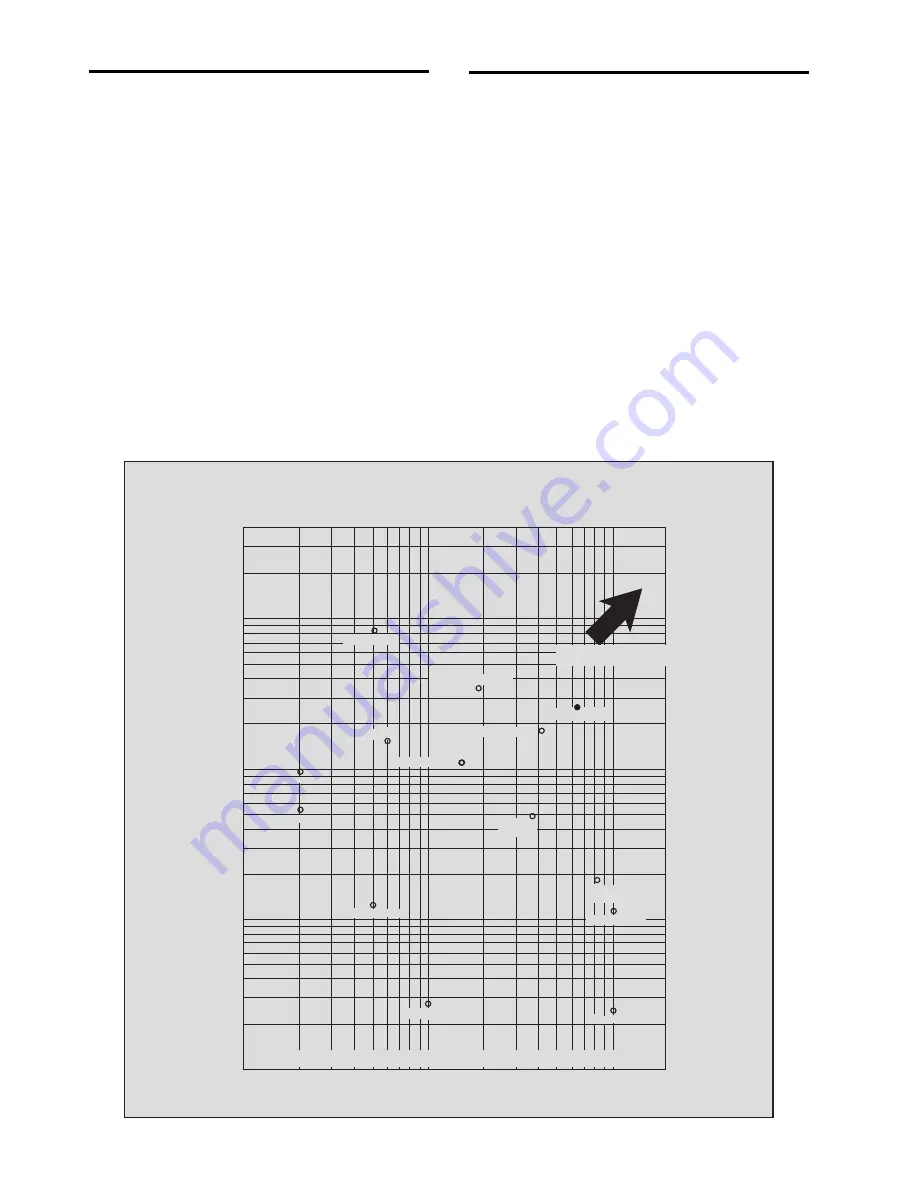
Internal loss(tan
δ
)
Pr
opagation velocity
X
Modulus of flexural rigidity:E/
ρ
2
X
10
3
()Figures in parenthesis represent propagation velocit
X
xflexural rigidity
X
tan
δ
= E/
ρ
2
X
tan
δ
.
Physical properties of diaphragms (internal loss/propagation
velocity/modulus of flexural rigidity)
NF-1 diaphragm
Beryllium (417 )
Optimum performance


































