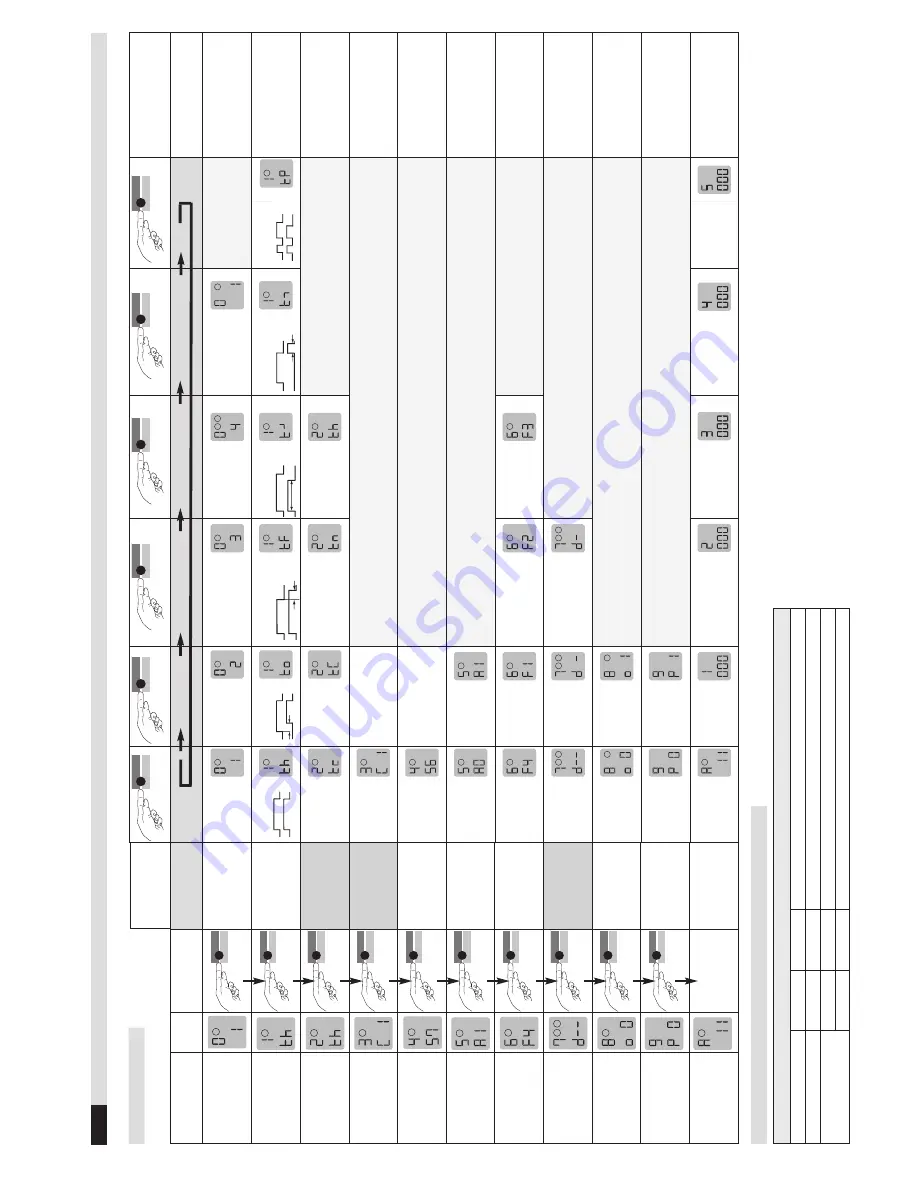
Fu
nc
ti
on
LC
D
di
sp
la
y
B
ut
to
n
op
er
at
io
n
fu
nc
ti
on
s
0
-
B
as
ic
f
un
ct
io
n
1
M
o
d
e
S
im
1
1
-
Ti
m
e
fu
nc
ti
on
1
M
o
d
e
S
im
1
2
-
Ti
m
e
un
it
1
M
o
d
e
S
im
1
Th
is
d
is
pl
ay
d
oe
s
no
t
ap
pe
ar
w
it
h
ti
m
e
fu
nc
ti
on
t
h
(
∞
)
3
-
Ti
m
e
fa
ct
or
1
M
o
d
e
S
im
1
Th
is
d
is
pl
ay
d
oe
s
no
t
ap
pe
ar
w
ih
t
im
e
fu
nc
ti
on
t
h
(
∞
)
4
-
S
en
si
ti
vi
ty
1
M
o
d
e
S
im
1
5
=
S
en
si
ti
vi
ty
5
-
A
ut
om
at
ic
S
en
si
tiv
ity
B
oo
st
A
S
B
1
M
o
d
e
S
im
1
A
S
B
s
ta
nd
s
fo
r
A
ut
om
at
ic
S
en
si
ti
-
vi
ty
B
oo
st
6
-
Fr
eq
ue
nc
y
1
M
o
d
e
S
im
1
7
-
D
ir
ec
ti
on
lo
gi
c
1
2
M
o
d
e
S
im
1
Th
is
d
is
pl
ay
a
pp
ea
rs
on
ly
w
ith
a
2-
lo
op
d
ev
ic
e
8
-
O
ut
pu
t
2
co
nf
ig
ur
at
io
n
1
M
o
d
e
S
im
1
9
-
Pr
ot
ec
ti
on
ag
ai
ns
t
po
w
er
fa
ilu
re
M
o
d
e
S
im
1
A
-
O
pe
ra
ti
ng
m
od
e
1
3
D
a
ta
S
im
2
D
a
ta
S
im
2
D
a
ta
S
im
2
D
a
ta
S
im
2
D
a
ta
S
im
2
D
a
ta
S
im
2
N
ot
es
D
oo
r/
ga
te
sy
st
em
s*
1
B
ar
rie
r s
ys
te
m
s
1
Q
ui
es
ce
nt
c
ur
re
nt
1
D
ire
ct
io
n
lo
gi
c
1
2
O
nl
y
2-
lo
op
d
ev
ic
e:
Lo
o
p
2
ac
tiv
at
ed
: «
1»
*
de
ac
tiv
at
ed
: «
0»
2
W
it
h
de
ac
ti
va
ti
on
o
f
Lo
op
2
th
e
ou
tp
ut
2
b
ec
om
es
co
nf
ig
ur
ab
le
g
8
∞
*
1
O
n
de
la
y
1
O
ff
d
el
ay
1
A
ct
iv
at
io
n
pu
ls
e
lo
op
1
Ti
m
e
fu
nc
t.
pu
ls
e
w
he
n
lo
op
is
e
xi
te
d
1
M
ax
. p
re
se
nc
e
1
0.
1
se
co
nd
1
1
se
co
nd
*
1
1
m
in
ut
e
1
1
ho
ur
1
Th
e
ti
m
e
un
it
m
ul
ti
pl
ie
d
by
th
e
ti
m
e
fa
ct
or
g
iv
es
t
he
se
t
ti
m
e.
1*
1
S
et
v
al
ue
b
et
w
ee
n
1
an
d
99
b
y
to
uc
hi
ng
o
r
ho
ld
in
g
th
e
«D
at
a»
bu
tt
on
4*
1
S
et
v
al
ue
b
et
w
. 1
(l
ow
es
t
) a
nd
9
(h
ig
he
st
s
en
si
.)
by
to
uc
hi
ng
o
r h
ol
di
ng
th
e
«D
at
a»
b
ut
to
n
S
et
ti
ng
r
es
tr
ic
ti
on
s:
ro
te
ct
io
n
ag
ai
ns
t
po
w
er
fa
ilu
re
(w
it
h
P1
):
V
al
ue
1
-5
S
w
it
ch
ed
o
ff
*
1
S
w
it
ch
ed
o
n
1
Fr
eq
ue
nc
y
F4
*
1
Fr
eq
ue
nc
y
F1
1
Fr
eq
ue
nc
y
F2
1
Fr
eq
ue
nc
y
F3
1
B
ot
h
di
re
ct
i-
on
s*
1
2
Lo
op
2
t
o
lo
op
1
1
2
Lo
op
1
t
o
lo
op
2
1
2
Th
e
di
re
ct
io
n
lo
gi
c
fu
nc
ti
on
ca
n
on
ly
b
e
im
pl
em
en
te
d
w
it
h
2
lo
op
s
an
d
a
2-
lo
op
de
vi
ce
O
ut
pu
t
2
is
sw
it
ch
ed
o
ff
2
O
ut
pu
t
2
is
ac
ti
va
te
d
2
Lo
op
2
h
as
t
o
be
de
ac
ti
va
te
d
«0
»
S
w
itc
he
d
of
f*
S
w
itc
he
d
on
If
pa
ra
m
et
er
9
=
P
1
pa
ra
m
et
er
5
m
us
t b
e
se
t t
o
of
f
(
5
=
A0
).
O
pe
ra
tin
g
m
od
e
1
Er
ro
r
m
em
or
y
sl
ot
1
Er
ro
r
m
em
or
y
sl
ot
2
Er
ro
r
m
em
or
y
sl
ot
3
Er
ro
r
m
em
or
y
sl
ot
3
Er
ro
r
m
em
or
y
sl
ot
5
Po
ss
ib
le
d
is
pl
ay
s
in
c
as
e
of
er
ro
r:
s
ee
c
ha
pt
er
6
o
f
th
es
e
op
er
at
in
g
in
st
ru
ct
io
ns
B
ut
to
n
op
er
at
io
n
pa
ra
m
et
er
4
.1
1
C
o
n
fi
g
u
ra
ti
o
n
m
o
d
e
Ta
bl
e
4.
1a
S
et
ti
ng
s
Ta
bl
e
4.
11
b
D
if
fe
re
nt
p
ro
du
ct
v
ar
ia
nt
s
(s
et
ti
ng
o
pt
io
ns
)
*
Fa
ct
or
y
se
tt
in
g
N
ot
e
on
2
-l
oo
p
de
vi
ce
: A
ft
er
lo
op
1
h
as
b
ee
n
se
t,
t
he
p
ar
am
et
er
s
fo
r
lo
op
2
a
re
s
et
(m
ak
e
th
e
se
tt
in
gs
u
si
ng
t
he
s
am
e
pr
oc
ed
ur
e)
a
nd
t
he
s
et
ti
ng
s
ar
e
no
t
sh
ow
n
in
t
he
t
ab
le
w
it
h
th
e
ex
ce
pt
io
n
of
t
he
d
ir
ec
ti
on
lo
gi
c
Lo
op
R
el
ay
tt
Pr
oL
oo
p2
O
ut
pu
t
2
N
ot
es
1-
lo
op
d
ev
ic
e,
2
r
el
ay
s
–
1*
/0
1
=
O
ut
pu
t
2
on
; 0
=
O
ut
pu
t
2
of
f
2-
lo
op
d
ev
ic
e,
2
r
el
ay
s
ac
ti
ve
–
Pa
ra
m
et
er
8
is
n
ot
p
os
si
bl
e
an
d
is
n
ot
d
is
pl
ay
ed
de
ac
ti
va
te
d
1/
0*
1
=
O
ut
pu
t
2
on
; 0
=
O
ut
pu
t
2
of
f
Lo
op
R
el
ay
Lo
op
R
el
ay
tt
Lo
op
R
el
ay
tt
Lo
op
R
el
ay
tt
Lo
op
R
el
ay
tt






















