24
Chapter 2: Documenting Polynomial Zero Finding
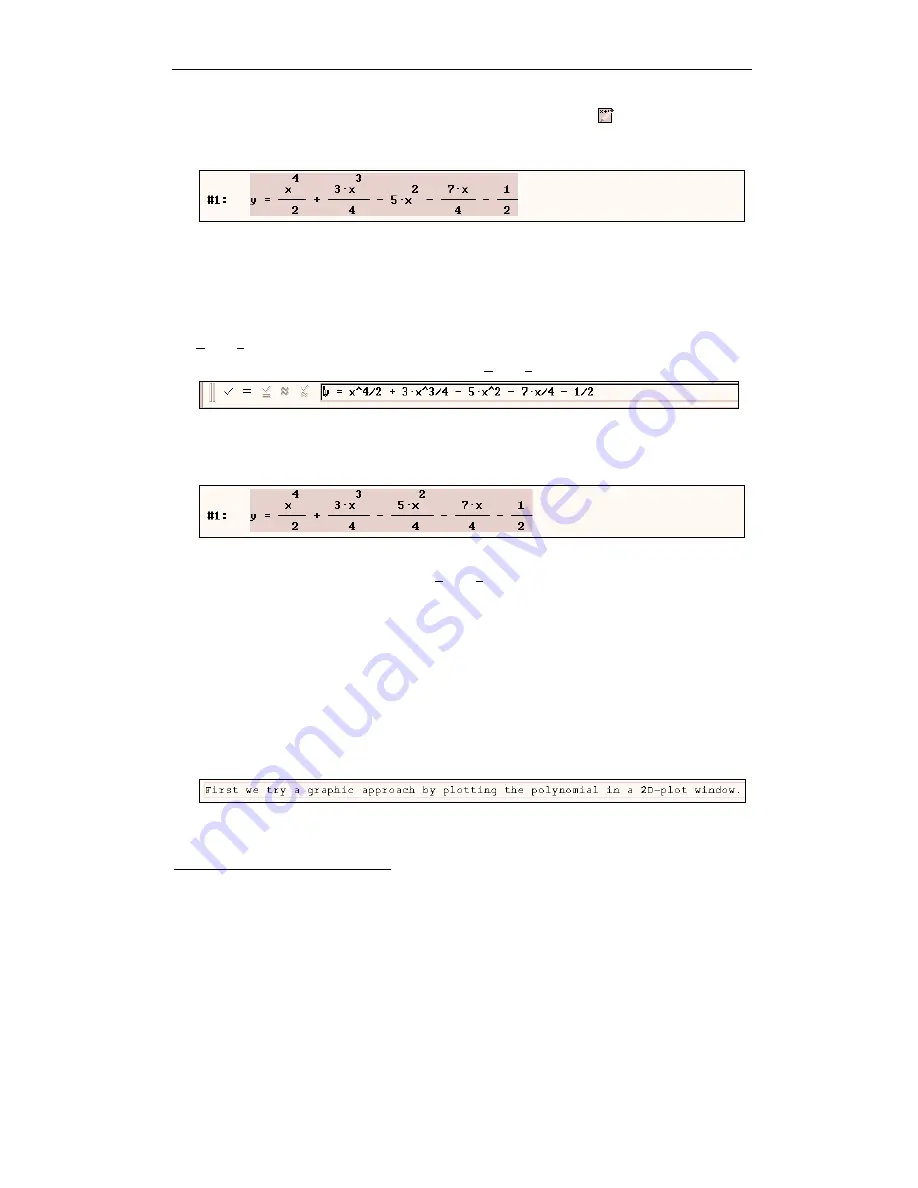
Enter the above polynomial by preparing for expression input with
, then typing:
y=x^4/2+3x^3/4-5x^2-7x/4-1/2
(Intentionally leave out the
/4
in the middle term.)
From here on
,
the key
(¢)
or the button
(_OK_)
will be displayed only in ambiguous situations.
It will not be used any more for simple inputs such as the above. It is important for some of the
features you are going to study and use in this chapter that you work with the above polynomial.
Therefore, make sure it was entered properly.
As you know, it was not! The
/4
in the middle term is missing. This is easily repaired by applying
the
Edit>Derive Object
command to the highlighted expression.
Edit the highlighted expression by selecting the
Edit>Derive Object
command.
This brings a copy of the expression into the entry line with the cursor positioned at its left end,
so the system is ready for editing.
Insert
/4
after
5x^2
, then end the input with
(¢)
.
The
(¢)
key performed a
replacement
of the old expression with the new one. There is no need
to delete the old expression when using the
Edit>Derive Object
command.
Consider looking at a house from several different positions. From each position you will see
details that you can’t see from other positions. Based on this idea, mathematicians use a variety
of different representations for mathematical objects. The fourth degree polynomial that you
entered is displayed as an
algebraic
representation. Next you will produce a
graphical
representation, because this representation is particularly useful for obtaining information about
the zeros. In other words, you will plot
1
its graph.
Since the major goal in this session is to properly document the mathematical work, . . .
. . . insert the text
“First we try a graphic approach by plotting the polynomial in a 2D-plot
window.”
1
“Plot” is a technical term. As such, it includes different aspects of drawing and graphical
representation. It does not stand for mathematical accuracy, and in this book it will be used with three
different meanings: for the activity of producing a graphical representation, for a graphical
representation as an object, and for the corresponding D
ERIVE
command.






























