Technosoft iPOS8015 BZ-CAT, Technical Reference

Page: 23 / 34

 Technosoft 2021 23 iPOS8015 BZ-CAT Technical Reference
Remarks:
1. An external electrolytic capacitor may be added bV
mot
and GND, to help reduce over-voltage during
load braking/ reversals. See paragraph
3.9.1.1
for details.
2. When the STO inputs are left unconnected, the motor outputs will be disabled. This provides a dual redundant
hardware protection that cannot be overdriven by the software or other hardware components.
3.9.1.1 Recommendations for Supply Wiring
The iPOS8015 BZ always requires two supply voltages: V
log
and V
mot
.
Use short, thick wires between the iPOS8015 BZ and the motor power supply. Connect power supply wires to all the
indicated pins. If the wires are longer than 2 meters, use twisted wires for the supply and ground return. For wires longer
than 20 meters, add a capacitor of at least 4. 700μF (rated at an appropriate voltage) right on the terminals of the
iPOS8015 BZ.
**It is recommended to connect the negative motor supply return (GND) to the Earth protection near the external motor
power supply terminals, i.e. between EARTH and -V
mot
external power supply.
3.9.2 Recommendations to limit over-voltage during braking
During abrupt motion brakes or reversals the regenerative energy is injected into the motor power supply. This may
cause an increase of the motor supply voltage (depending on the power supply characteristics). If the voltage bypasses
84V, the drive over-voltage protection is triggered and the drive power stage is disabled. In order to avoid this situation
you have 2 options:
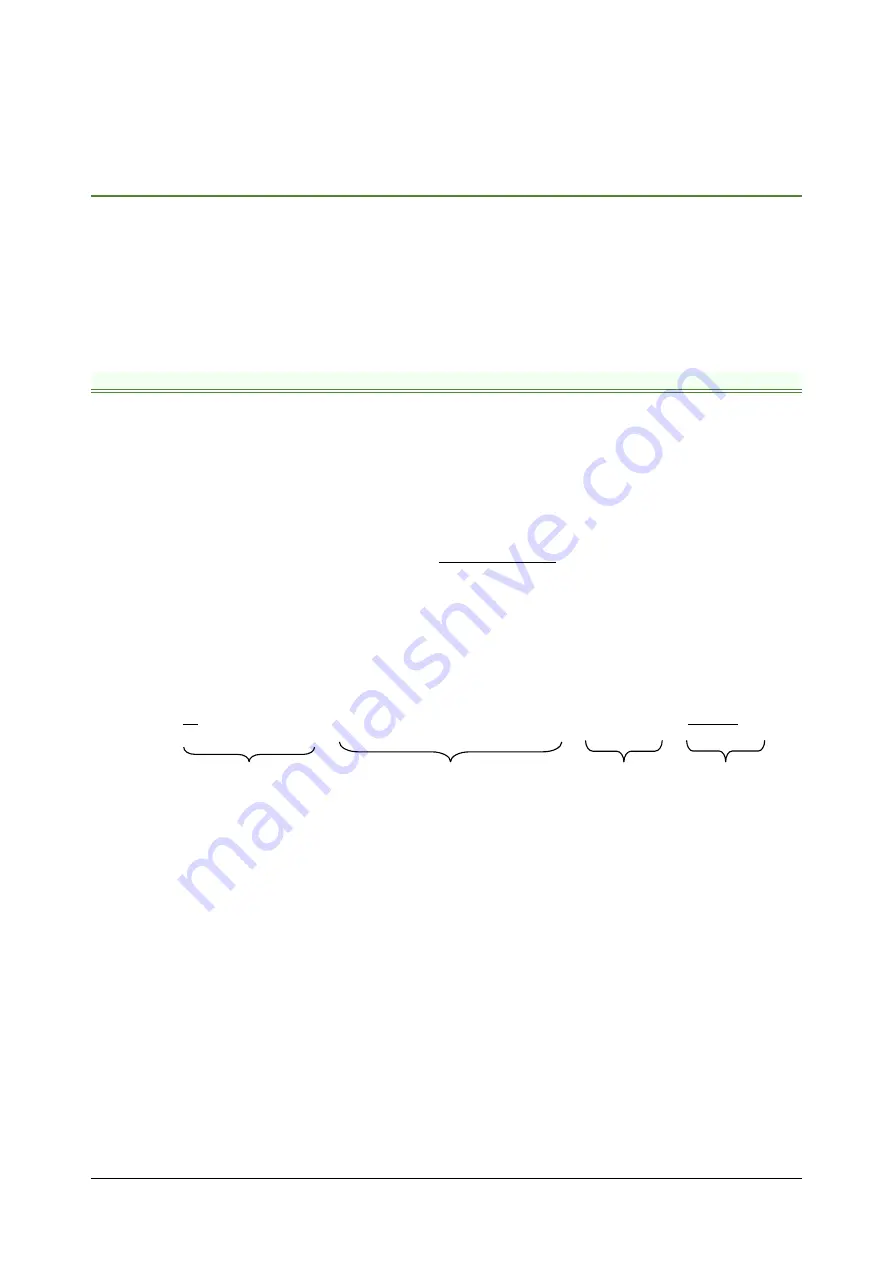
Option 1. Add a capacitor on the motor supply
big enough to absorb the overall energy flowing back to the supply.
The capacitor must be rated to a voltage equal or bigger than the maximum expected over-voltage and can be sized
with the formula:
2 2
2
NOM MAX
M
U U
E
C
−
×
≥
where:
U
MAX
= 84V is the over-voltage protection limit
U
NOM
is the nominal motor supply voltage
E
M
= the overall energy flowing back to the supply in Joules. In case of a rotary motor and load, E
M
can be
computed with the formula:
F
M d
d Ph
2
M final initial L M
2
M L M M
T
2
t
t R 3I ) h - )g(h m (m ) J (J
2
1
E
ϖ
ϖ
− − + + + =
where:
J
M
– total rotor inertia [kgm 2 ]
J
L
– total load inertia as seen at motor shaft after transmission [kgm 2 ]
ϖ
M
– motor angular speed before deceleration [rad/s]
m
M
– motor mass [kg] – when motor is moving in a non-horizontal plane
m
L
– load mass [kg] – when load is moving in a non-horizontal plane
g – gravitational acceleration i.e. 9.8 [m/s 2 ]
h
initial
– initial system altitude [m]
h
final
– final system altitude [m]
I
M
– motor current during deceleration [A
RMS
/phase]
R
Ph
– motor phase resistance [ Ω ]
t
d
– time to decelerate [s]
T
F
– total friction torque as seen at motor shaft [Nm] – includes load and transmission
In case of a linear motor and load, the motor inertia J
M
and the load inertia J
L
will be replaced by the motor mass and
the load mass measured in [kg], the angular speed
ϖ
M
will become linear speed measured in [m/s] and the friction
torque T
F
will become friction force measured in [N].
Kinetic energy Copper losses Friction losses Potential energy