c i r c u i t s by changing the signal generator frequency.
V o l t m e t e r is capable of directly reading a gain between
t w o points a s having t w o independent v o l t m e t e r s . A s an
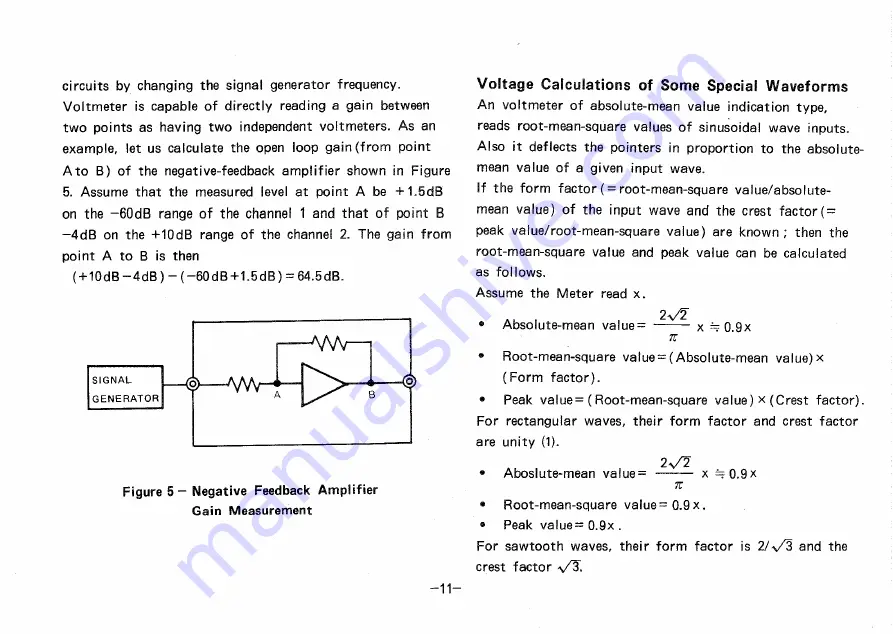
example, let us calculate the open loop gain ( f r o m point
A to B ) of the negative-feedback a m p l i f i e r shown in Figure
5. Assume t h a t the measured level a t point A be + 1.5dB
on the - 6 0 d B range of the channel 1 and t h a t of point B
- 4 d B on the + 1 0 d B range of the channel 2. The gain f r o m
point A to B is then
( + 1 0 d B - 4 d B ) - ( - 6 0 d B + 1 . 5 d B ) = 64.5dB.
S I G N A L
G E N E R A T O R
Figure 5
- .Negative Feedback Amplifier
Gain Measurement
Voltage Calculations of Some Special Waveforms
A n voltmeter of absolute-mean value indication type,
reads root-mean-square values of sinusoidal w a v e inputs.
A l s o it deflects the pointers in proportion to the absolute-
mean value of a given input w a v e .
If the f o r m f a c t o r ( = root-mean-square value/absolute-
mean v a l u e ) of the input wave and the crest f a c t o r ( =
peak vaIue/root-mean-square v a l u e ) are known ; then the
root-mean-square value and peak value can be calculated
as f o l l o w s .
Assume the Meter read x .
2 V ?
• Absolute-mean v a l u e = x = 0.9x
71
• Root-mean-square value = (Absolute-mean value) x
( F o r m f a c t o r ) .
• Peak v a l u e = (Root-mean-square v a l u e ) x ( C r e s t f a c t o r ) .
F o r rectangular w a v e s , their f o r m f a c t o r and crest f a c t o r
are u n i t y (1).
• Aboslute-mean v a l u e = — x = 0 . 9 x
71
• Root-mean-square v a l u e = 0 . 9 x .
• Peak v a l u e = 0.9x .
F o r s a w t o o t h w a v e s , their f o r m f a c t o r is 2 / > / 3 and the
crest f a c t o r
v
^3»
- 1 1 -
















