Beamage-M² User Manual
Revision 6
14
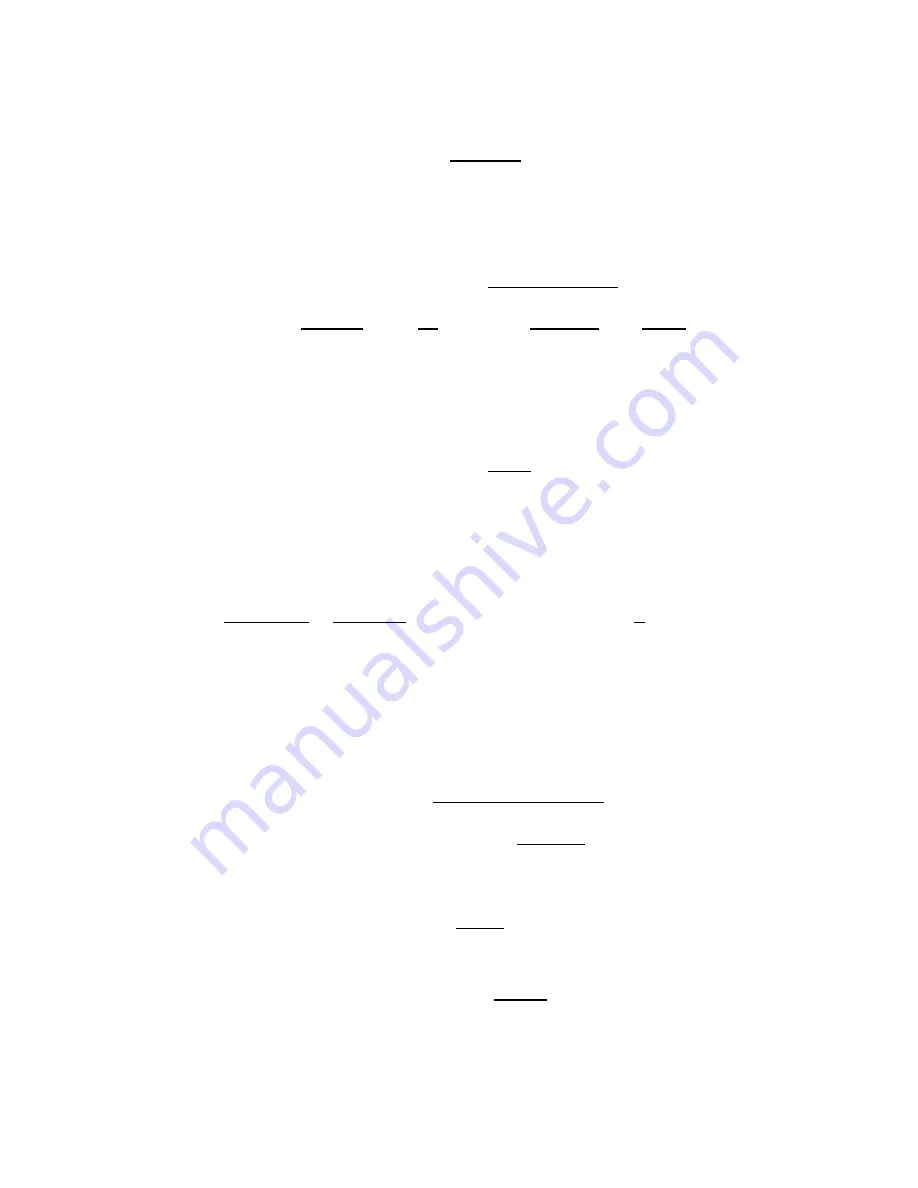
Mathematically, it is given by the following equation:
𝑧
𝑅𝑡ℎ
=
𝜋(
𝑤
0
𝑡ℎ
)
2
𝜆
Far from the beam waist, the beam expansion becomes linear and the theoretical divergence half-angle
𝜃
𝑡ℎ
) can be obtained by e
valuating the limit of the beam radius’
s
first derivative as the position tends towards infinity:
𝜃
𝑡ℎ
= 𝑙𝑖𝑚
𝑧→
∞
𝑑𝑤
𝑡ℎ
(𝑧)
𝑑𝑧
= 𝑙𝑖𝑚
𝑧→
∞
𝑑
𝑑𝑧
𝑤
0
𝑡ℎ
√1 + (
𝜆𝑧
𝜋(𝑤
0
𝑡ℎ
)
2
)
2
=
𝜆
𝜋𝑤
0
𝑡ℎ
For a laser beam that passes through a focusing lens of focal length
f
, the theoretical radius of the beam
𝑤
𝑓𝑡ℎ
at the focal spot of the lens can be obtained by multiplying the beam divergence half-angle with the
focal length
f
:
𝑤
𝑓
𝑡ℎ
= 𝑓
𝜃
𝑡ℎ
=
𝑓𝜆
𝜋
𝑤
0
𝑡ℎ
As mentioned, all of the equations above describe theoretical ideal Gaussian beams. However, they can
describe the propagation of real laser beams if we modify them slightly using the M
2
factor, which can be
mathematically defined by the following equations:
𝑀
2
=
𝜋𝜃
𝑒𝑥𝑝
𝑤
0
𝑒𝑥𝑝
𝜆
=
𝜃
𝑒𝑥𝑝
𝑤
0
𝑒𝑥𝑝
𝛩
𝑡ℎ
𝑤
0
𝑡ℎ
> 1 𝑏𝑒𝑐𝑎𝑢𝑠𝑒 𝜃
𝑒𝑥𝑝
𝑤
0
𝑒𝑥𝑝
>
𝜆
𝜋
= 𝛩
𝑡ℎ
𝑤
0
𝑡ℎ
With mathematics, it is easy to understand why small M
2
values correspond to low experimental
divergences and small experimental beam waist radiuses.
The experimental beam waist radius
𝑤
𝑒𝑥𝑝
(𝑧)
, the experimental half-angle divergence
𝜃
𝑒𝑥𝑝
, and the
experimental beam radius at the focal spot of the lens
𝑤
𝑓𝑒𝑥𝑝
are therefore given by the following
equations:
𝑤
𝑒𝑥𝑝
(𝑧) = 𝑤
0
𝑡ℎ
√𝑀
2
+ 𝑀
2
(
𝜆𝑧
𝜋(𝑤
0
𝑡ℎ
)
2
)
2
𝜃
𝑒𝑥𝑝
=
𝑀
2
𝜆
𝜋
𝑤
0
𝑒𝑥𝑝
𝑤
𝑓𝑒𝑥𝑝
= 𝑓𝜃
𝑒𝑥𝑝
=
𝑓𝑀
2
𝜆
𝜋𝑤
0
𝑒𝑥𝑝






























