Emissivity Correction
Appropriate correction for surface emissivity is required for accurate surface temperature measurements. The
simple (and commonly made) emissivity correction, dividing measured temperature by surface emissivity, is
incorrect because it does not account for reflected infrared radiation.
The radiation detected by an infrared radiometer includes two components: 1. radiation directly emitted by the
target surface, and 2. reflected radiation from the background. The second component is often neglected. The
magnitude of the two components in the total radiation detected by the radiometer is estimated using the
emissivity (
ε
) and reflectivity (1 -
ε
) of the target surface:
(
)
Background
et
arg
T
Sensor
E
1
E
E
−
+
=
(1)
where E
Sensor
is radiance [W m
-2
sr
-1
] detected by the radiometer, E
Target
is radiance [W m
-2
sr
-1
] emitted by the
target surface, E
Background
is radiance [W m
-2
sr
-1
] emitted by the background (when the target surface is outdoors
the background is generally the sky), and
ε
is the ratio of non-blackbody radiation emission (actual radiation
emission) to blackbody radiation emission at the same temperature (theoretical maximum for radiation emission).
Unless the target surface is a blackbody (
ε
= 1; emits and absorbs the theoretical maximum amount of energy
based on temperature), E
sensor
will include a fraction (1
–
ε
) of reflected radiation from the background.
Since temperature, rather than energy, is the desired quantity, Eq. (1) can be written in terms of temperature
using the Stefan-Boltzmann Law, E =
σ
T
4
(relates energy being emitted by an object to the fourth power of its
absolute temperature):
( )
4
Background
4
et
arg
T
4
Sensor
T
1
T
T
−
+
=
(2)
where T
Sensor
[K] is temperature measured by the infrared radiometer (brightness temperature), T
Target
[K] is actual
temperature of the target surface, T
Background
[K] is brightness temperature of the background (usually the sky), and
σ
is the Stefan-Boltzmann constant (5.67 x 10
-8
W m
-2
K
-4
). The power of 4 on the temperatures in Eq. (2) is valid for
the entire blackbody spectrum.
Rearrangement of Eq. (2) to solve for T
Target
yields the equation used to calculate the actual target surface
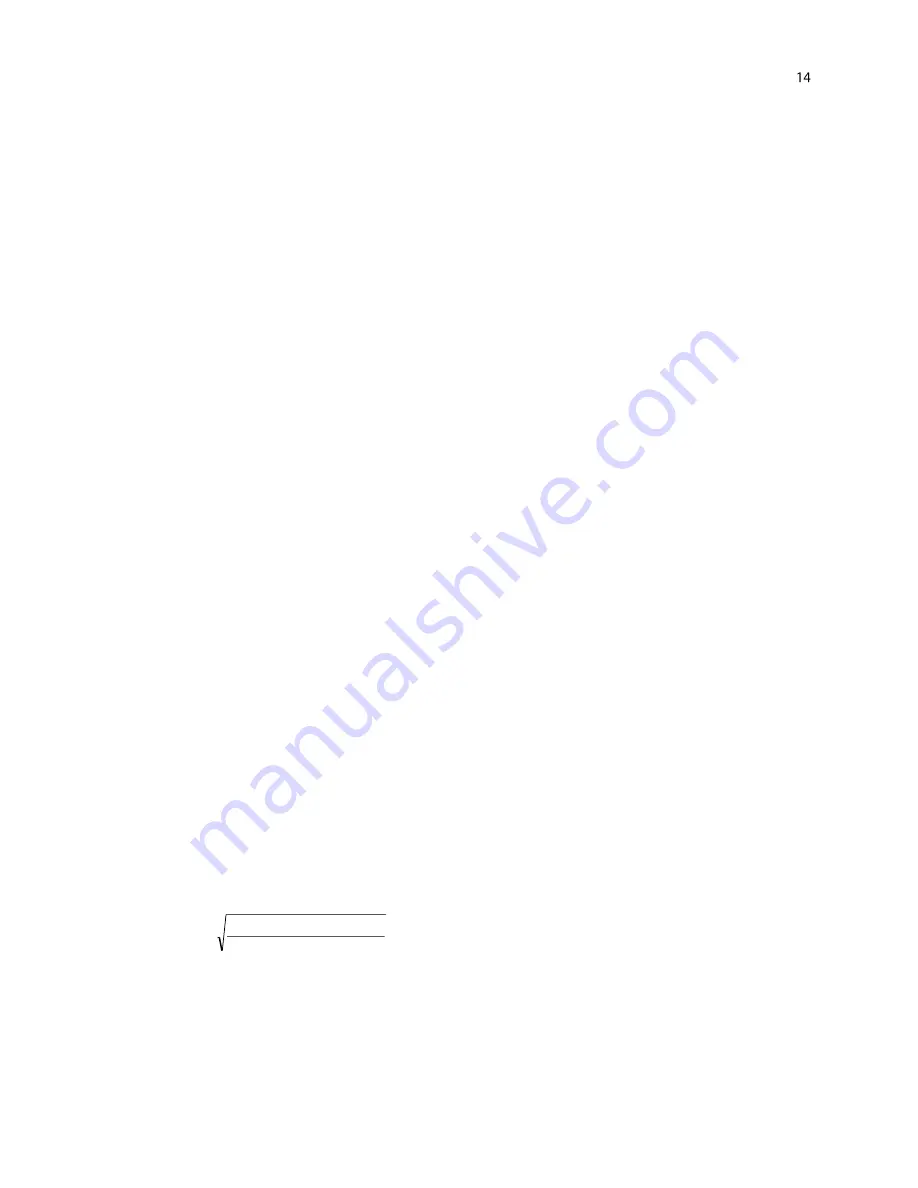
temperature (i.e., measured brightness temperature corrected for emissivity effects):
(
)
4
4
Ba ckground
4
Se nsor
et
arg
T
T
1
T
T
−
−
=
.
(3)




















