12
Photogate Timer
012-06379B
®
m
m
a
t
1
t
2
t
3
v
1
v
2
a
F
a
Glider Length,
L
=
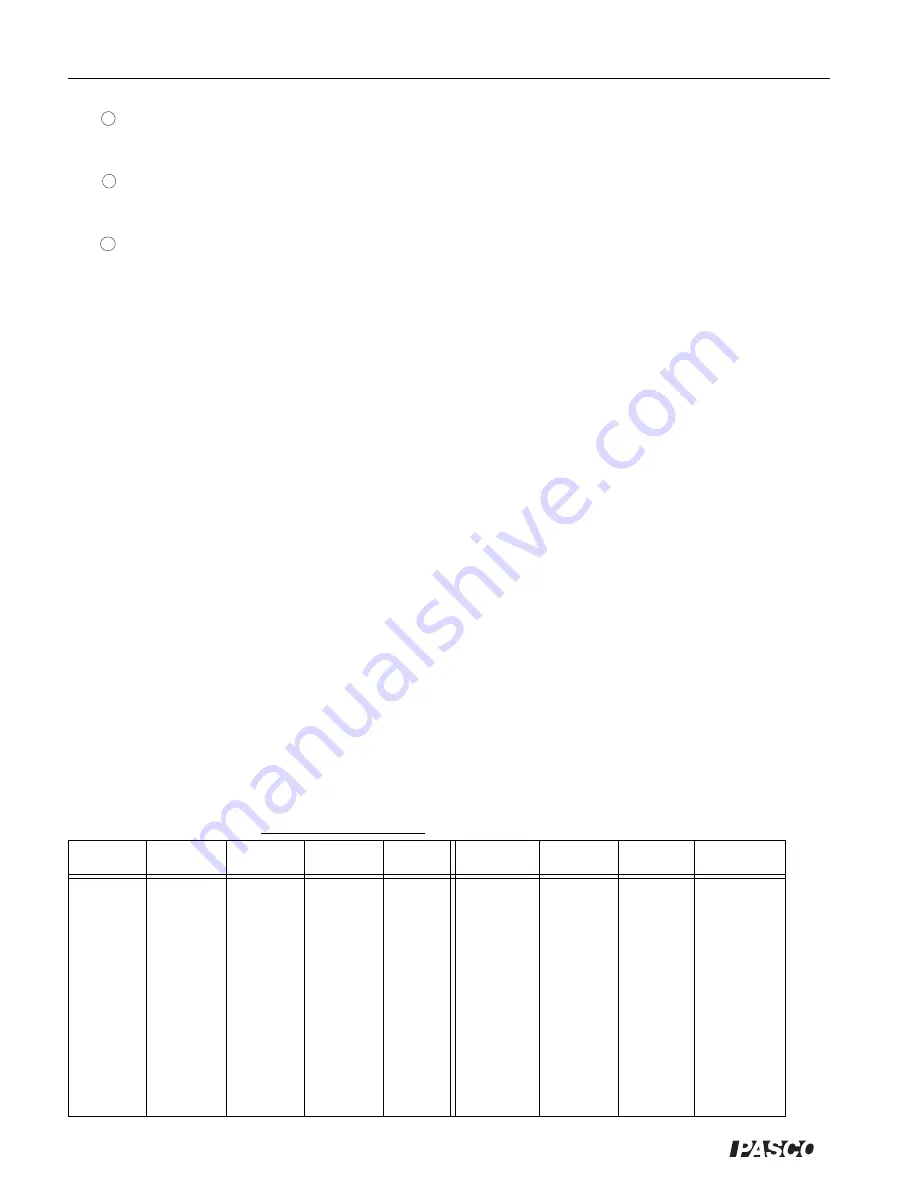
Table 4.1 Data and Calculations
12
Again, start the glider from
x
0
. This time measure and record
t
3
, the time it takes the glider to
pass between the photogates. Repeat this measurement four more times and record the aver-
age of these measurements as
t
3
in Table 4.1.
13
Vary
m
a
, by moving masses from the glider to the hanger (thus keeping the total mass,
m
+
m
a
, constant.) Record
m
and
m
a
and repeat steps 5 through 11. Try at least four different
values for
m
a
.
14
Now leave
m
a
constant at a previously used value. Vary
m
by adding or removing mass from
the glider. Repeat steps 5-11. Try at least four different values for
m
.
Calculations
For each set of experimental conditions:
c
Use the length of the glider and your average times to determine
v
1
and
v
2
, the average glider
velocity as it passed through each photogate.
d
Use the equation
a = (v
2
- v
1
)/t
3
to determine the average acceleration of the glider as it passed
between the two photogates.
e
Determine
F
a
, the force applied to the glider by the hanging mass.
(
F
a
=
m
a
g; g
= 9.8 m/s
2
= 980 cm/s
2
)
Analysis
c
Draw a graph showing average acceleration as a function of applied force,
F
a
,
.
d
Draw a second graph showing average acceleration as a function of the glider mass with M
a
being held constant.
e
Examine your graphs carefully. Are they straight lines? Use your graphs to determine the
relationship between applied force, mass, and average acceleration for the air track glider.
f
Discuss your results. In this experiment, you measured only the average acceleration of the
glider between the two photogates. Do you have reason to believe that your results also hold
true for the instantaneous acceleration? Explain. What further experiments might help extend
your results to include instantaneous acceleration?






























