FP-RTD-124 and cFP-RTD-124
8
ni.com
Unfortunately, not all TCR values are as well defined by standards
organizations, and the behavior of RTDs with the same TCR value
may vary from vendor to vendor. The variations are usually small,
and the built-in linearization algorithms of the [c]FP-RTD-124 are
appropriate for nearly all applications.
The [c]FP-RTD-124 uses a linearization curve known as the
Callendar-Van Dusen equation to measure the temperature of
RTDs. The equation is as follows:
Temperatures below 0
°
C:
R
T
=
R
0
[1
+
A
×
T
+
B
×
T
2
+
C
×
T
3
×
(
T
– 100
°
C)]
Temperatures above 0
°
C:
R
T
=
R
0
[1
+
A
×
T
+
B
×
T
2
]
T
= temperature in
°
C
R
T
= RTD resistance at temperature
T
R
0
= RTD nominal resistance at 0
°
C
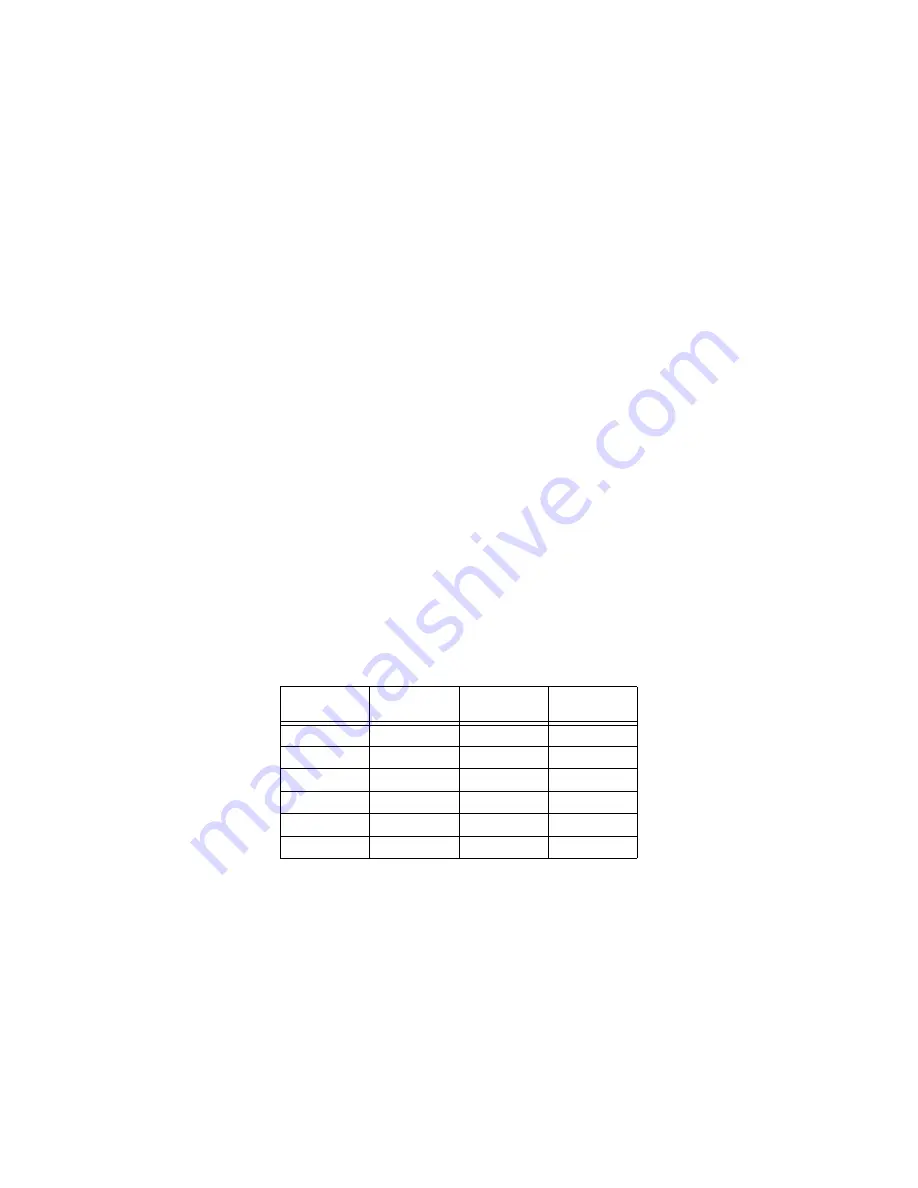
A, B, and C are coefficients given in Table 2.
Table 2 lists the coefficients used in this equation for each of the
TCR values that the [c]FP-RTD-124 supports. If you have a
nonstandard RTD that does not match one of these linearization
curves, measure the resistance with the [c]FP-RTD-124 and
convert the resistance to temperature in the manner suggested by
the RTD vendor.
Table 2.
Callendar-Van Dusen Coefficients Used by the [c]FP-RTD-124
TCR
m
Ω
/
Ω
/
°
C
A
(
°
C)
–1
B
(
°
C)
–2
C
(
°
C)
– 4
3.750
3.81
×
10
–3
–6.02
×
10
–7
–6.0
×
10
–12
3.851
3.9083
×
10
–3
–5.775
×
10
–7
–4.183
×
10
–12
3.911
3.9692
×
10
–3
–5.8495
×
10
–7
–4.233
×
10
–12
3.916
3.9739
×
10
–3
–5.870
×
10
–7
–4.4
×
10
–12
3.920
3.9787
×
10
–3
–5.8686
×
10
–7
–4.167
×
10
–12
3.928
3.9888
×
10
–3
–5.915
×
10
–7
–3.85
×
10
–12
































