GOLDBERG AND MÄKIVIRTA
AUTOMATED IN-SITU EQUALISATION
AES 114TH CONVENTION, AMSTERDAM, THE NETHERLANDS, 2003 MARCH 22-25
3
frequency
f
c
(typically 70…200 Hz in small spaces) is
often dominated by room modes and comb filtering
caused by low-order discrete reflections from room
boundaries. Sound reproduction can be problematic
because of this. For a room with a reverberation time
T
60
of 0.3 s the room mode bandwidth is approxi-
mately 2.2/T
60
= 7.3 Hz [23]. However, this does not
predict accurately what the decay rate of an individual
mode is as reverberation time represents the total
decay rate in diffuse field whereas modal decay rate
may vary.
Above
f
c
modal density becomes sufficiently high to
be described statistically. An unsmoothed room
transfer function shows a large number of high Q
notches. When frequency smoothing due to human
hearing is taken into account [31], the resulting
sensation is a rather smooth room transfer function
(Figure 3 and Figure 6).
In the time domain, early reflections before about 25
ms combine with the direct sound to produce tone
colouration (comb filtering effect). Reflections
arriving later than about 25 ms are less problematic as
they typically combine to produce the reverberation of
the room and are perceived as separate sound events
(echoes and reverberation) rather than tone colour-
ation. This part of the time domain response contrib-
utes to the sensations of envelopment and spacious-
ness.
2.3. Room Response Controls
The loudspeakers to be optimised have room response
controls [1,32]. The smaller loudspeakers have
simpler controls than the larger systems but the
philosophy of filtering is consistent across the range
(Tables 1-4).
The
treble tilt
control
is used to reduce the high
frequency energy. In the small two-way systems and
two way systems it is a level control of the treble
driver and has an effect down to about 4 kHz. In large
systems it has a noticeable effect only above 10 kHz
and has a roll-off character.
The
driver level
controls
can be used to shape the
broadband response of a loudspeaker. They control
the output level of each driver with frequency ranges
that are determined by the crossover filters.
The
bass tilt
control
compensates for a bass boost
seen when the loudspeaker is loaded by large nearby
boundaries [33-36]. This typically happens when a
loudspeaker is placed next to, or mounted into, an
acoustically hard wall. This filter is a first
order
shelving filter.
The
bass roll-off
control
compensates for a bass
boost often seen at the very lowest frequencies the
loudspeaker can reproduce. This typically happens
when the loudspeaker is mounted in the corner of a
room where the loudspeaker is able to couple very
efficiently to the room thereby exacerbating room
mode effects that dominate this region of the fre-
quency response. It is a notch filter with a centre
frequency set close to the low frequency cut-off of the
loudspeaker.
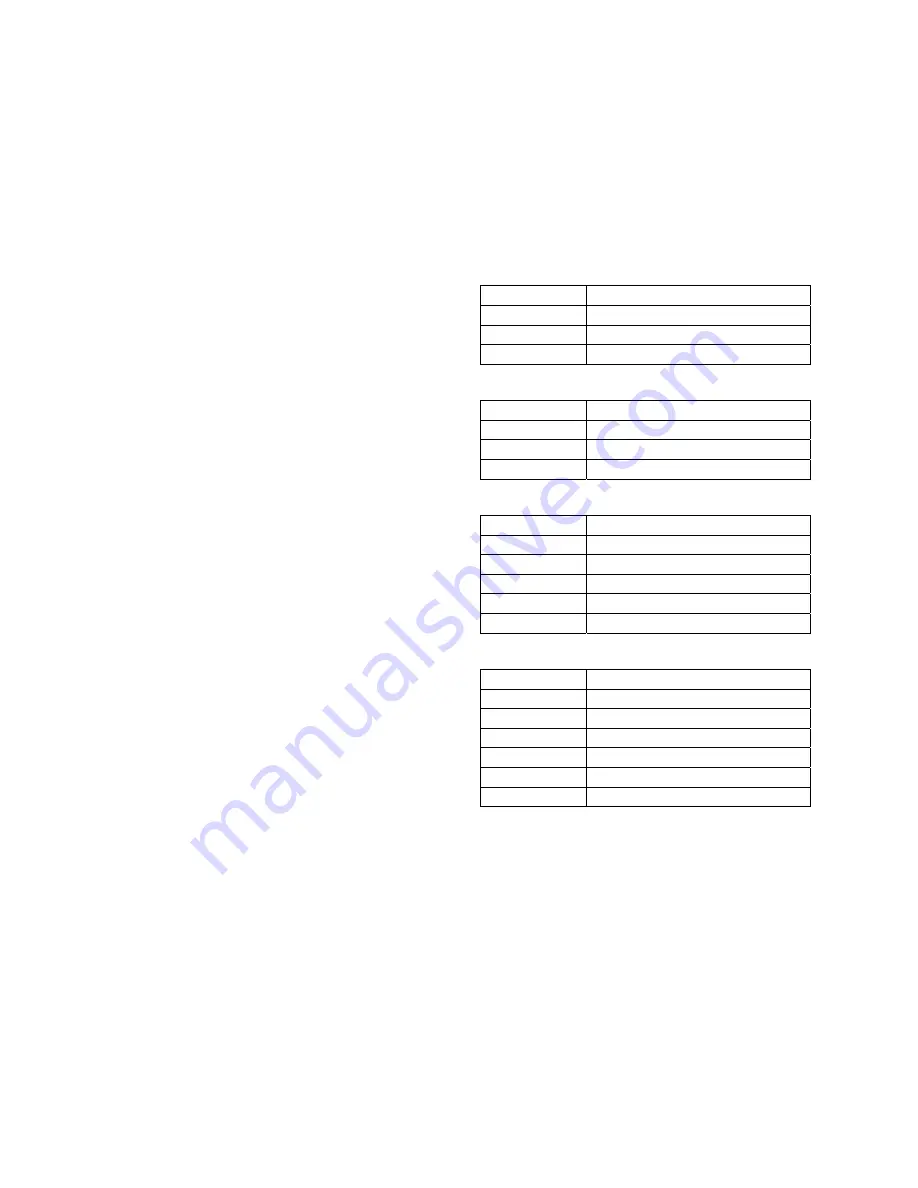
Table 1. Small two way room response controls.
Control type
Room response control settings, dB
Treble tilt
0, –2
Bass tilt
0, –2, –4, –6
Bass roll-off
0, –2
Table 2. Two way room response controls.
Control type
Room response control settings, dB
Treble tilt
+2, 0, –2, –4, driver mute
Bass tilt
0, –2, –4, –6, driver mute
Bass roll-off
0, –2, –4, –6, –8
Table 3. Three way room response controls.
Control type
Room response control settings, dB
Treble level
0, –1, –2, –3, –4, –5, –6, driver mute
Midrange level
0, –1, –2, –3, –4, –5, –6, driver mute
Bass level
0, –1, –2, –3, –4, –5, –6, driver mute
Bass tilt
0, –2, –4, –6, –8
Bass roll-off
0, –2, –4, –6, –8
Table 4. Large system room response controls.
Control type
Room response control settings, dB
Treble tilt
+1, 0, –1, –2, –3
Treble level
0, –1, –2, –3, –4, –5, –6, driver mute
Midrange level
0, –1, –2, –3, –4, –5, –6, driver mute
Bass level
0, –1, –2, –3, –4, –5, –6, driver mute
Bass tilt
0, –2, –4, –6, –8
Bass roll-off
0, –2, –4, –6, –8
3. ROOM EQUALISATION OPTIMISER
Optimisation involves the minimisation or maximisa-
tion of a scalar-valued objective function
E
(
x
),
( )
x
E
min
(1)
where,
x
is the vector of design parameters,
x
∈ℜ
n
.
Multi-objective optimisation is concerned with the
minimisation of a vector of objectives
E
(
x
) that may
be subject to constraints or bounds. Several robust
methods exist for optimising functions with design
parameters
x
having a continuous value range [37].


















