DAZZLER
TM
system manual Part I : installation & operation
2.1
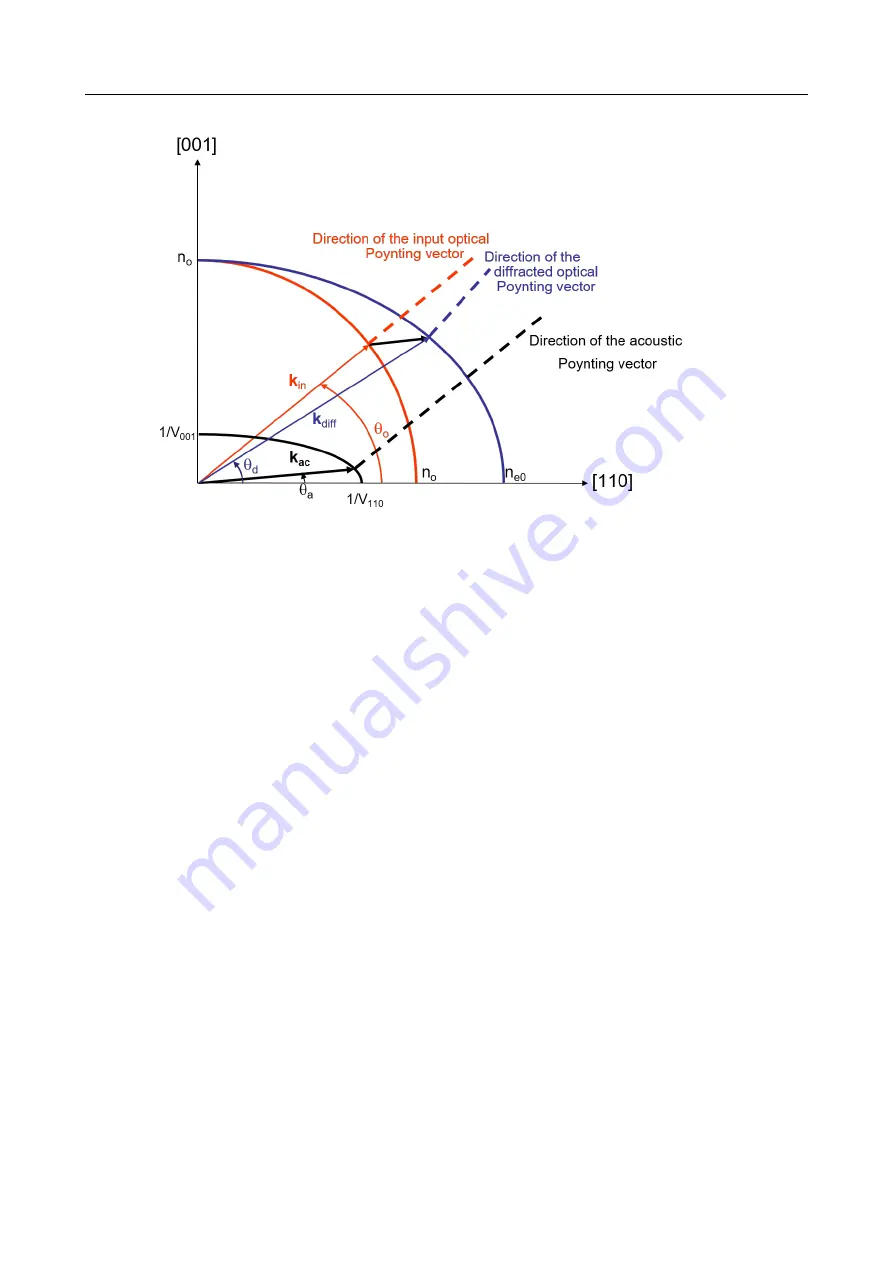
Figure 2.1: Phase-matching conditions and Poynting vector directions
~k
diff,e
(
ω
opt
) =
~k
in,o
(
ω
opt
) +
~k
ac
(
ω
ac
)
(2.2)
shows the ordinary and extraordinary (optical) index curves for the birefringent
cystal as well as the acoustic slowness (inverse velocity) curve in the plane defined by the [001]
and [110] axes. The incident optical and acoustic wave vectors make respectively an angle
θ
o
and
θ
a
with respect to the [110] axes. The acousto-optic interaction is not collinear in terms
of wave vectors. The diffracted wave propagates along the extraordinary axis with an angle
θ
d
(with respect to the [110] axis) given by the phase-matching conditions (
For given propagation directions of the optical and acoustic beams (
θ
o
and
θ
a
), the phase-
matching relationship can be viewed as an implicit equation which links acoustic and optical
frequencies. In thick crystals, this link is an almost one-to-one relationship (i.e. bijective)
between acoustic and optical frequencies. In other words, a single acoustic frequency diffracts
a single optical frequency.
2.1.2
Poynting vectors
In most acousto-optic modulators, a transverse acoustic wave interacts with the input optical
wave. The input optical Poynting vector is perpendicular to the acoustic one under the phase-
matching conditions. In the case of the AOPDF, the input optical and acoustic Poynting
vectors are collinear (
). The interaction is longitudinal (energy consideration) which
enables to maximize the interaction length between the two waves to obtain high spectral
resolution (section
) and diffraction efficiency (section
). This also entails
the quasi-bijective relationship between acoustic and optical frequencies.
ω
ac
/ω
opt
=
ν
ac
/ν
opt
=
α
(
ω
)
(2.3)
V3.00 - 8
th
April 2019




























