Appendix A. Calibrating KH20
A-2
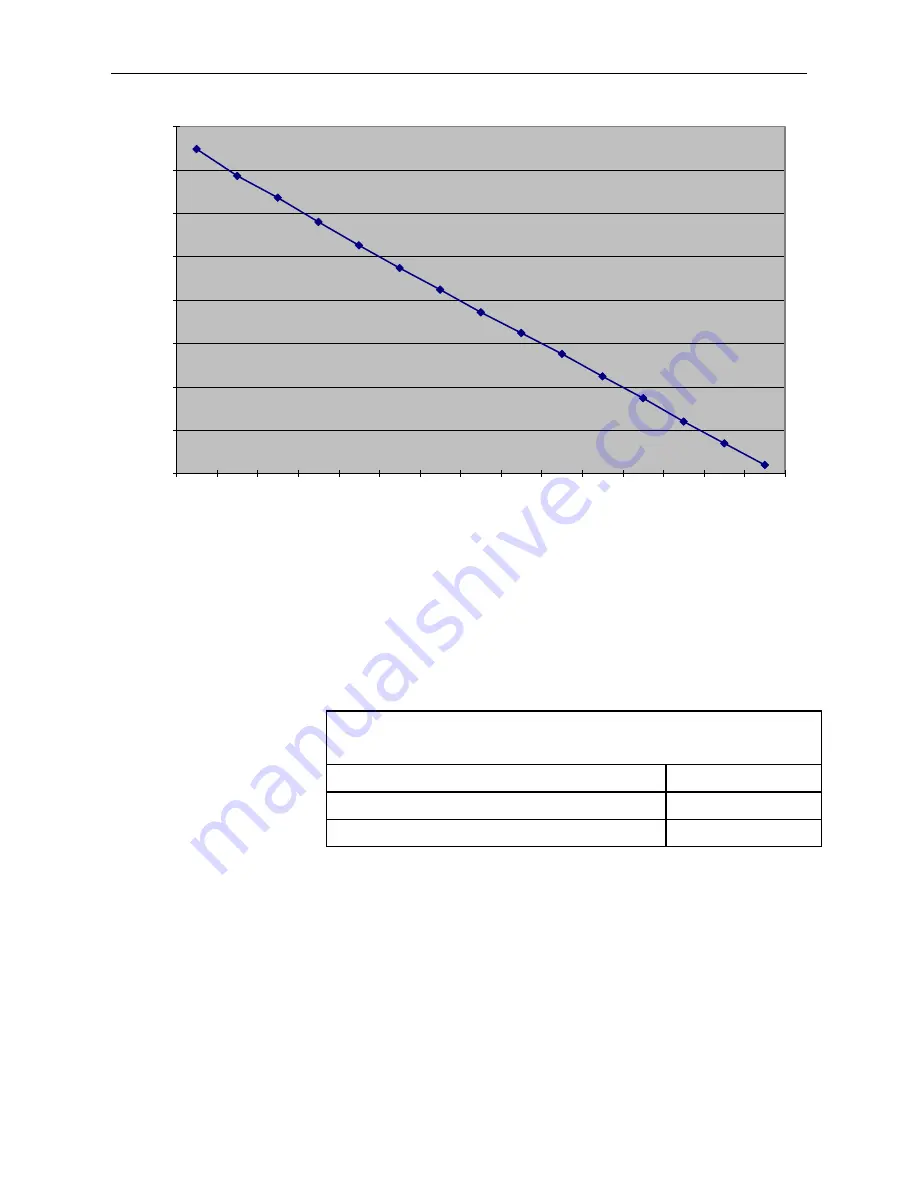
FIGURE A-1. KH20 ln(mV) vs. Vapor Density
We can perform the linear regression on the plot to obtain the slope for the
relationship between the ln(mV) and the vapor density. The slope for the graph
is the coefficient,
k
w
x
shows the result of linear regression
analysis. The slope is the product of the absorption coefficient of water vapor,
k
w
, and the KH20 path length,
x
.
TABLE A-1. Linear Regression Results for KH20 ln(mV)
vs. Vapor Density
Description
Values
Slope (xk
w
)
–0.205
Y Intercept (ln(V
0
)
8.033
If we substitute these values, along with the measured lnV into equation A-3,
we can obtain the water vapor density,
ρ
w
. Campbell Scientific performs the
calibration twice for each KH20: once with the window cleaned, and again
with the window scaled. We then break up the vapor density range into dry and
wet ranges, and compute the
k
w
values for each sub range, as well as for the full
range. If you know the vapor density range for your site, it is recommended
that you select the coefficient,
k
w
, that is appropriate for your site, the dry range
or the wet range. If the vapor range for the site is unknown, or if the vapor
range is on the border line between the dry and the wet ranges, use the value
for the full range. TABLE
shows the final calibration values the KH20
calibration certificate contains. The data shown in TABLE
actual KH20.
4
4.5
5
5.5
6
6.5
7
7.5
8
1.74
3.02
4.17
5.44
6.71
7.95
9.2
10.47 11.69 12.9 14.22 15.46 16.78 18.04 19.25
K
H
2
O O
u
tp
u
t (
m
V
)
Vapor Density (g/m
3
)






























