D
V
ESR
+
I
OUT
R
ESR
C
min
+
I
OUT
ǒ
V
OUT
*
V
BAT
Ǔ
ƒ
D
V
V
OUT
L
+
V
BAT
ǒ
V
OUT
–V
BAT
Ǔ
D
I
L
ƒ
V
OUT
TPS61090, TPS61091, TPS61092
www.ti.com
SLVS484C – JUNE 2003 – REVISED DECEMBER 2014
The second parameter for choosing the inductor is the desired current ripple in the inductor. Normally, it is
advisable to work with a ripple of less than 20% of the average inductor current. A smaller ripple reduces the
magnetic hysteresis losses in the inductor, as well as output voltage ripple and EMI. But in the same way,
regulation time at load changes rises. In addition, a larger inductor increases the total system costs. With those
parameters, it is possible to calculate the value for the inductor by using
Equation 5
:
(5)
Parameter f is the switching frequency and
Δ
I
L
is the ripple current in the inductor, i.e., 20% × I
L
. In this example,
the desired inductor has the value of 5.5 µH. With this calculated value and the calculated currents, it is possible
to choose a suitable inductor. Care has to be taken that load transients and losses in the circuit can lead to
higher currents as estimated in equation 4. Also, the losses in the inductor caused by magnetic hysteresis losses
and copper losses are a major parameter for total circuit efficiency.
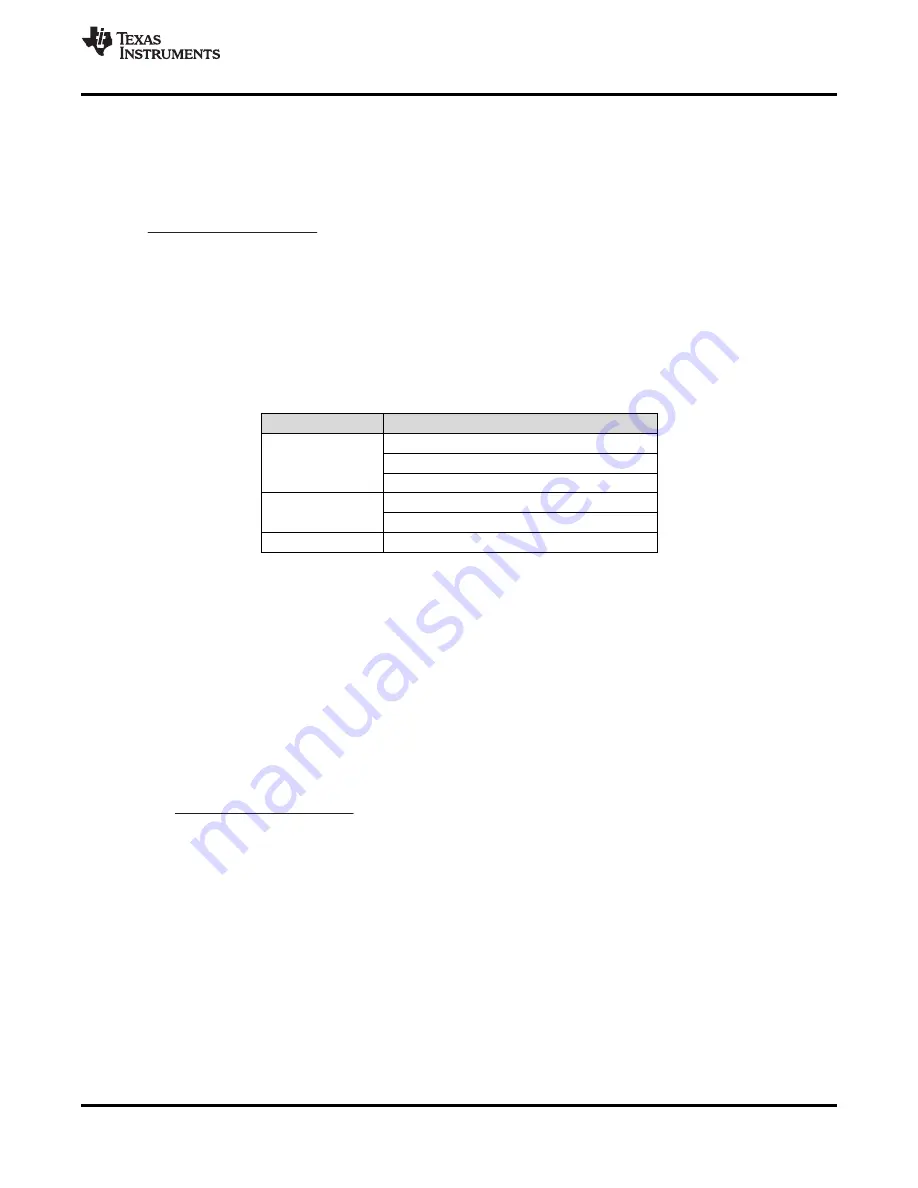
The following inductor series from different suppliers have been used with the TPS6109x converters:
Table 4. List of Inductors
VENDOR
INDUCTOR SERIES
CDRH6D28
Sumida
CDRH6D38
CDRH103R
Wurth Elektronik
WE-PD type L
WE-PD type XL
EPCOS
B82464G
10.2.1.2.4
Capacitor Selection
10.2.1.2.4.1
Input Capacitor
At least a 10-µF input capacitor is recommended to improve transient behavior of the regulator and EMI behavior
of the total power supply circuit. A ceramic capacitor or a tantalum capacitor with a 100-nF ceramic capacitor in
parallel, placed close to the IC, is recommended.
10.2.1.2.4.2
Output Capacitor DC-DC Converter
The major parameter necessary to define the minimum value of the output capacitor is the maximum allowed
output voltage ripple in steady state operation of the converter. This ripple is determined by two parameters of
the capacitor, the capacitance and the ESR. It is possible to calculate the minimum capacitance needed for the
defined ripple, supposing that the ESR is zero, by using equation
Equation 6
:
(6)
Parameter
f
is the switching frequency and
Δ
V is the maximum allowed ripple.
With a chosen ripple voltage of 10 mV, a minimum capacitance of 53 µF is needed. The total ripple is larger due
to the ESR of the output capacitor. This additional component of the ripple can be calculated using
Equation 7
:
(7)
An additional ripple of 40 mV is the result of using a tantalum capacitor with a low ESR of 80 m
Ω
. The total ripple
is the sum of the ripple caused by the capacitance and the ripple caused by the ESR of the capacitor. In this
example, the total ripple is 50 mV. Additional ripple is caused by load transients. This means that the output
capacitance needs to be larger than calculated above to meet the total ripple requirements. The output capacitor
has to completely supply the load during the charging phase of the inductor. A reasonable value of the output
capacitance depends on the speed of the load transients and the load current during the load change. With the
calculated minimum value of 53 µF and load transient considerations, a reasonable output capacitance value is
in a 100 µF range. For economical reasons this usually is a tantalum capacitor. Because of this the control loop
has been optimized for using output capacitors with an ESR of above 30 m
Ω
.
Copyright © 2003–2014, Texas Instruments Incorporated
Submit Documentation Feedback
15
Product Folder Links:
TPS61090 TPS61091 TPS61092













































