Schweitzer Engineering Laboratories SEL-351-5, Instruction Manual

Page: 139 / 706

3.59
Date Code 20070117
Instruction Manual SEL-351-5, -6, -7 Relay
Overcurrent, Voltage, Synchronism Check, Frequency, and Power Elements
Power Elements (Available in Firmware Version 7)
Timer:
±0.25 cycles and ±0.1% of setting
Single-Phase Power
Element Calculations
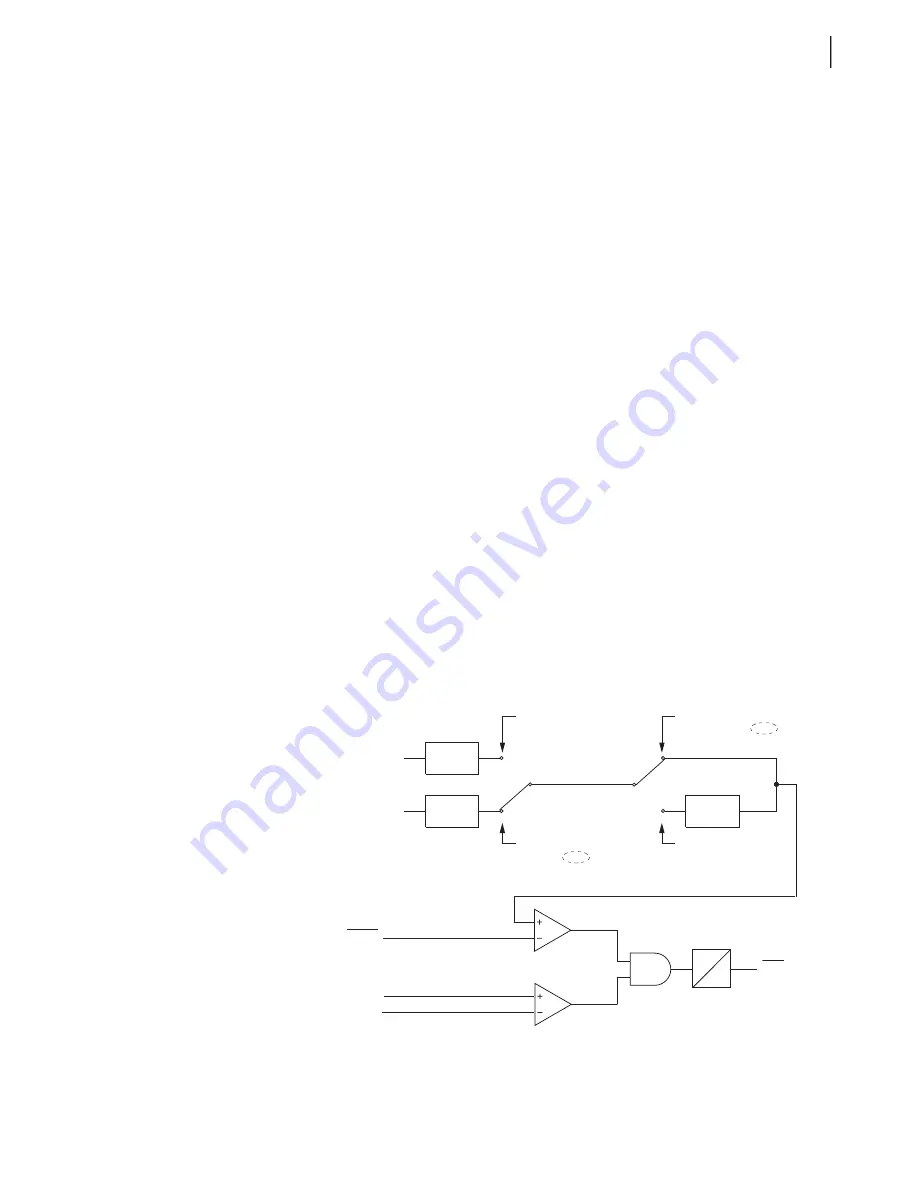
The numeric method used in the single-phase power elements uses line-to-
neutral voltage and phase current quantities. Each phase is calculated
separately, with the resulting power quantities subject to the minimum voltage
and current tests shown in the lower half of
Figure 3.36 .
Three-Phase Power
Element Calculations
The numeric method used in the three-phase power elements uses line-to-line
voltage and phase current quantities, corrected with zero-sequence voltage
and current when unbalanced. The following discussion assumes that all three
phase currents (I
A
, I
B
, and I
C
) are connected to the relay.
The resulting power quantities are subject to the minimum voltage and current
tests shown in the lower half of
Figure 3.37 .
For wye-connected relays (Global setting PTCONN = WYE), the three-phase
power is the same as the sum of the single-phase powers under any conditions
of unbalance, because the zero-sequence voltage (and current) is available.
For delta-connected relays (Global setting PTCONN = DELTA) with a broken
delta 3V0 connection (Global setting VSCONN = 3V0), the three-phase
power is the same as the sum of the theoretical single-phase powers under any
conditions of unbalance, because the zero-sequence voltage is available via
the
VS-NS terminals (provided the 3V0 source is on the same bus section as the
three-phase voltage inputs, the two signal sources cannot be isolated by
switching action, and the PTR and PTRS settings are properly entered).
For delta-connected relays (Global setting PTCONN = DELTA) with no
broken delta 3V0 connection (Global setting VSCONN = VS), the three-phase
power is the same as the sum of the theoretical single-phase powers only in
balanced conditions (either |3V0| = 0, or |3I0| = 0, or both). For unbalanced
conditions, the three-phase power element value will include an error term
that is proportional to the amount of unbalance.
Power Elements Logic
Operation
Figure 3.36 Single-Phase Power Elements Logic (+VARS Example Shown)
2-cycle
average
Phase A
Reactive Power
2-cycle
average
Phase A
Real Power
Switch in this position
if PWRnT = +WATTS, —WATTS
Multiply by —1
• (—I)
Switch in this position
if PWRnT = —WATTS, —VARS
Single-Phase VA sec
Sufficient
Signal
n = 1, 2, 3, or 4
I
nom
= 1 A sec or 5 A sec
Repeat for Phases B and C
Switch in this position
if PWRnT = +VARS , —VARS
Switch in this position
if PWRnT = +WATTS, +VARS
PWRnP
Setting
|V
A
|
40 V
PWRAn
Relay
Word
Bits
PWRnD
0