hp calculators
HP 20b Statistics – Rearranging Items
hp calculators
- 3 -
HP 20b Statistics – Rearranging Items - Version 1.0
In RPN mode, press:
52I5:c
Answer:
2,598,960 different hands.
Example 3: John has had a difficult week at work and is standing in front of the doughnut display at the local grocery
store. He is trying to determine the number of ways he can fill his bag with his 5 doughnuts from the 20
varieties in the display case. He considers the order in which the doughnuts are placed into the bag to be
unimportant. How many different ways can he put them in his bag?
Solution:
Since the order in which the doughnuts are placed in the bag does not matter, the problem is solved
as a combination.
In algebraic or chain mode, press:
20:c5=
In RPN mode, press:
20I5:c
Answer:
15,504 different ways.
Example 4: John has had a difficult week at work and is standing in front of the doughnut display at the local
grocery store. He is trying to determine the number of ways he can fill his bag with his 5 doughnuts
from the 20 varieties in the display case. He considers the order in which the doughnuts are placed into
the bag to be quite important. How many different ways can he put them in his bag?
Solution:
Since the order in which the doughnuts are placed in the bag matters, the problem is solved as a
permutation.
In algebraic or chain mode, press:
20:b5=
In RPN mode, press:
20I5:b
Answer:
1,860,480 different ways. John may be in front of the display case for some time.
Example 5: If you flip a coin 10 times, what is the probability that it comes up tails exactly 4 times?
Solution:
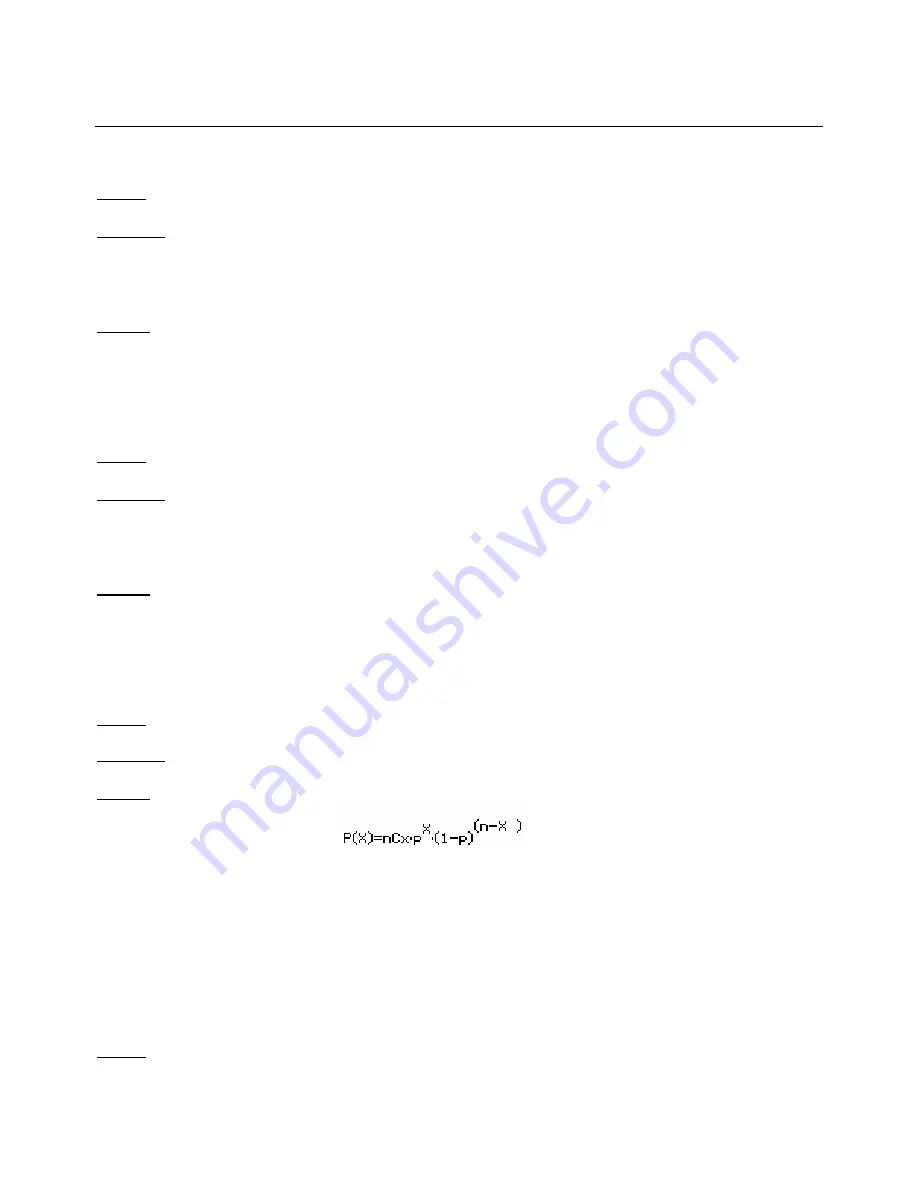
This is an example of the binomial probability distribution. The formula to find the answer is given by:
Figure 3
where P(X) is the probability of having X successes observed, nCx is the combination of n items taken
x at a time, and p is the probability of a success on each trial.
In algebraic or chain mode, press:
10:c4=*0.5:h4*
(1-0.5):h(10-4)=
In RPN mode, press:
10 I4:c0.5I4:h*
1I0.5-10I4-:h*
Answer:
0.21. If you flip a coin 10 times, there is a 21% (actually 20.51%) chance of seeing heads 4 times.




















