8-3
Installation Mode
5975119 BARCODATA 3300 TCR
P
LUS
121098
-0,5
-0 ,2 5
0
0 ,2 5
0,5
1
2
3
4
5
6
80
1 2 0
16 0
2 00
2 40
4 0
30
1 5
-1,5
-1
-0,5
0
0,5
1
1,5
1
2
3
4
5
6
80
120
160
200
240
40
90
60
30
Screen Width
Horizontal Screen Shift
0
-15
-30
Maximum 5 mm right lens shift
Maximum 5 mm left lens shift
0
-30
-60
-90
Screen Width
Vertical Screen Shift
Maximum 15 mm downwards lens shift
Maximum 15 mm upwards lens shift
no shift
5 mm
10 mm
-5 mm
-10 mm
2.5 mm
no shift
-2.5 mm
Horizontal shift is function of the Screen Width for
various lens shifts.
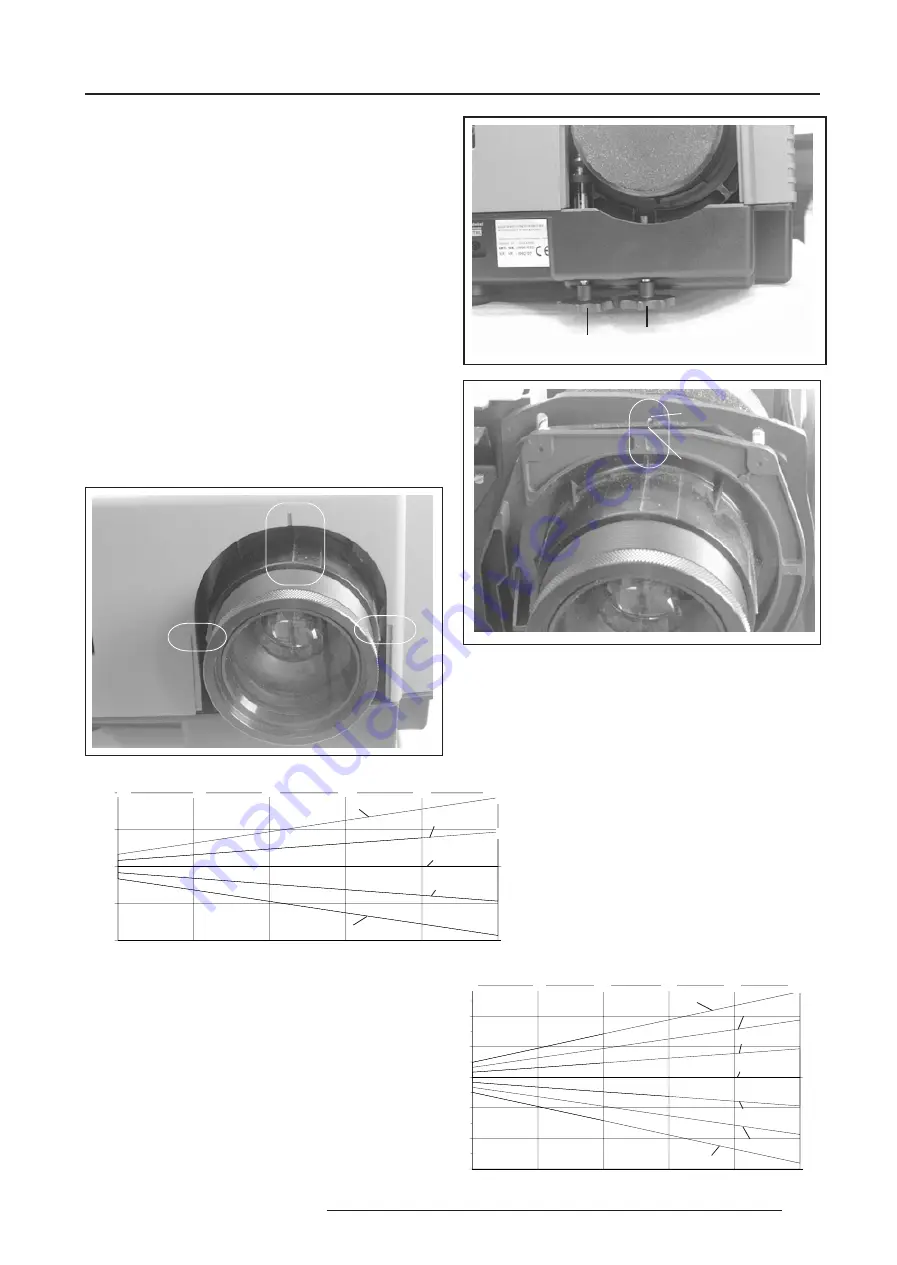
How to use the built-in adjustable lensholder.
With the lensholder, it is possible to shift the displayed image up or
down on the screen without moving the projector from its normal
position and without any geometric picture distortion. This built-in
feature is particularly useful for use in dual projector configurations
or for applications when the projector cannot be installed in its
standard position.
Features :
- Vertical and horizontal shift of the lens without removing the top
cover,
5 mm left and 5 mm right
15 mm up and 15 mm down.
- Makes it possible to project a geometric non-distorted image from
a non-standard position.
- The shift of the lensholder is not determined by the lens but depends
on the screen width.
- Can be used with all available lenses.
How to shift the image :
- First shift the image left or right until the correct horizontal position
is obtained. Turn clockwise to shift the image to the right, turn counter
clockwise to shift to the left. Use the hand screw.
Lens shift up/down
Lens shift left/right
hole
indication
line
Vertical shift is function of the Screen Width for various lens shifts.
Both the vertical and horizontal shift of the displayed picture can
be calculated as follows :
Screen shift = lens shift x 1 + (Screen width / LCD panel width)
LCD panel width for 3200 series : 0,06480 m (2.551 inch)




























